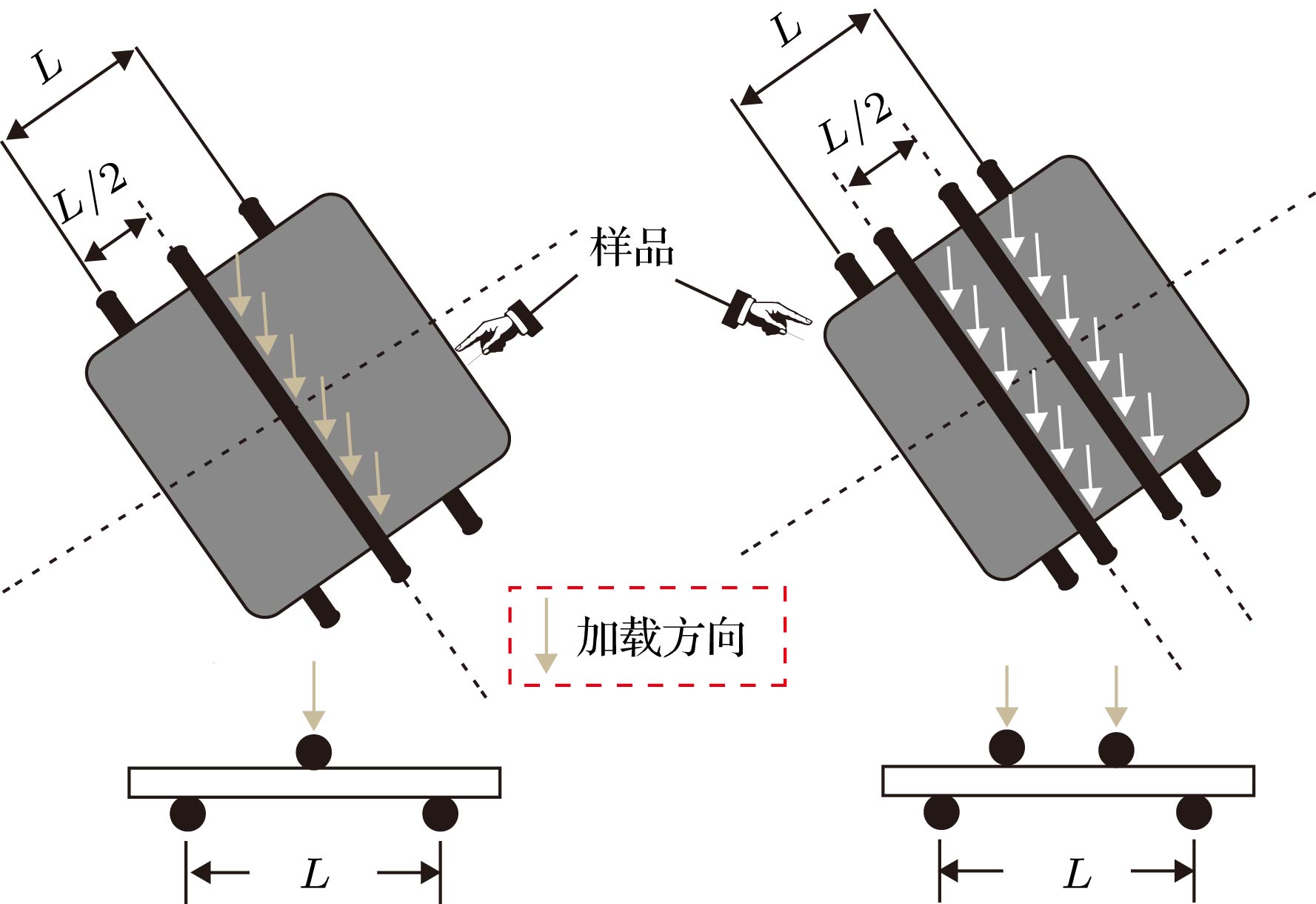
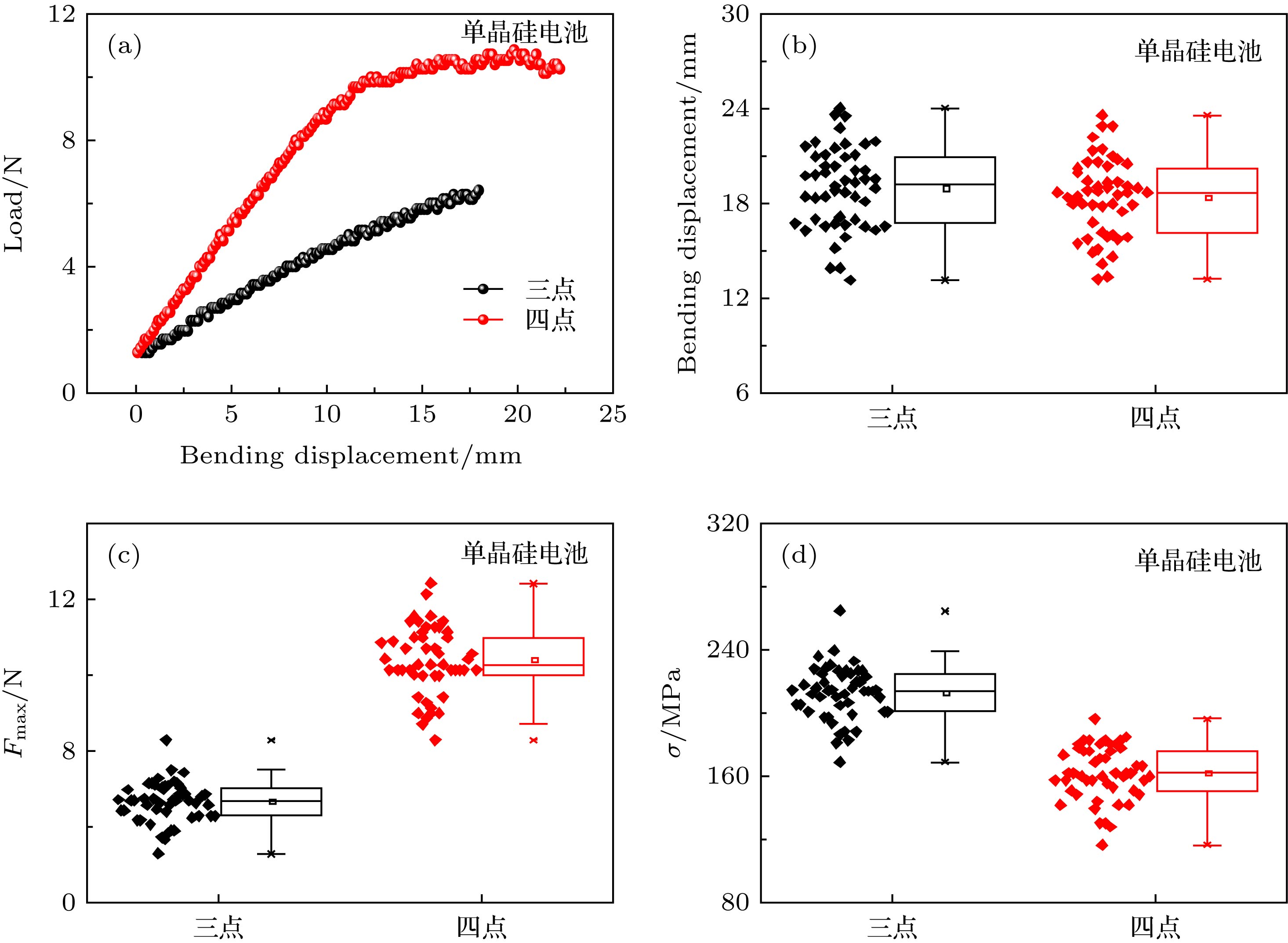
-
Silicon (Si)-wafer-based solar cells have dominated the global market with a share exceeding 90% due to their abundant source material and well-known physical and chemical properties. The brittleness of silicon material limits its further applications. It is necessary to investigate the material strength properties of Si wafer and/or Si solar cells, which can guide the fabrication process of Si solar cells to avoid breaking the Si wafers. The Si material strength properties have been extensively investigated by the methods of three-point bending test and four-point bending test. However, the difference between these two methods has not been studied so far. In this work, the mechanical strength properties of monocrystalline silicon (c-Si) wafer and bifacial c-Si solar cells are measured by three-point bending test and four-point bending test respectively. The average value of the maximum bending displacements has a little discrepancy between the results of the three-point bending test and four-point bending test methods. It is worth noting that the degree of dispersion of the Si wafer test results of the three-point bending test is larger than those of the four-point bending test. And the results of the dispersion of the Si bifacial solar cells, obtained from the two bending test methods, show no difference between them due to the existence of metalized electrodes. Whether the measured sample is Si wafer or Si solar cell, the average value of the maximum load, obtained from the four-point bending test, is higher than that from the three point-bending test method, and the average value of the fracture strength, obtained from the four-point bending test, is lower than that from the three-point bending test method. By establishing the models of different beams, the applied load gets dispersed through two bars of the four-point bending test method, whereas the applied load is directly applied to the sample through one bar of the three-point bending test method, which can explain the relatively large difference between these two test methods. -
Keywords:
- three point bending test /
- four point bending test /
- mechanical property /
- modeling
[1] Battaglia C, Cuevas A, de Wolf S 2016 Energ. Environ. Sci. 9 1552
Google Scholar
[2] Borrerolopez O, Vodenitcharova T, Hoffman M, Leo A J 2009 J. Am. Ceram. Soc. 92 2713
Google Scholar
[3] Paul I, Majeed B, Razeeb K M, Barton J 2006 Acta Mater. 54 3991
Google Scholar
[4] Funke C, Wolf S, Stoyan D 2009 J. Sol. Energy Eng. 131 011012
Google Scholar
[5] Schoenfelder S, Ebert M, Landesberger C, Bock K, Bagdahn J 2007 Microelectron. Reliab. 47 168
Google Scholar
[6] Sekhar H, Fukuda T, Tanahashi K 2018 Jpn. J. Appl. Phys. 57 08RB08
Google Scholar
[7] Li Z L, Wang L, Yang D R, Zhu X, Shi Y Z, Jiang W L 2011 Acta Energiae Solaris Sinica 32 225
[8] Wang P, Yu X G, Li Z L, Yang D R 2011 J. Cryst. Growth 318 230
Google Scholar
[9] Rengarajan K N, Radchenko I, Illya G, Handara V, Kunz M, Tamura N, Budiman A S 2016 8th International Conference on Materials for Advanced Technologies Singapore, 28 June–3 July, 2015 p76
[10] Heilbronn B, de Moro F, Jolivet E, Tupin E, Chau B, Varrot R, Drevet B, Bailly S, Rey D, Lignier H, Xi Y H, Mangelinck N, Reinhart G, Regula G 2015 Cryst. Res. Technol. 50 101
Google Scholar
[11] Barredo J, Parra V, Guerrero I, Fraile A, Hermanns L 2013 Prog. Photovoltaics 22 1204
[12] Woo J, Kim Y, Kim S, Jang J, Han H N, Choi K J, Kim I, Kim J 2017 Scripta Mater. 140 1
Google Scholar
[13] Oswald M, Loewenstein T, Schubert G, Schoenfelder S 2012 6th International Workshop on Crystalline Silicon Solar Cells Aixles-bains France, October 8–11, 2012
[14] Echizenya D, Sakamoto H, Sasaki K 2011 Procedia Engineering 10 1440
Google Scholar
[15] Haase F, Kasewieter J, Köntges M 2016 6th International Conference on Silicon Photovoltaics Chambery France, March 7–9, 2016 p554
[16] Popovich V A 2014 Microstructure and Mechanical Aspects of Multicrystalline Silicon Solar Cells (Netherlands: Delft University of Technology) p115
[17] Mansfield B R, Armstrong D E, Wilshaw P R, Murphy J D 2009 Solid State Phenomena 156–158 p55
[18] Cotterell B, Chen Z, Han J, Tan N 2003 J. Electron. Packaging 125 114
Google Scholar
[19] Sekhar H, Fukuda T, Tanahashi K, Shirasawa K, Takato H, Ohkubo K, Ono H, Sampei Y, Kobayashi T 2018 Jpn. J. Appl. Phys. 57 095501
Google Scholar
[20] Kaule F, Kohler B, Hirsch J, Schoenfeldera S, Lauscha D 2018 Sol. Energ. Mat. Sol. C. 185 511
Google Scholar
[21] Gou W X 2010 Mechanics of Materials (Beijing: Science Press) p152
-
图 1 双面电池结构示意图
Figure 1. The schematic diagram of the structure of bifacial solar cell.
图 2 测试参数示意图 (a) 三点弯曲; (b) 四点弯曲
Figure 2. The schematic of test parameter: (a) Three point bengding; (b) four point bengding.
图 3 硅片三点和四点弯曲测试数据对比 (a) 载荷与位移的变化曲线; (b) 最大弯曲位移; (c) 最大载荷; (d) 断裂强度
Figure 3. Parameter comparison of silicon wafer test of three point and four point bending test: (a) Force as function of the bending value; (b) maximum bending displacement; (c) maximum force; (d) fracture strength.
图 4 双面电池三点和四点弯曲测试数据对比 (a) 载荷与位移的变化曲线; (b) 最大弯曲位移; (c) 最大载荷; (d) 断裂强度
Figure 4. Parameter comparison of bifacial solar cells test of three point and four point bending test: (a) Force as function of the bending value; (b) maximum bending displacement; (c) maximum force; (d) fracture strength.
图 5 作用力和弯矩图 (a) 三点弯曲; (b) 四点弯曲
Figure 5. The model diagram of force and bending moment: (a) Three point bending test; (b) four point bending test.
表 1 三点弯曲和四点弯曲测试的比较
Table 1. Comparison of three point bending and four point bending tests.
单位 国家 样片 厚度 测试方法 研究内容 年份 参考文献 TNI-UCC 爱尔兰 单晶硅片 50—525 μm 三点弯曲 用统计方法建立了不同厚度单晶硅材料断裂强度的模型 2006 [3] Fraunhofer -IMM 德国 单晶硅片 48—200 μm 三点弯曲 分析了磨削、抛光、蚀刻工艺对薄硅试样机械强度的影响 2007 [5] RERC-FREI-AIST 日本 单晶硅片 200—250 μm 三点弯曲 金刚线切割时产生的应力损伤层对单晶硅片机械性能的影响 2008 [6] 浙江大学 中国 单晶硅电池 200 μm 三点弯曲 背电极花样对单晶硅电池的机械强度有明显影响 2011 [7] 浙江大学 中国 多晶硅片 220 μm 三点弯曲 铸锭多晶硅中, 锗掺杂能增强多晶硅片的机械强度 2011 [8] SUTD 新加坡 光伏组件 — 三点弯曲 封装材料对太阳能光伏组件的可靠性影响 2016 [9] Solarforce S.A. 法国 多晶硅片 60—140 μm 四点弯曲 研究了带状生长多晶硅的弯曲强度随不同工艺条件的变化 2015 [10] UNSW 澳大利亚 多晶硅片 200 μm 四点弯曲 硅片的边缘缺陷对多晶硅片断裂强度的影响 2009 [2] CMME 西班牙 多晶、单晶、类单晶 200 μm 四点弯曲 对相同厚度的多晶、单晶、类单晶的晶体硅片的机械强度进行了比较 2014 [11] IEP-TUBF 德国 多晶硅片 250—300 μm 四点弯曲 研究了损伤腐蚀对太阳能硅片力学性能的影响 2009 [4] UNIST 韩国 单晶硅片 50 μm 四点弯曲 同制绒工艺改变硅片表面形貌对晶体硅机械性能的影响 2017 [12] Fraunhofer -CSP 德国 多晶硅片 — 四点弯曲 激光钻孔对机械性能的影响 2012 [13] MEC 日本 多晶硅片 200—300 μm 四点弯曲 金刚线切割多晶硅片的弯曲强度, 并对不同强度值产生的原因进行了分析 2011 [14] ISFH 德国 光伏组件 — 四点弯曲 标准尺寸太阳能光伏组件在受压情况下的裂纹分布情况 2016 [15] 注: TNI-UCC, Tyndall National Institute, University College Cork (科克大学, 廷德尔国立研究所); Fraunhofer-IMM, Fraunhofer-Institute for Mechanics of Materials (弗劳恩霍夫材料力学研究所); RERC-FREI-AIST, Renewable Energy Research Center, Fukushima Renewable Energy Institute, National Institute of Advanced Industrial Science and Technology (国家先进工业科学技术研究所, 福岛可再生能源研究所, 可再生能源研究中心); SUTD, Singapore University of Technology and Design (新加坡科技设计大学) UNSW, University of New South Wales (新南威尔士大学); IEP-TUBF, Institute of Experimental Physics, TU Bergakademie Freiberg (弗莱贝格工业大学, 实验物理研究所); MEC, Mitsubishi Electric Corporation (三菱电力公司); Fraunhofer-CSP, Fraunhofer Center for Silicon Photovoltaics (弗劳恩霍夫硅光电中心); CMME, Centre for Modelling in Mechanical Engineering (机械工程建模中心); Solarforce S.A., 太阳力股份有限公司; ISFH, Institute for Solar Energy Research Hamelin (哈梅林太阳能研究所); UNIST, Ulsan National Institute of Science and Technology (蔚山国家科学技术研究院). 
DownLoad: CSV
-
[1] Battaglia C, Cuevas A, de Wolf S 2016 Energ. Environ. Sci. 9 1552
Google Scholar
[2] Borrerolopez O, Vodenitcharova T, Hoffman M, Leo A J 2009 J. Am. Ceram. Soc. 92 2713
Google Scholar
[3] Paul I, Majeed B, Razeeb K M, Barton J 2006 Acta Mater. 54 3991
Google Scholar
[4] Funke C, Wolf S, Stoyan D 2009 J. Sol. Energy Eng. 131 011012
Google Scholar
[5] Schoenfelder S, Ebert M, Landesberger C, Bock K, Bagdahn J 2007 Microelectron. Reliab. 47 168
Google Scholar
[6] Sekhar H, Fukuda T, Tanahashi K 2018 Jpn. J. Appl. Phys. 57 08RB08
Google Scholar
[7] Li Z L, Wang L, Yang D R, Zhu X, Shi Y Z, Jiang W L 2011 Acta Energiae Solaris Sinica 32 225
[8] Wang P, Yu X G, Li Z L, Yang D R 2011 J. Cryst. Growth 318 230
Google Scholar
[9] Rengarajan K N, Radchenko I, Illya G, Handara V, Kunz M, Tamura N, Budiman A S 2016 8th International Conference on Materials for Advanced Technologies Singapore, 28 June–3 July, 2015 p76
[10] Heilbronn B, de Moro F, Jolivet E, Tupin E, Chau B, Varrot R, Drevet B, Bailly S, Rey D, Lignier H, Xi Y H, Mangelinck N, Reinhart G, Regula G 2015 Cryst. Res. Technol. 50 101
Google Scholar
[11] Barredo J, Parra V, Guerrero I, Fraile A, Hermanns L 2013 Prog. Photovoltaics 22 1204
[12] Woo J, Kim Y, Kim S, Jang J, Han H N, Choi K J, Kim I, Kim J 2017 Scripta Mater. 140 1
Google Scholar
[13] Oswald M, Loewenstein T, Schubert G, Schoenfelder S 2012 6th International Workshop on Crystalline Silicon Solar Cells Aixles-bains France, October 8–11, 2012
[14] Echizenya D, Sakamoto H, Sasaki K 2011 Procedia Engineering 10 1440
Google Scholar
[15] Haase F, Kasewieter J, Köntges M 2016 6th International Conference on Silicon Photovoltaics Chambery France, March 7–9, 2016 p554
[16] Popovich V A 2014 Microstructure and Mechanical Aspects of Multicrystalline Silicon Solar Cells (Netherlands: Delft University of Technology) p115
[17] Mansfield B R, Armstrong D E, Wilshaw P R, Murphy J D 2009 Solid State Phenomena 156–158 p55
[18] Cotterell B, Chen Z, Han J, Tan N 2003 J. Electron. Packaging 125 114
Google Scholar
[19] Sekhar H, Fukuda T, Tanahashi K, Shirasawa K, Takato H, Ohkubo K, Ono H, Sampei Y, Kobayashi T 2018 Jpn. J. Appl. Phys. 57 095501
Google Scholar
[20] Kaule F, Kohler B, Hirsch J, Schoenfeldera S, Lauscha D 2018 Sol. Energ. Mat. Sol. C. 185 511
Google Scholar
[21] Gou W X 2010 Mechanics of Materials (Beijing: Science Press) p152
DownLoad:
Catalog
Metrics
- Abstract views: 14420
- PDF Downloads: 259
- Cited By: 0
