-
利用概率幅变分近似结合多重尺度法, 研究了探测光在两边产生点间隧道耦合的非对称阵列型三量子点电磁诱导透明介质的传播性质. 结果表明, 由于系统的色散效应和点间隧穿耦合产生的非线性效应相平衡, 系统能形成稳定传播的超低速时间光孤子. 有趣的是, 仅开启一边的点间隧穿耦合(即另一边关闭), 随着点间隧穿耦合强度的增加, 光孤子的速度呈现出先增大后减小的变化趋势, 但光孤子的幅度却一直增大. 两边两个点间隧穿耦合强度均开启后, 随着点间隧穿强度逐渐的增大, 光孤子的幅度随着点间隧穿强度的增大会出现逐渐减小, 直到出现一个拐点后才迅速增大; 而光孤子的速度相比较于单个隧穿强度的影响会明显降低, 且出现停滞的现象. 这些结果不但揭示出点间隧道耦合对三量子点电磁感应透明介质光孤子的动力学有着重要影响, 而且还预言在半导体量子点器件中可利用点间隧道耦合调节其光孤子传输的幅度.
Soliton, which can travel over long distance without attenuation or shape change due to the balance of the interplay between dispersion (or diffraction) and nonlinearity in nonlinear medium, becomes a good information carrier in quantum information processing and transmission. Up to now, the study on the optical solitons mainly focuses on ultra-cold atomic electromagnetic induction transparency (EIT) medium. This is mainly because ultra-cold atomic system can generate strong nonlinear effect under low light excitation. However, for the practical application, it is a big challenge to control accurately the optical soliton dynamics in the atomic EIT medium due to its low temperature (which approaches to absolute zero) and rarefaction. Fortunately, with the maturity of semiconductor quantum production technology, quantum dots have extensive application prospect in quantum information processing and transmission. So, in the paper, we study the optical soliton dynamics in a four-level asymmetric array-type three-quantum-dot EIT medium. Based on the current experimental results, we first propose a four-level asymmetric array-type three-quantum-dot EIT model. Subsequently, by using amplitude variable approach combined with multi-scale method, we study analytically the propagation of a probe pulse in this system. It is shown that when one (the another) inter-dot tunneling coupling is turned on (off), only a single transparency window appears in the center range of the probe field detuning. Only if two inter-dot tunneling couplings are turned on will two transparent windows be distributed on both sides of the central region of the probe field detuning. And the width of the single transparent window or the widths of two transparent windows become wider with the strength of the inter-dot tunneling coupling increasing. For the nonlinear case, by choosing appropriate parameters in the area of the transparency window, the stable propagation of soliton can be realized. Interestingly, we find that the strength of the inter-dot tunneling coupling has an important effect on the soliton dynamic behaviors. In the case that one (the another) inter-dot tunneling coupling is turned on (off), with the increase of strength of the inter-dot tunneling coupling, the velocity of the soliton exhibits a trend of first increasing and then decreasing, and the amplitude of the soliton presents a increasing trend for ever. For the case that two inter-dot tunneling couplings are turned on, with the strength of the two inter-dot tunneling coupling increasing, the velocity of the soliton presents a decreasing trend for ever, while the amplitude of the soliton exhibits a trend of first decreasing and then increasing. Thus, the amplitude modulation effect of optical soliton can be realized in semiconductor quantum dot devices. -
Keywords:
- electromagnetically induced transparency medium /
- inter-dot tunneling coupling /
- optical soliton dynamical behaviors
[1] Wang Y, Ding J W, Wang D L, et al. 2020 Chaos 30 123133
Google Scholar
[2] Song W W, Li Q Y, Li Z D, et al. 2010 Chin. Phys. B 19 070503
Google Scholar
[3] 张晓斐, 张培, 陈光平, 董彪, 谭仁兵, 张首刚 2015 64 060302
Google Scholar
Zhang X F, Zhang P, Chen G P, Dong B, Tan R B, Zhang S G 2015 Acta Phys. Sin. 64 060302
Google Scholar
[4] Zhang X F, Zhang P, He W Q, Lin X X 2011 Chin. Phys. B 20 020307
Google Scholar
[5] Li Z D, Guo Q Q, Guo Y, et al. 2021 Chin. Phys. B 30 107506
Google Scholar
[6] 李再东, 郭奇奇 2020 69 017501
Google Scholar
Li Z D, Guo Q Q 2020 Acta Phys. Sin. 69 017501
Google Scholar
[7] Guo H, Qiu X, Ma Y, et al. 2021 Chin. Phys. B 30 060310
Google Scholar
[8] Li Z D, Wang Y Y, He P B 2019 Chin. Phys. B 28 010504
Google Scholar
[9] 李吉, 刘伍明 2018 67 110302
Google Scholar
Li J, Liu W M 2018 Acta Phys. Sin. 67 110302
Google Scholar
[10] Xu D T, Chen Z M, Huang G X 2017 Opt. Express 25 19094
Google Scholar
[11] Hang C, Huang G X 2018 Phys. Rev. A 98 043840
Google Scholar
[12] Bai Z Y, Hang C, Huang G X 2013 Chin. Opt. Lett. 11 012701
Google Scholar
[13] Zhou S J, Wang D L, Dong Y Y, Bai Z Y, Ding J W 2022 Phys. Lett. A 448 128320
Google Scholar
[14] 邓瑞婕, 闫智辉, 贾晓军 2017 66 074201
Google Scholar
Deng R J, Yan Z H, Jia X J 2017 Acta. Phys. Sin. 66 074201
Google Scholar
[15] Liu J, Jing H, Ge M L 2004 Phys. Rev. A 70 55802
Google Scholar
[16] Si L G, Yang W X, Yang X X 2009 J. Opt. Soc. Am. B: Opt. Phys. 26 478
[17] Si L G, Yang W X, Lü X Y, Li J H, Yang X X 2009 Eur. Phys. J. D 55 161
Google Scholar
[18] Si L G, Yang W X, Liu J B, Li J H, Yang X X 2009 Opt. Express 17 7771
Google Scholar
[19] Wu Y 2005 Phys. Rev. A 71 053820
Google Scholar
[20] Wu Y, Deng L 2004 Phys. Rev. Lett. 93 143904
Google Scholar
[21] Wu Y, Deng L 2004 Opt. Lett. 29 2064
Google Scholar
[22] Dong Y Y, Wang D L, Wang Y, Ding J W 2018 Phys. Lett. A 382 2006
Google Scholar
[23] Chen Y, Chen Z M, Huang G X 2015 Phys. Rev. A 91 023820
Google Scholar
[24] Xu D T, Bai Z Y, Huang G X 2016 Phys. Rev. A 94 063857
Google Scholar
[25] Chen Y, Bai Z Y, Huang G X 2014 Phys. Rev. A 89 023835
Google Scholar
[26] Xu D T, Hang C, Huang G X 2018 Phys. Rev. A 98 043848
Google Scholar
[27] Chong S, Huang G X 2019 Phys. Rev. A 99 043821
Google Scholar
[28] Chen Z M, Bai Z Y, Li H J, Hang C, Huang G X 2015 Sci. Rep. 5 8211
Google Scholar
[29] Lodahl P, Mahmoodian S, Stobbe S 2015 Rev. Mod. Phys. 87 347
Google Scholar
[30] Liu H C, Gao M, Mccaffrey J, Wasilewski Z R, Fafard S 2001 Appl. Phys. Lett. 78 79
Google Scholar
[31] Santori C, Pelton M, Solomon G, Dale Y, Yamamoto Y 2001 Phys. Rev. Lett. 86 1502
Google Scholar
[32] Brus L E 1986 J. Phys. Chem. 90 2555
Google Scholar
[33] Marcinkevicius S, Gushterov A, Reithmaier J P 2008 Appl. Phys. Lett. 92 041113
Google Scholar
[34] Yang W X, Chen A X, Lee R K, Wu Y 2011 Phys. Rev. A 84 013835
Google Scholar
[35] 佘彦超, 张蔚曦, 王登龙 2011 60 064205
Google Scholar
She Y C, Zhang W X, Wang D L 2011 Acta. Phys. Sin. 60 064205
Google Scholar
[36] Zeng K H, Wang D L, She Y C, Luo X Q 2013 Eur. Phys. J. D 67 221
Google Scholar
[37] 曾宽宏, 王登龙, 佘彦超, 张蔚曦 2013 62 147801
Google Scholar
Zeng K H, Wang D L, She Y C, Zhang W X 2013 Acta. Phys. Sin. 62 147801
Google Scholar
[38] Xiang Z H, Huwer J, Szymanska J S, et al. 2020 Commun. Phys. 3 121
Google Scholar
[39] Childress L, Sørensen A S, Lukin M D 2004 Phys. Rev. A 69 042302
Google Scholar
[40] Ordonez G, Na K, Kim S 2006 Phys. Rev. A 73 022113
Google Scholar
[41] 唐宏, 王登龙, 张蔚曦, 丁建文, 肖思国 2017 66 034202
Google Scholar
Tang H, Wang D L, Zhang W X, Ding J W, Xiao S G 2017 Acta. Phys. Sin. 66 034202
Google Scholar
[42] 杨璇, 王胤, 王登龙, 丁建文 2020 69 174203
Google Scholar
Yang X, Wang Y, Wang D L, Ding J W 2020 Acta Phys. Sin. 69 174203
Google Scholar
[43] Michael S, Chow W W, Schneider H C 2013 Phys. Rev. B 88 125305
Google Scholar
[44] 王胤, 周驷杰, 陈桥, 邓永和 2023 72 084204
Google Scholar
Wang Y, Zhou S J, Chen Q, Deng Y H 2023 Acta. Phys. Sin. 72 084204
Google Scholar
[45] Wang Y, Zhou S J, Deng Y H, Chen Q 2023 Chin. Phys. B. 32 054203
Google Scholar
[46] She Y C, Zheng X J, Wang D L, et al. 2013 Opt. Express 21 17392
Google Scholar
[47] Wolters J, Buser G, Horsley A, Béguin L, Jöckel A, Jahn J P, Warburton R J, Treutlein P 2017 Phys. Rev. Lett. 119 060502
Google Scholar
[48] Waugh F R, Berry M J, Mar D J, Westervelt R M, Campman K L, Gossard A C 1995 Phys. Rev. Lett. 75 705
Google Scholar
[49] Krause B, Metzger T H, Rastelli A, Songmuang R, Kiravittaya S, Schmidt O G 2005 Phys. Rev. B 72 085339
Google Scholar
[50] Harris S E, Field J E, Imamoglu A. 1990 Phys. Rev. Lett. 64 1107
Google Scholar
[51] Tian S C, Wan R G, Tong C Z, Ning Y Q 2014 J. Opt. Soc. Am. B: Opt. Phys. 31 1436
Google Scholar
[52] Luo X Q, Li Z Z, Jing J, et al. 2018 Sci. Rep. 8 3107
Google Scholar
[53] Luo X Q, Li Z Z, Li T F, et al. 2018 Opt. Express 26 32585
Google Scholar
-
图 1 四能级非对称阵列型三量子点电磁感应透明介质能级结构示意图
Fig. 1. Energy level structure diagram of a four-level asymmetric array-type three quantum dots electromagnetically induced transparent medium.
图 2 关闭左点间隧穿耦合 ($ {\text{T}}{{\text{e}}_1} = 0 $) 仅开启右点间隧穿耦合情况下, 线性吸收系数$ {K}_{0{\mathrm{i}}} $随失谐$ {{{\varDelta }}}_{{\mathrm{p}}} $的变化情况. 其他参数: $ {\gamma }_{10}=3.3\;\text{μeV}$, $ {\gamma }_{20}={\gamma }_{30}={10}^{-4}{\gamma }_{10} $, $ -\hslash {\omega }_{12}= $$ \hslash {\omega }_{13}=10\;\;\text{μeV} $, $ {\kappa }_{01}=1976\;{{\mathrm{cm}}}^{-1}{\cdot}\text{μeV} $
Fig. 2. In the case that the left (right) inter-dot tunneling coupling is turned off (on), the linear absorption coefficient $ {K}_{0{\mathrm{i}}} $ as a function of the detuning $ {{{\varDelta }}}_{{\mathrm{p}}} $. Other parameters: $ {\gamma }_{10}=3.3\;\;\text{μeV}$, $ {\gamma }_{20}={\gamma }_{30}={10}^{-4}{\gamma }_{10} $, $ -\hslash {\omega }_{12}= \hslash {\omega }_{13}= $$ 10\;\;\text{μeV} $, and $ {\kappa }_{01}=1976\;{{\mathrm{cm}}}^{-1}{\cdot}\text{μeV} $.
图 3 关闭右点间隧穿耦合 (${\text{T}}{{\text{e}}_2} = 0$) 仅开启左点间隧穿耦合情况下, 线性吸收系数$ {K}_{0{\mathrm{i}}} $随失谐$ {{{\varDelta }}}_{{\mathrm{p}}} $的变化情况. 图中所使用的其他参数与图2一致
Fig. 3. In the case that the left (right) inter-dot tunneling coupling is turned on (off), the linear absorption coefficient $ {K}_{0{\mathrm{i}}} $ as a function of the detuning $ {{{\varDelta }}}_{{\mathrm{p}}} $. Other parameters used are the same as the Fig. 2.
图 4 左、右两边两个点间隧穿耦合均开启情况下, 线性吸收系数$ {K}_{0{\mathrm{i}}} $随失谐$ {{{\varDelta }}}_{{\mathrm{p}}} $的变化情况. 图中所使用的其他参数与图2一致
Fig. 4. Under both the left and right inter-dot tunneling coupling are turned on, the linear absorption coefficient $ {K}_{0{\mathrm{i}}} $ as a function of the detuning $ {{{\varDelta }}}_{{\mathrm{p}}} $. Other parameters used are the same as the Fig. 2.
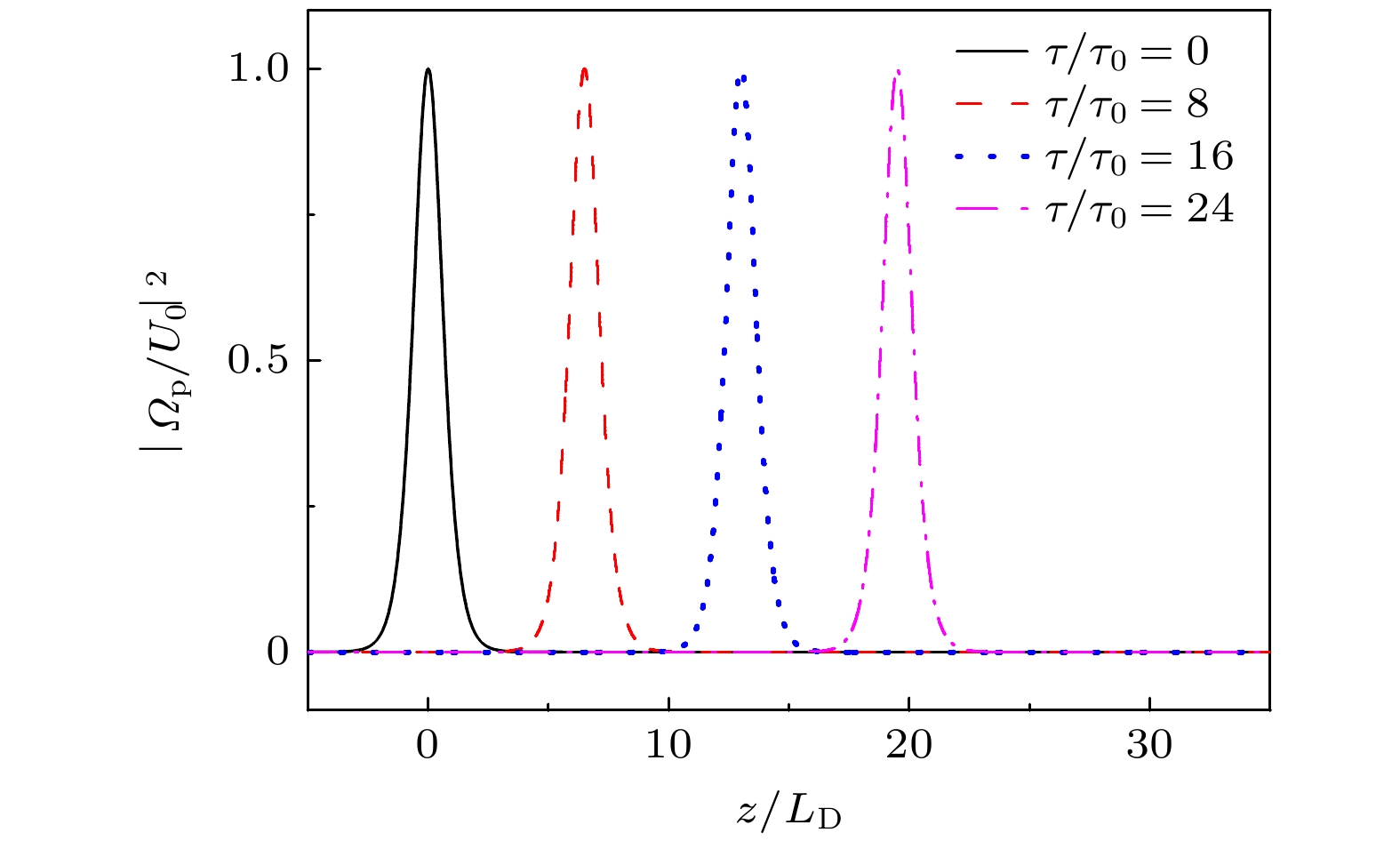
图 5 单点间隧穿耦合下三量子点EIT介质中光孤子的稳定性分析($ {\text{T}}{{\text{e}}_1} = 1\;{\text{meV}} $, $ {\text{T}}{{\text{e}}_2} = 0 $)
Fig. 5. Stability analysis of optical solitons in the three quantum dot EIT medium under the single inter-dot tunneling coupling effect ($ {\text{T}}{{\text{e}}_1} = 1\;{\text{meV}} $, $ {\text{T}}{{\text{e}}_2} = 0 $).
图 8 不同右点间隧穿强度下, 孤子的幅度随左点间隧穿强度Te1的变化
Fig. 8. Amplitude of the solitons as a function of the strength Te1 of the left inter-dot coupling with the different strength of the right inter-dot tunneling coupling.
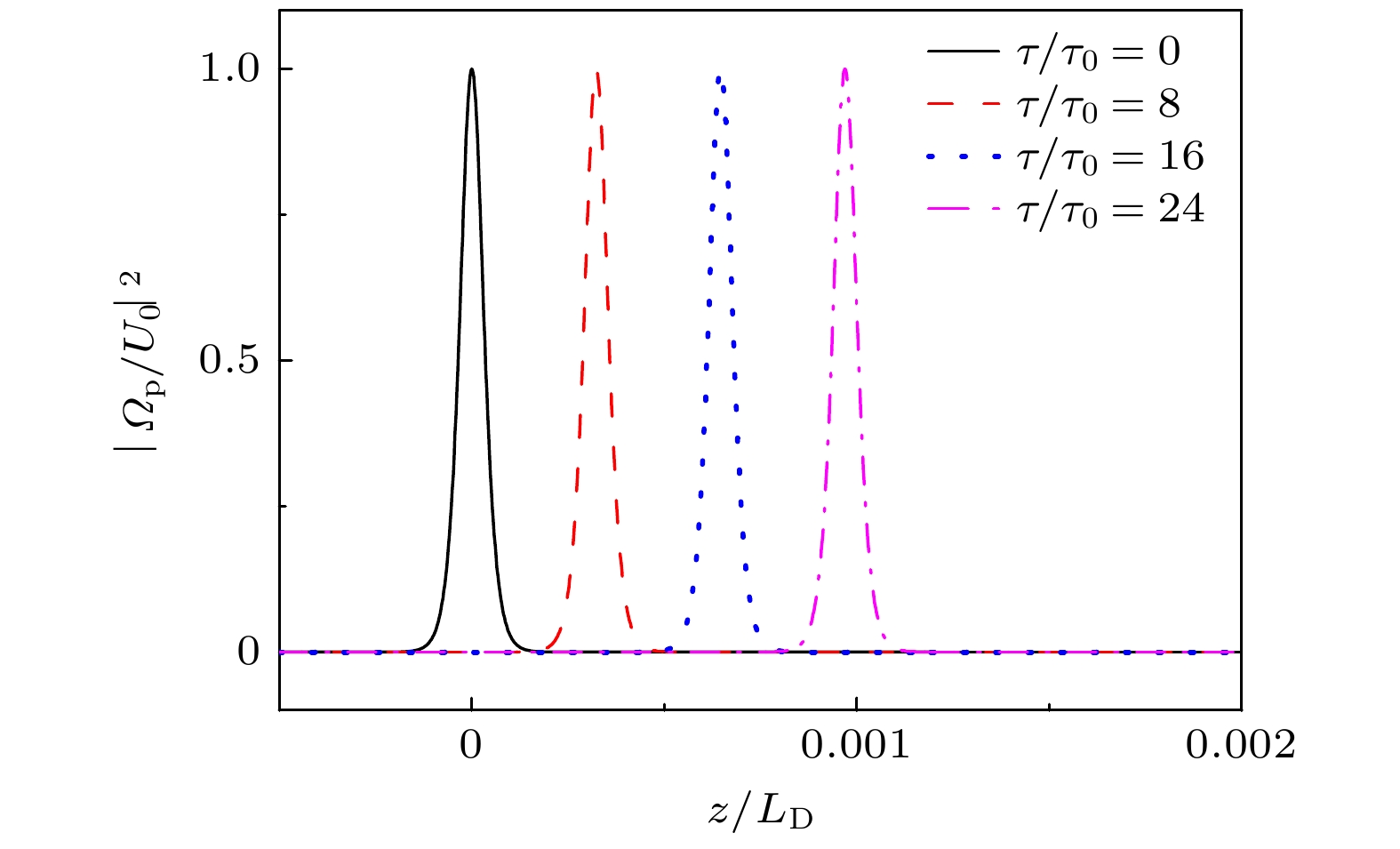
图 6 双点间隧穿耦合下三量子点EIT介质中光孤子的稳定性分析($ {\text{T}}{{\text{e}}_1} = 1\;{\text{meV}} $, $ {\text{T}}{{\text{e}}_2} = 0.5\;{\text{meV}} $)
Fig. 6. Stability analysis of optical solitons in three quantum dot EIT medium under the two single inter-dot tunneling coupling effect ($ {\mathrm{Te}}_1 = 1\;{\text{meV}} $, $ {\text{T}}{{\text{e}}_2} = 0.5\;{\text{meV}} $).
图 7 不同右点间隧穿强度下孤子的群速度随左点间隧穿强度Te1的变化
Fig. 7. Group velocity of the solitons as a function of the strength Te1 of the left inter-dot coupling with the different strength of the right inter-dot tunneling coupling.
-
[1] Wang Y, Ding J W, Wang D L, et al. 2020 Chaos 30 123133
Google Scholar
[2] Song W W, Li Q Y, Li Z D, et al. 2010 Chin. Phys. B 19 070503
Google Scholar
[3] 张晓斐, 张培, 陈光平, 董彪, 谭仁兵, 张首刚 2015 64 060302
Google Scholar
Zhang X F, Zhang P, Chen G P, Dong B, Tan R B, Zhang S G 2015 Acta Phys. Sin. 64 060302
Google Scholar
[4] Zhang X F, Zhang P, He W Q, Lin X X 2011 Chin. Phys. B 20 020307
Google Scholar
[5] Li Z D, Guo Q Q, Guo Y, et al. 2021 Chin. Phys. B 30 107506
Google Scholar
[6] 李再东, 郭奇奇 2020 69 017501
Google Scholar
Li Z D, Guo Q Q 2020 Acta Phys. Sin. 69 017501
Google Scholar
[7] Guo H, Qiu X, Ma Y, et al. 2021 Chin. Phys. B 30 060310
Google Scholar
[8] Li Z D, Wang Y Y, He P B 2019 Chin. Phys. B 28 010504
Google Scholar
[9] 李吉, 刘伍明 2018 67 110302
Google Scholar
Li J, Liu W M 2018 Acta Phys. Sin. 67 110302
Google Scholar
[10] Xu D T, Chen Z M, Huang G X 2017 Opt. Express 25 19094
Google Scholar
[11] Hang C, Huang G X 2018 Phys. Rev. A 98 043840
Google Scholar
[12] Bai Z Y, Hang C, Huang G X 2013 Chin. Opt. Lett. 11 012701
Google Scholar
[13] Zhou S J, Wang D L, Dong Y Y, Bai Z Y, Ding J W 2022 Phys. Lett. A 448 128320
Google Scholar
[14] 邓瑞婕, 闫智辉, 贾晓军 2017 66 074201
Google Scholar
Deng R J, Yan Z H, Jia X J 2017 Acta. Phys. Sin. 66 074201
Google Scholar
[15] Liu J, Jing H, Ge M L 2004 Phys. Rev. A 70 55802
Google Scholar
[16] Si L G, Yang W X, Yang X X 2009 J. Opt. Soc. Am. B: Opt. Phys. 26 478
[17] Si L G, Yang W X, Lü X Y, Li J H, Yang X X 2009 Eur. Phys. J. D 55 161
Google Scholar
[18] Si L G, Yang W X, Liu J B, Li J H, Yang X X 2009 Opt. Express 17 7771
Google Scholar
[19] Wu Y 2005 Phys. Rev. A 71 053820
Google Scholar
[20] Wu Y, Deng L 2004 Phys. Rev. Lett. 93 143904
Google Scholar
[21] Wu Y, Deng L 2004 Opt. Lett. 29 2064
Google Scholar
[22] Dong Y Y, Wang D L, Wang Y, Ding J W 2018 Phys. Lett. A 382 2006
Google Scholar
[23] Chen Y, Chen Z M, Huang G X 2015 Phys. Rev. A 91 023820
Google Scholar
[24] Xu D T, Bai Z Y, Huang G X 2016 Phys. Rev. A 94 063857
Google Scholar
[25] Chen Y, Bai Z Y, Huang G X 2014 Phys. Rev. A 89 023835
Google Scholar
[26] Xu D T, Hang C, Huang G X 2018 Phys. Rev. A 98 043848
Google Scholar
[27] Chong S, Huang G X 2019 Phys. Rev. A 99 043821
Google Scholar
[28] Chen Z M, Bai Z Y, Li H J, Hang C, Huang G X 2015 Sci. Rep. 5 8211
Google Scholar
[29] Lodahl P, Mahmoodian S, Stobbe S 2015 Rev. Mod. Phys. 87 347
Google Scholar
[30] Liu H C, Gao M, Mccaffrey J, Wasilewski Z R, Fafard S 2001 Appl. Phys. Lett. 78 79
Google Scholar
[31] Santori C, Pelton M, Solomon G, Dale Y, Yamamoto Y 2001 Phys. Rev. Lett. 86 1502
Google Scholar
[32] Brus L E 1986 J. Phys. Chem. 90 2555
Google Scholar
[33] Marcinkevicius S, Gushterov A, Reithmaier J P 2008 Appl. Phys. Lett. 92 041113
Google Scholar
[34] Yang W X, Chen A X, Lee R K, Wu Y 2011 Phys. Rev. A 84 013835
Google Scholar
[35] 佘彦超, 张蔚曦, 王登龙 2011 60 064205
Google Scholar
She Y C, Zhang W X, Wang D L 2011 Acta. Phys. Sin. 60 064205
Google Scholar
[36] Zeng K H, Wang D L, She Y C, Luo X Q 2013 Eur. Phys. J. D 67 221
Google Scholar
[37] 曾宽宏, 王登龙, 佘彦超, 张蔚曦 2013 62 147801
Google Scholar
Zeng K H, Wang D L, She Y C, Zhang W X 2013 Acta. Phys. Sin. 62 147801
Google Scholar
[38] Xiang Z H, Huwer J, Szymanska J S, et al. 2020 Commun. Phys. 3 121
Google Scholar
[39] Childress L, Sørensen A S, Lukin M D 2004 Phys. Rev. A 69 042302
Google Scholar
[40] Ordonez G, Na K, Kim S 2006 Phys. Rev. A 73 022113
Google Scholar
[41] 唐宏, 王登龙, 张蔚曦, 丁建文, 肖思国 2017 66 034202
Google Scholar
Tang H, Wang D L, Zhang W X, Ding J W, Xiao S G 2017 Acta. Phys. Sin. 66 034202
Google Scholar
[42] 杨璇, 王胤, 王登龙, 丁建文 2020 69 174203
Google Scholar
Yang X, Wang Y, Wang D L, Ding J W 2020 Acta Phys. Sin. 69 174203
Google Scholar
[43] Michael S, Chow W W, Schneider H C 2013 Phys. Rev. B 88 125305
Google Scholar
[44] 王胤, 周驷杰, 陈桥, 邓永和 2023 72 084204
Google Scholar
Wang Y, Zhou S J, Chen Q, Deng Y H 2023 Acta. Phys. Sin. 72 084204
Google Scholar
[45] Wang Y, Zhou S J, Deng Y H, Chen Q 2023 Chin. Phys. B. 32 054203
Google Scholar
[46] She Y C, Zheng X J, Wang D L, et al. 2013 Opt. Express 21 17392
Google Scholar
[47] Wolters J, Buser G, Horsley A, Béguin L, Jöckel A, Jahn J P, Warburton R J, Treutlein P 2017 Phys. Rev. Lett. 119 060502
Google Scholar
[48] Waugh F R, Berry M J, Mar D J, Westervelt R M, Campman K L, Gossard A C 1995 Phys. Rev. Lett. 75 705
Google Scholar
[49] Krause B, Metzger T H, Rastelli A, Songmuang R, Kiravittaya S, Schmidt O G 2005 Phys. Rev. B 72 085339
Google Scholar
[50] Harris S E, Field J E, Imamoglu A. 1990 Phys. Rev. Lett. 64 1107
Google Scholar
[51] Tian S C, Wan R G, Tong C Z, Ning Y Q 2014 J. Opt. Soc. Am. B: Opt. Phys. 31 1436
Google Scholar
[52] Luo X Q, Li Z Z, Jing J, et al. 2018 Sci. Rep. 8 3107
Google Scholar
[53] Luo X Q, Li Z Z, Li T F, et al. 2018 Opt. Express 26 32585
Google Scholar
下载:
计量
- 文章访问数: 1712
- PDF下载量: 34
- 被引次数: 0
