-
All-optical diodes and all-optical transistors are the basis of all-optical logic devices. We study the quantum statistical properties of all-optical diodes based on cavity quantum electrodynamics (QED)[
1 ], and discuss the squeezed properties of the output light after passing through the diode when coherent light and squeezed light are incident. Here we extend our research to all-optical transistor, and take all-optical transistor based on cavity optomechanical system as the research object. By changing the intensity of classical pump light, the all-optical transistor can effectively control the output of the probe light and realize optical amplification. We discuss the squeezed properties of the output light of the all-optical transistor with squeezed light and coherent light as the probe light. Our results show that when the probe light is coherent, the output light remains coherent no matter whether it works in the amplified region, and is not squeezed. When the input probe light is amplitude squeezed light, the output light is still squeezed light in the light amplification region of all-optical transistor, but the squeezed properties are modulated by the input light squeezed properties and system parameters. When the squeezed angle of the input probe squeezed light is 0°, the minimum squeezed parameter S1 of the output squeezed light decreases with the increase of the squeezed coefficient r of the input probe light, and the minimum value approaches to the squeezed limit of –0.25. But the change of squeezed angle of the input probe squeezed light changes has a great influence on the squeezed parameter S1,2 of the output light, and the squeezed properties will disappear. Only when the squeezed angle is an integer multiple of π, will the squeezed properties of the output light be best. This result has a potential application value in quantum measurement, weak signal detection, and other fields.-
Keywords:
- all-optical transisitor /
- squeeze light /
- cavity optomechanical system
[1] Li H Z, Xu J P, Wang D W, Xia X W, Yang Y P, Zhu S Y 2017 Phys. Rev. A 96 013832
Google Scholar
[2] Koppens F H L, Buizert C, Tielrooij K J, Vink I T, Nowack K C, Meunier T, ouwenhoven L P K, Vandersypen L M K 2006 Nature 442 766
Google Scholar
[3] Orrit M 2009 Nature 460 42
Google Scholar
[4] Chang D E, Sørensen A S, Demler E A, Lukin M D 2007 Nat. Phys. 3 807
Google Scholar
[5] Hwang J, Pototschnig M, Lettow R, Zumofen G, Renn A, Götzinger S, Sandoghdar V 2009 Nature 460 76
Google Scholar
[6] Li J J, Zhu K D 2011 Nanotechnology 22 055202
Google Scholar
[7] Tominaga J, Mihalcea C, Büchel D, Fukuda H, Nakano T, Atoda N 2001 Appl. Phys. Lett 78 2417
Google Scholar
[8] Hong F Y, Xiong S J 2008 Phys. Rev. A 78 013812
Google Scholar
[9] Hong F Y, Xiong S J 2008 Nanoscale. Res. Lett. 3 361
Google Scholar
[10] Dawes A M C 2009 Phys. Status. Solidi R 3 A17
Google Scholar
[11] Gröblacher S, Hammerer K, Vanner M R, Aspelmeyer M 2009 Nature 460 724
Google Scholar
[12] Genes C, Vitali D, Tombesi P, Gigan S, Aspelmeyer M 2008 Phys. Rev. A 77 033804
Google Scholar
[13] Wilson-Rae I, Nooshi N, Zwerger W, Kippenberg T J 2007 Phys. Rev. Lett. 99 093901
Google Scholar
[14] Agarwal G S, Huang S 2010 Phys. Rev. A 81 041803
Google Scholar
[15] Thompson J D, Zwickl B M, Jayich A M, Marquardt F, Girvin S M, Harris J G E 2008 Nature 452 72
Google Scholar
[16] Sankey J C, Yang C, Zwickl B M, Jayich A M, Harris J G E 2010 Nat. Phys. 6 707
Google Scholar
[17] Zhao Y, Wilson D J, Ni K K, Kimble H J 2012 Opt. Express 20 3586
Google Scholar
[18] Biancofiore C, Karuza M, Galassi M, Natali R, Tombesi P, Giuseppe G D, Vitali D 2011 Phys. Rev. A 84 033814
Google Scholar
[19] Weis S, Rivière R, Deléglise S, Gavartin E, Arcizet O, Schliesser A, Kippenberg T J 2010 Science 330 1520
Google Scholar
[20] Teufel J D, Li D, Allman M S, Cicak K, Sirois A J, Whittaker J D, Simmonds R W 2011 Nature 471 204
Google Scholar
[21] Safavi-Naeini A H, Alegre T P M, Chan J, Eichenfield M, Winger M, Lin Q, Hill J T, Chang D E, Painter O 2011 Nature 472 69
Google Scholar
[22] Nunnenkamp A, Børkje K, Girvin S M 2011 Phys. Rev. Lett. 107 063602
Google Scholar
[23] Marquardt F, Girvin S M 2009 Physics 2 40
Google Scholar
[24] Larson J, Horsdal M 2011 Phys. Rev. A 84 021804
Google Scholar
[25] Chen B, Jiang C, Li J J, Zhu K D 2011 Phys. Rev. A 84 055802
Google Scholar
[26] 彭堃墀 1991 物理 20 588
Google Scholar
Peng K C 1991 Physics 20 588
Google Scholar
[27] Brennecke F, Ritter S, Donner T, Esslinger T 2008 Science 322 235
Google Scholar
[28] Brennecke F, Donner T, Ritter S, Bourde T, Köh M, Esslinger T 2007 Nature 450 268
Google Scholar
[29] Masse F, Heikkilä T T, Pirkkalainen J M, Cho S U, Saloniemi H, Hakonen P J, Sillanpää M A 2011 Nature 480 351
Google Scholar
[30] 张岩, 于旭东, 邸克, 李卫, 张靖 2013 62 084204
Google Scholar
Zhang Y, Yu X D, Di K, Li W, Zhang J 2013 Acta Phys. Sin. 62 084204
Google Scholar
-
图 2 透射率
$ T={|{C}_{{{\rm o}{\rm u}{\rm t}}^{+}}/{E}_{{\rm p}{\rm r}}|}^{2}$ 在不同的抽运光功率下, 随探测光与腔模的频率失谐量$ {\varDelta }{'}_{{\rm p}{\rm r}{\rm c}}={\omega }_{{\rm p}{\rm r}}-{\omega }_{{\rm c}}{'}$ 的变化, 抽运光的频率满足蓝失谐条件$ {\varDelta }_{{\rm c}}=-{\omega }_{m}$ Figure 2. The relationship between the transmissivity
$ T={|{C}_{{{\rm o}{\rm u}{\rm t}}^{+}}/{E}_{{\rm p}{\rm r}}|}^{2}$ and the frequency detuning of the probe light and the cavity mode ($ {\varDelta }{'}_{{\rm p}{\rm r}{\rm c}}={\omega }_{{\rm p}{\rm r}}-{\omega }_{{\rm c}}{'}$ ) at different pump power. The frequency of the pump light satisfies the condition of blue detuning($ {\varDelta }_{{\rm c}}=-{\omega }_{m}$ ).
图 3 在输入的探测光为压缩光的情况下, 输出光的线性部分的压缩分量
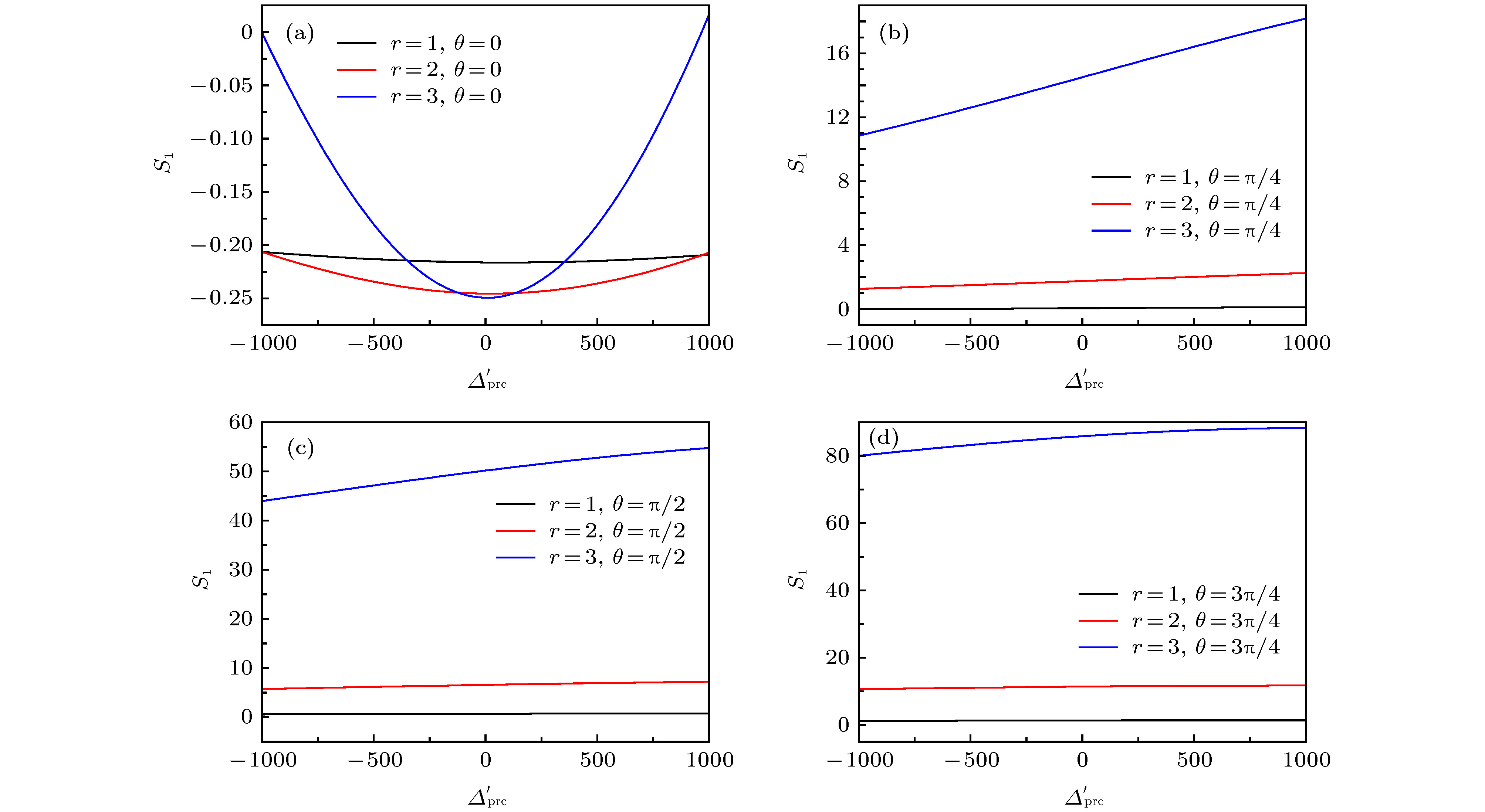
$ {S}_{1}$ 随有效探测-腔失谐量$ {\varDelta }{'}_{{\rm p}{\rm r}{\rm c}}={\omega }_{{\rm p}{\rm r}}-{\omega }_{{\rm c}}{'}$ 的变化, 探测压缩光的压缩角θ为(a) 0, (b) π/4, (c) π/2 (d) 3π/4Figure 3. When the input probe light is squeeze light, the squeeze component
$ {S}_{1}$ of the linear part of the output light varies with the effective detection-cavity detuning value ($ {\varDelta }{'}_{{\rm p}{\rm r}{\rm c}}={\omega }_{{\rm p}{\rm r}}-{\omega }_{{\rm c}}{'}$ ). The squeeze angle of the probe light is (a) 0, (b) π/4, (c) π/2 (d) 3π/4
图 4 输出光的线性部分的压缩量
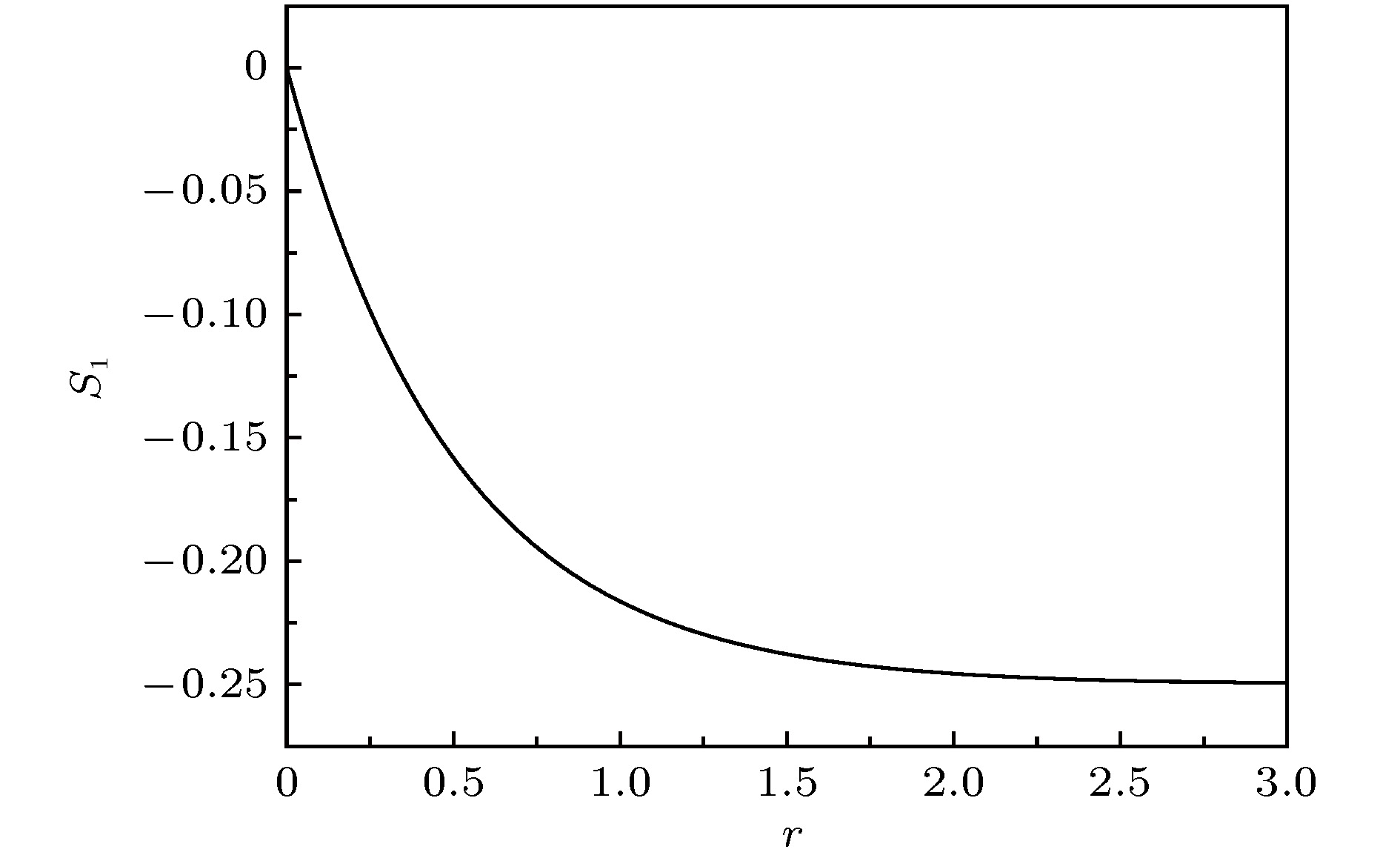
$ {S}_{1}$ 随入射探测光的压缩幅r的变化, 入射探测光的压缩角为θ = 0, 频率与腔模共振$ {\varDelta }{'}_{{\rm p}{\rm r}{\rm c}}=0$ Figure 4. The variation of the squeeze parameter S1 of the linear part of the output light with the squeeze amplitude r of the incident probe light, the squeeze angle of the incident probe light is θ = 0, and the frequency is resonant with the cavity mode
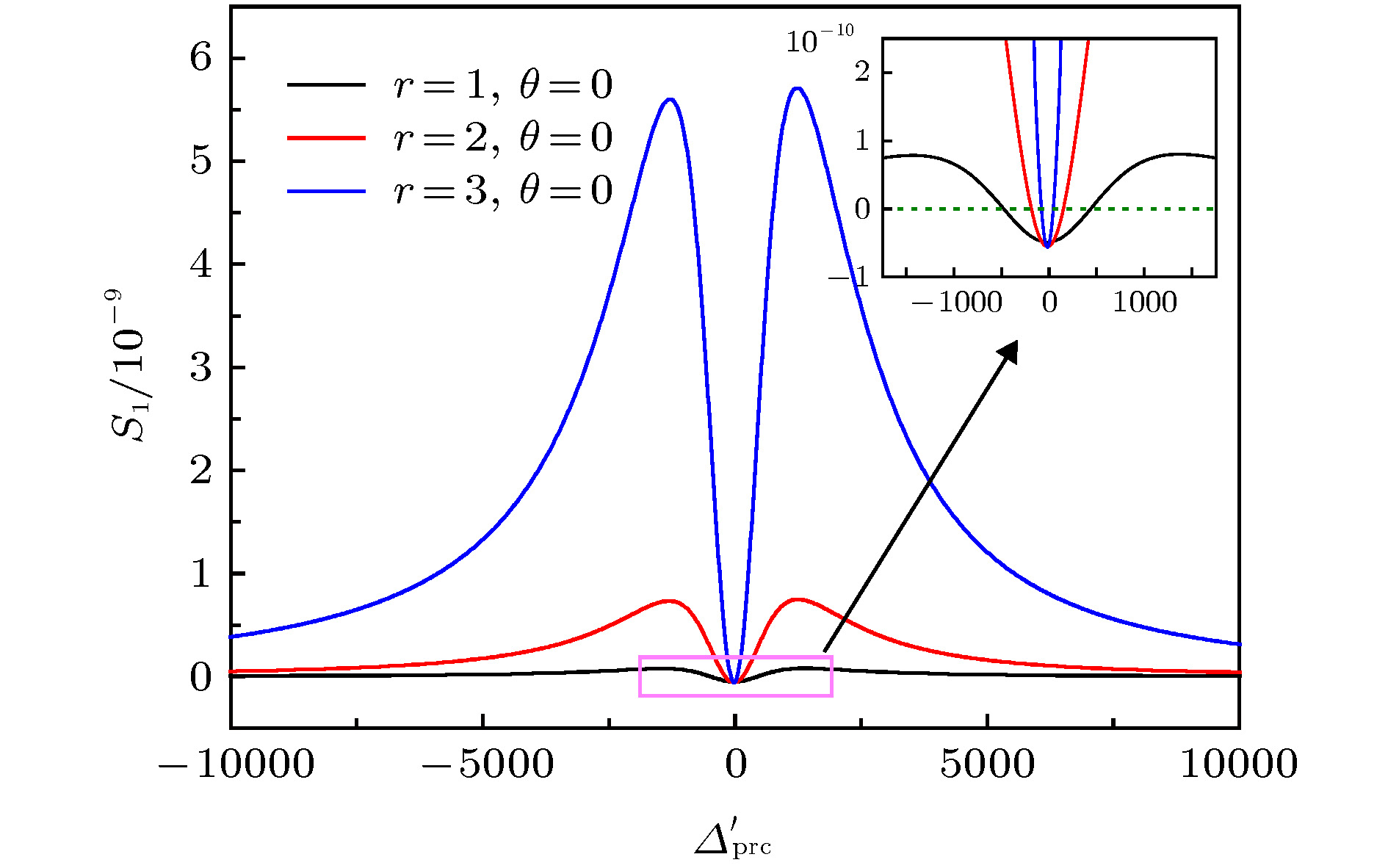
$ {\varDelta }{'}_{{\rm p}{\rm r}{\rm c}}=0$ .
图 5 在不同入射压缩光压缩幅的情况下, 输出光中的非线性部分的压缩量
$ {S}_{1}$ 随入射探测压缩光频率的变化.$ {\varDelta }{'}_{{\rm p}{\rm r}{\rm c}}={\omega }_{{\rm p}{\rm r}}-{\omega }_{{\rm c}}{'}$ 为探测光与腔模的频率失谐Figure 5. The squeeze parameter S1 of the non-linear part of the output light varies with the frequency of the incident probe squeeze light in the case of different squeeze amplitudes of the incident squeeze light,
$ \varDelta{'} _{\rm{prc}}={{\omega }_{\rm{pr}}}-\omega_{\rm{c}}{'}$ is the frequency detuning between the probe light and the cavity mode. -
[1] Li H Z, Xu J P, Wang D W, Xia X W, Yang Y P, Zhu S Y 2017 Phys. Rev. A 96 013832
Google Scholar
[2] Koppens F H L, Buizert C, Tielrooij K J, Vink I T, Nowack K C, Meunier T, ouwenhoven L P K, Vandersypen L M K 2006 Nature 442 766
Google Scholar
[3] Orrit M 2009 Nature 460 42
Google Scholar
[4] Chang D E, Sørensen A S, Demler E A, Lukin M D 2007 Nat. Phys. 3 807
Google Scholar
[5] Hwang J, Pototschnig M, Lettow R, Zumofen G, Renn A, Götzinger S, Sandoghdar V 2009 Nature 460 76
Google Scholar
[6] Li J J, Zhu K D 2011 Nanotechnology 22 055202
Google Scholar
[7] Tominaga J, Mihalcea C, Büchel D, Fukuda H, Nakano T, Atoda N 2001 Appl. Phys. Lett 78 2417
Google Scholar
[8] Hong F Y, Xiong S J 2008 Phys. Rev. A 78 013812
Google Scholar
[9] Hong F Y, Xiong S J 2008 Nanoscale. Res. Lett. 3 361
Google Scholar
[10] Dawes A M C 2009 Phys. Status. Solidi R 3 A17
Google Scholar
[11] Gröblacher S, Hammerer K, Vanner M R, Aspelmeyer M 2009 Nature 460 724
Google Scholar
[12] Genes C, Vitali D, Tombesi P, Gigan S, Aspelmeyer M 2008 Phys. Rev. A 77 033804
Google Scholar
[13] Wilson-Rae I, Nooshi N, Zwerger W, Kippenberg T J 2007 Phys. Rev. Lett. 99 093901
Google Scholar
[14] Agarwal G S, Huang S 2010 Phys. Rev. A 81 041803
Google Scholar
[15] Thompson J D, Zwickl B M, Jayich A M, Marquardt F, Girvin S M, Harris J G E 2008 Nature 452 72
Google Scholar
[16] Sankey J C, Yang C, Zwickl B M, Jayich A M, Harris J G E 2010 Nat. Phys. 6 707
Google Scholar
[17] Zhao Y, Wilson D J, Ni K K, Kimble H J 2012 Opt. Express 20 3586
Google Scholar
[18] Biancofiore C, Karuza M, Galassi M, Natali R, Tombesi P, Giuseppe G D, Vitali D 2011 Phys. Rev. A 84 033814
Google Scholar
[19] Weis S, Rivière R, Deléglise S, Gavartin E, Arcizet O, Schliesser A, Kippenberg T J 2010 Science 330 1520
Google Scholar
[20] Teufel J D, Li D, Allman M S, Cicak K, Sirois A J, Whittaker J D, Simmonds R W 2011 Nature 471 204
Google Scholar
[21] Safavi-Naeini A H, Alegre T P M, Chan J, Eichenfield M, Winger M, Lin Q, Hill J T, Chang D E, Painter O 2011 Nature 472 69
Google Scholar
[22] Nunnenkamp A, Børkje K, Girvin S M 2011 Phys. Rev. Lett. 107 063602
Google Scholar
[23] Marquardt F, Girvin S M 2009 Physics 2 40
Google Scholar
[24] Larson J, Horsdal M 2011 Phys. Rev. A 84 021804
Google Scholar
[25] Chen B, Jiang C, Li J J, Zhu K D 2011 Phys. Rev. A 84 055802
Google Scholar
[26] 彭堃墀 1991 物理 20 588
Google Scholar
Peng K C 1991 Physics 20 588
Google Scholar
[27] Brennecke F, Ritter S, Donner T, Esslinger T 2008 Science 322 235
Google Scholar
[28] Brennecke F, Donner T, Ritter S, Bourde T, Köh M, Esslinger T 2007 Nature 450 268
Google Scholar
[29] Masse F, Heikkilä T T, Pirkkalainen J M, Cho S U, Saloniemi H, Hakonen P J, Sillanpää M A 2011 Nature 480 351
Google Scholar
[30] 张岩, 于旭东, 邸克, 李卫, 张靖 2013 62 084204
Google Scholar
Zhang Y, Yu X D, Di K, Li W, Zhang J 2013 Acta Phys. Sin. 62 084204
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7072
- PDF Downloads: 75
- Cited By: 0
