-
本文提出了一种通过压缩驱动放置一个YIG小球的腔量子电动力学(QED)系统产生两体和三体纠缠的理论方案. 微波腔场与铁磁共振(FMR)模和静磁(MS)模的强耦合导致腔内光子、FMR模和MS模之间互相产生纠缠. 稳态情况下, 腔内光子、FMR模和MS模之间可以产生三体纠缠, 其三体纠缠的最小剩余共生纠缠度随非线性增益的增加而增大. 进一步研究发现, 该三体纠缠与MS模式的耗散系数有关, 最小剩余共生纠缠随MS模耗散系数的减小而增大. 同时还发现, 压缩驱动导致的三体纠缠对温度不敏感, 具有很好的鲁棒性. 结果表明磁-腔QED系统是研究宏观量子现象的一个强有力平台.Utilizing optical nonlinearity for generating the entanglement is still a most widely used approach due to its quality and simplicity. Here in this paper, we propose a theoretical scheme to generate bipartite and tripartite entanglement in a cavity quantum electrodynamics (QED) system with one Yttrium iron garnet (YIG) sphere by using a squeezed drive. In such a system, the parametric down-conversion process is used to generate the nonlinearity and further increase the coupling between cavity and YIG. Thus, the enhanced coupling between the microwave cavity photons and the ferromagnetic resonance (FMR) mode/magnetostatic (MS) mode results in bipartite entanglements. By using the mean field theory, we show that the bipartite entanglements strongly depend on the detuning of the cavity and magnon mode. When the driving field is tuned to be resonant with the FMR mode, but the MS mode is far off-resonant, the entanglement between photons and the FMR mode reaches its maximum. However, when the driving field is tuned to be resonant with the MS mode, but the FMR mode is detuned very well, the entanglement between photons and the MS mode reaches its maximum. We show that the dissipation of the FMR/MS mode affects the entanglement greatly, and the bipartite entanglement decreases as the dissipation rate of the FMR/MS mode increases. Under the steady-state approximation, we also show that the tripartite entanglement can be generated, and the minimum residual contangle increases with the enhancement of the nonlinear gain coefficient. With the nonlinearity induced by the parametric down conversion process, the interaction between the driving field and the magnetic-cavity QED system leads to the tripartite entanglement involving the cavity photons, FMR mode and the MS mode. Likewise, we show that the tripartite entanglement also strongly depends on the dissipation rate of MS mode, and the minimum residual contangle increases as the dissipation rate of the MS mode decreases. We also show that the squeezed field induced tripartite entanglement is insensitive to the temperature and has good robustness. Our results suggest that the magnetic-cavity QED system could provide a promising platform for studying the macroscopic quantum phenomena, and the squeezing field opens a new method of generating the entanglement.
-
Keywords:
- squeezed drive /
- logarithmic negativity /
- minimum residual contangle
[1] Forn-Díaz P, Lamata L, Rico E, Kono J, Solano E 2019 Rev. Mod. Phys. 91 025005
 Google Scholar
Google Scholar
[2] Reiserer A, Rempe G 2015 Rev. Mod. Phys. 87 1379
 Google Scholar
Google Scholar
[3] Hou K, Bao D Q, Zhu C J, Yang Y P 2019 Quantum. Inf. Process. 18 104
 Google Scholar
Google Scholar
[4] Hou K, Bao D Q, Zhu C J, Yang Y P 2019 Laser Phys. 29 015201
 Google Scholar
Google Scholar
[5] Han Y F, Zhu C J, Huang X S, Yang Y P 2018 Phys. Rev. A 98 033828
 Google Scholar
Google Scholar
[6] Lin J Z, Hou K, Zhu C J, Yang Y P 2019 Phys. Rev. A 99 053850
 Google Scholar
Google Scholar
[7] Zhang P F, Zhang Y C, Li G, Du J J, Zhang Y F, Guo Y Q, Wang J M, Zhang T C, Li W D 2011 Chin. Phys. Lett. 28 044203
 Google Scholar
Google Scholar
[8] Ashhab S 2013 Phys. Rev. A 87 013826
 Google Scholar
Google Scholar
[9] Han Y F, Zhu C J, Huang X S, Yang Y P 2018 Chin. Phys. B 27 124206
 Google Scholar
Google Scholar
[10] Ridolfo A, Leib M, Savasta S, Hartmann M J 2012 Phys. Rev. Lett. 109 193602
 Google Scholar
Google Scholar
[11] Zhu C J, Yang Y P, Agarwal G S 2017 Phys. Rev. A 95 063842
 Google Scholar
Google Scholar
[12] Hou K, Zhu C J, Yang Y P, Agarwal G S 2019 Phys. Rev. A 100 063817
 Google Scholar
Google Scholar
[13] Huebl H, Zollitsch C W, Lotze J, Hocke F, Greifenstein M, Marx A, Gross R, Goennenwein S T B 2013 Phys. Rev. Lett. 111 127003
 Google Scholar
Google Scholar
[14] Tabuchi Y, Ishino S, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2014 Phys. Rev. Lett. 113 083603
 Google Scholar
Google Scholar
[15] Goryachev M, Farr W G, Creedon D L, Fan Y, Kostylev M, Tobar M E 2014 Phys. Rev. Appl. 2 054002
 Google Scholar
Google Scholar
[16] Zhang X F, Zou C L, Jiang L, Tang H X 2014 Phys. Rev. Lett. 113 156401
 Google Scholar
Google Scholar
[17] Kittel C 1948 Phys. Rev. 73 155
 Google Scholar
Google Scholar
[18] Bai L H, Harder M, Chen Y P, Fan X, Xiao JQ, Hu C M 2015 Phys. Rev. Lett. 114 227201
 Google Scholar
Google Scholar
[19] Zhang D K, Wang X M, Li T F, Lou X Q, Wu W D, Nori F, You J Q 2015 Npj Quantum. Inf. 1 15014
 Google Scholar
Google Scholar
[20] Bourhill J, Kostylev N, Goryachev M, Creedon D L, Tobar M E 2016 Phys. Rev. B 93 144420
 Google Scholar
Google Scholar
[21] Kostylev N, Goryachev M, Tobar M E 2016 Appl. Phys. Lett. 108 062402
 Google Scholar
Google Scholar
[22] Wang Y P, Zhang G Q, Zhang D K, Liu T F, Hu C M, You J Q 2018 Phys. Rev. Lett. 120 057202
 Google Scholar
Google Scholar
[23] Bai L H, Harder M, Hyde P, Zhang Z H, Hu C M, Chen Y P, Xiao J Q 2017 Phys. Rev. Lett. 118 217201
 Google Scholar
Google Scholar
[24] Zhang X F, Zou C L, Zhu N, Marquardt F, Jiang L, Tang H X 2015 Nat. Commun. 6 8914
 Google Scholar
Google Scholar
[25] Wang B, Liu Z X, Kong C, Xiong H, Wu Y 2018 Opt. Express 26 20248
 Google Scholar
Google Scholar
[26] Kong C, Wang B, Liu Z X, Xiong H, Wu Y 2019 Opt. Express 27 5544
 Google Scholar
Google Scholar
[27] Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2015 Science 349 405
 Google Scholar
Google Scholar
[28] Li J, Zhu S Y, Agarwal G S 2018 Phys. Rev. Lett. 121 203601
 Google Scholar
Google Scholar
[29] Li J, Zhu S Y 2019 New J. Phys. 21 085001
 Google Scholar
Google Scholar
[30] Zhang Z D, Scully M O, Agarwal G S 2019 Phys. Rev. Res. 1 023021
 Google Scholar
Google Scholar
[31] Nair J M P, Agarwal G S 2020 Appl. Phys. Lett. 117 084001
[32] Zhu C J, Ping L L, Yang Y P, Agarwal G S 2020 Phys. Rev. Lett. 124 073602
 Google Scholar
Google Scholar
[33] Bao D Q, Zhu C J, Yang Y P, Agarwal G S 2019 Opt. Express 27 15540
 Google Scholar
Google Scholar
[34] Stancil D D, Prabhakar A 2009 Spin Waves Theory and Applications (New York: Springer) pp139−168
[35] Holstein T, Primakoff H 1940 Phys. Rev. 58 1098
 Google Scholar
Google Scholar
[36] Gardiner C W, Zoller P 2000 Quantum Noise (New York: Springer) pp164−179
[37] Vitali D, Gigan S, Ferreira A, Bohm H R, Tombesi P, Guerreiro A, Vedral V, Zeilinger A, Aspelmeyer M 2007 Phys. Rev. Lett. 98 030405
 Google Scholar
Google Scholar
[38] Parks P C, Hahn V 1993 Stability Theory (New York: Prentice Hall) pp57−71
[39] Vidal G, Werner R F 2002 Phys. Rev. A 65 032314
 Google Scholar
Google Scholar
[40] Plenio M B 2005 Phys. Rev. Lett. 95 090503
 Google Scholar
Google Scholar
[41] Adesso G, Illuminati F 2006 New J. Phys. 8 15
 Google Scholar
Google Scholar
[42] Adesso G, Illuminati F 2007 J. Phys. A: Math. Theor. 40 7821
 Google Scholar
Google Scholar
-
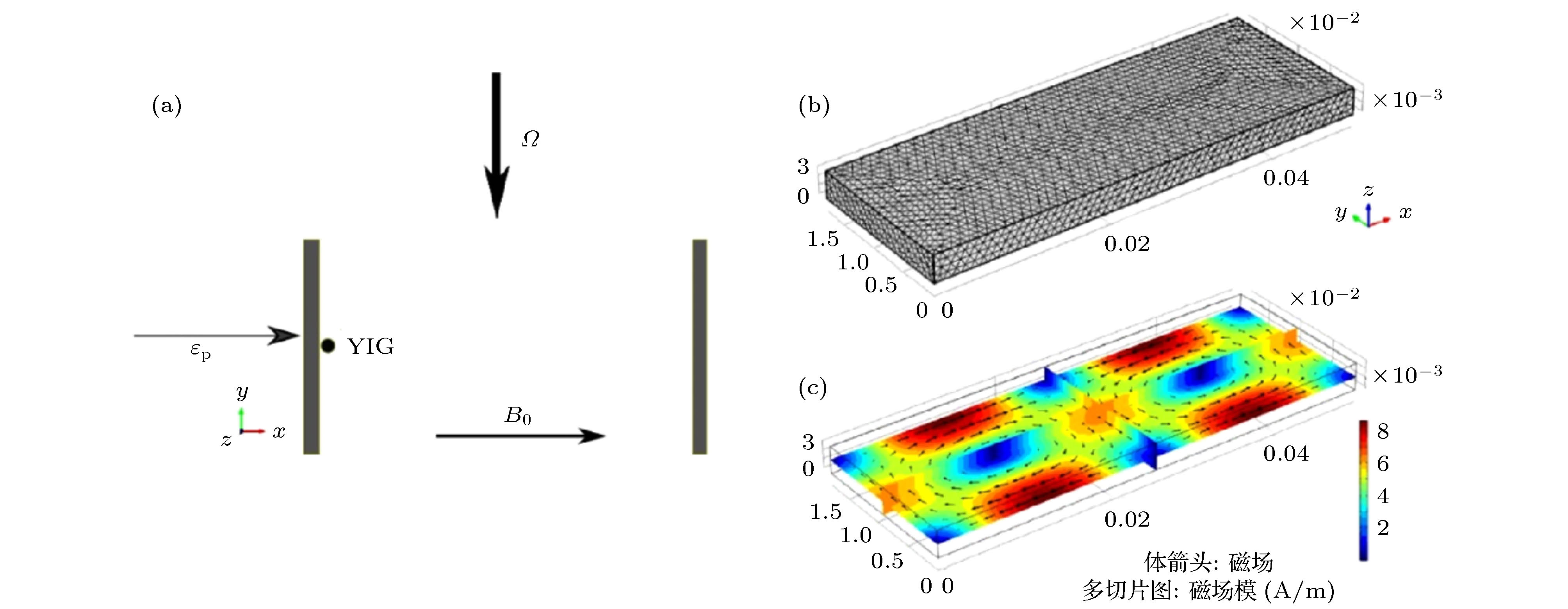
图 1 (a) 磁-腔QED系统示意图. YIG小球位于腔中短边中心处, 该处微波腔的TE102模式的磁场沿y轴方向, 静磁场沿x轴方向; (b) 利用Comsol模拟微波腔TE102的网格划分示意图; (c) 微波腔TE102模式的磁场方向和磁场强度
Fig. 1. (a) Schematic diagram of a magnetic-cavity QED system. A YIG sphere is located at the center of one short edge in the cavity, where magnetic field of microwave cavity mode TE102 is along y-axis direction, and static magnetic field is along x-axis direction; (b) schematic diagram of grid of microwave cavity mode TE102 by Comsol simulation; (c) magnetic field direction and magnetic field intensity of the microwave cavity mode TE102.
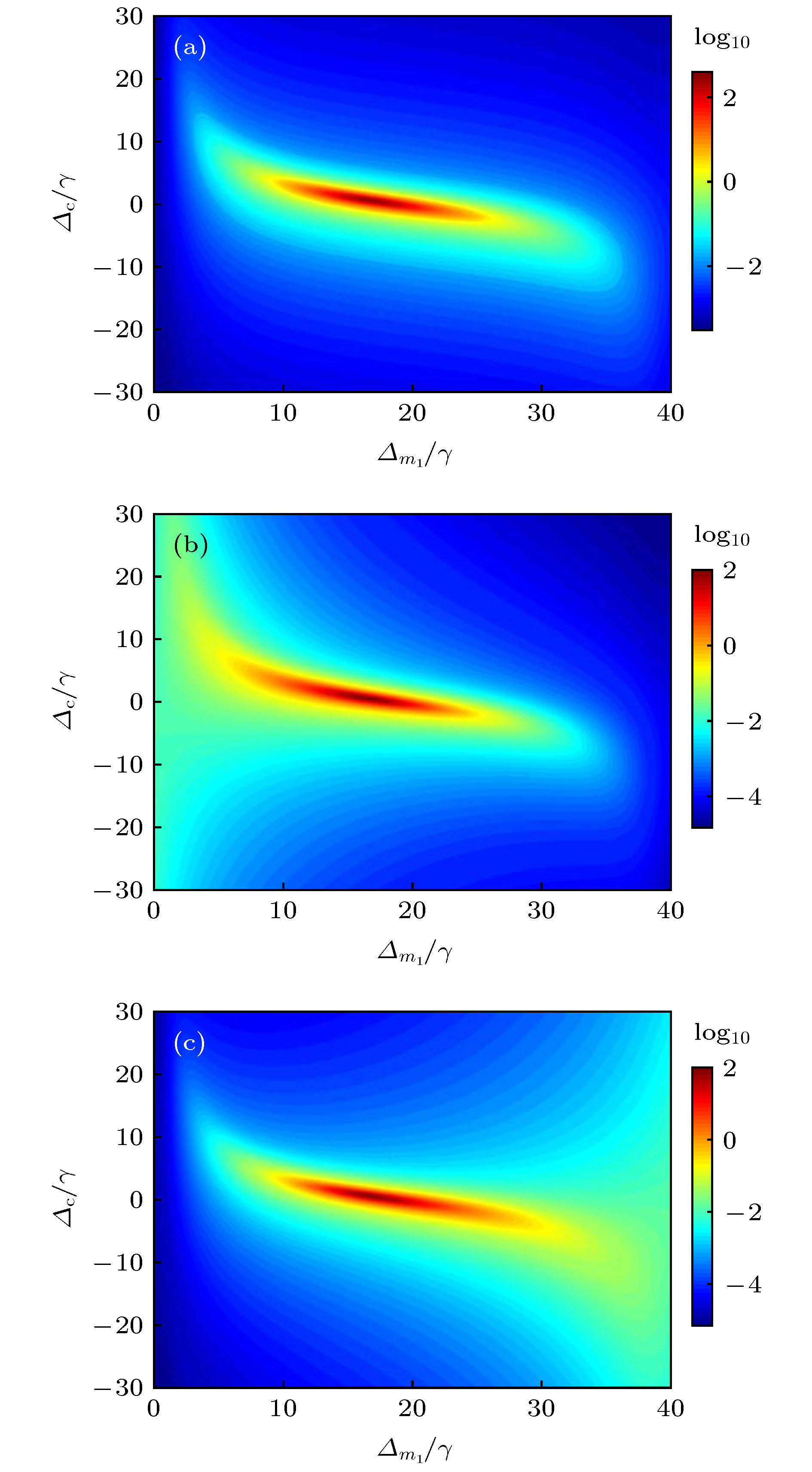
图 2 (a) 平均光子数、(b) FMR模的平均磁子数和 (c) MS模的平均磁子数随失谐量
${\varDelta _{{m_1}}}$ 和${\varDelta _{\rm{c}}}$ 的变化关系, 其中$\gamma /2{\rm{\pi = 1}}{\rm{.0\;MHz}}$ . 取${\varepsilon _{\rm{p}}} = \gamma $ ,$\varOmega = 1.5\gamma $ , 其他参数已在正文中给出Fig. 2. (a) Average photon number, (b) FMR mode average magnon number, and (c) MS mode average magnon number versus detunings
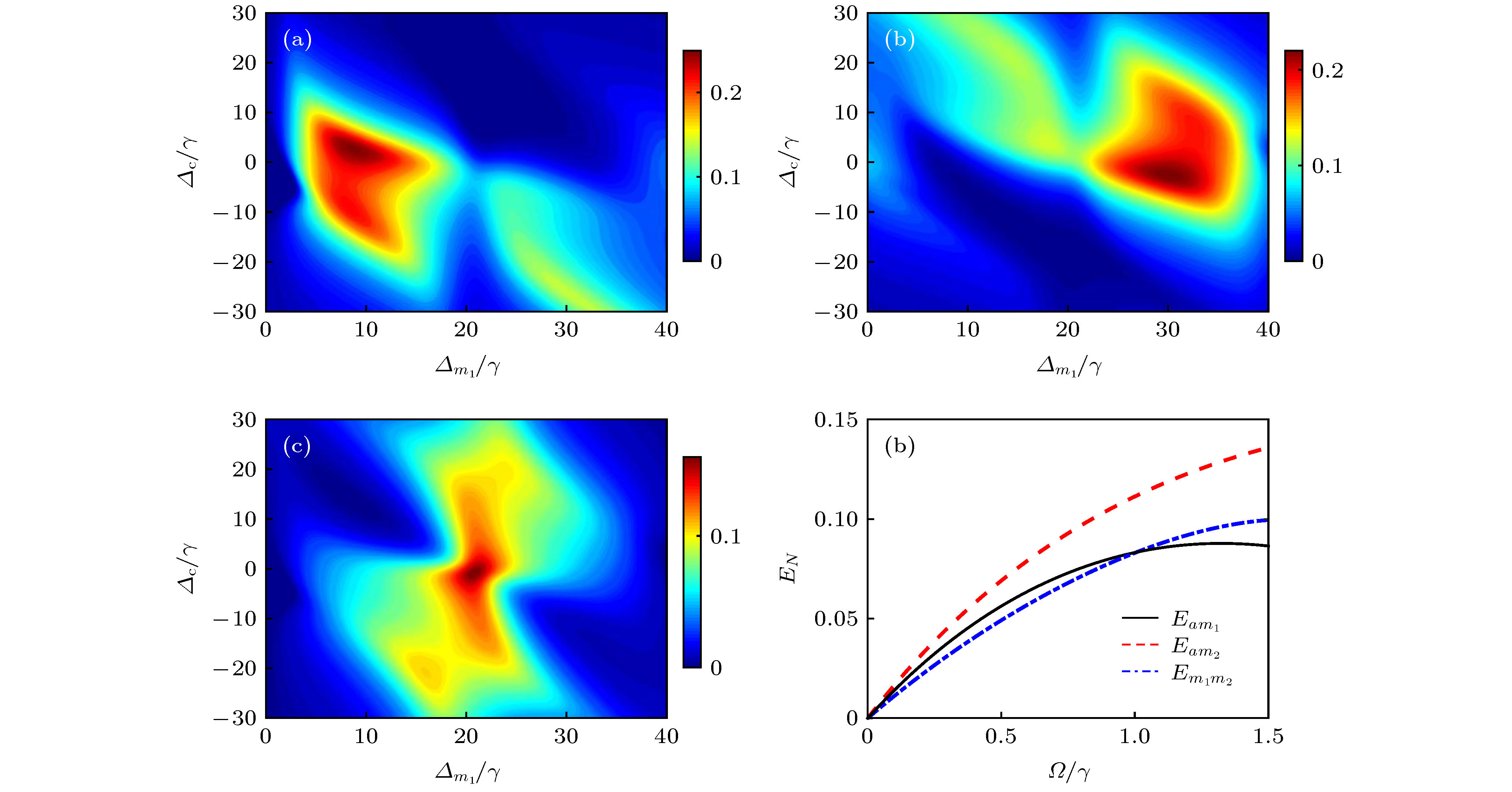
${\varDelta _{{m_1}}}$ and${\varDelta _{\rm{c}}}$ , where,$\gamma /2\rm{\pi} = 1{\rm{.0\;MHz}}$ . We take${\varepsilon _{\rm{p}}} = \gamma $ ,$\varOmega = 1.5\gamma $ . Other parameters are given in the text.图 3 两体纠缠的负值度 (a)
${E_{a{m_1}}}$ , (b)${E_{a{m_2}}}$ 和(c)${E_{{m_1}{m_2}}}$ 随失谐量${\varDelta _{{m_1}}}$ 和${\varDelta _{\rm{c}}}$ 的变化关系. 其中${\varepsilon _{\rm{p}}} = \gamma $ ,$\varOmega = 1.5\gamma $ . (d)负值度${E_{a{m_1}}}$ (实线)、${E_{a{m_2}}}$ (虚线)和${E_{{m_1}{m_2}}}$ (点划线)随非线性增益$\varOmega $ 的变化. 其中${\varepsilon _{\rm{p}}} = \gamma $ ,${\varDelta _{\rm{c}}} = - 2.5\gamma $ ,${\varDelta _{{m_1}}} = 23\gamma $ . 其他参数与图2一致Fig. 3. Density plot of logarithmic negativity related to bipartite entanglement (a)
${E_{a{m_1}}}$ , (b)${E_{a{m_2}}}$ , and (c)${E_{{m_1}{m_2}}}$ versus detunings${\varDelta _{{m_1}}}$ and${\varDelta _{\rm{c}}}$ , where${\varepsilon _{\rm{p}}} = \gamma $ ,$\varOmega = 1.5\gamma $ . (d) Logarithmic negativity${E_{a{m_1}}}$ (solid),${E_{a{m_2}}}$ (dashed), and${E_{{m_1}{m_2}}}$ (dot-dashed) versus the nonlinear gain coefficient$\varOmega $ , we take${\varepsilon _{\rm{p}}} = \gamma $ ,${\varDelta _{\rm{c}}} = - 2.5\gamma $ and${\varDelta _{{m_1}}} = 23\gamma $ . The other parameters are the same as in Fig.2.图 4 (a) 不同非线性增益情况下, 三体纠缠的最小剩余共生纠缠
$R_\tau ^{{\rm{min}}}$ 随失谐量${\varDelta _{{m_1}}}$ 的变化关系; (b) 最小剩余共生纠缠$R_\tau ^{{\rm{min}}}$ 随温度T的变化, 其中${\varepsilon _{\rm{p}}} = \gamma $ ,${\varDelta _{\rm{c}}} = - 2.5\gamma $ . 虚线、实线、点线和点划线分别对应非线性相互作用强度$\varOmega = 1.65\gamma $ ,$\varOmega = 1.5\gamma $ ,$\varOmega = 1.0\gamma $ 和$\varOmega = 0.5\gamma $ 的情况. 在图4(b)中, 取${\varDelta _{{m_1}}} = 23\gamma $ , 其他参数与图2一致Fig. 4. (a) Tripartite entanglement in terms of the minimum residual contangle
$R_\tau ^{{\rm{min}}}$ versus detuning${\varDelta _{{m_1}}}$ ; (b) robust against temperature of the minimum residual contangle$R_\tau ^{{\rm{min}}}$ , Where${\varepsilon _{\rm{p}}} = \gamma $ ,${\varDelta _{\rm{c}}} = - 2.5\gamma $ . The dashed line, solid line, dotted line, and dash-dot line indicate nonlinear interaction strength$\varOmega = 1.65\gamma $ ,$\varOmega = 1.5\gamma $ ,$\varOmega = 1.0\gamma $ , and$\varOmega = 0.5\gamma $ , respectively. At the same time, we take${\varDelta _{{m_1}}} = 23\gamma $ for Fig. 4 (b). The other parameters are the same as in Fig. 2.图 5 三体纠缠的最小剩余共生纠缠
$R_\tau ^{{\rm{min}}}$ 随失谐量${\varDelta _{{m_1}}}$ 和耗散系数${\kappa _2}$ 的变化关系; 设定${\varepsilon _{\rm{p}}} = \gamma $ ,$\varOmega = 1.5\gamma $ ,${\varDelta _{\rm{c}}} = - 2.5\gamma $ , 从左到右,${\kappa _2}$ 所取的数值分别为$1.7\gamma $ ,$2.1\gamma $ ,$2.5\gamma $ ,$2.9\gamma $ 以及$3.3\gamma $ . 其他参数与图2一致Fig. 5. Tripartite entanglement in terms of the minimum residual contangle
$R_\tau ^{{\rm{min}}}$ versus detuning${\varDelta _{{m_1}}}$ and dissipation rates${\kappa _2}$ , setting${\varepsilon _{\rm{p}}} = \gamma $ ,$\varOmega = 1.5\gamma $ and${\varDelta _{\rm{c}}} = - 2.5\gamma $ , the lines denote$1.7\gamma $ ,$2.1\gamma $ ,$2.5\gamma $ ,$2.9\gamma $ , and$3.3\gamma $ for${\kappa _2}$ from left to right. The other parameters are the same as in Fig. 2. -
[1] Forn-Díaz P, Lamata L, Rico E, Kono J, Solano E 2019 Rev. Mod. Phys. 91 025005
 Google Scholar
Google Scholar
[2] Reiserer A, Rempe G 2015 Rev. Mod. Phys. 87 1379
 Google Scholar
Google Scholar
[3] Hou K, Bao D Q, Zhu C J, Yang Y P 2019 Quantum. Inf. Process. 18 104
 Google Scholar
Google Scholar
[4] Hou K, Bao D Q, Zhu C J, Yang Y P 2019 Laser Phys. 29 015201
 Google Scholar
Google Scholar
[5] Han Y F, Zhu C J, Huang X S, Yang Y P 2018 Phys. Rev. A 98 033828
 Google Scholar
Google Scholar
[6] Lin J Z, Hou K, Zhu C J, Yang Y P 2019 Phys. Rev. A 99 053850
 Google Scholar
Google Scholar
[7] Zhang P F, Zhang Y C, Li G, Du J J, Zhang Y F, Guo Y Q, Wang J M, Zhang T C, Li W D 2011 Chin. Phys. Lett. 28 044203
 Google Scholar
Google Scholar
[8] Ashhab S 2013 Phys. Rev. A 87 013826
 Google Scholar
Google Scholar
[9] Han Y F, Zhu C J, Huang X S, Yang Y P 2018 Chin. Phys. B 27 124206
 Google Scholar
Google Scholar
[10] Ridolfo A, Leib M, Savasta S, Hartmann M J 2012 Phys. Rev. Lett. 109 193602
 Google Scholar
Google Scholar
[11] Zhu C J, Yang Y P, Agarwal G S 2017 Phys. Rev. A 95 063842
 Google Scholar
Google Scholar
[12] Hou K, Zhu C J, Yang Y P, Agarwal G S 2019 Phys. Rev. A 100 063817
 Google Scholar
Google Scholar
[13] Huebl H, Zollitsch C W, Lotze J, Hocke F, Greifenstein M, Marx A, Gross R, Goennenwein S T B 2013 Phys. Rev. Lett. 111 127003
 Google Scholar
Google Scholar
[14] Tabuchi Y, Ishino S, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2014 Phys. Rev. Lett. 113 083603
 Google Scholar
Google Scholar
[15] Goryachev M, Farr W G, Creedon D L, Fan Y, Kostylev M, Tobar M E 2014 Phys. Rev. Appl. 2 054002
 Google Scholar
Google Scholar
[16] Zhang X F, Zou C L, Jiang L, Tang H X 2014 Phys. Rev. Lett. 113 156401
 Google Scholar
Google Scholar
[17] Kittel C 1948 Phys. Rev. 73 155
 Google Scholar
Google Scholar
[18] Bai L H, Harder M, Chen Y P, Fan X, Xiao JQ, Hu C M 2015 Phys. Rev. Lett. 114 227201
 Google Scholar
Google Scholar
[19] Zhang D K, Wang X M, Li T F, Lou X Q, Wu W D, Nori F, You J Q 2015 Npj Quantum. Inf. 1 15014
 Google Scholar
Google Scholar
[20] Bourhill J, Kostylev N, Goryachev M, Creedon D L, Tobar M E 2016 Phys. Rev. B 93 144420
 Google Scholar
Google Scholar
[21] Kostylev N, Goryachev M, Tobar M E 2016 Appl. Phys. Lett. 108 062402
 Google Scholar
Google Scholar
[22] Wang Y P, Zhang G Q, Zhang D K, Liu T F, Hu C M, You J Q 2018 Phys. Rev. Lett. 120 057202
 Google Scholar
Google Scholar
[23] Bai L H, Harder M, Hyde P, Zhang Z H, Hu C M, Chen Y P, Xiao J Q 2017 Phys. Rev. Lett. 118 217201
 Google Scholar
Google Scholar
[24] Zhang X F, Zou C L, Zhu N, Marquardt F, Jiang L, Tang H X 2015 Nat. Commun. 6 8914
 Google Scholar
Google Scholar
[25] Wang B, Liu Z X, Kong C, Xiong H, Wu Y 2018 Opt. Express 26 20248
 Google Scholar
Google Scholar
[26] Kong C, Wang B, Liu Z X, Xiong H, Wu Y 2019 Opt. Express 27 5544
 Google Scholar
Google Scholar
[27] Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2015 Science 349 405
 Google Scholar
Google Scholar
[28] Li J, Zhu S Y, Agarwal G S 2018 Phys. Rev. Lett. 121 203601
 Google Scholar
Google Scholar
[29] Li J, Zhu S Y 2019 New J. Phys. 21 085001
 Google Scholar
Google Scholar
[30] Zhang Z D, Scully M O, Agarwal G S 2019 Phys. Rev. Res. 1 023021
 Google Scholar
Google Scholar
[31] Nair J M P, Agarwal G S 2020 Appl. Phys. Lett. 117 084001
[32] Zhu C J, Ping L L, Yang Y P, Agarwal G S 2020 Phys. Rev. Lett. 124 073602
 Google Scholar
Google Scholar
[33] Bao D Q, Zhu C J, Yang Y P, Agarwal G S 2019 Opt. Express 27 15540
 Google Scholar
Google Scholar
[34] Stancil D D, Prabhakar A 2009 Spin Waves Theory and Applications (New York: Springer) pp139−168
[35] Holstein T, Primakoff H 1940 Phys. Rev. 58 1098
 Google Scholar
Google Scholar
[36] Gardiner C W, Zoller P 2000 Quantum Noise (New York: Springer) pp164−179
[37] Vitali D, Gigan S, Ferreira A, Bohm H R, Tombesi P, Guerreiro A, Vedral V, Zeilinger A, Aspelmeyer M 2007 Phys. Rev. Lett. 98 030405
 Google Scholar
Google Scholar
[38] Parks P C, Hahn V 1993 Stability Theory (New York: Prentice Hall) pp57−71
[39] Vidal G, Werner R F 2002 Phys. Rev. A 65 032314
 Google Scholar
Google Scholar
[40] Plenio M B 2005 Phys. Rev. Lett. 95 090503
 Google Scholar
Google Scholar
[41] Adesso G, Illuminati F 2006 New J. Phys. 8 15
 Google Scholar
Google Scholar
[42] Adesso G, Illuminati F 2007 J. Phys. A: Math. Theor. 40 7821
 Google Scholar
Google Scholar
计量
- 文章访问数: 8338
- PDF下载量: 175
- 被引次数: 0













 下载:
下载: