-
In 2011, Kumar et al. (2011 Phys. Rev. A 84 043402) studied the light force acting on a beam of neutral two-level atoms superimposed on a few-cycle-pulse Gaussian laser field under both resonant and off-resonant conditions by solving the optical Bloch equation beyond the rotating-wave approximation, and they found that under resonant condition the transverse component of the light force shows oscillatory behavior but vanishes when a time average is taken, and the time averaged longitudinal force is nonzero only when the Rabi frequency is smaller than the resonant frequency and vanishes when the Rabi frequency is equal to or larger than the resonant frequency. In this paper, we investigate further the strong nonlinear optical interaction between a two-level atomic system and a femtosecond Gaussian laser pulse by solving numerically the full-wave optical Bloch equations through using the predictor-corrector method. It is found that the light forces and the light potentials are sensitive to the value of the Rabi frequency and the detuning of the laser field. Under the resonant condition, the instant light forces induced by the femtosecond laser pulse change their signs as a function of time. The instant longitudinal light force changes its sign at twice the Rabi frequency, while the instant transverse light force changes its sign at twice the light carrier-wave frequency. However, none of the time-averaged light forces is zero, showing periodical oscillation characters as a function of Rabi frequency. Both of the time-averaged longitudinal and transverse light forces oscillate at the Rabi frequency corresponding to the pulse area of 2 ${\text{π}}$ . The time-averaged transverse light force shows also a trend of enhancement with Rabi frequency increasing, and the time-averaged longitudinal light force shows also a saturation trend with the increase of the Rabi frequency. The optical potential depends strongly on the detuning. It changes gradually from repulsive potential to attractive potential when the detuning defined here changes from negative to positive detuning. When the field is nearly resonant, the optical potential then oscillates between repulsive and attractive potentials. Therefore, neutral atoms can be focused, defocused, trapped, splitted or steered by the femtosecond laser field with appropriate detuning and Rabi frequency.[1] Meystre P 2001 Atom Optics (New York: Springer) p1
[2] 印建平 2012 原子光学—基本概念、原理、技术及其应用 (上海: 上海交通大学出版社) p12
Yin J P 2012 Atomic Optics: Basic Concepts, Principles, Techniques and Applications (Shanghai: Shanghai Jiao Tong University Press) p12 (in Chinese)
[3] Ashkin A 1970 Phys. Rev. Lett. 24 156
Google Scholar
[4] Hänsch T W, Schawlow A L 1975 Opt. Commun. 13 68
Google Scholar
[5] Chu S, Hollberg L, Bjorkholm J E, Cable A, Ashkin A 1985 Phys. Rev. Lett. 55 48
Google Scholar
[6] Anderson M H, Ensher J R, Matthews M R, Wieman C E, Cornell E A 1995 Science 269 198
Google Scholar
[7] Davis K B, Mewes M O, Andrews M R, van Druten N J, Durfee D S, Kurn D M, Ketterle W 1995 Phys. Rev. Lett. 75 3969
Google Scholar
[8] Cheuk L W, Nichols M A, Okan M, Gersdorf T, Ramasesh V V, Bakr W S, Lompe T, Zwierlein M W 2015 Phys. Rev. Lett. 114 193001
Google Scholar
[9] Parsons M F, Huber F, Mazurenko A, Chiu C S, Setiawan W, Wooley-Brown K, Blatt S, Greiner M 2015 Phys. Rev. Lett. 114 213002
Google Scholar
[10] Haller E, Hudson J, Kelly A, Cotta D A, Peaudecerf B, Bruce G D, Kuhr S 2015 Nat. Phys. 11 738
Google Scholar
[11] 王义遒 2007 原子的激光冷却与陷俘 (北京: 北京大学出版社) p101
Wang Y Q 2007 Laser Cooling and Trapping of Atoms (Beijing: Beijing University Press) p101 (in Chinese)
[12] Metcalf H 2017 Rev. Mod. Phys. 89 041001
Google Scholar
[13] van der Straten P, Metcalf H 2016 Atoms and Molecules Interacting with Light (Cambridge: Cambridge University Press) p1
[14] Jiang Y, Narushima T, Okamoto H 2010 Nat. Phys. 6 1005
Google Scholar
[15] Garbin V, Cojoc D, Ferrari E, Proietti R Z, Cabrini S, Fabrizio E D 2005 Jpn. J. Appl. Phys. 44 5773
Google Scholar
[16] Eichmann U, Nubbemeyer T, Rottke H, Sandner W 2009 Nature 461 1261
Google Scholar
[17] Kumar P, Sarma A K 2012 Phys. Rev. A 86 053414
Google Scholar
[18] Kumar P, Sarma A K 2014 Phys. Rev. A 89 033422
Google Scholar
[19] Cai X, Lin Q 2013 Eur. Phys. J. D 67 246
Google Scholar
[20] Allen L, Eberly J H 1987 Optical Resonance and Two-Level Atoms (New York: Dover Publications, Inc) p41
[21] 张琴, 金康, 唐远河, 屈光辉 2011 60 053204
Zhang Q, Jin K, Tang Y H, Qu G H 2011 Acta Phys. Sin. 60 053204 (in Chinese)
[22] Wang Z L, Yin J P 2008 Chin. Phys. B 17 2466
Google Scholar
[23] Xing J, Chen X, Zhu S, Zhang R 2003 Chin. Opt. Lett. 1 122
[24] Kumar P, Sarma A K 2011 Phys. Rev. A 84 043402
Google Scholar
[25] Lembessis V E, Ellinas D 2005 J. Opt. B: Quant. Sem. Opt. 7 319
[26] Liu B, Jin G, Sun R, He J, Wang J 2017 Opt. Express 25 15861
Google Scholar
[27] Han Y C 2017 J. Phys. B: At. Mol. Opt. Phys. 50 225401
Google Scholar
[28] Liu J C, Wang C K, Gel’mukhanov F 2007 Phys. Rev. A 76 043422
Google Scholar
[29] Cai X, Zheng J, Lin Q 2013 Phys. Rev. A 87 043401
Google Scholar
[30] Boyd R W 2010 Nonlinear Optics (Singapore: Elsevier Pte Ltd) p158
[31] Liu J C, Guo F F, Zhao Y N, Li X Z 2018 Chin. Phys. B 27 104209
Google Scholar
[32] Liu J C, Zhang Y Q, Chen L 2014 J. Mod. Opt. 61 781
Google Scholar
[33] 刘纪彩, 赵珂, 宋玉志, 王传奎 2006 55 1803
Google Scholar
Liu J C, Zhao K, Song Y Z, Wang C K 2006 Acta Phys. Sin. 55 1803
Google Scholar
[34] Liu J C, Sun Y P, Wang C K, Ågren H, Gel’mukhanov F 2010 Phys. Rev. A 81 043412
Google Scholar
[35] Sun Y P, Liu J C, Wang C K, Ge’lmukhanov F 2010 Phys. Rev. A 81 013812
Google Scholar
[36] Butt H J, Cappella B, Kappl M 2005 Surf. Sci. Rep. 59 1
[37] Sukhov S V 2018 J. Commun. Technol. Electr. 63 1137
[38] Florin E L, Pralle A, Hörber J K, Stelzer E H K 1997 J. Stru. Bio. 119 202
Google Scholar
[39] Munday J N, Capasso F, Parsegian V A 2009 Nature 457 170
Google Scholar
[40] Antognozzi M, Bermingham C R, Harniman R L, Simpson S, Senior J, Hayward R, Hoerber H, Dennis M R, Bekshaev A Y, Bliokh K Y, Nori F 2016 Nat. Phys. 12 731
Google Scholar
[41] Tumkur T, Yang X, Zhang C, Yang J, Zhang Y, Naik G V, Nordlander P, Halas N J 2018 Nano Lett. 18 2040
Google Scholar
[42] Guan D, Hang Z H, Marcet Z, Liu H, Kravchenko I I, Chan C T, Chan H B, Tong P 2016 Sci. Rep. 5 16216
[43] Jahng J, Ladani F T, Khan R M, Li X, Lee E S, Potma E O 2015 Opt. Lett. 40 5058
Google Scholar
-
图 1 不同峰值拉比频率
$G_ {\rm{R}}^0$ 取值条件下, 在高斯光束束腰所在平面内$z = 0$ , 距离光轴$r =$ 0.7071${\text{μ}}{\rm{m}}$ 处(a)原子横向光力${F_ {\rm{T}}}(t)$ (实线)和纵向光力${F_ {\rm{L}}}(t)$ (虚线)的时间分布情况, (b)粒子数反转$w(t)$ 的时间演化, 其中对应$G_ {\rm{R}}^0 = 0.1\omega $ ,$0.25\omega $ ,$0.5\omega $ 和$\omega $ , 在$r =$ 0.7071${\text{μ}}{\rm{m}}$ 位置处的脉冲面积分别为$A=0.913{\text{π}} $ ,$2.2825{\text{π}} $ ,$4.565{\text{π}} $ 和$9.13{\text{π}}$ ; 光场的失谐量取值$\varDelta = 0$ Figure 1. (a) Temporal evolution of the transverse light force
${F_ {\rm{T}}}(t)$ (solid lines) and the longitudinal light forces${F_ {\rm{L}}}(t)$ (dashed lines); (b) temporal evolution of the population inversion$w(t)$ for different values of the peak Rabi frequencies$G_ {\rm{R}}^0$ at$z = 0$ ,$r = $ 0.7071${\text{μ}}{\rm{m}}$ . Pulse area$A$ at$z = 0$ ,$r = $ 0.7071${\text{μ}}{\rm{m}}$ equals respectively$0.913{\text{π}} $ ,$2.2825{\text{π}} $ ,$4.565{\text{π}} $ and$9.13{\text{π}}$ for the peak Rabi frequency of$G_ {\rm{R}}^0 = 0.1\omega $ ,$0.25\omega $ ,$0.5\omega $ and$\omega $ . Field detuning$\varDelta = 0$ .
图 2 在高斯光束束腰所在平面内距离光轴
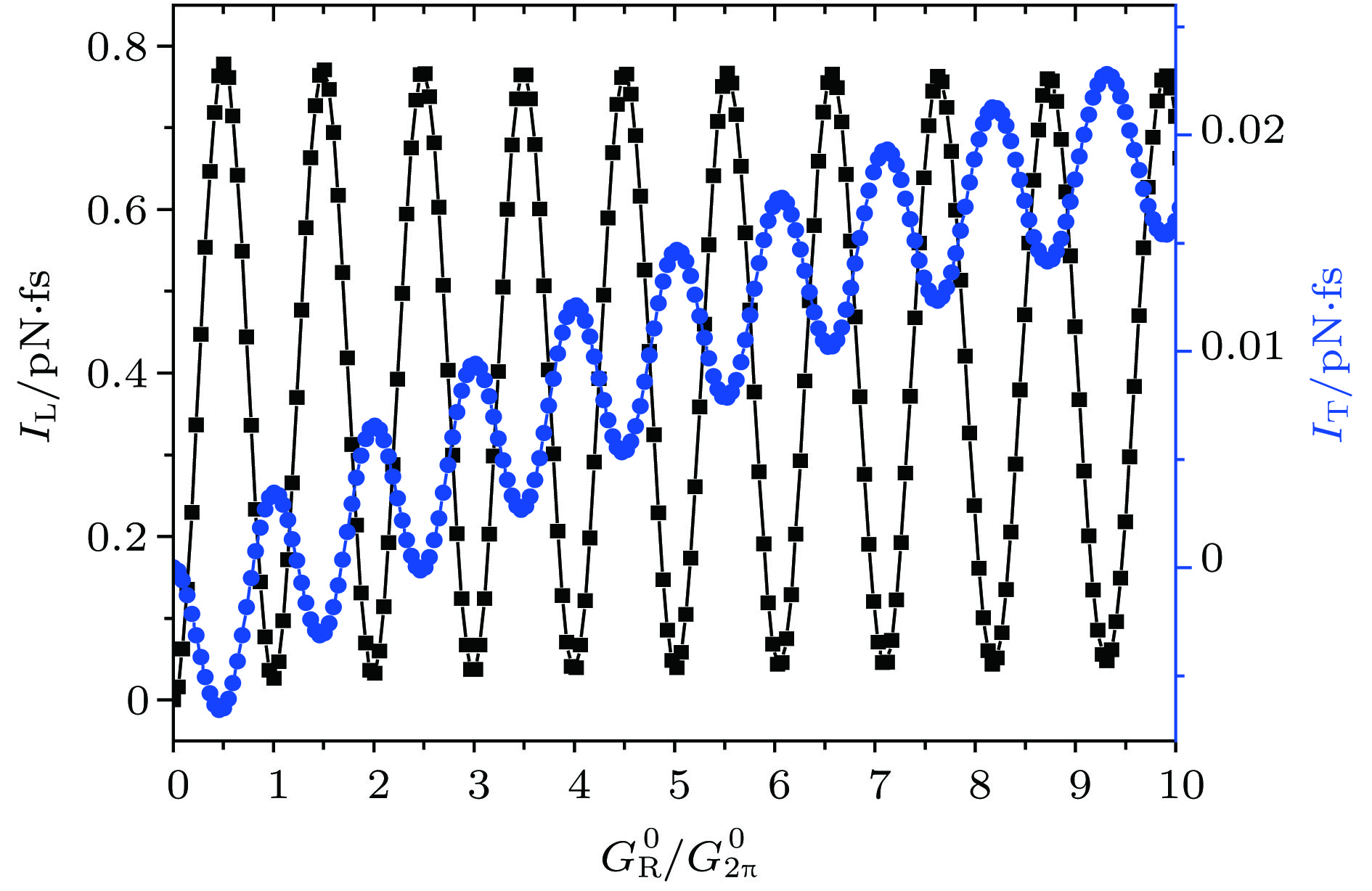
$r = $ 0.7071${\text{μ}}{\rm{m}}$ 处, 原子所受纵向冲量${I_ {\rm{L}}}(r, z)$ (正方形点缀曲线)与横向冲量${I_ {\rm{T}}}(r, z)$ (圆形点缀曲线)随输入脉冲的峰值拉比频率$G_ {\rm{R}}^0 = {d_{10}}{E_0}/\hbar $ 取值的演化情况, 其中$G_{{\text{2π}}}^0$ 对应脉冲面积为$2{\text{π}}$ 时的电场峰值拉比频率, 失谐量取值$\varDelta = 0$ Figure 2. Evolution of the longitudinal impulse
${I_ {\rm{L}}}(r, z)$ (square-dotted curve) and the transverse impulse${I_ {\rm{T}}}(r, z)$ (circle-dotted curve) as a function of the peak Rabi frequency$G_ {\rm{R}}^0 = {d_{10}}{E_0}/\hbar $ at$z = 0$ ,$r =$ 0.7071${\text{μ}}{\rm{m}}.$ $G_{2{\text{π}}}^0$ is the peak Rabi frequency when the area of the pulse equals$2{\text{π}}.$ Detuning$\varDelta = 0$ .
图 3 不同失谐量
$\varDelta = {\omega _{10}} - \omega $ 取值情况下, 在光脉冲强度最强时刻, 束腰所在平面内光学势随径向距离$r$ 的分布情况(拉比频率$G_ {\rm{R}}^0 = 2.0{\omega _{10}}$ )Figure 3. Distribution of the optical potential as function of the transverse distance
$r$ at the central time of the laser pulse with different detunings$\varDelta = {\omega _{10}} - \omega $ for$G_ {\rm{R}}^0 = 2.0{\omega _{10}}$ ,$z = 0$ . -
[1] Meystre P 2001 Atom Optics (New York: Springer) p1
[2] 印建平 2012 原子光学—基本概念、原理、技术及其应用 (上海: 上海交通大学出版社) p12
Yin J P 2012 Atomic Optics: Basic Concepts, Principles, Techniques and Applications (Shanghai: Shanghai Jiao Tong University Press) p12 (in Chinese)
[3] Ashkin A 1970 Phys. Rev. Lett. 24 156
Google Scholar
[4] Hänsch T W, Schawlow A L 1975 Opt. Commun. 13 68
Google Scholar
[5] Chu S, Hollberg L, Bjorkholm J E, Cable A, Ashkin A 1985 Phys. Rev. Lett. 55 48
Google Scholar
[6] Anderson M H, Ensher J R, Matthews M R, Wieman C E, Cornell E A 1995 Science 269 198
Google Scholar
[7] Davis K B, Mewes M O, Andrews M R, van Druten N J, Durfee D S, Kurn D M, Ketterle W 1995 Phys. Rev. Lett. 75 3969
Google Scholar
[8] Cheuk L W, Nichols M A, Okan M, Gersdorf T, Ramasesh V V, Bakr W S, Lompe T, Zwierlein M W 2015 Phys. Rev. Lett. 114 193001
Google Scholar
[9] Parsons M F, Huber F, Mazurenko A, Chiu C S, Setiawan W, Wooley-Brown K, Blatt S, Greiner M 2015 Phys. Rev. Lett. 114 213002
Google Scholar
[10] Haller E, Hudson J, Kelly A, Cotta D A, Peaudecerf B, Bruce G D, Kuhr S 2015 Nat. Phys. 11 738
Google Scholar
[11] 王义遒 2007 原子的激光冷却与陷俘 (北京: 北京大学出版社) p101
Wang Y Q 2007 Laser Cooling and Trapping of Atoms (Beijing: Beijing University Press) p101 (in Chinese)
[12] Metcalf H 2017 Rev. Mod. Phys. 89 041001
Google Scholar
[13] van der Straten P, Metcalf H 2016 Atoms and Molecules Interacting with Light (Cambridge: Cambridge University Press) p1
[14] Jiang Y, Narushima T, Okamoto H 2010 Nat. Phys. 6 1005
Google Scholar
[15] Garbin V, Cojoc D, Ferrari E, Proietti R Z, Cabrini S, Fabrizio E D 2005 Jpn. J. Appl. Phys. 44 5773
Google Scholar
[16] Eichmann U, Nubbemeyer T, Rottke H, Sandner W 2009 Nature 461 1261
Google Scholar
[17] Kumar P, Sarma A K 2012 Phys. Rev. A 86 053414
Google Scholar
[18] Kumar P, Sarma A K 2014 Phys. Rev. A 89 033422
Google Scholar
[19] Cai X, Lin Q 2013 Eur. Phys. J. D 67 246
Google Scholar
[20] Allen L, Eberly J H 1987 Optical Resonance and Two-Level Atoms (New York: Dover Publications, Inc) p41
[21] 张琴, 金康, 唐远河, 屈光辉 2011 60 053204
Zhang Q, Jin K, Tang Y H, Qu G H 2011 Acta Phys. Sin. 60 053204 (in Chinese)
[22] Wang Z L, Yin J P 2008 Chin. Phys. B 17 2466
Google Scholar
[23] Xing J, Chen X, Zhu S, Zhang R 2003 Chin. Opt. Lett. 1 122
[24] Kumar P, Sarma A K 2011 Phys. Rev. A 84 043402
Google Scholar
[25] Lembessis V E, Ellinas D 2005 J. Opt. B: Quant. Sem. Opt. 7 319
[26] Liu B, Jin G, Sun R, He J, Wang J 2017 Opt. Express 25 15861
Google Scholar
[27] Han Y C 2017 J. Phys. B: At. Mol. Opt. Phys. 50 225401
Google Scholar
[28] Liu J C, Wang C K, Gel’mukhanov F 2007 Phys. Rev. A 76 043422
Google Scholar
[29] Cai X, Zheng J, Lin Q 2013 Phys. Rev. A 87 043401
Google Scholar
[30] Boyd R W 2010 Nonlinear Optics (Singapore: Elsevier Pte Ltd) p158
[31] Liu J C, Guo F F, Zhao Y N, Li X Z 2018 Chin. Phys. B 27 104209
Google Scholar
[32] Liu J C, Zhang Y Q, Chen L 2014 J. Mod. Opt. 61 781
Google Scholar
[33] 刘纪彩, 赵珂, 宋玉志, 王传奎 2006 55 1803
Google Scholar
Liu J C, Zhao K, Song Y Z, Wang C K 2006 Acta Phys. Sin. 55 1803
Google Scholar
[34] Liu J C, Sun Y P, Wang C K, Ågren H, Gel’mukhanov F 2010 Phys. Rev. A 81 043412
Google Scholar
[35] Sun Y P, Liu J C, Wang C K, Ge’lmukhanov F 2010 Phys. Rev. A 81 013812
Google Scholar
[36] Butt H J, Cappella B, Kappl M 2005 Surf. Sci. Rep. 59 1
[37] Sukhov S V 2018 J. Commun. Technol. Electr. 63 1137
[38] Florin E L, Pralle A, Hörber J K, Stelzer E H K 1997 J. Stru. Bio. 119 202
Google Scholar
[39] Munday J N, Capasso F, Parsegian V A 2009 Nature 457 170
Google Scholar
[40] Antognozzi M, Bermingham C R, Harniman R L, Simpson S, Senior J, Hayward R, Hoerber H, Dennis M R, Bekshaev A Y, Bliokh K Y, Nori F 2016 Nat. Phys. 12 731
Google Scholar
[41] Tumkur T, Yang X, Zhang C, Yang J, Zhang Y, Naik G V, Nordlander P, Halas N J 2018 Nano Lett. 18 2040
Google Scholar
[42] Guan D, Hang Z H, Marcet Z, Liu H, Kravchenko I I, Chan C T, Chan H B, Tong P 2016 Sci. Rep. 5 16216
[43] Jahng J, Ladani F T, Khan R M, Li X, Lee E S, Potma E O 2015 Opt. Lett. 40 5058
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7673
- PDF Downloads: 77
- Cited By: 0
