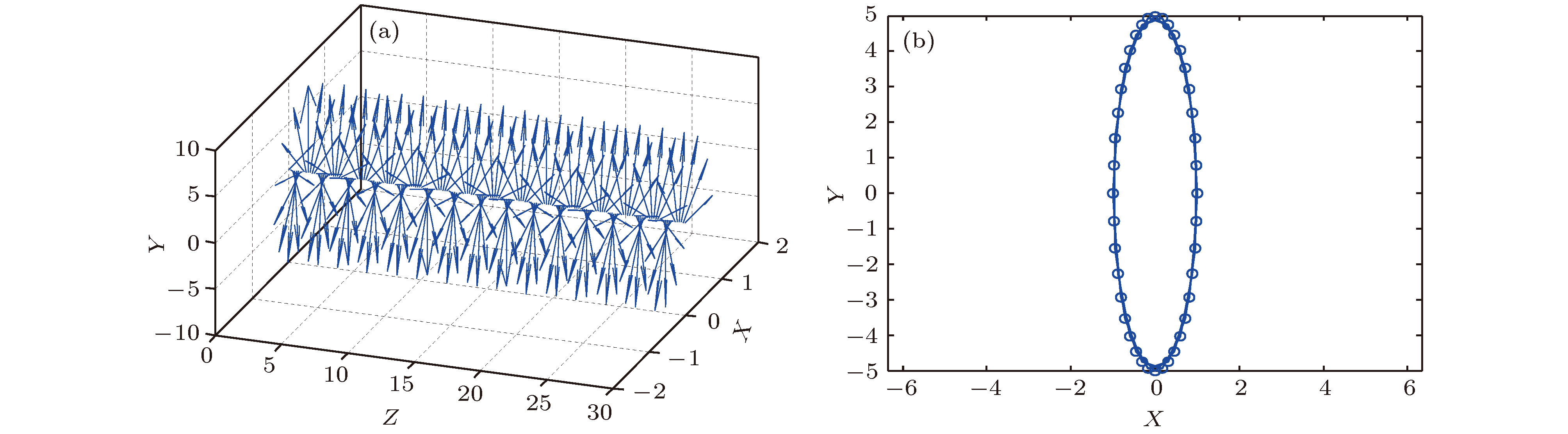
-
As a widely utilized information carrier, polarization microwave shows plenty of merits. Quantum microwave is booming gradually due to the development of superconducting technology, which makes it a promising potential to apply quantum entanglement to polarization microwave. In this paper, we introduce the concept of continuous variable polarization entanglement. Meanwhile, a scheme of polarization entanglement in microwave domain is proposed and simulated. The detail derivations are given and discussed. Polarization entangled microwaves are prepared by combining quadrature entangled signals and strong coherent signals on polarization beam splitters, and quadrature entangled signals are prepared by utilizing Josephson mixer. In order to probe the polarization entanglement between output signals, inseparability of Stokes vectors
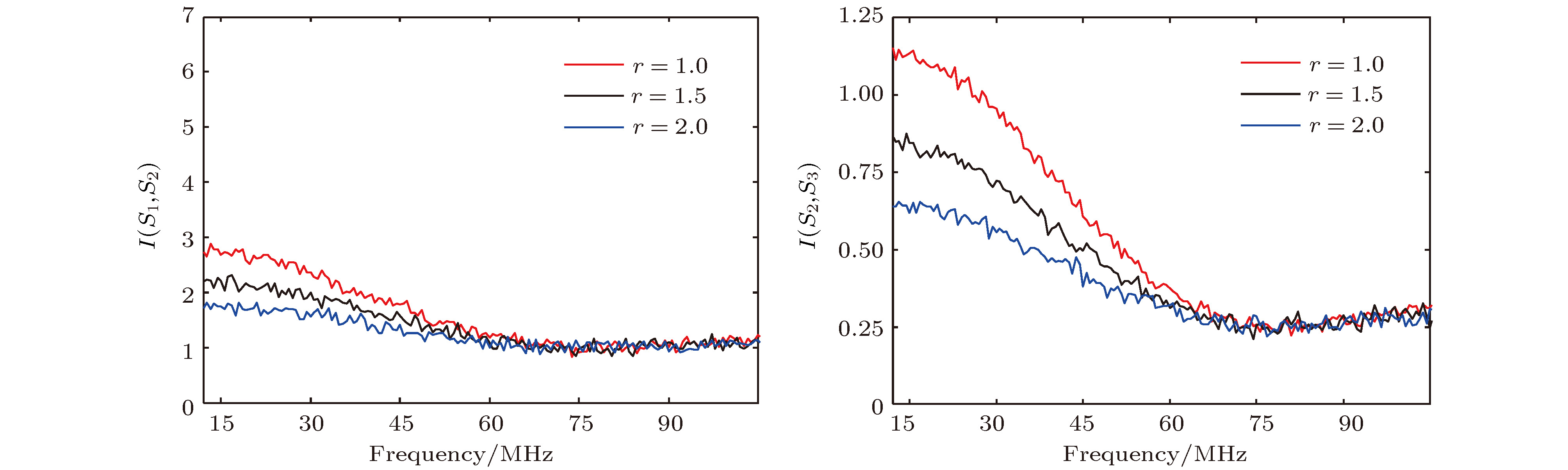
$I({\hat S_1},{\hat S_2})$ and$I({\hat S_2},{\hat S_3})$ , is analyzed in 100 MHz operation bandwidth of Josephson mixer. The relation between inseparability I and squeezing degree r and between inseparability I and amplitude ratio Q are analyzed respectively. The results show that$I({\hat S_1},{\hat S_2})$ is sensitive to the variation of Q, while$I({\hat S_2},{\hat S_3})$ is sensitive to the change of r. The physical reasons for these results are explored and discussed. Apart from these,$I({\hat S_1},{\hat S_2})$ remains its value above 1 under the condition in this paper, but on the contrary,$I({\hat S_2},{\hat S_3})$ keeps its value well below 1. It proves that${\hat S_2}$ and${\hat S_3}$ of Stokes vectors are inseparable from each other, thus output signals${\hat E_a}$ and${\hat E_b}$ of our scheme exhibit bipartite entanglement. The best entanglement appears nearly at about 70 MHz, at this point the minimum$I({\hat S_2},{\hat S_3})$ value is 0.25.-
Keywords:
- continuous variable /
- polarization entanglement /
- Josephson mixer /
- Stokes vectors /
- inseparability
[1] Einstein A, Podolsky B, Rosen N 1935 Phys. Rev. 47 777
 Google Scholar
Google Scholar
[2] Liu C C, Wang D, Sun W Y, Ye L 2017 Quantum Inf. Process. 16 219
 Google Scholar
Google Scholar
[3] Ourjoumtsev A 2010 Nat. Photon. 4 136
 Google Scholar
Google Scholar
[4] Bowen W P, Treps N, Schnabel R, Ralph T C, Lam P K 2003 J. Opt. B: Quantum Semiclassical Opt. 5 s467
 Google Scholar
Google Scholar
[5] Zhou L, Ou-Yang Y, Wang L, Sheng Y B 2017 Quantum Inf. Process. 16 151
 Google Scholar
Google Scholar
[6] Bowen W P, Treps N, Schnabel R, Lam P K 2002 Phys. Rev. Lett. 89 253601
 Google Scholar
Google Scholar
[7] Korolkova N, Leuchs G, Loudon R, Ralph T C, Silberhorn C 2002 Phys. Rev. A 65 052306
 Google Scholar
Google Scholar
[8] Guo J, Cai C X, Ma L, Liu K, Sun H X, Gao J R 2017 Sci. Rep. 7 4434
 Google Scholar
Google Scholar
[9] 吴量, 刘艳红, 邓瑞婕, 闫智辉, 贾晓军 2017 光学学报 5 0527001
Wu L, Liu Y H, Deng R J, Yan Z H, Jia X J 2017 Acta Opt. Sin. 5 0527001
[10] Wu L, Yan Z H, Liu Y H, Deng R J, Jia X J, Xie C D, Peng K C 2016 Appl. Phys. Lett. 108 161102
 Google Scholar
Google Scholar
[11] 周瑶瑶, 蔚娟, 闫智辉, 贾晓军 2018 光学学报 7 0727001
Zhou Y Y, Yu J, Yan Z H, Jia X J 2018 Acta Opt. Sin. 7 0727001
[12] Chen Y F, Hover D, Sendelbach S, Maurer L N 2011 Phys. Rev. Lett. 107 217401
 Google Scholar
Google Scholar
[13] Hofheinz M, Huard B, Portier F 2016 C. R. Phys. 17 679
 Google Scholar
Google Scholar
[14] Flurin E, Roch N, Mallet F, Devoret M H, Huard B 2012 Phys. Rev. Lett. 109 183901
 Google Scholar
Google Scholar
[15] Roch N, Flurin E, Nguyen F, Morfin P, Campagne-Ibarcq P, Devoret M H, Huard B 2012 Phys. Rev. Lett. 108 147701
 Google Scholar
Google Scholar
[16] Flurin E, Roch N, Pillet J D, Mallet F, Huard B 2015 Phys. Rev. Lett. 114 090503
 Google Scholar
Google Scholar
[17] Robson B A 1974 The Theory of Polarization Phenomena (Oxford: Clarendon)
[18] Christopher S R 1998 Radio Sci. 33 1617
 Google Scholar
Google Scholar
[19] Duan L M, Giedke G, Cirac J I, Zoller P 2000 Phys. Rev. Lett. 84 2722
 Google Scholar
Google Scholar
[20] Flurin E 2014 Ph. D. Dissertation (Berkeley: University of California)
[21] Sneep J G, Verhoeven C J M 1990 IEEE J. Solid-State Circuits 25 692
 Google Scholar
Google Scholar
-
表 1 方案部分参数
Table 1. Part of the parameters in the scheme.
参数
名称输入信号
频率/GHz输入信号
振幅压缩参量 r 极化分量
振幅比值 Q参数值 5 1 2 5 -
[1] Einstein A, Podolsky B, Rosen N 1935 Phys. Rev. 47 777
 Google Scholar
Google Scholar
[2] Liu C C, Wang D, Sun W Y, Ye L 2017 Quantum Inf. Process. 16 219
 Google Scholar
Google Scholar
[3] Ourjoumtsev A 2010 Nat. Photon. 4 136
 Google Scholar
Google Scholar
[4] Bowen W P, Treps N, Schnabel R, Ralph T C, Lam P K 2003 J. Opt. B: Quantum Semiclassical Opt. 5 s467
 Google Scholar
Google Scholar
[5] Zhou L, Ou-Yang Y, Wang L, Sheng Y B 2017 Quantum Inf. Process. 16 151
 Google Scholar
Google Scholar
[6] Bowen W P, Treps N, Schnabel R, Lam P K 2002 Phys. Rev. Lett. 89 253601
 Google Scholar
Google Scholar
[7] Korolkova N, Leuchs G, Loudon R, Ralph T C, Silberhorn C 2002 Phys. Rev. A 65 052306
 Google Scholar
Google Scholar
[8] Guo J, Cai C X, Ma L, Liu K, Sun H X, Gao J R 2017 Sci. Rep. 7 4434
 Google Scholar
Google Scholar
[9] 吴量, 刘艳红, 邓瑞婕, 闫智辉, 贾晓军 2017 光学学报 5 0527001
Wu L, Liu Y H, Deng R J, Yan Z H, Jia X J 2017 Acta Opt. Sin. 5 0527001
[10] Wu L, Yan Z H, Liu Y H, Deng R J, Jia X J, Xie C D, Peng K C 2016 Appl. Phys. Lett. 108 161102
 Google Scholar
Google Scholar
[11] 周瑶瑶, 蔚娟, 闫智辉, 贾晓军 2018 光学学报 7 0727001
Zhou Y Y, Yu J, Yan Z H, Jia X J 2018 Acta Opt. Sin. 7 0727001
[12] Chen Y F, Hover D, Sendelbach S, Maurer L N 2011 Phys. Rev. Lett. 107 217401
 Google Scholar
Google Scholar
[13] Hofheinz M, Huard B, Portier F 2016 C. R. Phys. 17 679
 Google Scholar
Google Scholar
[14] Flurin E, Roch N, Mallet F, Devoret M H, Huard B 2012 Phys. Rev. Lett. 109 183901
 Google Scholar
Google Scholar
[15] Roch N, Flurin E, Nguyen F, Morfin P, Campagne-Ibarcq P, Devoret M H, Huard B 2012 Phys. Rev. Lett. 108 147701
 Google Scholar
Google Scholar
[16] Flurin E, Roch N, Pillet J D, Mallet F, Huard B 2015 Phys. Rev. Lett. 114 090503
 Google Scholar
Google Scholar
[17] Robson B A 1974 The Theory of Polarization Phenomena (Oxford: Clarendon)
[18] Christopher S R 1998 Radio Sci. 33 1617
 Google Scholar
Google Scholar
[19] Duan L M, Giedke G, Cirac J I, Zoller P 2000 Phys. Rev. Lett. 84 2722
 Google Scholar
Google Scholar
[20] Flurin E 2014 Ph. D. Dissertation (Berkeley: University of California)
[21] Sneep J G, Verhoeven C J M 1990 IEEE J. Solid-State Circuits 25 692
 Google Scholar
Google Scholar
Catalog
Metrics
- Abstract views: 7675
- PDF Downloads: 63
- Cited By: 0




















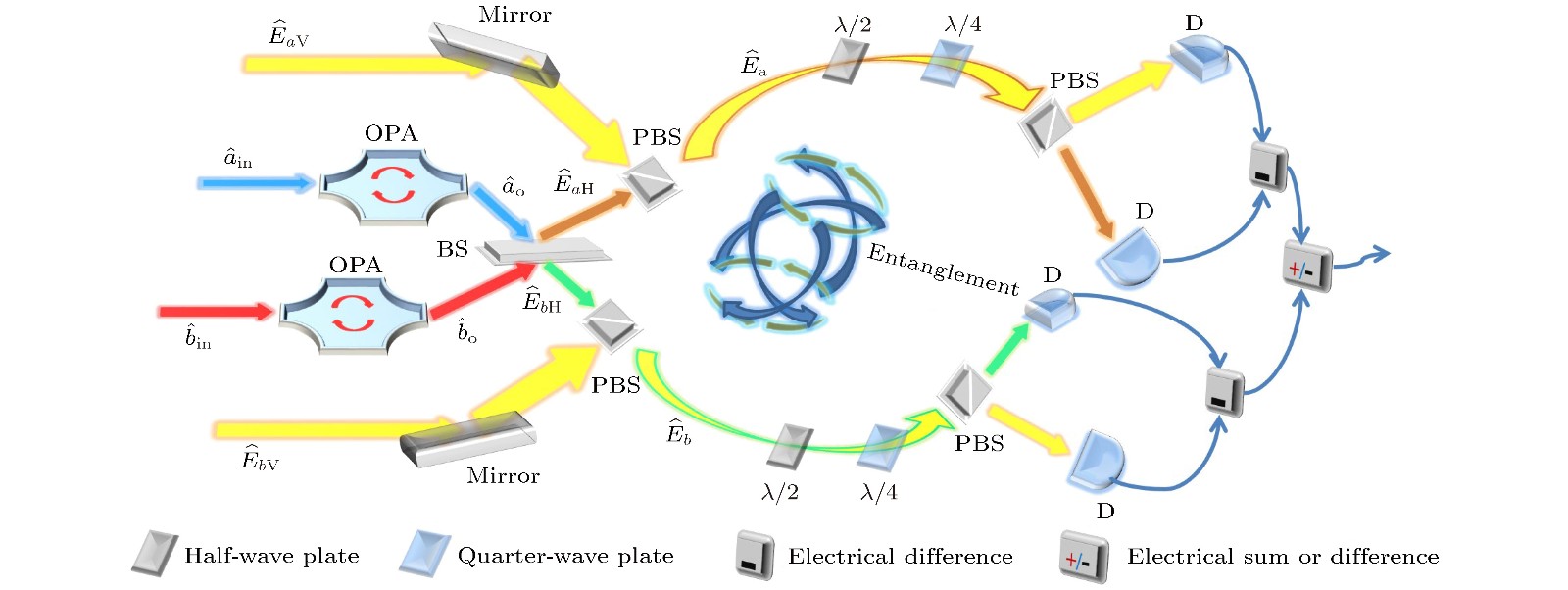
 DownLoad:
DownLoad: