巨腔系统中的光子屏蔽

朱明杰; 赵微; 王治海

doi:10.7498/aps.72.20230049

摘要

随着巨原子研究的展开, 量子干涉效应的应用逐步广泛. 基于量子干涉效应, 利用海森伯-郎之万方程, 研究了巨腔与波导耦合系统的相位调控, 打破了传统小原子与波导耦合体系中偶极近似的限制. 研究表明, 通过给系统外加一对相位差为$\phi$的驱动, 在三腔系统中, 当系统达到稳态时, 腔阵列波导的中间腔与单模巨腔内的光子数可以实现双向屏蔽. 将研究拓展至多腔系统中时, 可在不同条件下分别实现巨腔与耦合腔阵列波导的单向和双向屏蔽. 基于量子干涉效应的光子调控, 该系统可以实现腔的光子数为0, 希望在量子器件设计和量子信息处理等领域实现新应用.

关键词:

Abstract

In the traditional quantum optics and waveguide quantum electrodynamics, atom is usually considered as a point like dipole. However, the successful coupling between a superconducting transmon and surface acoustic wave gives birth to a giant atom, which interacts with the waveguide via more than two points. In the giant atom setup, the dipole approximation breaks down the nonlocal light-matter interaction, it brings lots of unconventional quantum effects, which are presented by the phase interference. As a simplification, the giant resonator, which supports equal energy interval, can be regarded as a linear version of the giant atom. Like the giant atom system, the giant resonator is also coupled to the resonator array waveguide via two sites. According to the quantum interference effect, we study the phase control in giant resonator and the cavities in the waveguide. For a coupled three-resonator system, we reveal the characteristics of the steady state via the Heisenberg-Langevin equations when the driving and dissipation are both present. In such a system, the steady state can be coherently controlled by adjusting the phase difference $\phi$ between the two classical driving fields. We analytically give the existence condition of dark cavity. The results show that only when the middle cavity and the giant resonator are both ideal, can one realize the flash and shielding. Furthermore, we generalize the above study in three resonator system to the multiple cavity system to investigate the photonic flash and shielding. We find that when the number of the middle resonators is $4n+1\, (n\in {Z})$, the bidirectional photonic shielding occurs, that is, the giant resonator can shield the middle resonators in the waveguide and vice versa. On the contrary, when there are $4n+3$ middle resonators in the giant resonator regime, only the directional photonic shielding happens, that is, the giant resonator can shield the waveguide, but the waveguide cannot shield the giant resonator. The above interesting photonic flash and shielding comes from the quantum interference effect. That is, the driving field injects the photons into the waveguide, and the photons propagate in different directions. In the overlapped regime, the photon carrying different phase undergoes destructive interference and acts as a dark resonator. We hope that the interference based photonic control scheme can be applied to the field of quantum device designing.

Keywords:

作者及机构信息

东北师范大学物理学院, 量子科学中心, 长春　130024

通信作者: 王治海, wangzh761@nenu.edu.cn

基金项目: 国家重点研发计划(批准号: 2021YFE0193500)资助的课题

Authors and contacts

Center for Quantum Sciences, School of Physics, Northeast Normal University, Changchun 130024, China

Corresponding author: Wang Zhi-Hai, wangzh761@nenu.edu.cn

Funds: Project supported by the National Key R&D Program of China (Grant No. 2021YFE0193500)

文章全文 : translate this paragraph

参考文献

[1]	Leibfried D, Blatt R, Monroe C, Wineland D 2003 Rev. Mod. Phys. 75 281 Google Scholar
[2]	Haroche S 2013 Rev. Mod. Phys. 85 1083 Google Scholar
[3]	You J Q, Nori F 2011 Nature 474 589 Google Scholar
[4]	Xiang Z L, Ashhab S, You J Q, Nori F 2013 Rev. Mod. Phys. 85 623 Google Scholar
[5]	张智明 2015 量子光学 (北京: 科学出版社) 第26—28页 Zhang Z M 2015 Quantum Optics (Beijing: Science Press) pp26–28 (in Chinese)
[6]	Cai Q Y, Jia W Z 2021 Phys. Rev. A 104 033710 Google Scholar
[7]	Kockum A F, Delsing P, Johansson G 2014 Phys. Rev. A 90 013837 Google Scholar
[8]	Zhao W, Wang Z H 2020 Phys. Rev. A 101 053855 Google Scholar
[9]	Kockum A F 2020 Internation Symposiun on Mathematics, Quantum Theory, and Cryptography Fukuoka, Japan, September 25–27, 2019 p125
[10]	Gustafsson M V, Aref T, Kockum A F, Ekström M K, Johansson G, Delsing P 2014 Science 346 207 Google Scholar
[11]	Guo L Z, Grimsmo A, Kockum A F, Pletyukhov M, Johansson G 2017 Phys. Rev. A 95 053821 Google Scholar
[12]	Kockum A F, Johansson G, Nori F 2018 Phys. Rev. Lett. 120 140404 Google Scholar
[13]	Kannan B, Ruckriegel M J, Campbell D L, Kockum A F, Braumüller J, Kim D K, Kjaergaard M, Krantz P, Melville A, Niedzielski B M, Vepsäläinen A, Winik R, Yoder J L, Nori F, Orlando T P, Gustavsson S, Oliver W D 2020 Nature 583 775 Google Scholar
[14]	Vadiraj A M, Ask A, McConkey T G, Nsanzineza I, Chang C W S, Kockum A F, Wilson C M 2021 Phys. Rev. A 103 023710 Google Scholar
[15]	Zhou L, Yang S, Liu Y X, Sun C P, Nori F 2009 Phys. Rev. A 80 062109 Google Scholar
[16]	Zhou L, Gong Z R, Liu Y X, Sun C P, Nori F 2008 Phys. Rev. Lett. 101 100501 Google Scholar
[17]	程木田 2011 60 117301 Google Scholar Cheng M T 2011 Acta Phys. Sin. 60 117301 Google Scholar
[18]	Witthaut D, SØrensen A S 2010 New J.Phys. 12 043052 Google Scholar
[19]	Zang X F, Jiang C 2010 J. Phys. B: At. Mol. Opt. Phys. 43 065505 Google Scholar
[20]	Liao J Q, Gong Z R, Zhou L, Liu Y X, Sun C P, Nori F 2010 Phys. Rev. A 81 042304 Google Scholar
[21]	石永强, 孔维龙, 吴存仁, 张文轩, 谭磊 2017 66 054204 Google Scholar Shi Y Q, Kong W L, Wu R C, Zhang W X, Tan L 2017 Acta Phys. Sin. 66 054204 Google Scholar
[22]	海莲, 张莎, 李维银, 谭磊 2017 66 154203 Google Scholar Hai L, Zhang S, Li W Y, Tan L 2017 Acta Phys. Sin. 66 154203 Google Scholar
[23]	Shen J T, Fan S H 2009 Phys. Rev. A 79 023838 Google Scholar
[24]	Qin W, Nori F 2016 Phys. Rev. A 93 032337 Google Scholar
[25]	Zhou L, Yang L P, Li Y, Sun C P 2013 Phys. Rev. Lett. 111 103604 Google Scholar
[26]	Du X P, Cao Q, Dang N, Tan L 2021 Eur. Phys. J. D 75 79 Google Scholar
[27]	Wang Z H, Zhou L, Li Y, Sun C P 2014 Phys. Rev. A 89 053813 Google Scholar
[28]	陆赫林, 杜春光 2016 65 214204 Google Scholar Lu H L, Du C G 2016 Acta Phys. Sin. 65 214204 Google Scholar
[29]	Lei F C, Gao M, Du C G, Jing Q L, Long G L 2015 Opt. Express 23 011508 Google Scholar
[30]	Yan X B, Gu K H, Fu C B, Cui C L, Wang R, Wu J H 2014 Eur. Phys. J. D 68 126 Google Scholar
[31]	Yan X B, Gu K H, Fu C B, Cui C L, Wu J H 2014 Chin. Phys. B 23 114201 Google Scholar
[32]	王雅君, 王俊萍, 张文慧, 李瑞鑫, 田龙, 郑耀辉 2021 70 204202 Google Scholar Wang Y J, Wang J P, Zhang W H, Li R X, Tian L, Zheng Y H 2021 Acta Phys. Sin. 70 204202 Google Scholar
[33]	Yin Y, Chen Y, Sank D, O’Malley P J J, White T C, Barends R, Kelly J, Lucero E, Mariantoni M, Megrant A, Neill C, Vainsencher A, Wenner J, Korotkov A N, Cleland A N, Martinis J M 2013 Phys. Rev. Lett. 110 107001 Google Scholar
[34]	Meher N, Sivakumar S, Panigrahi P K 2017 Sci. Rep. 7 9251 Google Scholar
[35]	熊芳, 冯晓强, 谭磊 2016 65 044205 Google Scholar Xiong F, Feng X Q, Tan L 2016 Acta Phys. Sin. 65 044205 Google Scholar
[36]	Wang H, Blencowe M P, Armour A D, Rimberg A J 2017 Phys. Rev. B 96 104503 Google Scholar
[37]	Armour A D, Blencowe M P, Brahimi E, Rimberg A J 2013 Phys. Rev. Lett. 111 247001 Google Scholar
[38]	Wang Z H, Xu X W, Li Y 2017 Phys. Rev. A 95 013815 Google Scholar
[39]	Cui X Y, Wang Z H, Wu J H 2018 Commun. Theor. Phys. 70 215 Google Scholar
[40]	张智明 2015 量子光学 (北京: 科学出版社) 第173—177页 Zhang Z M 2015 Quantum Optics (Beijing: Science Press) pp173–177 (in Chinese)
[41]	Roushan P, Neill C, Tangpanitanon J, Bastidas V M, Megrant A, Barends R, Chen Y, Chen Z, Chiaro B, Martinis J 2017 Science 358 1175 Google Scholar
[42]	Ma R, Saxberg B, Owens C, Leung N, Lu Y, Simon J, Schuster D I 2019 Nature 566 51 Google Scholar

施引文献

图 1 系统模型示意图

Fig. 1. Schematic diagram of system model

下载: 全尺寸图片幻灯片

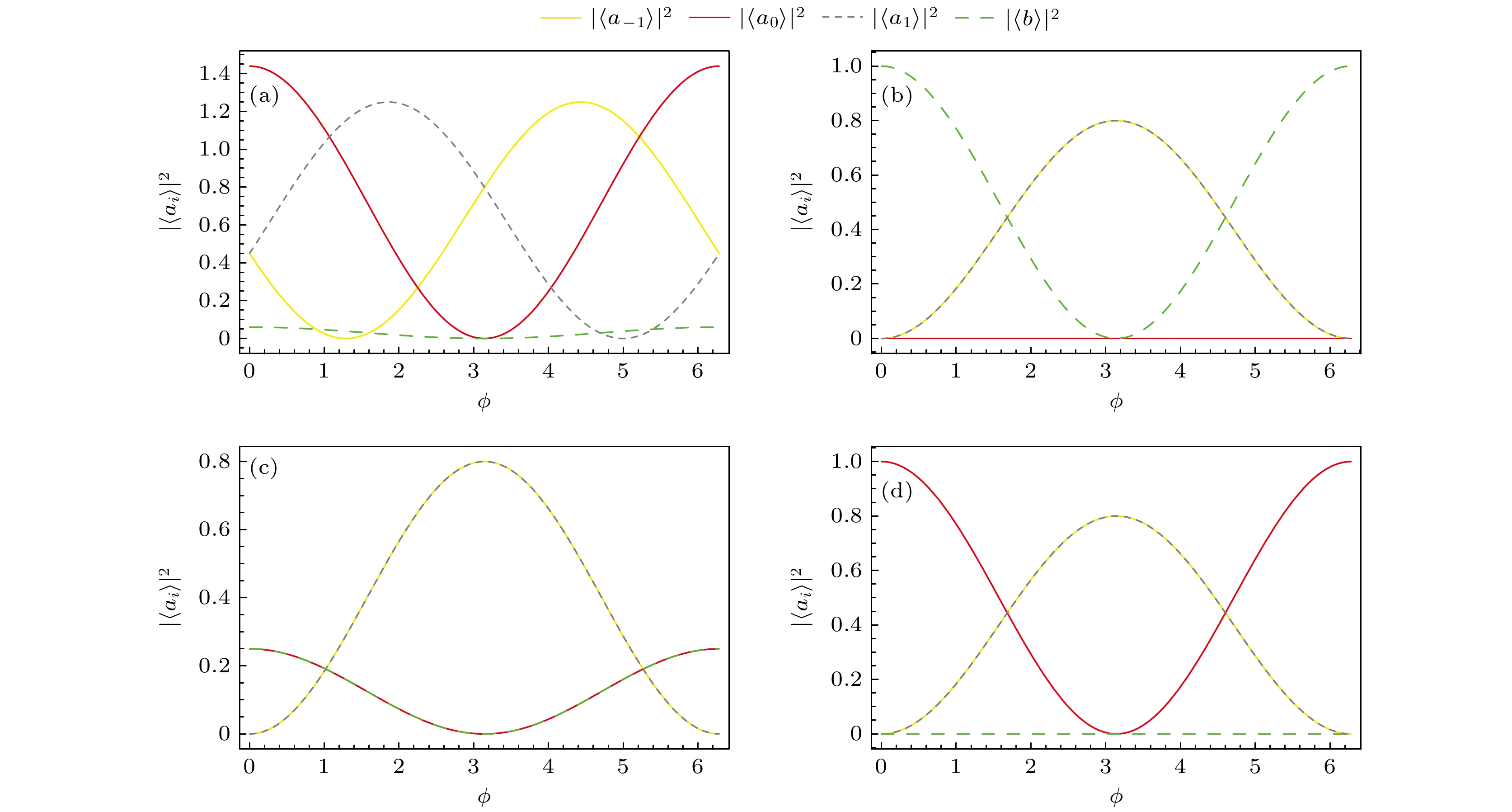
图 2 系统平均光子数随相位差变化图　(a)由暗腔条件所得图像, $ \lambda=J=\varDelta=\varDelta_{0}=\varDelta_{b}=\gamma_{b}=\gamma_{0}=\gamma $, $J_{0}= $$ \dfrac{1}{2\sqrt{6}}\gamma$; (b) $ b $腔为理想腔, $ \lambda=J=J_{0}=\varDelta=\varDelta_{0}=\gamma_{0}=\gamma $, $ \varDelta_{b}=\gamma_{b}=0 $; (c)$ a_{0} $腔与$ b $腔都为理想腔, $ \lambda=J=\varDelta= $$ J_{0}=\gamma=1 $, $\varDelta_{0}=\gamma_{0}= $$ 0$, $ \varDelta_{b}=\gamma_{b}=0 $; (d)$ a_{0} $腔为理想腔, $ \lambda=J=J_{0}=\varDelta=\varDelta_{b}=\gamma_{b}=\gamma $, $ \varDelta_{0}=\gamma_{0}=0 $

Fig. 2. Diagram of system average photon number versus phase difference: (a) Under the dark cavity conditions, $ \lambda=J=\varDelta=\varDelta_{0}=\varDelta_{b}=\gamma_{b}=\gamma_{0}=\gamma $, $J_{0}=\dfrac{1}{2\sqrt{6}}\gamma$; (b) b cavity is ideal, $ \lambda=J=J_{0}=\varDelta=\varDelta_{0}=\gamma_{0}=\gamma $, $ \varDelta_{b}=\gamma_{b}=0 $; (c) b and $ a_{0} $ cavities are ideal, $ \lambda=J=\varDelta=J_{0}=\gamma=1 $, $ \varDelta_{0}=\gamma_{0}=0 $, $ \varDelta_{b}=\gamma_{b}=0 $; (d) $ a_{0} $ cavity is ideal, $ \lambda=J=J_{0}=\varDelta=\varDelta_{b}= $$ \gamma_{b}=\gamma $, $ \varDelta_{0}=\gamma_{0}=0 $

下载: 全尺寸图片幻灯片

图 3 耦合腔阵列为多腔时系统模型示意图

Fig. 3. Schematic diagram of system for coupled resonator array

下载: 全尺寸图片幻灯片

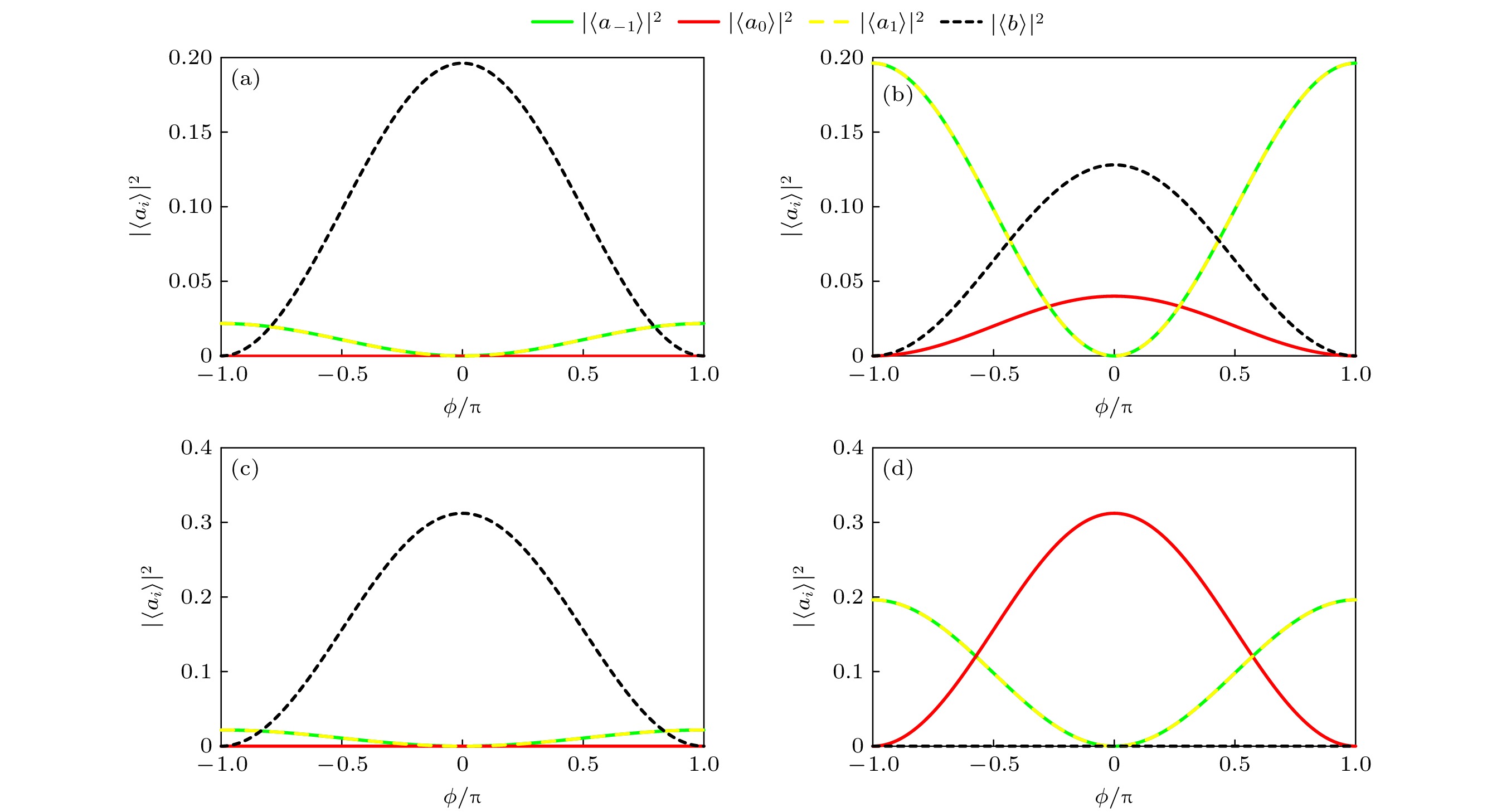
图 4 多腔系统平均光子数随相位差变化图　(a)$ b $腔为理想腔时, $N=8,\; j=4$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=0 $, $ \varDelta_{1}=\gamma_{1}=1 $; (b)中间腔为理想腔时, $N=8,\; j=4$, $ J=J_{0}= $$ \lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=1 $, $ \varDelta_{1}=\gamma_{1}=0 $; (c) $ b $腔为理想腔时, $N=8,\; j=5$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=0 $, $ \varDelta_{1}=\gamma_{1}=1 $; (d)中间腔为理想腔时, $N=8,\; j=5$, $J=J_{0}=\lambda= $$ \varDelta=\gamma=1$, $ \varDelta_{b}=\gamma_{b}=1 $, $ \varDelta_{1}=\gamma_{1}=0 $

Fig. 4. Diagram of the average photon number changing with phase difference in a multi cavities system: (a) b cavity is ideal, $ N=8,\; j=4 $, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=0 $, $ \varDelta_{1}= \gamma_{1}=1 $; (b) the middle cavities are ideal, $N=8,\; j=4$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=1 $, $ \varDelta_{1}=\gamma_{1}=0 $; (c) b cavity is ideal, $N=8,\; j=5$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}= $$ 0 $, $ \varDelta_{1}=\gamma_{1}=1 $; (d) the middle cavities are ideal, $N=8,\; j=5$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=1 $, $ \varDelta_{1}=\gamma_{1}=0 $

下载: 全尺寸图片幻灯片

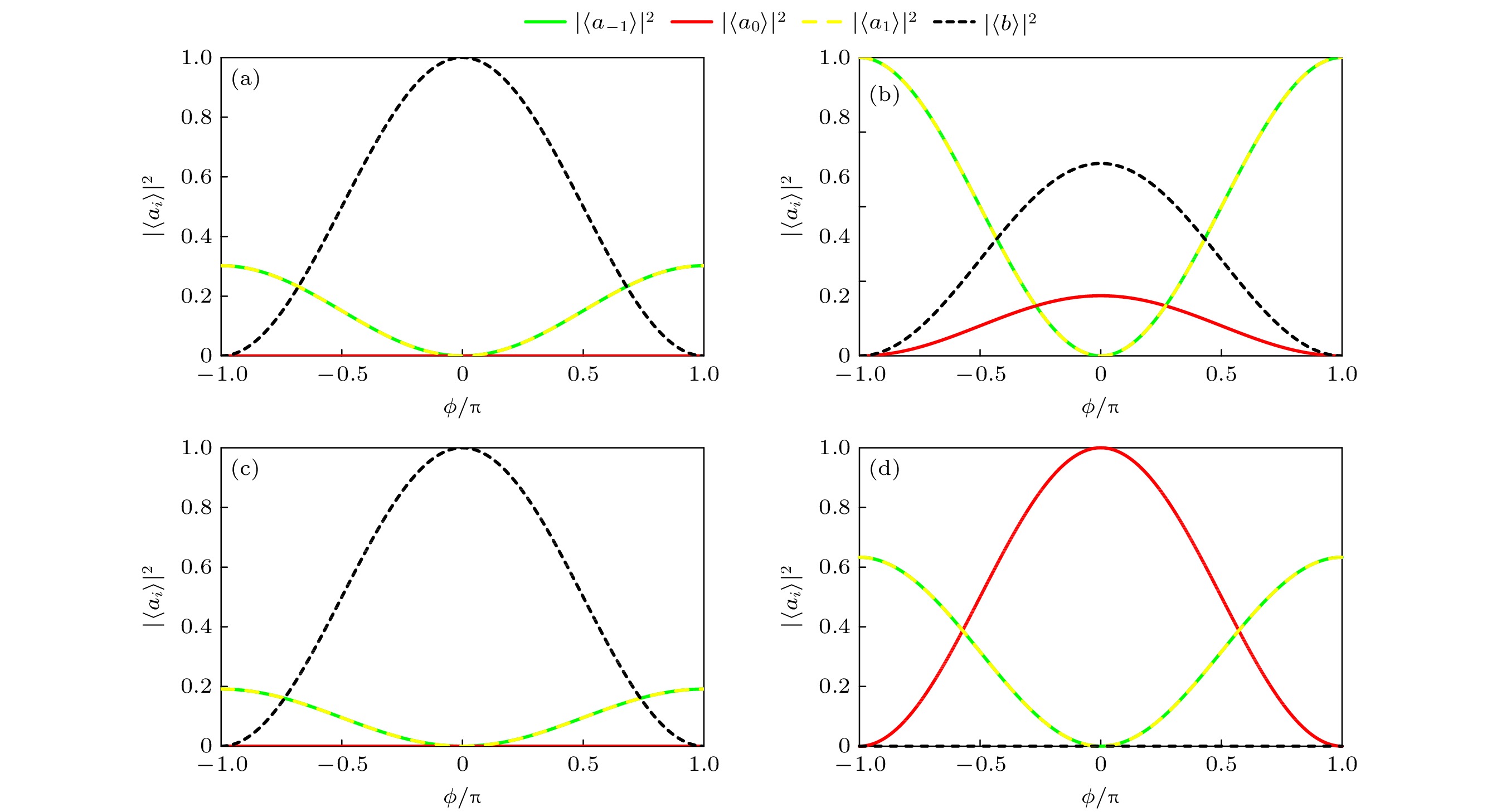
图 5 驱动在$ a_{\pm j} $腔系统平均光子数随相位差变化图　(a)$ b $腔为理想腔时, $N=7,\; j=2$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $\varDelta_{b}=\gamma_{b}= $$ 0$, $ \varDelta_{1}=\gamma_{1}=1 $; (b)中间腔为理想腔时, $N=7,\; j=2$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=1 $, $ \varDelta_{1}=\gamma_{1}=0 $; (c)$ b $腔为理想腔时, $N=7,\; j=3$, $ J=J_{0}=\lambda= $$ \varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=0 $, $ \varDelta_{1}=\gamma_{1}=1 $; (d)中间腔为理想腔时, $N=7,\; j=3$, $J= J_{0}= \lambda= $$ \varDelta= \gamma=1$, $ \varDelta_{b}=\gamma_{b}=1 $, $ \varDelta_{1}=\gamma_{1}=0 $

Fig. 5. Diagram of the average photon number changing with phase difference when driving in the $ a_{\pm j} $ cavities: (a) b cavity is ideal, $N=7,\; j=2$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=0 $, $ \varDelta_{1}=\gamma_{1}=1 $; (b) the middle cavities are ideal, $N=7,\; j=2$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=1 $, $ \varDelta_{1}=\gamma_{1}=0 $; (c) b cavity is ideal, $N=7,\; j=3$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}= $$ 0 $, $ \varDelta_{1}= \gamma_{1}=1 $; (d) the middle cavities are ideal, $N=7, \;j=3$, $ J=J_{0}=\lambda=\varDelta=\gamma=1 $, $ \varDelta_{b}=\gamma_{b}=1 $, $ \varDelta_{1}=\gamma_{1}=0 $

下载: 全尺寸图片幻灯片

map

[1]	Leibfried D, Blatt R, Monroe C, Wineland D 2003 Rev. Mod. Phys. 75 281 Google Scholar
[2]	Haroche S 2013 Rev. Mod. Phys. 85 1083 Google Scholar
[3]	You J Q, Nori F 2011 Nature 474 589 Google Scholar
[4]	Xiang Z L, Ashhab S, You J Q, Nori F 2013 Rev. Mod. Phys. 85 623 Google Scholar
[5]	张智明 2015 量子光学 (北京: 科学出版社) 第26—28页 Zhang Z M 2015 Quantum Optics (Beijing: Science Press) pp26–28 (in Chinese)
[6]	Cai Q Y, Jia W Z 2021 Phys. Rev. A 104 033710 Google Scholar
[7]	Kockum A F, Delsing P, Johansson G 2014 Phys. Rev. A 90 013837 Google Scholar
[8]	Zhao W, Wang Z H 2020 Phys. Rev. A 101 053855 Google Scholar
[9]	Kockum A F 2020 Internation Symposiun on Mathematics, Quantum Theory, and Cryptography Fukuoka, Japan, September 25–27, 2019 p125
[10]	Gustafsson M V, Aref T, Kockum A F, Ekström M K, Johansson G, Delsing P 2014 Science 346 207 Google Scholar
[11]	Guo L Z, Grimsmo A, Kockum A F, Pletyukhov M, Johansson G 2017 Phys. Rev. A 95 053821 Google Scholar
[12]	Kockum A F, Johansson G, Nori F 2018 Phys. Rev. Lett. 120 140404 Google Scholar
[13]	Kannan B, Ruckriegel M J, Campbell D L, Kockum A F, Braumüller J, Kim D K, Kjaergaard M, Krantz P, Melville A, Niedzielski B M, Vepsäläinen A, Winik R, Yoder J L, Nori F, Orlando T P, Gustavsson S, Oliver W D 2020 Nature 583 775 Google Scholar
[14]	Vadiraj A M, Ask A, McConkey T G, Nsanzineza I, Chang C W S, Kockum A F, Wilson C M 2021 Phys. Rev. A 103 023710 Google Scholar
[15]	Zhou L, Yang S, Liu Y X, Sun C P, Nori F 2009 Phys. Rev. A 80 062109 Google Scholar
[16]	Zhou L, Gong Z R, Liu Y X, Sun C P, Nori F 2008 Phys. Rev. Lett. 101 100501 Google Scholar
[17]	程木田 2011 60 117301 Google Scholar Cheng M T 2011 Acta Phys. Sin. 60 117301 Google Scholar
[18]	Witthaut D, SØrensen A S 2010 New J.Phys. 12 043052 Google Scholar
[19]	Zang X F, Jiang C 2010 J. Phys. B: At. Mol. Opt. Phys. 43 065505 Google Scholar
[20]	Liao J Q, Gong Z R, Zhou L, Liu Y X, Sun C P, Nori F 2010 Phys. Rev. A 81 042304 Google Scholar
[21]	石永强, 孔维龙, 吴存仁, 张文轩, 谭磊 2017 66 054204 Google Scholar Shi Y Q, Kong W L, Wu R C, Zhang W X, Tan L 2017 Acta Phys. Sin. 66 054204 Google Scholar
[22]	海莲, 张莎, 李维银, 谭磊 2017 66 154203 Google Scholar Hai L, Zhang S, Li W Y, Tan L 2017 Acta Phys. Sin. 66 154203 Google Scholar
[23]	Shen J T, Fan S H 2009 Phys. Rev. A 79 023838 Google Scholar
[24]	Qin W, Nori F 2016 Phys. Rev. A 93 032337 Google Scholar
[25]	Zhou L, Yang L P, Li Y, Sun C P 2013 Phys. Rev. Lett. 111 103604 Google Scholar
[26]	Du X P, Cao Q, Dang N, Tan L 2021 Eur. Phys. J. D 75 79 Google Scholar
[27]	Wang Z H, Zhou L, Li Y, Sun C P 2014 Phys. Rev. A 89 053813 Google Scholar
[28]	陆赫林, 杜春光 2016 65 214204 Google Scholar Lu H L, Du C G 2016 Acta Phys. Sin. 65 214204 Google Scholar
[29]	Lei F C, Gao M, Du C G, Jing Q L, Long G L 2015 Opt. Express 23 011508 Google Scholar
[30]	Yan X B, Gu K H, Fu C B, Cui C L, Wang R, Wu J H 2014 Eur. Phys. J. D 68 126 Google Scholar
[31]	Yan X B, Gu K H, Fu C B, Cui C L, Wu J H 2014 Chin. Phys. B 23 114201 Google Scholar
[32]	王雅君, 王俊萍, 张文慧, 李瑞鑫, 田龙, 郑耀辉 2021 70 204202 Google Scholar Wang Y J, Wang J P, Zhang W H, Li R X, Tian L, Zheng Y H 2021 Acta Phys. Sin. 70 204202 Google Scholar
[33]	Yin Y, Chen Y, Sank D, O’Malley P J J, White T C, Barends R, Kelly J, Lucero E, Mariantoni M, Megrant A, Neill C, Vainsencher A, Wenner J, Korotkov A N, Cleland A N, Martinis J M 2013 Phys. Rev. Lett. 110 107001 Google Scholar
[34]	Meher N, Sivakumar S, Panigrahi P K 2017 Sci. Rep. 7 9251 Google Scholar
[35]	熊芳, 冯晓强, 谭磊 2016 65 044205 Google Scholar Xiong F, Feng X Q, Tan L 2016 Acta Phys. Sin. 65 044205 Google Scholar
[36]	Wang H, Blencowe M P, Armour A D, Rimberg A J 2017 Phys. Rev. B 96 104503 Google Scholar
[37]	Armour A D, Blencowe M P, Brahimi E, Rimberg A J 2013 Phys. Rev. Lett. 111 247001 Google Scholar
[38]	Wang Z H, Xu X W, Li Y 2017 Phys. Rev. A 95 013815 Google Scholar
[39]	Cui X Y, Wang Z H, Wu J H 2018 Commun. Theor. Phys. 70 215 Google Scholar
[40]	张智明 2015 量子光学 (北京: 科学出版社) 第173—177页 Zhang Z M 2015 Quantum Optics (Beijing: Science Press) pp173–177 (in Chinese)
[41]	Roushan P, Neill C, Tangpanitanon J, Bastidas V M, Megrant A, Barends R, Chen Y, Chen Z, Chiaro B, Martinis J 2017 Science 358 1175 Google Scholar
[42]	Ma R, Saxberg B, Owens C, Leung N, Lu Y, Simon J, Schuster D I 2019 Nature 566 51 Google Scholar

[1]	闫玮植, 范青, 杨鹏飞, 李刚, 张鹏飞, 张天才. 微光学腔内单原子的俘获及其耦合强度的精确调控. , 2023, 72(11): 114202. doi: 10.7498/aps.72.20222220
[2]	钱其升, 刘慧研, 查永鹏, 倪海彬. 非对称共轴腔结构色产生与调控. , 2022, 71(8): 084103. doi: 10.7498/aps.71.20211337
[3]	郑赟杰, 王晨阳, 谢双媛, 许静平, 羊亚平. 含多个相干耦合人工原子的单模腔的输入输出特性. , 2022, 71(24): 244204. doi: 10.7498/aps.71.20221456
[4]	李泽宇, 姜去寒, 马腾洲, 袁英豪, 陈麟. 基于太赫兹石墨烯等离激元的多参数相位可调谐结构及其应用. , 2021, 70(22): 224202. doi: 10.7498/aps.70.20210445
[5]	朱纪霖, 高东宝, 曾新吾. 基于相位变换声镊的单个微粒平面移动操控. , 2021, 70(21): 214302. doi: 10.7498/aps.70.20210981
[6]	魏伟华, 李木天, 刘墨南. 基于飞秒激光直写的单向单模耦合微腔. , 2018, 67(6): 064203. doi: 10.7498/aps.67.20172395
[7]	海莲, 张莎, 李维银, 谭磊. 耦合腔阵列与-型三能级原子非局域耦合系统中单光子的传输特性研究. , 2017, 66(15): 154203. doi: 10.7498/aps.66.154203
[8]	石永强, 孔维龙, 吴仁存, 张文轩, 谭磊. 耗散耦合腔阵列耦合量子化腔场驱动三能级体系中的单光子输运. , 2017, 66(5): 054204. doi: 10.7498/aps.66.054204
[9]	戚志明, 梁文耀. 表层厚度渐变一维耦合腔光子晶体的反射相位特性及其应用. , 2016, 65(7): 074201. doi: 10.7498/aps.65.074201
[10]	熊芳, 冯晓强, 谭磊. 双光子过程耗散耦合腔阵列中的量子相变. , 2016, 65(4): 044205. doi: 10.7498/aps.65.044205
[11]	李红霞, 江阳, 白光富, 单媛媛, 梁建惠, 马闯, 贾振蓉, 訾月姣. 有源环形谐振腔辅助滤波的单模光电振荡器. , 2015, 64(4): 044202. doi: 10.7498/aps.64.044202
[12]	周静, 王鸣, 倪海彬, 马鑫. 环形狭缝腔阵列光学特性的研究. , 2015, 64(22): 227301. doi: 10.7498/aps.64.227301
[13]	鲍佳, 谭磊. 失谐对耗散耦合腔阵列体系超流-绝缘相变的影响. , 2014, 63(8): 084201. doi: 10.7498/aps.63.084201
[14]	倪志鹏, 王秋良, 严陆光. 短腔、自屏蔽磁共振成像超导磁体系统的混合优化设计方法. , 2013, 62(2): 020701. doi: 10.7498/aps.62.020701
[15]	王争, 赵新杰, 何明, 周铁戈, 岳宏卫, 阎少林. 嵌入到Fabry-Perot谐振腔的双晶约瑟夫森结阵列的阻抗匹配和相位锁定研究. , 2010, 59(5): 3481-3487. doi: 10.7498/aps.59.3481
[16]	王燕花, 任文华, 刘艳, 谭中伟, 简水生. 相位修正的耦合模理论用于计算光纤Bragg光栅法布里-珀罗腔透射谱. , 2008, 57(10): 6393-6399. doi: 10.7498/aps.57.6393
[17]	杜晓宇, 郑婉华, 任刚, 王科, 邢名欣, 陈良惠. 二维光子晶体耦合腔阵列的慢波效应研究. , 2008, 57(1): 571-575. doi: 10.7498/aps.57.571
[18]	冯立娟, 江海涛, 李宏强, 张冶文, 陈鸿. 光子晶体耦合腔波导的色散特性. , 2005, 54(5): 2102-2105. doi: 10.7498/aps.54.2102
[19]	谭华堂, 甘仲惟, 李高翔. 与压缩真空库耦合的单模腔内三量子点中激子纠缠. , 2005, 54(3): 1178-1183. doi: 10.7498/aps.54.1178
[20]	张光寅, 张潮波, 丁欣, 宋峰, 郭曙光, 孟凡臻. 固体激光腔动力学稳定性的调控. , 2002, 51(2): 253-258. doi: 10.7498/aps.51.253

计量

文章访问数: 2830
PDF下载量: 88
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

巨腔系统中的光子屏蔽