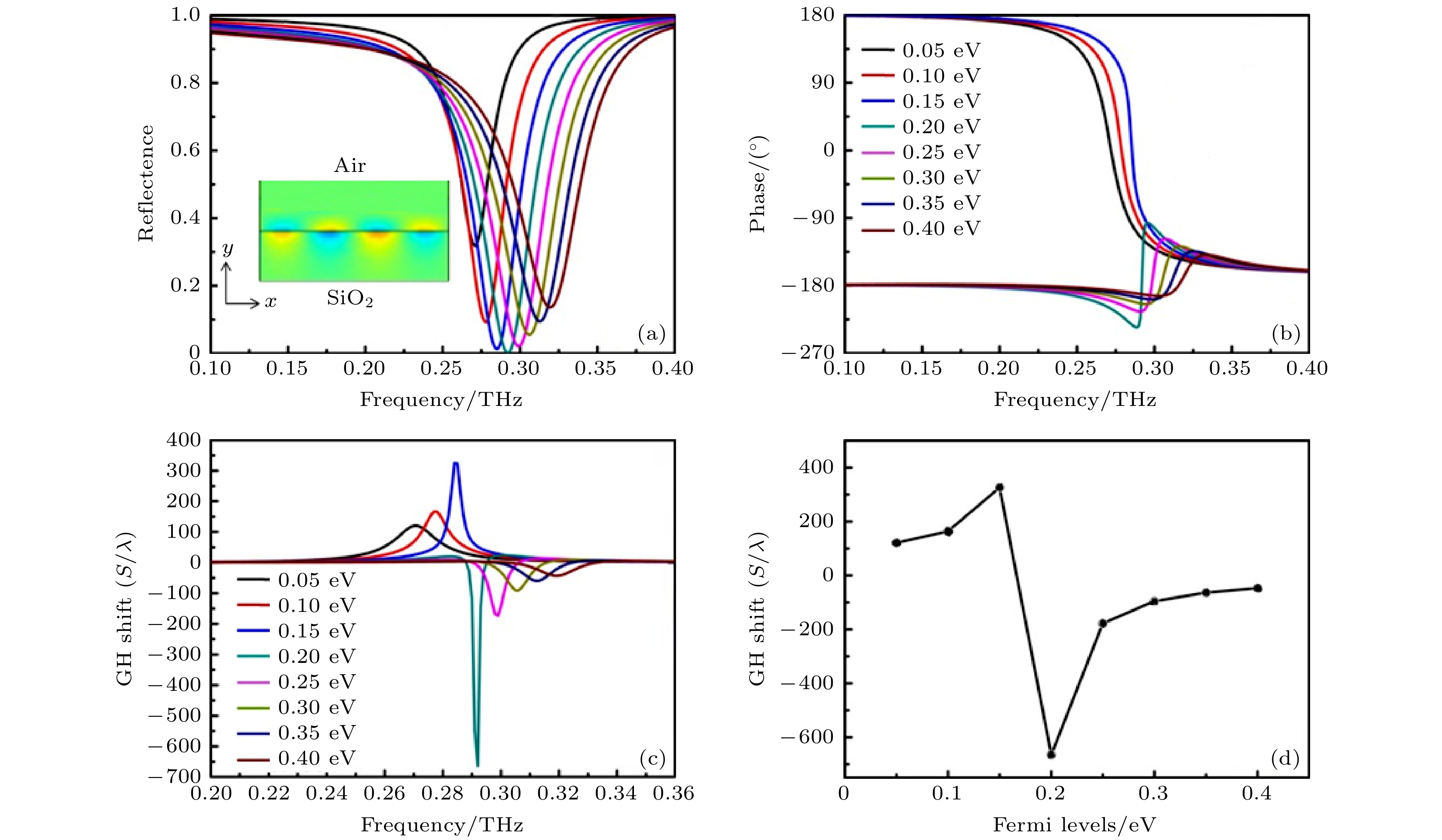
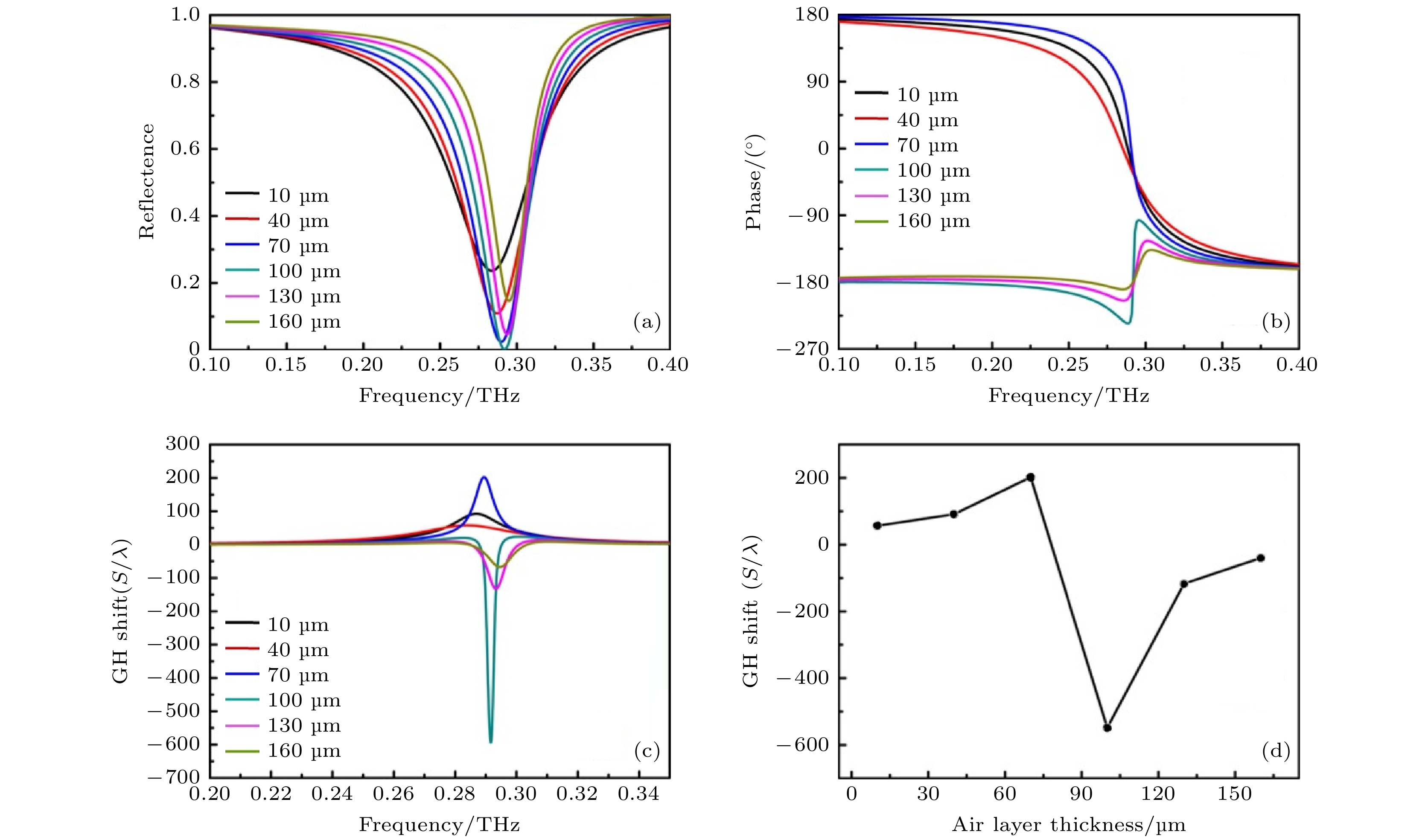
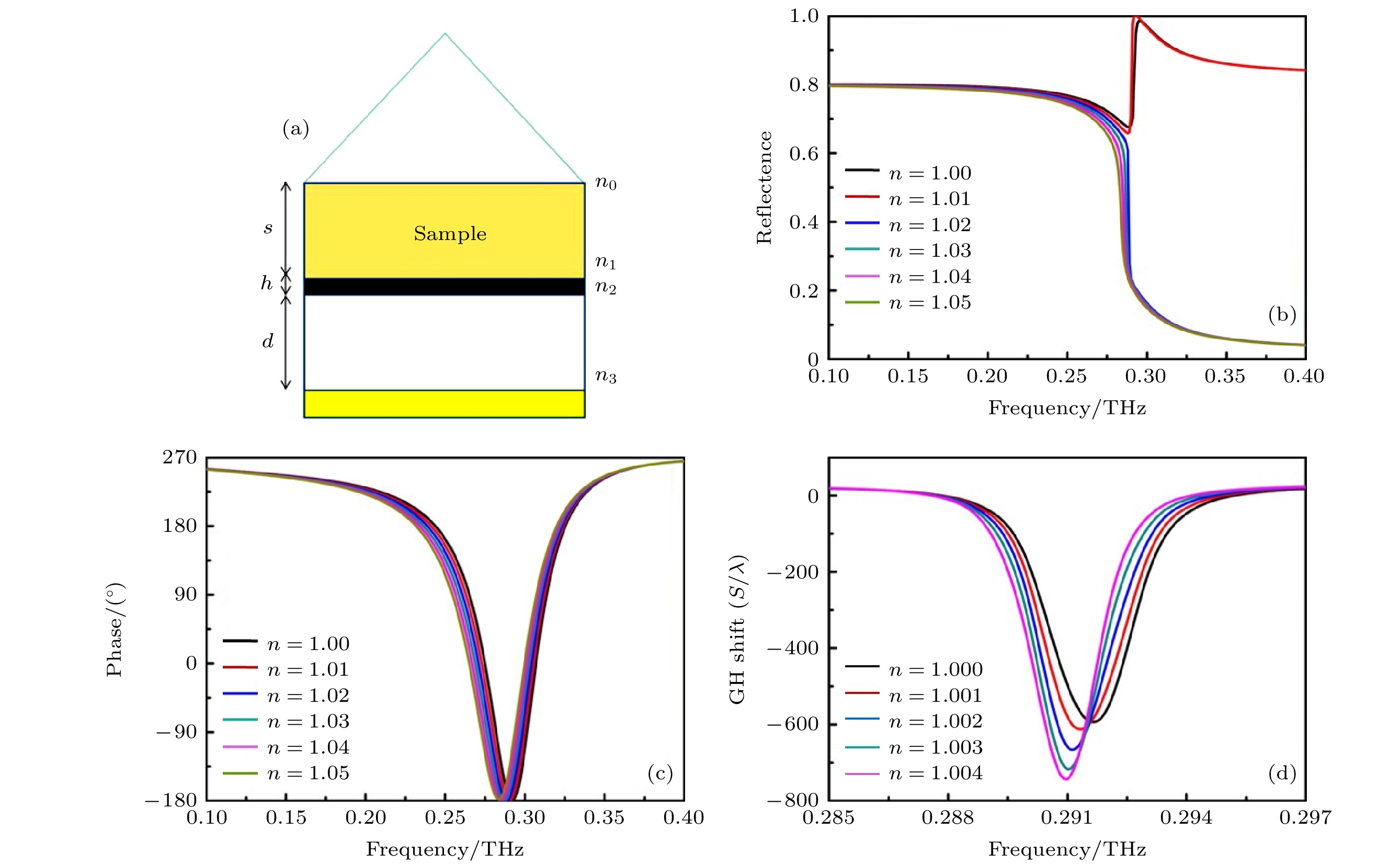
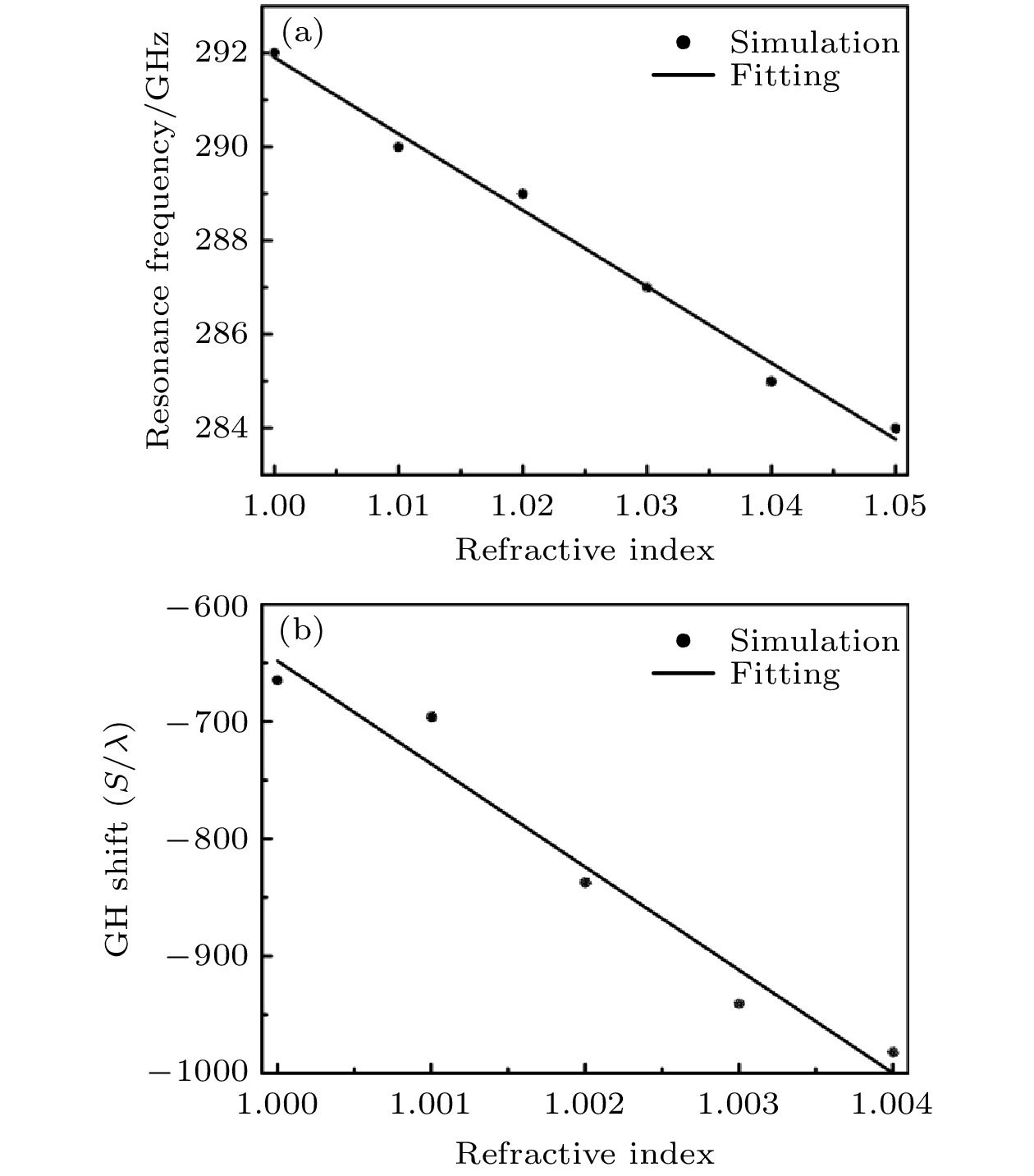
-
太赫兹波的振幅和相位进行主动调控由于在太赫兹功能器件方面的广泛应用, 受到了广泛关注. 目前采用的金属-介质-金属超表面结构结合石墨烯等二维材料可实现太赫兹振幅/相位的动态调控, 但存在调控自由度少(电压或光强)以及超表面结构加工工艺复杂及价格昂贵等缺点. 本文提出了一种棱镜耦合石墨烯等离激元结构的相位调控结构. 该结构不仅可以通过通常方式调控费米能级实现对相位的调控, 还可以通过调控空气隙的厚度和预铺石墨烯的层数改变结构的本征损耗和辐射损耗, 从而对结构的相位进行调控, 这是由结构中的本征损耗和辐射损耗的差值决定, 与结构处于欠耦合/过耦合状态密切相关. 对结构相位的调控还会导致太赫兹古斯汉欣位移大小和正负的选择. 进一步, 本文阐述了结构的欠耦合和过耦合状态对古斯汉欣位移的符号有重要影响. 结果表明, 通过对空气隙的厚度和石墨烯的费米能级进行动态调控, 改变系统的本征损耗和辐射损耗, 可以实现相位的调控, 最终实现过阻尼到欠阻尼的转变. 在此物理过程中, 系统的古斯汉欣位移也会发生明显的变化. 与金属-介质-金属超表面结构相位调控器相比, 本文提出的结构具有工艺简单(不需要微结构加工工艺), 可调谐自由度高(可利用石墨烯费米能级和空气隙动态调控, 还可通过控制石墨烯层数调控)等优点. 本文结果为多参数可调谐的太赫兹传感器件的发展开辟了新的途径.The active modulation of the amplitude and phase of terahertz wave has been widely adopted in terahertz functional devices. The current metal-insulator-metal metasurface structure combined with two-dimensional materials such as graphene can realize dynamic control of terahertz amplitude/phase, but it has some disadvantages such as less freedom of control (voltage or light intensity), complex processing technology and high price of metasurface structure. In this article, we propose a prism-coupled matel-insulator-graphene (MIG) phase regulation structure. This structure can not only control the phase by adjusting the Fermi level in the usual way, but also change the intrinsic loss and radiation loss of the structure by adjusting the thickness of the air gap and the number of layers of pre-spread graphene, so that the phase of the structure can be controlled, which is determined by the difference between intrinsic loss and radiation loss of the fabric, which is closely related to this structure staying in the under-coupling/over-coupling state. The adjustment of the structural phase can also lead the magnitude of the terahertz Goos–Hänchen(GH) displacement and its positive sign and negative sign to be selected. Furthermore, it is shown that the under-coupling state and the over-coupling state of the structure have an important effect on the coincidence of the Goos–Hanchen (GH) displacement. The results show that by dynamically adjusting the thickness of the air gap and the Fermi level of graphene, and changing the eigenloss and radiation loss of the system, the phase regulation can be achieved. Finally, the transition from overdamped to underdamped state is realized. In this physical process, the GH displacement of the system will also change obviously. This paper puts forward the structure of the process with simple processing technology (no need to microstructure), tunable high degrees of freedom (available graphene Fermi level and air gap dynamic regulation, also could be regulated and controlled by controlling the graphene layers) in comparison with the phase modulator of metal-insulator-metal super surface structure. The results of this paper open up a new way of developing the multi-parameter tunable terahertz sensor components.
-
Keywords:
- terahertz /
- graphene /
- phase transition /
- Goos–Hänchen shift
[1] Hao J M, Ren Q J, An Z H, Huang X Q, Chen Z H, Qiu M, Zhou L 2009 Phys. Rev. A 80 023807
Google Scholar
[2] Hao J M, Yuan Y, Ran L X, Jiang T, Kong J A, Chan C T, Zhou L 2007 Phys. Rev. Lett. 99 063908
Google Scholar
[3] Chen L, Liao D G, Guo X G, Zhao J Y, Zhu Y M, Zhuang S L 2019 Front. Inform. Technol. Electron. Eng. 20 591
Google Scholar
[4] Sievenpiper D, Zhang L J, Broas R F J, et al. 1999 IEEE Trans. Microwave Theory Tech. 47 2059
Google Scholar
[5] Zhou L, Wen W J, Chan C T, Sheng P 2003 Appl. Phys. Lett. 83 3257
Google Scholar
[6] Anders P, Sergey I B 2013 Opt. Express 21 27438
Google Scholar
[7] Dai Ch L, Sun G Q, Hu L Y, Xiao Y K, Zhang Z P, Qu L T 2020 InfoMat. 2 12039
[8] Ding L, Qiu T Y, Zhang J, Wen X 2019 J. Opt. 21 125602
Google Scholar
[9] Hu T, Bingham C M, Strikwerda A C, et al. 2008 Phys. Rev. B 78 241103
Google Scholar
[10] Hao J M, Wang J, Liu X L, Willie J P, Zhou L, Qiu M 2010 Appl. Phys. Lett. 96 251104
Google Scholar
[11] Liu N, Martin M, Thomas W, Mario H, Harald G 2010 Nano Lett. 10 2342
Google Scholar
[12] Claire M W, Liu X L, Willie J P 2012 Adv. Mater. 24 OP98
[13] Sun S L, Yang K Y, Wang C M, et al. 2012 Nano Lett. 12 6223
Google Scholar
[14] Anders P, Ole A, Ilya P R, Sergey I B 2013 Sci. Rep. 3 2155
Google Scholar
[15] Li X, Xiao S Y, Cai B G, He Q, Cui T J, Zhou L 2012 Opt. Lett. 37 4940
Google Scholar
[16] Anders P, Michael G. N, René L E, Sergey I B 2013 Nano Lett. 13 829
Google Scholar
[17] Chen L, Wei M Y, Zang X F, Zhu Y M, Zhuang S L 2016 Sci. Rep. 6 22027
Google Scholar
[18] Chen L, Xu N N, Leena S, Cui T J, Ranjan S, Zhu Y M, Zhang W L 2017 Adv. Opt. Mater. 5 1600960
Google Scholar
[19] Xu J J, Liao D G, Gupta M, Zhu Y M, Zhuang S L, Singh R, Chen L 2021 Adv. Opt. Mater. 9 2100024
[20] Miao Z Q, Wu Q, Li X, He Q, Ding K, An Z H, Zhang Y B, Zhou L 2015 Phys. Rev. X 5 041027
[21] Qu C, Ma S J, Hao J M, Qiu M, et al. 2015 Phys. Rev. Lett. 115 235503
Google Scholar
[22] Qing Y M, Ma H F, Cui T J 2018 Opt. Express 26 32442
Google Scholar
[23] Cong L Q, Pitchappa P, Lee C K, Singh R 2017 Adv. Mater. 29 1700733
Google Scholar
[24] Chen L, Ge Y F, Zang X F, et al. 2019 IEEE Trans. Terahertz Sci. Technol. 9 643
Google Scholar
[25] Yin S, Shi X T, Huang W, Zhang W T, Hu F R, Qin Z J, Xiong X M 2019 Electronics 8 1528
Google Scholar
[26] Li J S, Wu J F, Zhang L 2014 IEEE Photonics J. 6 2374591
[27] Alaee R, Farhat M, Rockstuhl C, Lederer F 2012 Opt. Express 20 28017
Google Scholar
[28] Chen L, Cao Z Q, Shen Q S, Deng X X 2007 J. Lightwave Technol. 25 539
Google Scholar
[29] Artmann K 1948 Ann. Phys. 437 87
Google Scholar
[30] Chen L, Cao Z Q, Ou F, Li H G, Shen Q S, Qiao H C 2007 Opt. Lett. 32 1432
Google Scholar
[31] Chen L, Zhu Y M, Zang X F, Cai B, Li Z, Xie L, Zhuang S L 2013 Light Sci. Appl. 2 e60
Google Scholar
-
图 2 不同费米能级下石墨烯电导率的实部虚部随频率的变化曲线 (a) 石墨烯电导率实部 (b) 石墨烯电导率虚部
Fig. 2. Curves of real and imaginary parts of graphene conductivity with frequency at different Fermi levels: (a) The real part of graphene conductivity; (b) the imaginary part of graphene conductivity.
图 3 在不同费米能级下, 系统的反射率(a)、相位(b)和GH位移(c)随频率的对应关系; (d)GH位移随费米能级的变化产生的突变
Fig. 3. The reflectance(a), phase (b), and GH shift (c) of the system are corresponding to frequency at different Fermi levels; (d) GH shifts with respect to Fermi levels.
图 4 在不同的空气层厚度下, 系统的反射率(a), 相位(b)和GH位移(c)随频率的对应关系; (d)GH位移随空气隙厚度的变化产生的突变
Fig. 4. The reflectance(a), phase (b) and GH displacement (c) of the system are corresponding to the frequency at different air layer thicknesses; (d) GH shifts with respect to air layer thicknesses
图 5 在不同的石墨烯层数下, 系统的反射率(a), 相位(b)和GH位移(c)随频率的对应关系; (d)GH位移随石墨烯层数的变化产生的突变
Fig. 5. The reflectance (a), phase (b), and GH shift (c) of the system are corresponding to frequency at different graphene layers; (d) GH shifts with respect to number of graphene layers.
图 7 在空气腔中(a)不同的折射率变化下, 系统的反射率(c), 相位(b)和GH位移(d)随频率的对应关系
Fig. 7. The reflectance (c), phase (b) and GH displacement (d) of the system as a function of frequency under different refractive index changes in the air cavity (a).
图 8 (a) 反射共振频率随折射率的变化(灵敏度为160 GHz/RIU); (b) GH位移强度随折射率的变化(灵敏度为2.1×104 λ/RIU)
Fig. 8. (a) Reflection resonant frequency as a function of refractive index (sensitivity is 160 GHz/RIU); (b) GH shift intensity as a function of refractive index (Sensitivity is 2.1 × 104 λ /RIU).
-
[1] Hao J M, Ren Q J, An Z H, Huang X Q, Chen Z H, Qiu M, Zhou L 2009 Phys. Rev. A 80 023807
Google Scholar
[2] Hao J M, Yuan Y, Ran L X, Jiang T, Kong J A, Chan C T, Zhou L 2007 Phys. Rev. Lett. 99 063908
Google Scholar
[3] Chen L, Liao D G, Guo X G, Zhao J Y, Zhu Y M, Zhuang S L 2019 Front. Inform. Technol. Electron. Eng. 20 591
Google Scholar
[4] Sievenpiper D, Zhang L J, Broas R F J, et al. 1999 IEEE Trans. Microwave Theory Tech. 47 2059
Google Scholar
[5] Zhou L, Wen W J, Chan C T, Sheng P 2003 Appl. Phys. Lett. 83 3257
Google Scholar
[6] Anders P, Sergey I B 2013 Opt. Express 21 27438
Google Scholar
[7] Dai Ch L, Sun G Q, Hu L Y, Xiao Y K, Zhang Z P, Qu L T 2020 InfoMat. 2 12039
[8] Ding L, Qiu T Y, Zhang J, Wen X 2019 J. Opt. 21 125602
Google Scholar
[9] Hu T, Bingham C M, Strikwerda A C, et al. 2008 Phys. Rev. B 78 241103
Google Scholar
[10] Hao J M, Wang J, Liu X L, Willie J P, Zhou L, Qiu M 2010 Appl. Phys. Lett. 96 251104
Google Scholar
[11] Liu N, Martin M, Thomas W, Mario H, Harald G 2010 Nano Lett. 10 2342
Google Scholar
[12] Claire M W, Liu X L, Willie J P 2012 Adv. Mater. 24 OP98
[13] Sun S L, Yang K Y, Wang C M, et al. 2012 Nano Lett. 12 6223
Google Scholar
[14] Anders P, Ole A, Ilya P R, Sergey I B 2013 Sci. Rep. 3 2155
Google Scholar
[15] Li X, Xiao S Y, Cai B G, He Q, Cui T J, Zhou L 2012 Opt. Lett. 37 4940
Google Scholar
[16] Anders P, Michael G. N, René L E, Sergey I B 2013 Nano Lett. 13 829
Google Scholar
[17] Chen L, Wei M Y, Zang X F, Zhu Y M, Zhuang S L 2016 Sci. Rep. 6 22027
Google Scholar
[18] Chen L, Xu N N, Leena S, Cui T J, Ranjan S, Zhu Y M, Zhang W L 2017 Adv. Opt. Mater. 5 1600960
Google Scholar
[19] Xu J J, Liao D G, Gupta M, Zhu Y M, Zhuang S L, Singh R, Chen L 2021 Adv. Opt. Mater. 9 2100024
[20] Miao Z Q, Wu Q, Li X, He Q, Ding K, An Z H, Zhang Y B, Zhou L 2015 Phys. Rev. X 5 041027
[21] Qu C, Ma S J, Hao J M, Qiu M, et al. 2015 Phys. Rev. Lett. 115 235503
Google Scholar
[22] Qing Y M, Ma H F, Cui T J 2018 Opt. Express 26 32442
Google Scholar
[23] Cong L Q, Pitchappa P, Lee C K, Singh R 2017 Adv. Mater. 29 1700733
Google Scholar
[24] Chen L, Ge Y F, Zang X F, et al. 2019 IEEE Trans. Terahertz Sci. Technol. 9 643
Google Scholar
[25] Yin S, Shi X T, Huang W, Zhang W T, Hu F R, Qin Z J, Xiong X M 2019 Electronics 8 1528
Google Scholar
[26] Li J S, Wu J F, Zhang L 2014 IEEE Photonics J. 6 2374591
[27] Alaee R, Farhat M, Rockstuhl C, Lederer F 2012 Opt. Express 20 28017
Google Scholar
[28] Chen L, Cao Z Q, Shen Q S, Deng X X 2007 J. Lightwave Technol. 25 539
Google Scholar
[29] Artmann K 1948 Ann. Phys. 437 87
Google Scholar
[30] Chen L, Cao Z Q, Ou F, Li H G, Shen Q S, Qiao H C 2007 Opt. Lett. 32 1432
Google Scholar
[31] Chen L, Zhu Y M, Zang X F, Cai B, Li Z, Xie L, Zhuang S L 2013 Light Sci. Appl. 2 e60
Google Scholar
下载:
计量
- 文章访问数: 6090
- PDF下载量: 137
- 被引次数: 0
