-
Radiation pressure in an optomechanical system can be used to generate various quantum phenomena. Recently, one paid more attention to the study of optical nonreciprocity in an optomechanical system, and nonreciprocal devices are indispensable for building quantum networks and ubiquitous in modern communication technology. Here in this work, we study how to realize the perfect optical nonreciprocity in a two-cavity optomechanical system with blue-detuned driving. Our calculations show that the optical nonreciprocity comes from the quantum interference of signal transmission between two possible paths corresponding to the two interactions in this system, i.e. optomechanical interaction and linearly-coupled interaction. According to the standard input-output relation of optical field in cavity optomechanics, we obtain the expression of output optical field, from which we can derive the essential conditions to achieve the perfect optical nonreciprocity, and find there are two sets of coupling strengths both of which can realize the perfect optical nonreciprocal transmission. Because the system is driven by blue-detuned driving, the system is stable only under some conditions which we can obtain according to the Routh-Hurwitz criterion. Due to the blue-detuned driving, there will be transmission gain (transmission amplitude is greater than 1) in the nonreciprocal transmission spectrum. We also find that the bandwidth of nonreciprocal transmission spectrum is in proportion to mechanical decay rate if mechanical decay rate is much less than the cavity decay rate. In other words, in a realistic optomechanical parameter regime, where mechanical decay rate is much less than cavity decay rate, the bandwidth of nonreciprocal transmission spectrum is very narrow. Our results can also be applied to other parametrically coupled three-mode bosonic systems and may be used to realize the state transfer process and optical nonreciprocal transmission in an optomechanical system.
-
Keywords:
- cavity optomechanics /
- optical nonreciprocity /
- optical isolator
[1] Jalas D, Petrov A, Eich M, et al. 2013 Nat. Photonics 7 579
Google Scholar
[2] Aplet L J, Carson J W 1964 Appl. Opt. 3 544
Google Scholar
[3] Aspelmeyer M, Kippenberg T J, Marquardt F 2014 Rev. Mod. Phys. 86 1391
Google Scholar
[4] 陈雪, 刘晓威, 张可烨, 袁春华, 张卫平 2015 64 164211
Google Scholar
Chen X, Liu X W, Zhang K Y, Yuan C H, Zhang W P 2015 Acta Phys. Sin. 64 164211
Google Scholar
[5] 陈华俊, 方贤文, 陈昌兆, 李洋 2016 65 194205
Google Scholar
Chen H J, Fang X W, Chen C Z, Li Y 2016 Acta Phys. Sin. 65 194205
Google Scholar
[6] 严晓波, 杨柳, 田雪冬, 刘一谋, 张岩 2014 63 204201
Google Scholar
Yan X B, Yang L, Tian X D, Liu Y M, Zhang Y 2014 Acta Phys. Sin. 63 204201
Google Scholar
[7] 班章, 梁静秋, 吕金光, 梁中翥, 冯思悦 2018 67 070701
Google Scholar
Zhang B, Liang J Q, Lü J G, Liang Z Z, Feng S Y 2018 Acta Phys. Sin. 67 070701
Google Scholar
[8] Chen R X, Shen L T, Yang Z B, Wu H Z, Zheng S B 2014 Phys. Rev. A 89 023843
Google Scholar
[9] Liao J Q, Wu Q Q, Nori F 2014 Phys. Rev. A 89 014302
Google Scholar
[10] Yan X B 2017 Phys. Rev. A 96 053831
Google Scholar
[11] He Q Y, Ficek Z 2014 Phys. Rev. A 89 022332
Google Scholar
[12] 张秀龙, 鲍倩倩, 杨明珠, 田雪松 2018 67 104203
Google Scholar
Zhang X L, Bao Q Q, Yang M Z, Tian X S 2018 Acta Phys. Sin. 67 104203
Google Scholar
[13] Kiesewetter S, He Q Y, Drummond P D, Reid M D 2014 Phys. Rev. A 90 043805
Google Scholar
[14] Lin Q, He B, Ghobadi R, Simon C 2014 Phys. Rev. A 90 022309
Google Scholar
[15] He Q Y, Reid M D 2013 Phys. Rev. A 88 052121
Google Scholar
[16] Yan X B, Deng Z J, Tian X D, Wu J H 2019 Opt. Express 27 024393
Google Scholar
[17] He B, Yang L, Lin Q, Xiao M 2017 Phys. Rev. Lett. 118 233604
Google Scholar
[18] Li Y, Wu L A, Wang Z D 2011 Phys. Rev. A 83 043804
Google Scholar
[19] Deng Z J, Li Y, Gao M, Wu C W 2012 Phys. Rev. A 85 025804
Google Scholar
[20] Liu Y C, Wen H Y, Wei W C, Xiao Y F 2013 Chin. Phys. B 22 114213
Google Scholar
[21] Huang S M, Agarwal G S 2009 Phys. Rev. A 79 013821
Google Scholar
[22] Agarwal G S, Huang S M 2010 Phys. Rev. A 81 041803(R)
Google Scholar
[23] Shu J 2011 Chin. Phys. Lett. 28 104203
Google Scholar
[24] 陈华俊, 米贤武 2011 60 124206
Google Scholar
Chen H J, Mi X W 2011 Acta Phys. Sin. 60 124206
Google Scholar
[25] Han Y, Cheng J, Zhou L 2011 J. Phys. B 44 165505
Google Scholar
[26] Zhang J Q, Li Y, Feng M, Xu Y 2012 Phys. Rev. A 86 053806
Google Scholar
[27] Lü X Y, Zhang W M, Ashhab S, Wu Y, Nori F 2013 Sci. Rep. 3 2943
Google Scholar
[28] Zhou L, Cheng J, Han Y, Zhang W P 2013 Phys. Rev. A 88 063854
Google Scholar
[29] He B 2012 Phys. Rev. A 85 063820
Google Scholar
[30] Cao C, Mi S C, Gao Y P, He L Y, Yang D, Wang T J, Zhang R, Wang C 2016 Sci. Rep. 6 22920
Google Scholar
[31] Cao C, Mi S C, Wang T J, Zhang R, Wang C 2016 IEEE J. Quantum Electron. 52 1
[32] Cao C, Chen X, Duan Y W, Fan L, Zhang R, Wang T J, Wang C 2017 Optik 130 659
Google Scholar
[33] Xiong X R, Gao Y P, Liu X F, Cao C, Wang T J, Wang C 2018 Sci. China-Phys. Mech. Astron. 61 090322
Google Scholar
[34] 石海泉, 谢智强, 徐勋卫, 刘念华 2018 67 044203
Google Scholar
Shi H Q, Xie Z Q, Xu X W, Liu N H 2018 Acta Phys. Sin. 67 044203
Google Scholar
[35] Manipatruni S, Robinson J T, Lipson M 2009 Phys. Rev. Lett. 102 213903
Google Scholar
[36] Hafezi M, Rabl P 2012 Opt. Express 20 7672
Google Scholar
[37] Wang Z, Shi L, Liu Y, Xu X, Zhang X 2015 Sci. Rep. 5 8657
Google Scholar
[38] Shen Z, Zhang Y L, Chen Y, Zou C L, Xiao Y F, Zou X B, Sun F W, Guo G C, Dong C H 2016 Nat. Photonics 10 657
Google Scholar
[39] Peterson G A, Lecocq F, Cicak K, Simmonds R W, Aumentado J, Teufel J D 2017 Phys. Rev. X 7 031001
[40] Barzanjeh S, Wulf M, Peruzzo M, Kalaee M, Dieterle P B, Painter O, Fink J M 2017 Nat. Commun. 8 953
Google Scholar
[41] Fang K, Luo J, Metelmann A, Matheny M H, Marquardt F, Clerk A A, Painter O 2017 Nat. Phys. 13 465
Google Scholar
[42] Maayani S, Dahan R, Kligerman Y, Moses E, Hassan A U, Jing H, Nori F, Christodoulides D N, Carmon T 2018 Nature (London)
558 569 Google Scholar
[43] Xu X W, Li Y 2015 Phys. Rev. A 91 053854
Google Scholar
[44] Xu X W, Li Y, Chen A X, Liu Y 2016 Phys. Rev. A 93 023827
Google Scholar
[45] Tian L, Li Z 2017 Phys. Rev. A 96 013808
Google Scholar
[46] Malz D, Tóth L D, Bernier N R, Feofanov A K, Kippenberg T J, Nunnenkamp A 2018 Phys. Rev. Lett. 120 023601
Google Scholar
[47] Jiang Y, Maayani S, Carmon T, Nori F, Jing H 2018 Phys. Rev. Applied 10 064037
Google Scholar
[48] Li Y, Huang Y Y, Zhang X Z, Tian L 2017 Opt. Express 25 18907
Google Scholar
[49] Huang R, Miranowicz A, Liao J Q, Nori F, Jing H 2018 Phys. Rev. Lett. 121 153601
Google Scholar
[50] Xu X W, Zhao Y J, Wang H, Jing H, Chen A X 2018 arXiv: 1809.07596
[51] Lü H, Jiang Y, Wang Y Z, Jing H 2017 Photonics Res. 5 367
Google Scholar
[52] Habraken S J M, Stannigel K, Lukin M D, Zoller P, Rabl P 2012 New J. Phys. 14 115004
Google Scholar
[53] Seif A, DeGottardi W, Esfarjani K, Hafezi M 2018 Nat. Commun. 9 1207
Google Scholar
[54] Thompson J D, Zwickl B M, Jayich A M, Marquardt F, Girvin S M, Harris J G E 2008 Nature (London)
452 72 Google Scholar
[55] Jayich A M, Sankey J C, Zwickl B M, Yang C, Thompson J D, Girvin S M, Clerk A A, Marquardt F, Harris J G E 2008 New J. Phys. 10 095008
Google Scholar
[56] Sankey J C, Yang C, Zwickl B M, Jayich A M, Harris J G E 2010 Nat. Phys. 6 707
Google Scholar
[57] Agarwal G S, Huang S 2014 New J. Phys. 16 033023
Google Scholar
[58] Yan X B, Cui C L, Gu K H, Tian X D, Fu C B, Wu J H 2014 Opt. Express 22 4886
Google Scholar
[59] DeJesus E X, Kaufman C 1987 Phys. Rev. A 35 5288
Google Scholar
-
图 1 双腔光力学系统示意图, 两光学腔通过光力相互作用与一个力学振子相耦合, 振幅为
$\varepsilon_{\rm c}$ 和$\varepsilon_{\rm d}$ ($\varepsilon_{\rm L}$ 和$\varepsilon_{\rm R}$ )的强耦合场 (探测场)分别从左右两侧驱动腔模$c_{1}$ 和$c_{2}$ , 同时两腔模之间存在线性耦合相互作用JFig. 1. A two-cavity optomechanical system with a mechanical resonator interacted with two cavities. Two strong coupling fields (probe fields) with amplitudes
$\varepsilon_{\rm c}$ and$\varepsilon_{\rm d}$ ($\varepsilon_{\rm L}$ and$\varepsilon _{\rm R}$ ) are used to drive cavity$c_{1}$ and$c_{2}$ respectively. Meanwhile, the two cavities are linearly coupled to each other with coupling strength J
图 2 传输振幅
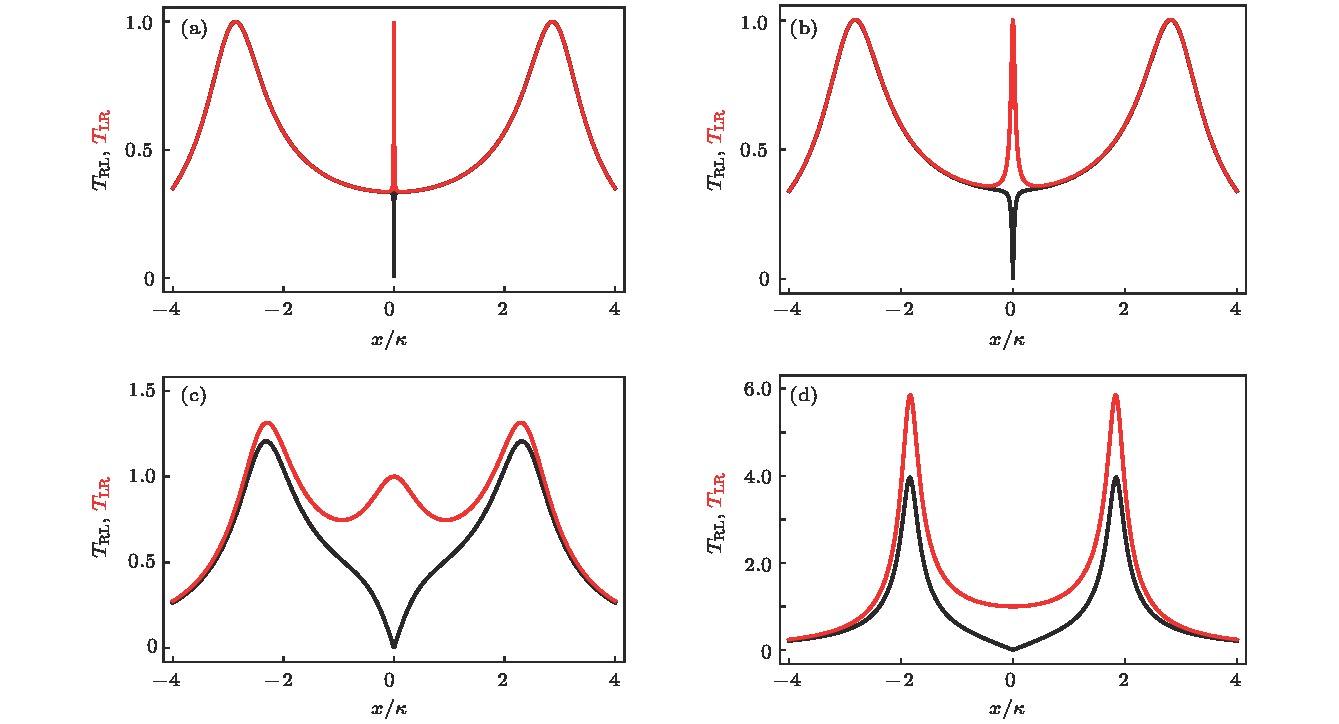
$T_{\rm {LR}}$ (红线)和$T_{\rm {RL}}$ (黑线)在不同力学振子耗散速率下随着标准化失谐$x/\kappa$ 的变化曲线 (a)$\gamma/\kappa$ =1/100; (b)$\gamma/\kappa$ =1/10; (c)$\gamma/\kappa$ =1; (d)$\gamma/\kappa$ =2; 其他参数:$\theta=-\dfrac{{\text{π}}}{2}$ ,$G =G_{+}$ 和$J=J_{+}$ (见(16)式)Fig. 2. Transmission amplitudes
$T_{\rm {LR}}$ (red line) and$T_{\rm {RL}}$ (black line) are plotted vs normalized detuning$x/\kappa$ for different cavity damping rate: (a)$\gamma/\kappa$ =1/100; (b)$\gamma/\kappa$ =1/10; (c)$\gamma/\kappa$ =1; (d)$\gamma/\kappa$ =2. Other parameters:$\theta=-\dfrac{{\text{π}}}{2}$ ,$G =G_{+}$ and$J=J_{+}$ according to Eq. (16)
图 3 传输振幅
$T_{\rm {LR}}$ (红线)和$T_{\rm {RL}}$ (黑线)在不同力学振子耗散速率下随着标准化失谐$x/\kappa$ 的变化曲线 (a)$\gamma/\kappa$ =1/100; (b)$\gamma/\kappa$ =1/10; (c)$\gamma/\kappa$ =1; (d)$\gamma/\kappa$ =10. 其他参数:$\theta=-\dfrac{{\text{π}}}{2}$ ,$G =G_{-}$ 和$J=J_{-}$ (见(16)式)Fig. 3. Transmission amplitudes
$T_{\rm {LR}}$ (red line) and$T_{\rm {RL}}$ (black line) are plotted vs normalized detuning$x/\kappa$ for different mechancial damping rate: (a)$\gamma/\kappa$ =1/100; (b)$\gamma/\kappa$ =1/10; (c)$\gamma/\kappa$ =1; (d)$\gamma/\kappa$ =10. Other parameters:$\theta=-\dfrac{{\text{π}}}{2}$ ,$G =G_{-}$ and$J=J_{-}$ according to Eq. (16)
图 4 传输振幅
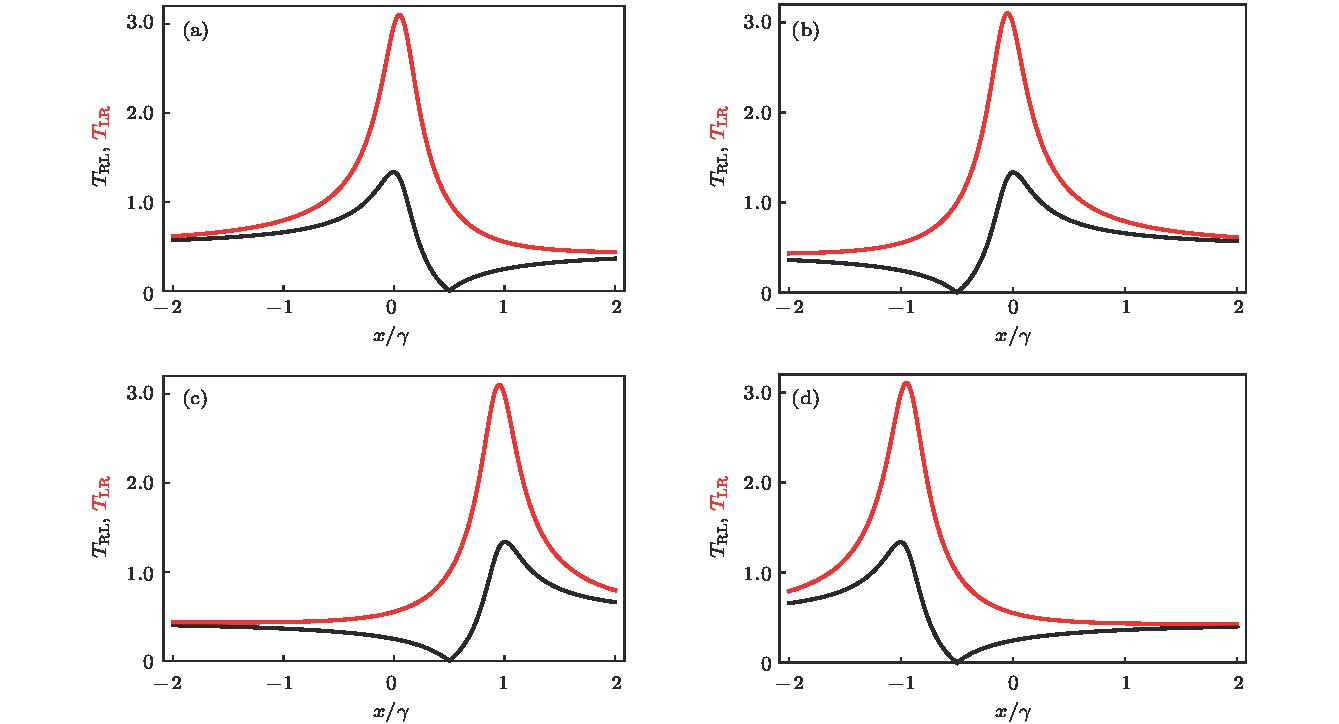
$T_{\rm {LR}}$ (红线)和$T_{\rm {RL}}$ (黑线)在不同非互易相位差θ和耦合强度G时随着标准化失谐$x/\gamma$ 的变化曲线 (a)$\theta=-\dfrac{{\text{π}}}{4}$ 和$G=G_{-}$ ; (b)$\theta=-\dfrac{{\text{π}}}{4}$ 和$G=G_{+}$ ; (c)$\theta=-\dfrac{3{\text{π}}}{4}$ 和$G=G_{-}$ ; (d)$\theta=-\dfrac{3{\text{π}}}{4}$ 和$G=G_{+}$ ; 其他参数:$\gamma/\kappa=10^{-3}$ ,$J=J_{\pm}$ 和$G=G_{\pm}$ (见(19)式)Fig. 4. Transmission amplitudes
$T_{\rm {LR}}$ (red line) and$T_{\rm {RL}}$ (black line) are plotted vs normalized detuning$x/\gamma$ for different nonreciprocal phase θ and coupling strength G: (a)$\theta=-\dfrac{{\text{π}}}{4}$ and$G=G_{-}$ ; (b)$\theta=-\dfrac{{\text{π}}}{4}$ and$G=G_{+}$ ; (c)$\theta=-\dfrac{3{\text{π}}}{4}$ and$G=G_{-}$ ; (d)$\theta=-\dfrac{3{\text{π}}}{4}$ and$G=G_{+}$ . Other parameters:$\gamma/\kappa=10^{-3}$ , coupling strengths$J=J_{\pm}$ and$G=G_{\pm}$ according to Eq. (19) -
[1] Jalas D, Petrov A, Eich M, et al. 2013 Nat. Photonics 7 579
Google Scholar
[2] Aplet L J, Carson J W 1964 Appl. Opt. 3 544
Google Scholar
[3] Aspelmeyer M, Kippenberg T J, Marquardt F 2014 Rev. Mod. Phys. 86 1391
Google Scholar
[4] 陈雪, 刘晓威, 张可烨, 袁春华, 张卫平 2015 64 164211
Google Scholar
Chen X, Liu X W, Zhang K Y, Yuan C H, Zhang W P 2015 Acta Phys. Sin. 64 164211
Google Scholar
[5] 陈华俊, 方贤文, 陈昌兆, 李洋 2016 65 194205
Google Scholar
Chen H J, Fang X W, Chen C Z, Li Y 2016 Acta Phys. Sin. 65 194205
Google Scholar
[6] 严晓波, 杨柳, 田雪冬, 刘一谋, 张岩 2014 63 204201
Google Scholar
Yan X B, Yang L, Tian X D, Liu Y M, Zhang Y 2014 Acta Phys. Sin. 63 204201
Google Scholar
[7] 班章, 梁静秋, 吕金光, 梁中翥, 冯思悦 2018 67 070701
Google Scholar
Zhang B, Liang J Q, Lü J G, Liang Z Z, Feng S Y 2018 Acta Phys. Sin. 67 070701
Google Scholar
[8] Chen R X, Shen L T, Yang Z B, Wu H Z, Zheng S B 2014 Phys. Rev. A 89 023843
Google Scholar
[9] Liao J Q, Wu Q Q, Nori F 2014 Phys. Rev. A 89 014302
Google Scholar
[10] Yan X B 2017 Phys. Rev. A 96 053831
Google Scholar
[11] He Q Y, Ficek Z 2014 Phys. Rev. A 89 022332
Google Scholar
[12] 张秀龙, 鲍倩倩, 杨明珠, 田雪松 2018 67 104203
Google Scholar
Zhang X L, Bao Q Q, Yang M Z, Tian X S 2018 Acta Phys. Sin. 67 104203
Google Scholar
[13] Kiesewetter S, He Q Y, Drummond P D, Reid M D 2014 Phys. Rev. A 90 043805
Google Scholar
[14] Lin Q, He B, Ghobadi R, Simon C 2014 Phys. Rev. A 90 022309
Google Scholar
[15] He Q Y, Reid M D 2013 Phys. Rev. A 88 052121
Google Scholar
[16] Yan X B, Deng Z J, Tian X D, Wu J H 2019 Opt. Express 27 024393
Google Scholar
[17] He B, Yang L, Lin Q, Xiao M 2017 Phys. Rev. Lett. 118 233604
Google Scholar
[18] Li Y, Wu L A, Wang Z D 2011 Phys. Rev. A 83 043804
Google Scholar
[19] Deng Z J, Li Y, Gao M, Wu C W 2012 Phys. Rev. A 85 025804
Google Scholar
[20] Liu Y C, Wen H Y, Wei W C, Xiao Y F 2013 Chin. Phys. B 22 114213
Google Scholar
[21] Huang S M, Agarwal G S 2009 Phys. Rev. A 79 013821
Google Scholar
[22] Agarwal G S, Huang S M 2010 Phys. Rev. A 81 041803(R)
Google Scholar
[23] Shu J 2011 Chin. Phys. Lett. 28 104203
Google Scholar
[24] 陈华俊, 米贤武 2011 60 124206
Google Scholar
Chen H J, Mi X W 2011 Acta Phys. Sin. 60 124206
Google Scholar
[25] Han Y, Cheng J, Zhou L 2011 J. Phys. B 44 165505
Google Scholar
[26] Zhang J Q, Li Y, Feng M, Xu Y 2012 Phys. Rev. A 86 053806
Google Scholar
[27] Lü X Y, Zhang W M, Ashhab S, Wu Y, Nori F 2013 Sci. Rep. 3 2943
Google Scholar
[28] Zhou L, Cheng J, Han Y, Zhang W P 2013 Phys. Rev. A 88 063854
Google Scholar
[29] He B 2012 Phys. Rev. A 85 063820
Google Scholar
[30] Cao C, Mi S C, Gao Y P, He L Y, Yang D, Wang T J, Zhang R, Wang C 2016 Sci. Rep. 6 22920
Google Scholar
[31] Cao C, Mi S C, Wang T J, Zhang R, Wang C 2016 IEEE J. Quantum Electron. 52 1
[32] Cao C, Chen X, Duan Y W, Fan L, Zhang R, Wang T J, Wang C 2017 Optik 130 659
Google Scholar
[33] Xiong X R, Gao Y P, Liu X F, Cao C, Wang T J, Wang C 2018 Sci. China-Phys. Mech. Astron. 61 090322
Google Scholar
[34] 石海泉, 谢智强, 徐勋卫, 刘念华 2018 67 044203
Google Scholar
Shi H Q, Xie Z Q, Xu X W, Liu N H 2018 Acta Phys. Sin. 67 044203
Google Scholar
[35] Manipatruni S, Robinson J T, Lipson M 2009 Phys. Rev. Lett. 102 213903
Google Scholar
[36] Hafezi M, Rabl P 2012 Opt. Express 20 7672
Google Scholar
[37] Wang Z, Shi L, Liu Y, Xu X, Zhang X 2015 Sci. Rep. 5 8657
Google Scholar
[38] Shen Z, Zhang Y L, Chen Y, Zou C L, Xiao Y F, Zou X B, Sun F W, Guo G C, Dong C H 2016 Nat. Photonics 10 657
Google Scholar
[39] Peterson G A, Lecocq F, Cicak K, Simmonds R W, Aumentado J, Teufel J D 2017 Phys. Rev. X 7 031001
[40] Barzanjeh S, Wulf M, Peruzzo M, Kalaee M, Dieterle P B, Painter O, Fink J M 2017 Nat. Commun. 8 953
Google Scholar
[41] Fang K, Luo J, Metelmann A, Matheny M H, Marquardt F, Clerk A A, Painter O 2017 Nat. Phys. 13 465
Google Scholar
[42] Maayani S, Dahan R, Kligerman Y, Moses E, Hassan A U, Jing H, Nori F, Christodoulides D N, Carmon T 2018 Nature (London)
558 569 Google Scholar
[43] Xu X W, Li Y 2015 Phys. Rev. A 91 053854
Google Scholar
[44] Xu X W, Li Y, Chen A X, Liu Y 2016 Phys. Rev. A 93 023827
Google Scholar
[45] Tian L, Li Z 2017 Phys. Rev. A 96 013808
Google Scholar
[46] Malz D, Tóth L D, Bernier N R, Feofanov A K, Kippenberg T J, Nunnenkamp A 2018 Phys. Rev. Lett. 120 023601
Google Scholar
[47] Jiang Y, Maayani S, Carmon T, Nori F, Jing H 2018 Phys. Rev. Applied 10 064037
Google Scholar
[48] Li Y, Huang Y Y, Zhang X Z, Tian L 2017 Opt. Express 25 18907
Google Scholar
[49] Huang R, Miranowicz A, Liao J Q, Nori F, Jing H 2018 Phys. Rev. Lett. 121 153601
Google Scholar
[50] Xu X W, Zhao Y J, Wang H, Jing H, Chen A X 2018 arXiv: 1809.07596
[51] Lü H, Jiang Y, Wang Y Z, Jing H 2017 Photonics Res. 5 367
Google Scholar
[52] Habraken S J M, Stannigel K, Lukin M D, Zoller P, Rabl P 2012 New J. Phys. 14 115004
Google Scholar
[53] Seif A, DeGottardi W, Esfarjani K, Hafezi M 2018 Nat. Commun. 9 1207
Google Scholar
[54] Thompson J D, Zwickl B M, Jayich A M, Marquardt F, Girvin S M, Harris J G E 2008 Nature (London)
452 72 Google Scholar
[55] Jayich A M, Sankey J C, Zwickl B M, Yang C, Thompson J D, Girvin S M, Clerk A A, Marquardt F, Harris J G E 2008 New J. Phys. 10 095008
Google Scholar
[56] Sankey J C, Yang C, Zwickl B M, Jayich A M, Harris J G E 2010 Nat. Phys. 6 707
Google Scholar
[57] Agarwal G S, Huang S 2014 New J. Phys. 16 033023
Google Scholar
[58] Yan X B, Cui C L, Gu K H, Tian X D, Fu C B, Wu J H 2014 Opt. Express 22 4886
Google Scholar
[59] DeJesus E X, Kaufman C 1987 Phys. Rev. A 35 5288
Google Scholar
下载:
计量
- 文章访问数: 9217
- PDF下载量: 159
- 被引次数: 0
