-
光学非互易器件是光学系统中重要的组成部分, 例如光隔离器. 为了提高这类器件的隔离特性, 提出一种双腔双光力系统, 系统以两种大小不同的光力耦合强度分别与两光学腔相耦合. 该系统在红失谐场的驱动下, 通过调控相位差既可以实现光非互易现象, 又能决定光在系统中透过与隔离的方向, 这种性质来源于光力耦合相互作用和光学腔模耦合之间的量子干涉效应. 研究过程中把系统中相关算符用其平均值加各自的相对涨落值来表示, 然后由涨落值利用输入-输出关系得到系统的传输振幅, 进而得到系统隔离率. 讨论了系统隔离率随光力耦合强度变化的分布情况, 发现两个力学振子的共同作用能使系统有更高的容错率. 该系统通过选用一个品质因数更高的力学振子, 能够实现两种特定频率光的较大隔离率, 并且同时能够保证共振频率信号的反向传输 .
Optical non-reciprocal devices such as the isolators are quite important components in optical systems. To realize the non-reciprocal transmission of the light, the Lorenz reciprocity theorem must be broken first and the main method is that Faraday magnetic rotation effect is used to change the polarization state of the signal through magneto-optical materials. However, this method is difficult to achieve on-chip integration. So using optomechanical system is presented to overcome the difficulty. In order to improve the isolation characteristics of the device, a double-cavity double-optomechanical system, which is coupled to two optical modes by two mechanical oscillators with two different optomechanical coupling strengths, is proposed. Driven by the red detuning field in such a system, the non-reciprocal phenomenon can be realized by regulating the phase difference, and the direction of light transmission and isolation can be determined as well. This property is determined by the quantum interference effect between the optomechanical coupling strengths and the couplings of the optical cavity modes. The method is that the relative operators are represented by their average value plus their relative fluctuations, and then according to the input-output relationship the transmission amplitude and the isolation rate are obtained. We mainly discuss the distribution of the isolation rate as a function of the optomechanical coupling strength. The results are that the combined action of two mechanical modes can make the system have higher fault tolerance rate. The other mechanical mode can make the system achieve a large isolation rate at two specific frequencies and the reverse transmission in the resonant frequency signals at the same time. -
Keywords:
- double-cavity double-optomechanical system /
- nonreciprocal property /
- transmission amplitude /
- isolation rate
[1] Xia C C, Yan X B, Tian X D, Gao F 2019 Opt. Commun. 451 197
Google Scholar
[2] 朱雪松, 刘星雨, 张岩 2022 71 150701
Google Scholar
Zhu X S, Liu X Y, Zhang Y 2022 Acta Phys. Sin. 71 150701
Google Scholar
[3] Jing Y W 2022 Sci. Rep. 12 5844
Google Scholar
[4] Jiang C, Song L N, Li Y 2018 Phys. Rev. A 97 053812
Google Scholar
[5] Asadchy V S, Mirmoosa M S, Diaz-Rubio A, Fan S, Tretyakov S A 2020 Proc. IEEE 108 1684
Google Scholar
[6] Xia K Y, Lu G W, Lin G W, Cheng Y Q, Niu Y P, Gong S Q, Twamley J 2014 Phys. Rev. A 90 043802
Google Scholar
[7] Scheucher M, Hilico A, Will E, Volz J, Rauschenbeutel A 2016 Science 354 1577
Google Scholar
[8] Tang J S, Nie W, Tang L, Chen M Y, Su X, Lu Y Q, Nori F, Xia K Y 2022 Phys. Rev. Lett. 128 203602
Google Scholar
[9] Hu Y Q, Qi Y H, You Y, Zhang S C, Lin G W, Li X L, Gong J B, Gong S Q, Niu Y P 2021 Phys. Rev. Appl. 16 014046
Google Scholar
[10] Peterson C W, Benalcazar W A, Lin M, Hughes T L, Bahl G 2019 Phys. Rev. Lett. 123 063901
Google Scholar
[11] Hu X X, Wang Z B, Zhang P F, Chen G J, Zhang Y L, Li G, Zou X B, Zhang T C, Tang H X, Dong C H, Guo G C, Zou C L 2021 Nat. Commun. 12 2389
Google Scholar
[12] Liang C, Liu B, Xu A N, Wen X, Lu C C, Xia K Y, Tey M K, Liu Y C, You L 2020 Phys. Rev. Lett. 125 123901
Google Scholar
[13] Huang X Y, Lu C C, Liang C, Tao H G, Liu Y C 2021 Light Sci. Appl. 10 30
Google Scholar
[14] Tang L, Tang J S, Chen M Y, Nori F, Xiao M, Xia K Y 2022 Phys. Rev. Lett. 128 083604
Google Scholar
[15] Barzanjeh S, Wulf M, Peruzzo M, Kalaee M, Dieterle P B, Painter O, Fink J M 2017 Nat. Commun. 8 953
Google Scholar
[16] Peterson G A, Lecocq F, Cicak K, Simmonds R W, Aumentado J, Teufel J D 2017 Phys. Rev. X 7 031001
Google Scholar
[17] Tang J D, Cai Q Z, Cheng Z D, Xu N, Peng G Y, Chen P Q, Wang D G, Xia Z W, Wang Y, Song H Z, Zhou Q, Deng G W 2022 Phys. Lett. A 429 127966
Google Scholar
[18] 刘妮, 王建芬, 梁九卿 2020 69 064202
Google Scholar
Liu N, Wang J F, Liang J Q 2020 Acta Phys. Sin. 69 064202
Google Scholar
[19] Jiang C, Liu Y L, Sillanpaa M A 2021 Phys. Rev. A 104 013502
Google Scholar
[20] Xiao R J, Pan G X, Xiu X M 2021 Chin. Phys. B 30 034209
Google Scholar
[21] Yan K X, Zhang Y C, Cui Y S, Jiang C 2020 Opt. Commun. 475 126249
Google Scholar
[22] Qu K N, Agarwal G S 2013 Phys. Rev. A 87 63813
Google Scholar
[23] He Y 2016 Phys. Rev. A 94 063804
Google Scholar
[24] Singh S K, Parvez M, Abbas T, Peng J X, Mazaheri M, Asjad M 2022 Phys. Lett. A 442 128181
Google Scholar
[25] Xu X W, Li Y 2015 Phys. Rev. A 91 053854
Google Scholar
[26] DeJesus E X, Kaufman C 1987 Phys. Rev. A 35 5288
Google Scholar
[27] Weis S, Riviere R, Deleglise S, Gavartin E, Arcizet O, Schliesser A, Kippenberg T J 2010 Science 330 1520
Google Scholar
[28] Ullah K 2019 Chin. Phys. B 28 114209
Google Scholar
[29] Chen Y T, Du L, Liu Y M, Zhang Y 2020 Opt. Express 28 7095
Google Scholar
[30] 张利巍, 李贤丽, 杨柳 2019 68 170701
Google Scholar
Zhang L W, Li X L, Yang L 2019 Acta Phys. Sin. 68 170701
Google Scholar
-
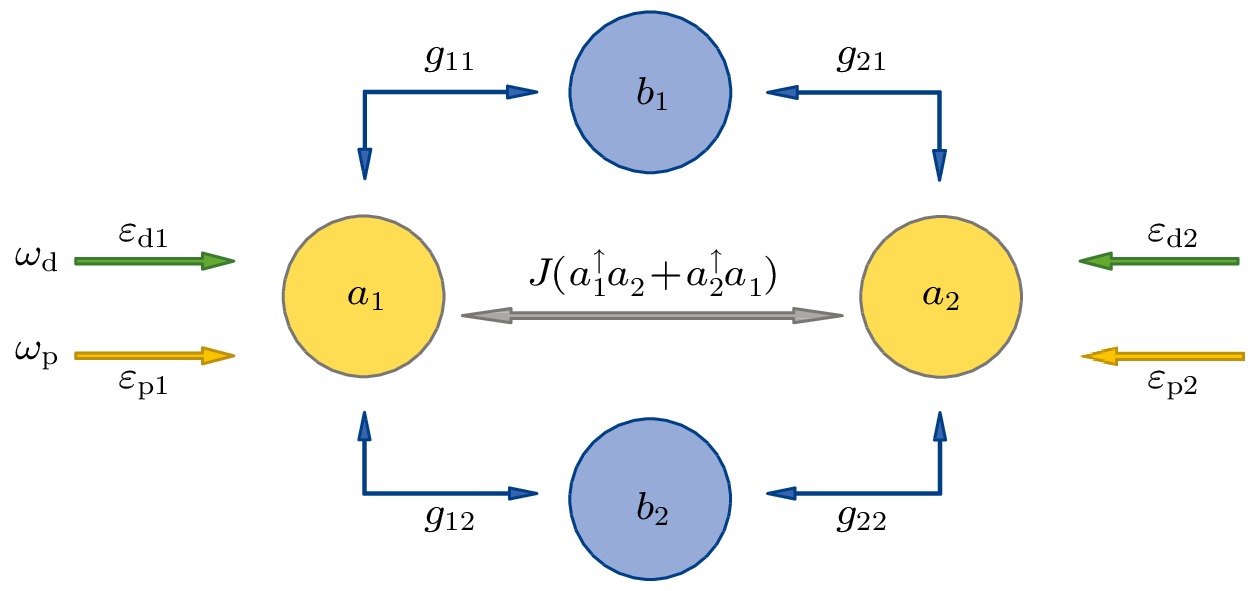
图 1 双腔双光力系统示意图, 其中光学腔
${a_i}$ 通过光力耦合相互作用${g_{ij}}$ 与力学振子${b_j}$ 相耦合, 同时两光学腔之间存在腔模线性耦合相互作用, 腔模两侧存在振幅为${\varepsilon _{{\text{d}}i}}$ 的驱动场和${\varepsilon _{{\text{p}}i}}$ 的探测场Fig. 1. Diagram of double-cavity dual-optomechanical system. The optical cavity
${a_i}$ is coupled to the mechanical oscillator${b_j}$ by the optomechanical coupling interaction$ {g}_{ij}$ . And there is a cavity mode linear coupling interaction between two optical cavities, and there are the coupling fields with amplitude${\varepsilon _{{\text{d}}i}}$ and the probe fields with amplitude${\varepsilon _{{\text{p}}i}}$ on both sides of the cavity modes.
图 2
${x / \kappa } = 0$ 时, 传输振幅T随相位差α的变化 (其他参数:${J/\kappa } = C = 1$ )Fig. 2. Transmission amplitudes T at
${x / \kappa } = 0$ are plotted against the phase difference α (Other parameters:${J/\kappa } = $ $ C = 1$ ).
图 3 单个力学振子b1影响下, 不同方向输入场
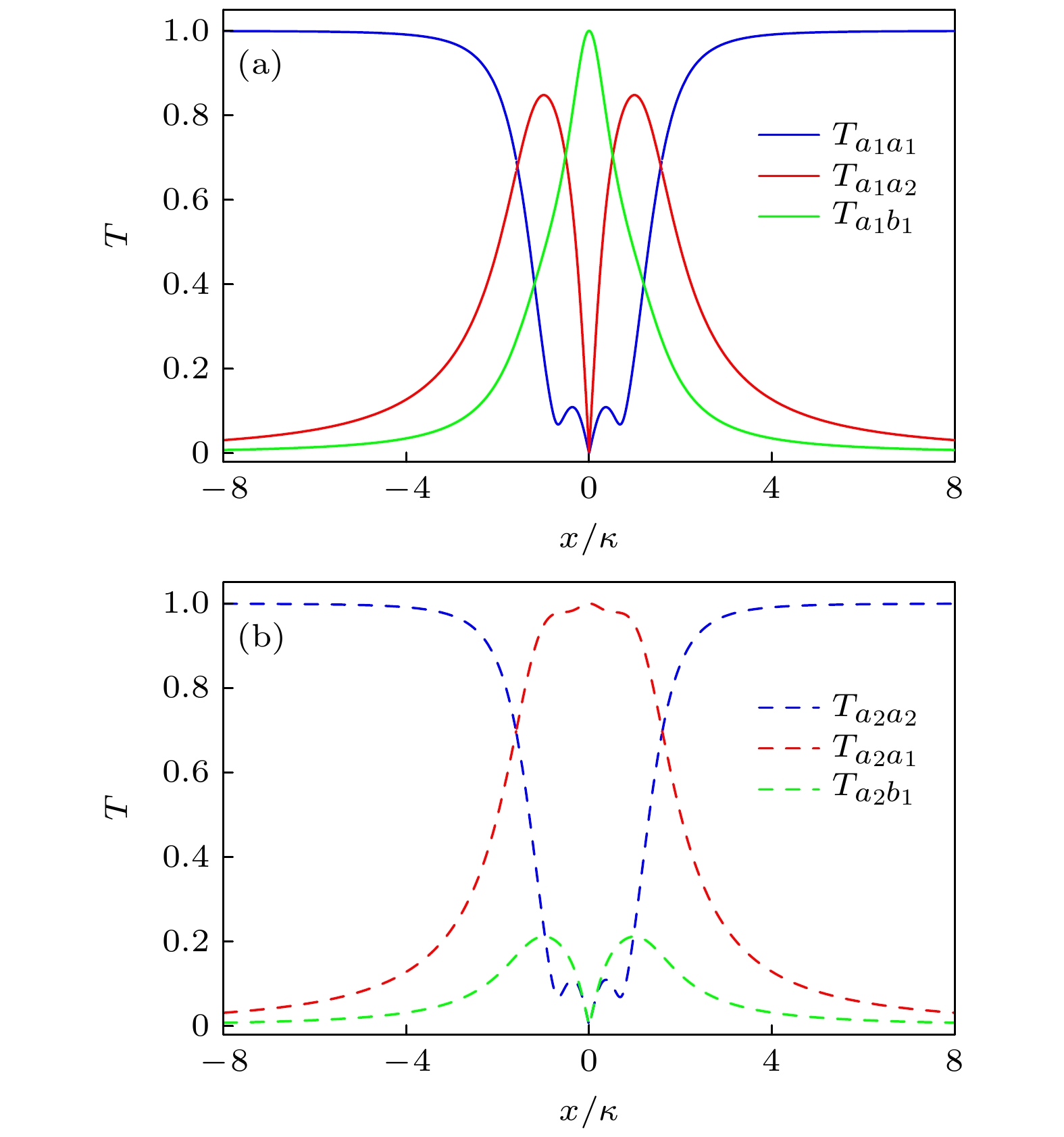
${\varepsilon _{{\text{p}}1}}$ (a)和${\varepsilon _{{\text{p}}2}}$ (b)的传输振幅T随标准化失谐$x/\kappa $ 的变化 (其他参数:$\alpha = {{\text{π }} \mathord{\left/ {\vphantom {{\text{π }} 2}} \right. } 2}$ ,${J \mathord{\left/ {\vphantom {J \kappa }} \right. } \kappa } = 1$ ,${{{\gamma _1}} \mathord{\left/ {\vphantom {{{\gamma _1}} \kappa }} \right. } \kappa } = 0.25$ ,${G_2} = 0$ ,${{{G_1}}/ \kappa } = 0.5$ )Fig. 3. Transmission amplitude T of input fields
${\varepsilon _{{\text{p}}1}}$ (a) and${\varepsilon _{{\text{p}}2}}$ (b) in different directions as a function of normalized detuning$x/\kappa $ for only a single mechanical oscillator b1 (Other parameters:$\alpha = {{\text{π }}/2}$ ,${J/ \kappa } = 1$ ,${{{\gamma _1}} / \kappa } = 0.25$ ,${G_2} = 0$ ,${{{G_1}} / \kappa } = 0.5$ )
图 4 x = 0时, 单向隔离率I 随有效光力耦合强度
${G_1}$ 的变化 (给定的参数:$\alpha = {{\text{π }}/ 2}$ ,${J/ \kappa } = 1$ ,${G_2} = 0$ ,${{{\gamma _1}}/ \kappa } = $ $ 0.25$ )Fig. 4. Unidirectional isolation rate I at x = 0 versus effective optomechanical coupling strengths
${G_1}$ (The given parameters:$\alpha = {{\text{π }}/ 2}$ ,${J / \kappa } = 1$ ,${G_2} = 0$ ,${{{\gamma _1}} / \kappa } = 0.25$ )
图 5 x = 0时, 单向隔离率I随有效光力耦合强度
${G_1}$ 和${G_2}$ 的变化 (给定的参数:$\alpha = {{\text{π }}/ 2}$ ,${J/\kappa } = 1$ ,${{{\gamma _1}}/ \kappa } = 0.25$ ,${{{\gamma _2}}/ \kappa } = 9$ )Fig. 5. Unidirectional isolation rate I at x = 0 versus the effective optomechanical coupling strengths G1 and G2 (The given parameters:
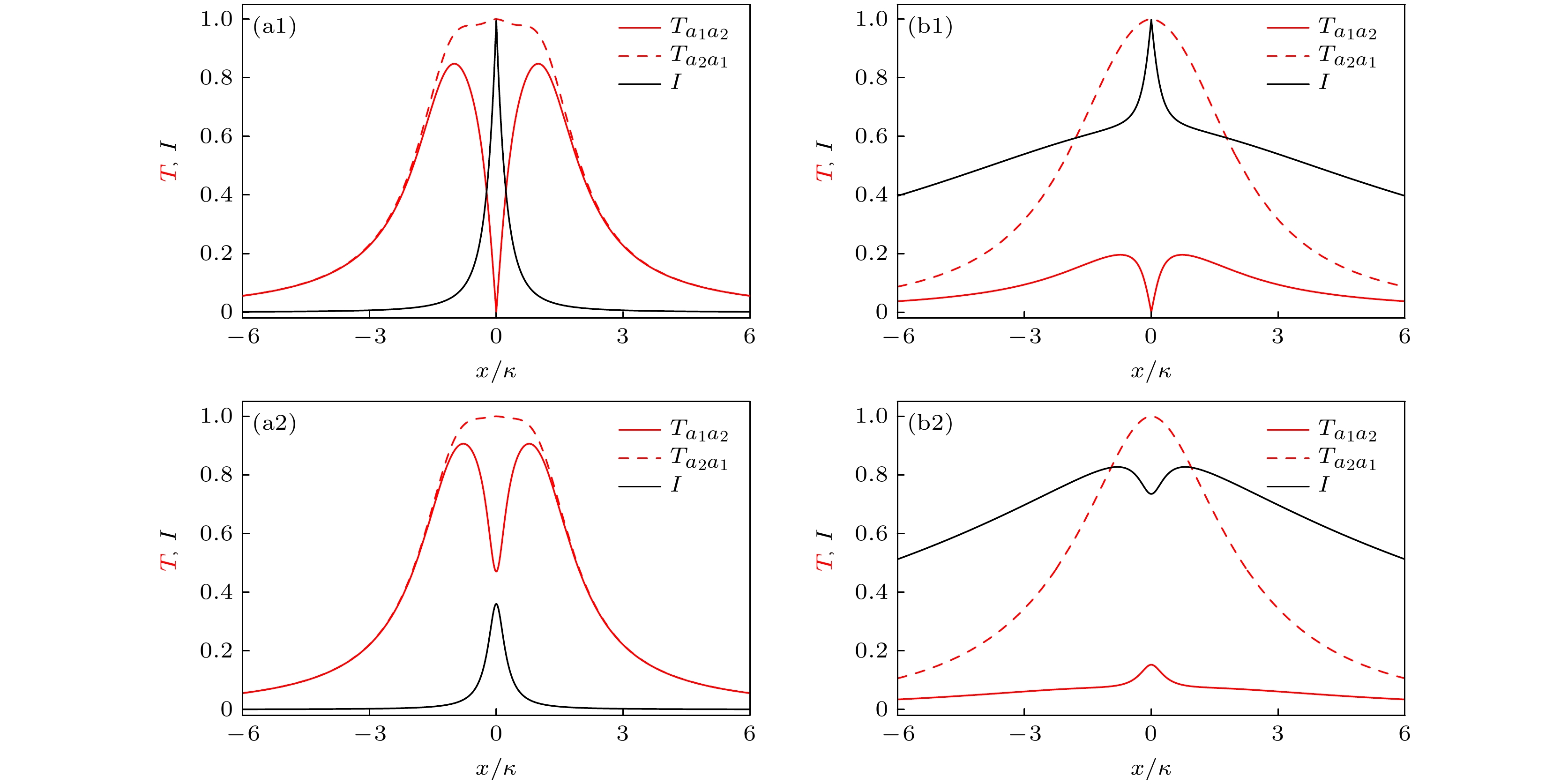
$\alpha = {{\text{π }}/ 2}$ ,${J/ \kappa } = 1$ ,${{{\gamma _1}}/\kappa } = 0.25$ ,${{{\gamma _2}}/\kappa } = $ $ 9$ ).
图 6 透射振幅T和对应的隔离率I随标准化失谐
$x/\kappa $ 的变化 (其他参数与图5相同) (a1) G2 = 0, G1/κ = 0.5; (a2) G2 = 0, G1/κ = 0.3; (b1) G1/κ = 0.3, G2/κ = 2.4; (b2) G1/κ = 0.3, G2/κ = 3.0Fig. 6. Transmission amplitudes T and the corresponding isolation rate I versus normalized detuning
$x/\kappa $ (The given parameters are the same as the ones in Fig. 5): (a1) G1/κ = 0.5; (a2) G1/κ = 0.3; (b1) G2/κ = 2.4; (b2) G2/κ = 3.0.
图 7 透射振幅T和对应的隔离率I随标准化失谐
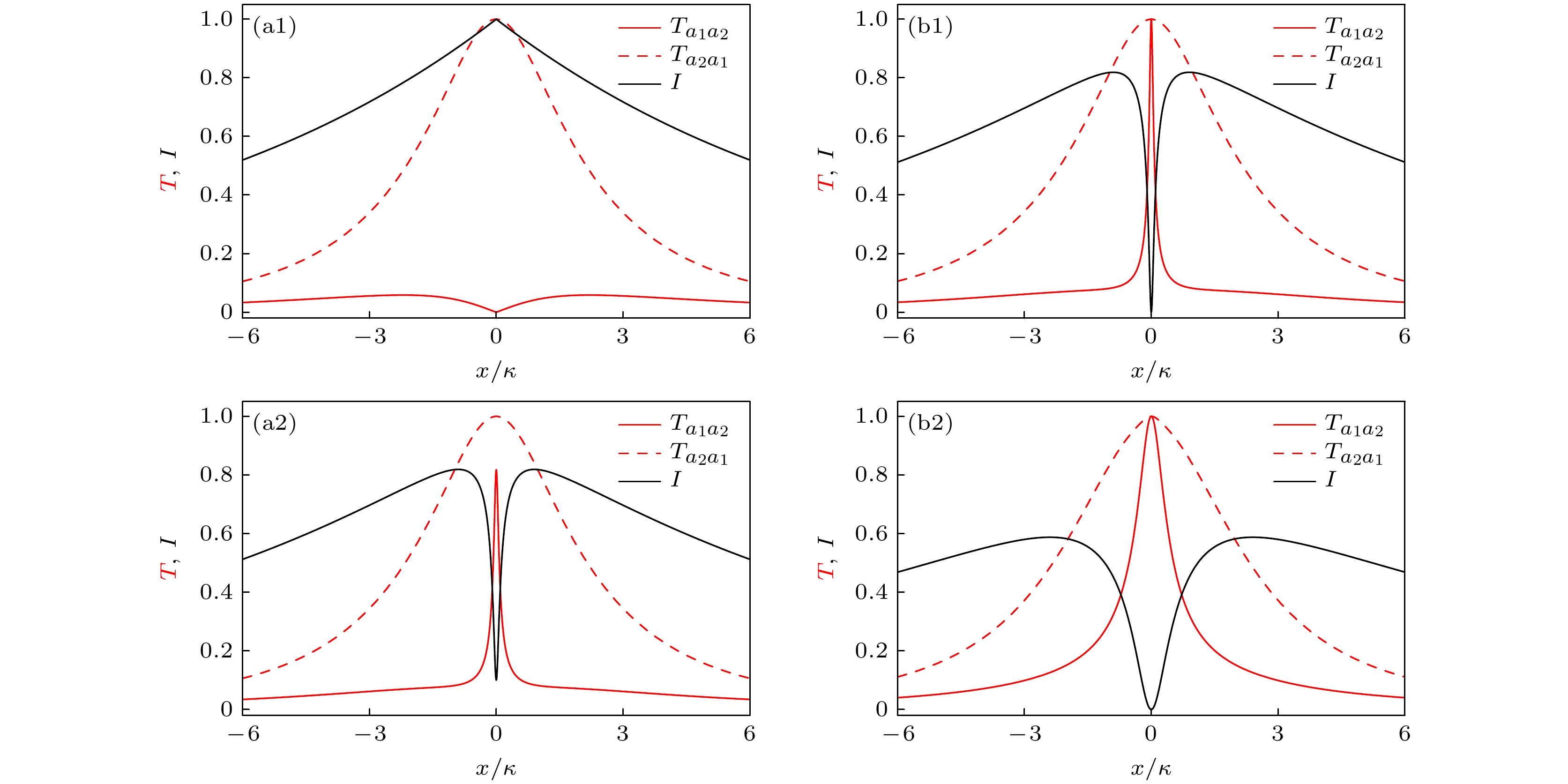
$x/\kappa $ 的变化 (其他参数:$\alpha = {{\text{π }} / 2}$ ,${J /\kappa } = 1$ ,${{{\gamma _2}}/ \kappa } = 9$ ,${{{G_2}} / \kappa } = 3$ ) (a1) γ1/κ = 0.01, G1/κ = 0; (a2) γ1/κ = 0.01, G1/κ = 0.3; (b1) γ1 = 0, G1/κ = 0.3; (b2) γ1 = 0, G1/κ = 0.8Fig. 7. Transmission amplitudes T and the corresponding isolation rate I versus normalized detuning
$x/\kappa $ (The other parameters are$\alpha = {{\text{π }}/ 2}$ ,${J / \kappa } = 1$ ,${{{\gamma _2}}/ \kappa } = 9$ ,${{{G_2}} / \kappa } = 3$ ): (a1) γ1/κ = 0.01, G1/κ = 0; (a2) γ1/κ = 0.01, G1/κ = 0.3; (b1) γ1 = 0, G1/κ = 0.3; (b2) γ1 = 0, G1/κ = 0.8.
图 8 隔离率I随有效光力耦合强度
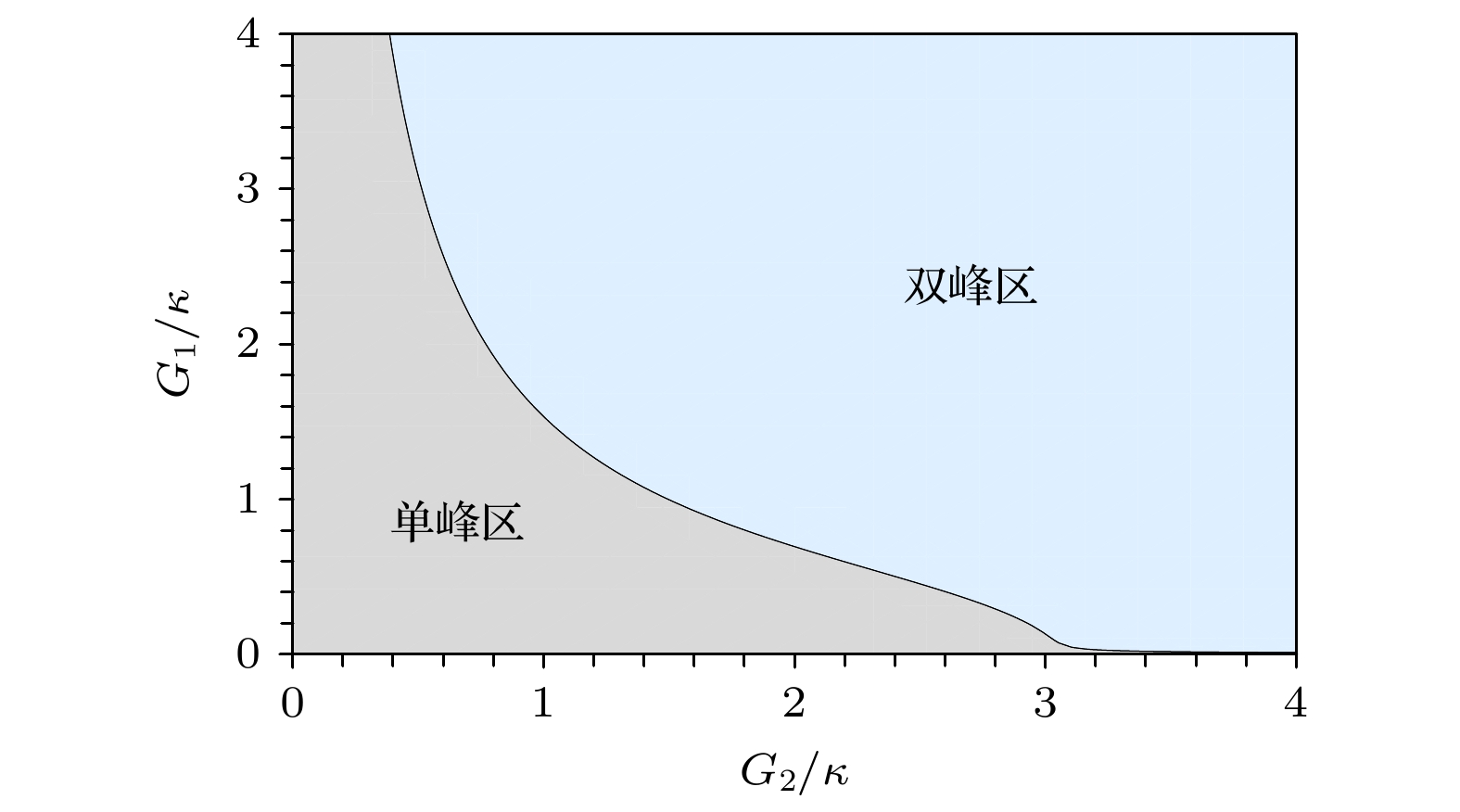
${{{G_1}}/\kappa }$ 和${{{G_2}} / \kappa }$ 的单峰区及双峰区 (其他参数与图5相同)Fig. 8. Single peak region and double peak region of the isolation rate I versus the effective optomechanical coupling strengths
${{{G_1}} / \kappa }$ and${{{G_2}} / \kappa }$ (Other parameters are the same as the ones in Fig. 5). -
[1] Xia C C, Yan X B, Tian X D, Gao F 2019 Opt. Commun. 451 197
Google Scholar
[2] 朱雪松, 刘星雨, 张岩 2022 71 150701
Google Scholar
Zhu X S, Liu X Y, Zhang Y 2022 Acta Phys. Sin. 71 150701
Google Scholar
[3] Jing Y W 2022 Sci. Rep. 12 5844
Google Scholar
[4] Jiang C, Song L N, Li Y 2018 Phys. Rev. A 97 053812
Google Scholar
[5] Asadchy V S, Mirmoosa M S, Diaz-Rubio A, Fan S, Tretyakov S A 2020 Proc. IEEE 108 1684
Google Scholar
[6] Xia K Y, Lu G W, Lin G W, Cheng Y Q, Niu Y P, Gong S Q, Twamley J 2014 Phys. Rev. A 90 043802
Google Scholar
[7] Scheucher M, Hilico A, Will E, Volz J, Rauschenbeutel A 2016 Science 354 1577
Google Scholar
[8] Tang J S, Nie W, Tang L, Chen M Y, Su X, Lu Y Q, Nori F, Xia K Y 2022 Phys. Rev. Lett. 128 203602
Google Scholar
[9] Hu Y Q, Qi Y H, You Y, Zhang S C, Lin G W, Li X L, Gong J B, Gong S Q, Niu Y P 2021 Phys. Rev. Appl. 16 014046
Google Scholar
[10] Peterson C W, Benalcazar W A, Lin M, Hughes T L, Bahl G 2019 Phys. Rev. Lett. 123 063901
Google Scholar
[11] Hu X X, Wang Z B, Zhang P F, Chen G J, Zhang Y L, Li G, Zou X B, Zhang T C, Tang H X, Dong C H, Guo G C, Zou C L 2021 Nat. Commun. 12 2389
Google Scholar
[12] Liang C, Liu B, Xu A N, Wen X, Lu C C, Xia K Y, Tey M K, Liu Y C, You L 2020 Phys. Rev. Lett. 125 123901
Google Scholar
[13] Huang X Y, Lu C C, Liang C, Tao H G, Liu Y C 2021 Light Sci. Appl. 10 30
Google Scholar
[14] Tang L, Tang J S, Chen M Y, Nori F, Xiao M, Xia K Y 2022 Phys. Rev. Lett. 128 083604
Google Scholar
[15] Barzanjeh S, Wulf M, Peruzzo M, Kalaee M, Dieterle P B, Painter O, Fink J M 2017 Nat. Commun. 8 953
Google Scholar
[16] Peterson G A, Lecocq F, Cicak K, Simmonds R W, Aumentado J, Teufel J D 2017 Phys. Rev. X 7 031001
Google Scholar
[17] Tang J D, Cai Q Z, Cheng Z D, Xu N, Peng G Y, Chen P Q, Wang D G, Xia Z W, Wang Y, Song H Z, Zhou Q, Deng G W 2022 Phys. Lett. A 429 127966
Google Scholar
[18] 刘妮, 王建芬, 梁九卿 2020 69 064202
Google Scholar
Liu N, Wang J F, Liang J Q 2020 Acta Phys. Sin. 69 064202
Google Scholar
[19] Jiang C, Liu Y L, Sillanpaa M A 2021 Phys. Rev. A 104 013502
Google Scholar
[20] Xiao R J, Pan G X, Xiu X M 2021 Chin. Phys. B 30 034209
Google Scholar
[21] Yan K X, Zhang Y C, Cui Y S, Jiang C 2020 Opt. Commun. 475 126249
Google Scholar
[22] Qu K N, Agarwal G S 2013 Phys. Rev. A 87 63813
Google Scholar
[23] He Y 2016 Phys. Rev. A 94 063804
Google Scholar
[24] Singh S K, Parvez M, Abbas T, Peng J X, Mazaheri M, Asjad M 2022 Phys. Lett. A 442 128181
Google Scholar
[25] Xu X W, Li Y 2015 Phys. Rev. A 91 053854
Google Scholar
[26] DeJesus E X, Kaufman C 1987 Phys. Rev. A 35 5288
Google Scholar
[27] Weis S, Riviere R, Deleglise S, Gavartin E, Arcizet O, Schliesser A, Kippenberg T J 2010 Science 330 1520
Google Scholar
[28] Ullah K 2019 Chin. Phys. B 28 114209
Google Scholar
[29] Chen Y T, Du L, Liu Y M, Zhang Y 2020 Opt. Express 28 7095
Google Scholar
[30] 张利巍, 李贤丽, 杨柳 2019 68 170701
Google Scholar
Zhang L W, Li X L, Yang L 2019 Acta Phys. Sin. 68 170701
Google Scholar
下载:
计量
- 文章访问数: 3694
- PDF下载量: 135
- 被引次数: 0
