Multifractal state and mobility edges in a periodically driven non-reciprocal Aubry-André model

WANG Yujia; XU Zhihao

doi:10.7498/aps.74.20241633

Abstract

In this work, we investigate the delocalization-localization transition of Floquet eigenstates in a driven chain with an incommensurate Aubry-André (AA) on-site potential and a small non-reciprocal hopping term that is driven periodically in time. The driving protocol is chosen such that the Floquet Hamiltonian corresponds to a localized phase in the high-frequency limit and a delocalized phase in the low-frequency limit. By numerically calculating the inverse participation ratio and the fractal dimension $D_q$, we identify a clear delocalization-localization transition of the Floquet eigenstates at a critical frequency $\omega_{c}\approx0.318\pi$. This transition aligns with the real-to-complex spectrum transition of the Floquet Hamiltonian. For the driven frequency $\omega>\omega_{\mathrm{c}}$, the system resides in a localized phase, and we observe the emergence of CAT states—linear superposition of localized single particle states—in the Floquet spectrum. These states exhibit weight distributions concentrated around a few nearby sites of the chain, forming two peaks of unequal weight due to the non-reciprocal effect, distinguishing them from the Hermitic case. In contrast, for $\omega<\omega_{\mathrm{c}}$, we identify the presence of a mobility edge over a range of driving frequencies, separating localized states (above the edge) from multifractal and extended states (below the edge). Notably, multifractal states are observed in the Floquet eigenspectrum across a broad frequency range. Importantly, we highlight that the non-driven, non-reciprocal AA model does not support multifractal states nor a mobility edge in its spectrum. Thus, our findings reveal unique dynamical signatures that do not exist in the non-driven non-Hermitian scenario, providing a fresh perspective on the localization properties of periodically driven systems. Finally, we provide a possible circuit experiment scheme for the periodically driven non-reciprocal AA model. In the following work, we will extend our research to clean systems, such as Stark models, to explore the influence of periodic driving on their localization properties.

Keywords:

Authors and contacts

WANG Yujia¹,
XU Zhihao^1,2

1.
State Key Laboratory of Quantum Optics Technologies and Devices, Institute of Theoretical Physics, Shanxi University, Taiyuan 030006, China
2.
Collaborative Innovation Center of Extreme Optics, Shanxi University, Taiyuan 030006, China

Corresponding author: XU Zhihao, xuzhihao@sxu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 12375016), the Fundamental Research Program of Shanxi Province, China (Grant No. 20210302123442), the Open Research Fund of Beijing National Laboratory for Condensed Matter Physics, China (Grant No. 2023BNLCMPKF001), and the Fund for Shanxi “1331 Project” Key Subjects, China.

FullText HTML

References

[1]	Anderson P W 1958 Phys. Rev. 109 1492 Google Scholar
[2]	Tang L Z, Zhang G Q, Zhang L F, Zhang D W 2021 Phys. Rev. A 103 033325 Google Scholar
[3]	Schulz M, Hooley C A, Moessner R, Pollmann F 2019 Phys. Rev. Lett. 122 040606 Google Scholar
[4]	Lin X S, Chen X M, Guo G C, Gong M 2023 Phys. Rev. B 108 174206 Google Scholar
[5]	Dai Q, Lu Z P, Xu Z H 2023 Phys. Rev. B 108 144207 Google Scholar
[6]	Liu H, Lu Z P, Xia X, Xu Z H 2024 New J. Phys. 26 093007 Google Scholar
[7]	Li H, Dong Z L, Longhi S, Liang Q, Xie D Z, Yan B 2022 Phys. Rev. Lett. 129 220403 Google Scholar
[8]	Ganeshan S, Pixley J H, Das Sarma S 2015 Phys. Rev. Lett. 114 146601 Google Scholar
[9]	Aditya S, Sengupta K, Sen D 2023 Phys. Rev. B 107 035402 Google Scholar
[10]	Qi R, Cao J P, Jiang X P 2023 Phys. Rev. B 107 224201 Google Scholar
[11]	Zuo Z W, Kang D W 2022 Phys. Rev. A 106 013305 Google Scholar
[12]	Xu Z H, Xia X, Chen S 2021 Phys. Rev. B 104 224204 Google Scholar
[13]	Wang Y C, Xia X, Zhang L, Yao H P, Chen S, You J G, Zhou Q, Liu X J 2020 Phys. Rev. Lett. 125 196604 Google Scholar
[14]	Tang Q Y, He Y 2024 Phys. Rev. B 109 224204 Google Scholar
[15]	Zhou L W 2021 Phys. Rev. Res. 3 033184 Google Scholar
[16]	Liu J H, Xu Z H 2023 Phys. Rev. B 108 184205 Google Scholar
[17]	Lin Q, Li T Y, Xiao L, Wang K K, Yi W, Xue P 2022 Nat. Commun. 13 3229 Google Scholar
[18]	Hatano N, Nelson D R 1996 Phys. Rev. Lett. 77 570 Google Scholar
[19]	Hatano N, Nelson D R 1997 Phys. Rev. B 56 8651 Google Scholar
[20]	Zhou L W, Wang Q H, Wang H L, Gong J B 2018 Phys. Rev. A 98 022129 Google Scholar
[21]	Zhou L W, Gong J B 2018 Phys. Rev. A 97 063603 Google Scholar
[22]	Tiwari V, Bhakuni D S, Sharma A 2024 Phys. Rev. B 109 L161104 Google Scholar
[23]	Ji C R, Zhou S D, Xie A, Jiang Z Y, Sheng X H, Ding L, Ke Y G, Wang H Q, Zhuang S L 2023 Phys. Rev. B 108 054310 Google Scholar
[24]	Mukherjee B, Sen A, Sen D, Sengupta K 2016 Phys. Rev. B 94 155122 Google Scholar
[25]	Mukherjee B, Mohan P, Sen D, Sengupta K 2018 Phys. Rev. B 97 205415 Google Scholar
[26]	Mukherjee B 2018 Phys. Rev. B 98 235112 Google Scholar
[27]	Yang K, Zhou L W, Ma W C, Kong X, Wang P F, Qin X, Rong X, Wang Y, Shi F Z 2019 Phys. Rev. B 100 085308 Google Scholar
[28]	Zhou L W, Du Q Q 2021 New J. Phys. 23 063041 Google Scholar
[29]	Zhou L W 2019 Phys. Rev. B 100 184314 Google Scholar
[30]	Else D V, Bauer B, Nayak C 2016 Phys. Rev. Lett. 117 090402 Google Scholar
[31]	Mukherjee B, Nandy S, Sen A, Sen D, Sengupta K 2020 Phys. Rev. B 101 245107 Google Scholar
[32]	Mukherjee B, Sen A, Sen D, Sengupta K 2020 Phys. Rev. B 102 014301 Google Scholar
[33]	Liu H, Xiong T S, Zhang W, An J H 2019 Phys. Rev. A 100 023622 Google Scholar
[34]	Wu H, An J H 2020 Phys. Rev. B 102 041119 Google Scholar
[35]	Wu H, An J H 2022 Phys. Rev. B 105 L121113 Google Scholar
[36]	Bai S Y, An J H 2020 Phys. Rev. A 102 060201 Google Scholar
[37]	Sarkar M, Ghosh R, Sen A, Sengupta K 2021 Phys. Rev. B 103 184309 Google Scholar
[38]	Sarkar M, Ghosh R, Sen A, Sengupta K 2022 Phys. Rev. B 105 024301 Google Scholar
[39]	Tong Q J, An J H, Gong J B, Luo H G, Oh C H 2013 Phys. Rev. B 87 201109 Google Scholar
[40]	Roy S, Mishra T, Tanatar B, Basu S 2021 Phys. Rev. Lett. 126 106803 Google Scholar
[41]	Ahmed A, Ramachandran A, Khaymovich I M, Sharma A 2022 Phys. Rev. B 106 205119 Google Scholar
[42]	Roy S, Khaymovich I M, Das A, Moessner R 2018 Sci. Post. Phys. 4 025 Google Scholar
[43]	成恩宏, 郎利君 2022 71 160301 Google Scholar Cheng E H, Lang L J 2022 Acta Phys. Sin. 71 160301 Google Scholar

Cited By

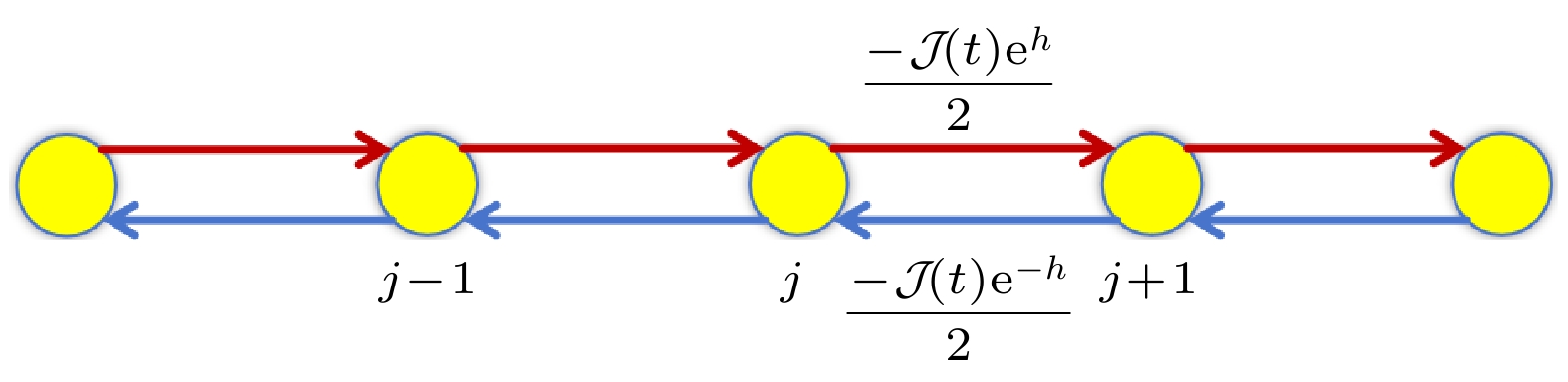
图 1 一维非互易模型示意图. 红线和蓝线代表不同跃迁强度, 其中$ \mathcal{J}(t) $如(1)式所示, $ h $ 代表非互易强度, $ j $代表格点

Figure 1. Schematic diagram of the one-dimensional non-reciprocal model. The red and blue lines represent different hopping amplitudes, $ h $ is non-reciprocal amplitudes and $ j $ is the site index.

DownLoad: Full-Size Img PowerPoint

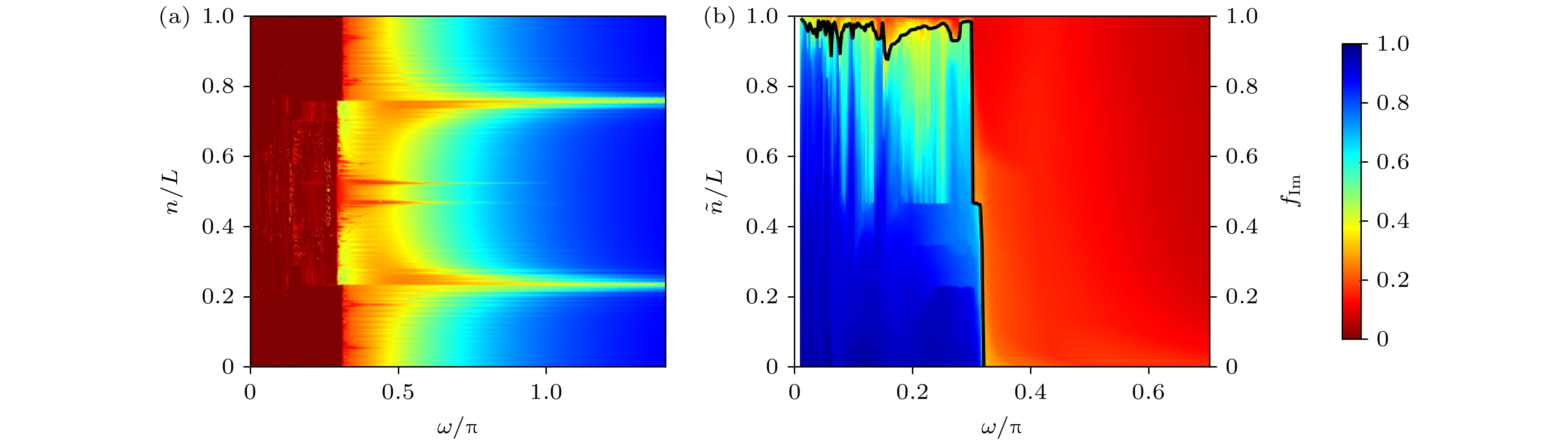
图 2 (a)逆参与率$ I^{(2)}_{n} $随着能级指标$ n/L $和频率$ \omega $的变化情况(这里, 能量实部升序排列). (b)分形维度$ D_{2} $随着能级指标$ \tilde{n}/L $和频率$ \omega $的变化情况, 以及复能量占比$ f_{\mathrm{Im}} $(黑色实线)随频率$ \omega $的变化图, 其中能级指标$ \tilde{n} $以逆参与率值的大小升序排列. 这里$ L=2048 $

Figure 2. (a) Plot of $ I^{(2)}_{n} $ as a function of the normalized eigenfunction index $ n/L $ and $ \omega $. Here, the real part of the eigenvalues is ordered in ascending order. (b) Plot of the fractal dimension $ D_{2} $ as a function of $ \tilde{n}/L $ and $ \omega $, and $ f_{\mathrm{Im}} $ (solid black line) as a function of $ \omega $, where the energies sort in increasing order of the inverse participation ratio. Here, $ L=2048 $.

DownLoad: Full-Size Img PowerPoint

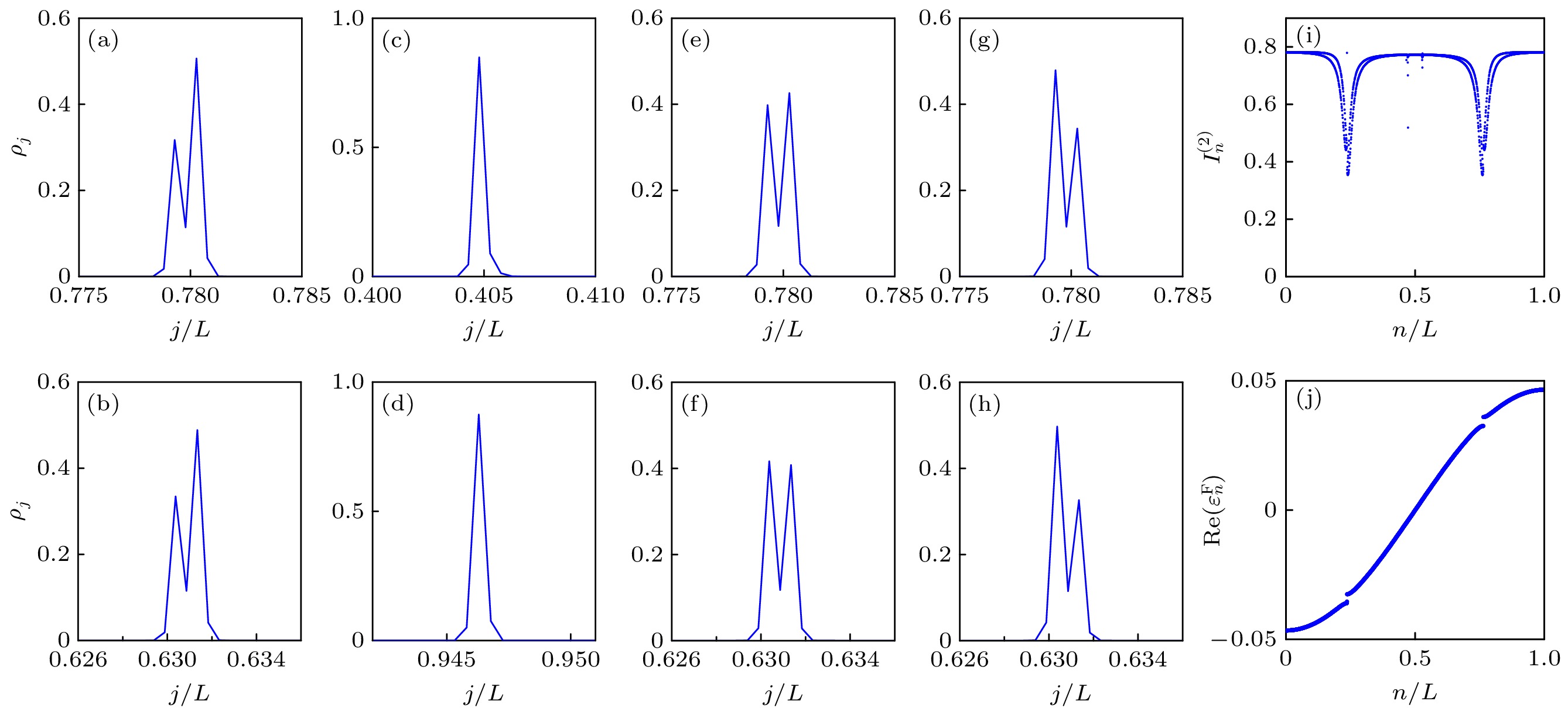
图 3 (a), (e)和(g) [(b), (f)和(h)]分别为$ h=0.1 $, 0和$ -0.1 $时, 位于能级$ n/L\approx0.24 $ ($ n/L\approx0.76 $)处的密度分布$ \rho_j $; (c)和(d)分别为$ h=0.1 $时, 能级$ n/L\approx1/3 $和$ 1/2 $处的密度分布$ \rho_j $; (i) $ I_n^{(2)} $随着能级$ n/L $变化的情况; (j) 能量实部$ \mathrm{Re}(\varepsilon_n^{{\mathrm{F}}}) $随着能级指标$ n/L $的分布情况. 这里选取 $ L=2048 $和$ \omega=\pi $, 并且能级指标按照能量实部升序排列

Figure 3. (a), (e), and (g) [(b), (f), and (h)] Plot of the density distributions $ \rho_j $ at $ n/L\approx0.24 $ ($ n/L\approx0.76 $) with $ h=0.1 $, 0, and $ -0.1 $, respectively; (c) and (d) plot of $ \rho_j $ with $ h=0.1 $ at $ n/L\approx1/3 $ and $ 1/2 $, respectively; (i) $ I_n^{(2)} $ as a function of $ n/L $; (j) plot of $ \mathrm{Re}(\varepsilon_n^{{\mathrm{F}}}) $ as a function of $ n/L $. Here, $ L=2048 $, $ \omega=\pi $, and the real part of eigenvalues is ordered in ascending order.

DownLoad: Full-Size Img PowerPoint

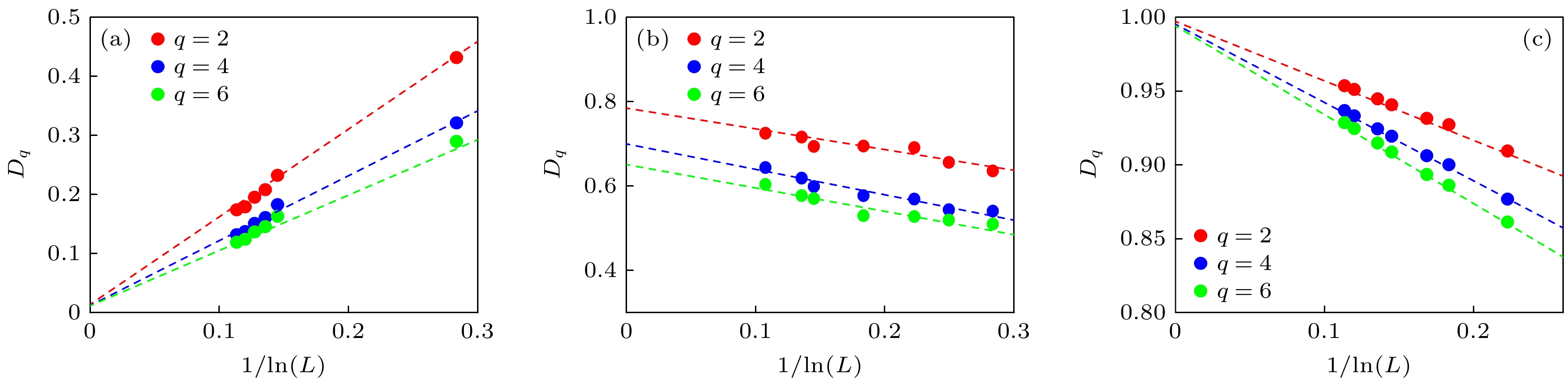
图 4 (a)—(c)分别展示了在频率$ \omega=0.235\pi $时, 能级$ \tilde{n}/L\approx 0.995 $, $ 0.7 $和$ 0.2 $处对应于不同$ q $的分形维度$ D_q $随着尺寸$ 1/\ln{(L)} $的变化情况. 这里能级按照逆参与率值的升序排列

Figure 4. (a)–(c) Plot of the fractal dimensions $ D_q $ as a function of $ 1/\ln{(L)} $ with different $ q $ and $ \omega=0.235\pi $ at $ \tilde{n}/L\approx 0.995 $, $ 0.7 $, and $ 0.2 $, respectively. Here, the energies sort in increasing order of the inverse participation ratio.

DownLoad: Full-Size Img PowerPoint

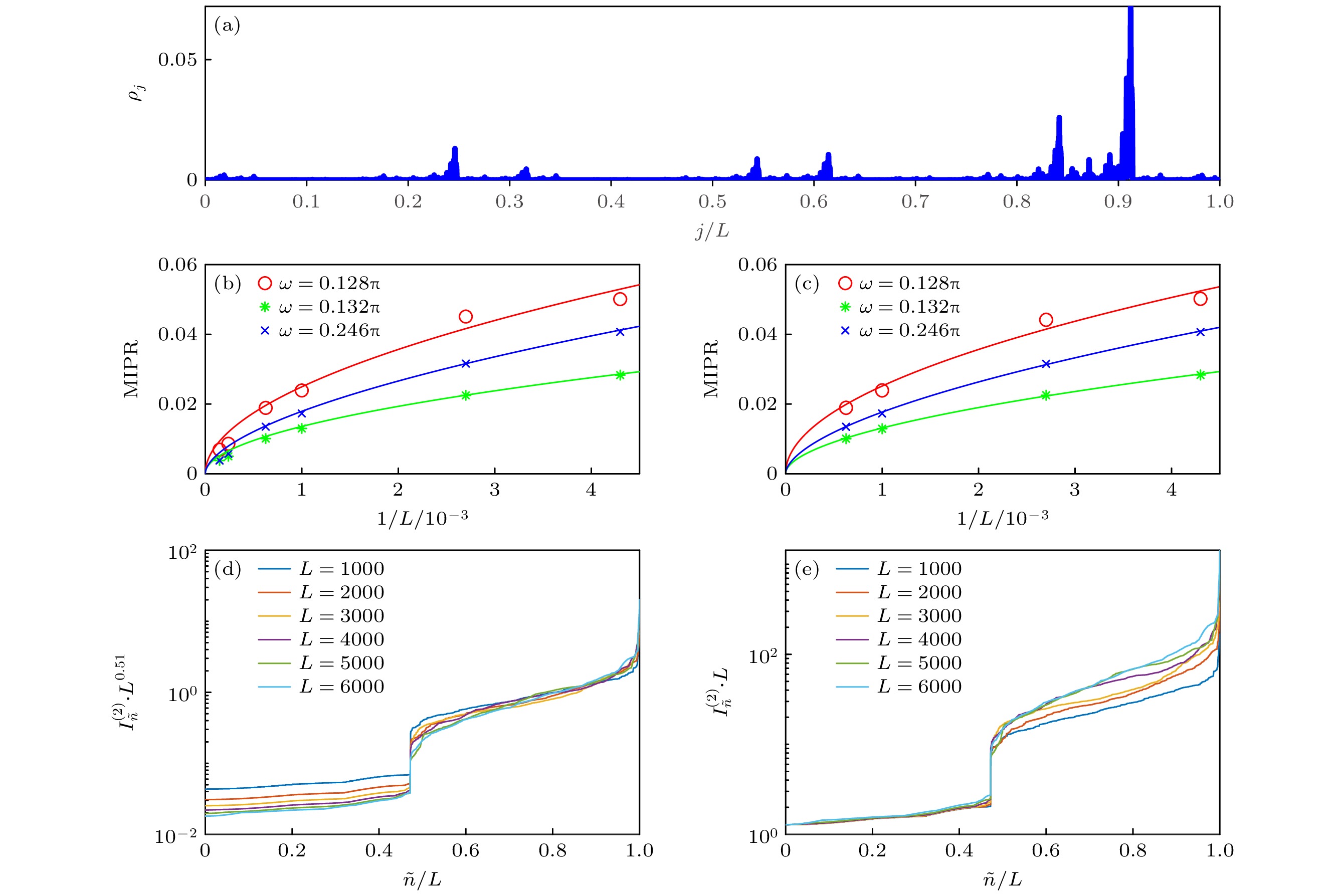
图 5 (a)频率$ \omega=0.132\pi $时, $ \tilde{n}/L=0.75 $处波函数的密度分布$ \rho_j $; (b)不同频率处, MIPR的标度分析; (c)图(b)频率下, 在参数$ h=0.1 $和$ \mu=0.05 $附近随机选取20个数进行无序平均后, MIPR的标度分析; (d), (e)频率$ \omega=0.132\pi $时, $ I_{\tilde{n}}^{(2)}\cdot L^{0.51} $与$ I_{\tilde{n}}^{(2)}\cdot L $随不同尺寸的缩放图. 这里能级按照逆参与率值的升序排列

Figure 5. (a) Density distribution $ \rho_j $ with $ \omega=0.132\pi $ at $ \tilde{n}/L=0.75 $; (b) the scaling of the MIPR for different $ \omega $; (c) the scaling of MIPR after averaging random 20 parameters near $ h = 0.1 $ and $ \mu = 0.05 $ with the frequency in panel (b); (d), (e) the scaling of $ I_{\tilde{n}}^{(2)}\cdot L^{0.51} $ and $ I_{\tilde{n}}^{(2)}\cdot L $ as a function of $ L $ with $ \omega=0.132\pi $. Here, the energies sort in increasing order of the inverse participation ratio.

DownLoad: Full-Size Img PowerPoint

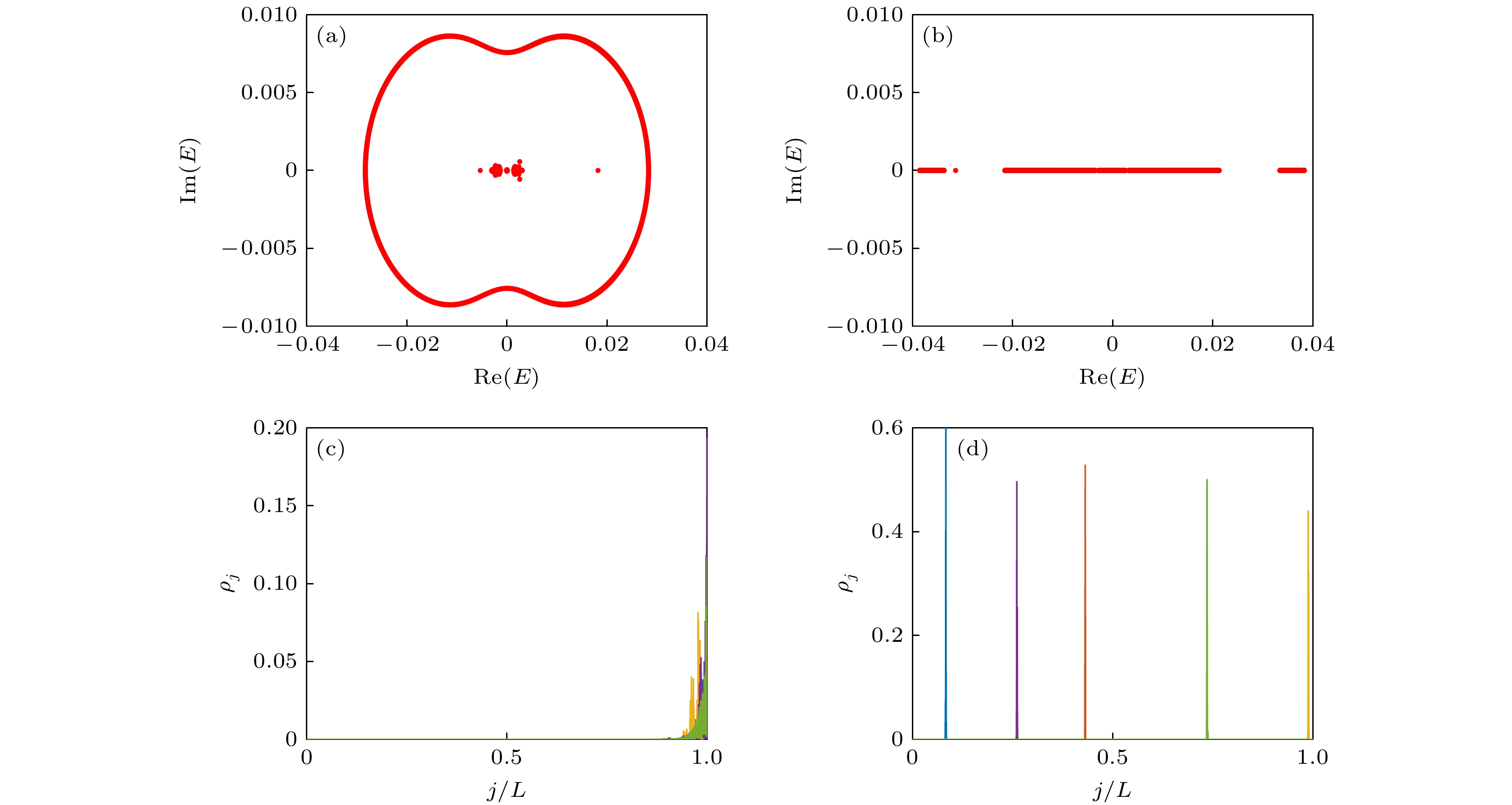
图 6 频率$ \omega=0.132\pi $ (a)和$ \omega=0.5\pi $ (b)时在复空间的能谱图; (c)在开边界条件下, 当频率$ \omega=0.132\pi $时环上随机5个能级所对应的密度分布$ \rho_j $; (d)在开边界条件下, 当频率$ \omega=0.5\pi $时随机5个能级所对应的密度分布$ \rho_j $, 这里$ L=2048 $

Figure 6. Energy spectrum with $ \omega=0.132\pi $ (a) and $ \omega=0.5\pi $ (b) in complex space; (c) the density distribution $ \rho_j $ for 5 random energy levels on the ring under open boundary conditions with frequency $ \omega = 0.132\pi $; (d) the density distribution $ \rho_j $ for 5 random energy levels under open boundary conditions with frequency $ \omega = 0.5\pi $. Here, $ L=2048 $.

DownLoad: Full-Size Img PowerPoint

map

[1]	Anderson P W 1958 Phys. Rev. 109 1492 Google Scholar
[2]	Tang L Z, Zhang G Q, Zhang L F, Zhang D W 2021 Phys. Rev. A 103 033325 Google Scholar
[3]	Schulz M, Hooley C A, Moessner R, Pollmann F 2019 Phys. Rev. Lett. 122 040606 Google Scholar
[4]	Lin X S, Chen X M, Guo G C, Gong M 2023 Phys. Rev. B 108 174206 Google Scholar
[5]	Dai Q, Lu Z P, Xu Z H 2023 Phys. Rev. B 108 144207 Google Scholar
[6]	Liu H, Lu Z P, Xia X, Xu Z H 2024 New J. Phys. 26 093007 Google Scholar
[7]	Li H, Dong Z L, Longhi S, Liang Q, Xie D Z, Yan B 2022 Phys. Rev. Lett. 129 220403 Google Scholar
[8]	Ganeshan S, Pixley J H, Das Sarma S 2015 Phys. Rev. Lett. 114 146601 Google Scholar
[9]	Aditya S, Sengupta K, Sen D 2023 Phys. Rev. B 107 035402 Google Scholar
[10]	Qi R, Cao J P, Jiang X P 2023 Phys. Rev. B 107 224201 Google Scholar
[11]	Zuo Z W, Kang D W 2022 Phys. Rev. A 106 013305 Google Scholar
[12]	Xu Z H, Xia X, Chen S 2021 Phys. Rev. B 104 224204 Google Scholar
[13]	Wang Y C, Xia X, Zhang L, Yao H P, Chen S, You J G, Zhou Q, Liu X J 2020 Phys. Rev. Lett. 125 196604 Google Scholar
[14]	Tang Q Y, He Y 2024 Phys. Rev. B 109 224204 Google Scholar
[15]	Zhou L W 2021 Phys. Rev. Res. 3 033184 Google Scholar
[16]	Liu J H, Xu Z H 2023 Phys. Rev. B 108 184205 Google Scholar
[17]	Lin Q, Li T Y, Xiao L, Wang K K, Yi W, Xue P 2022 Nat. Commun. 13 3229 Google Scholar
[18]	Hatano N, Nelson D R 1996 Phys. Rev. Lett. 77 570 Google Scholar
[19]	Hatano N, Nelson D R 1997 Phys. Rev. B 56 8651 Google Scholar
[20]	Zhou L W, Wang Q H, Wang H L, Gong J B 2018 Phys. Rev. A 98 022129 Google Scholar
[21]	Zhou L W, Gong J B 2018 Phys. Rev. A 97 063603 Google Scholar
[22]	Tiwari V, Bhakuni D S, Sharma A 2024 Phys. Rev. B 109 L161104 Google Scholar
[23]	Ji C R, Zhou S D, Xie A, Jiang Z Y, Sheng X H, Ding L, Ke Y G, Wang H Q, Zhuang S L 2023 Phys. Rev. B 108 054310 Google Scholar
[24]	Mukherjee B, Sen A, Sen D, Sengupta K 2016 Phys. Rev. B 94 155122 Google Scholar
[25]	Mukherjee B, Mohan P, Sen D, Sengupta K 2018 Phys. Rev. B 97 205415 Google Scholar
[26]	Mukherjee B 2018 Phys. Rev. B 98 235112 Google Scholar
[27]	Yang K, Zhou L W, Ma W C, Kong X, Wang P F, Qin X, Rong X, Wang Y, Shi F Z 2019 Phys. Rev. B 100 085308 Google Scholar
[28]	Zhou L W, Du Q Q 2021 New J. Phys. 23 063041 Google Scholar
[29]	Zhou L W 2019 Phys. Rev. B 100 184314 Google Scholar
[30]	Else D V, Bauer B, Nayak C 2016 Phys. Rev. Lett. 117 090402 Google Scholar
[31]	Mukherjee B, Nandy S, Sen A, Sen D, Sengupta K 2020 Phys. Rev. B 101 245107 Google Scholar
[32]	Mukherjee B, Sen A, Sen D, Sengupta K 2020 Phys. Rev. B 102 014301 Google Scholar
[33]	Liu H, Xiong T S, Zhang W, An J H 2019 Phys. Rev. A 100 023622 Google Scholar
[34]	Wu H, An J H 2020 Phys. Rev. B 102 041119 Google Scholar
[35]	Wu H, An J H 2022 Phys. Rev. B 105 L121113 Google Scholar
[36]	Bai S Y, An J H 2020 Phys. Rev. A 102 060201 Google Scholar
[37]	Sarkar M, Ghosh R, Sen A, Sengupta K 2021 Phys. Rev. B 103 184309 Google Scholar
[38]	Sarkar M, Ghosh R, Sen A, Sengupta K 2022 Phys. Rev. B 105 024301 Google Scholar
[39]	Tong Q J, An J H, Gong J B, Luo H G, Oh C H 2013 Phys. Rev. B 87 201109 Google Scholar
[40]	Roy S, Mishra T, Tanatar B, Basu S 2021 Phys. Rev. Lett. 126 106803 Google Scholar
[41]	Ahmed A, Ramachandran A, Khaymovich I M, Sharma A 2022 Phys. Rev. B 106 205119 Google Scholar
[42]	Roy S, Khaymovich I M, Das A, Moessner R 2018 Sci. Post. Phys. 4 025 Google Scholar
[43]	成恩宏, 郎利君 2022 71 160301 Google Scholar Cheng E H, Lang L J 2022 Acta Phys. Sin. 71 160301 Google Scholar

[1]	HAO Jiaxin, XU Zhihao. Localization transition in a two-particle system with complex interaction modulation. Acta Physica Sinica, 2025, 74(6): 067201. doi: 10.7498/aps.74.20241691
[2]	Feng Xi-Xi, Chen Wen, Gao Xian-Long. Application of metric space method in quantum information in quasi-periodic systems. Acta Physica Sinica, 2024, 73(4): 040501. doi: 10.7498/aps.73.20231605
[3]	Liu Hui, Lu Zhan-Peng, Xu Zhi-Hao. Delocalization-localization transitions in 1D non-Hermitian cross-stitch lattices. Acta Physica Sinica, 2024, 73(13): 137201. doi: 10.7498/aps.73.20240510
[4]	Lu Zhan-Peng, Xu Zhi-Hao. Reentrant localization phenomenon in one-dimensional cross-stitch lattice with flat band. Acta Physica Sinica, 2024, 73(3): 037202. doi: 10.7498/aps.73.20231393
[5]	Ren Guo-Liang, Shen Kai-Bo, Liu Yong-Jia, Liu Ying-Guang. Thermal conduction mechanism of graphene-like carbon nitride structure (C₃N). Acta Physica Sinica, 2023, 72(1): 013102. doi: 10.7498/aps.72.20221441
[6]	Wu Jin, Lu Zhan-Peng, Xu Zhi-Hao, Guo Li-Ping. Mobility edges and reentrant localization induced by superradiance. Acta Physica Sinica, 2022, 71(11): 113702. doi: 10.7498/aps.71.20212246
[7]	Xu Zhi-Hao, Huangfu Hong-Li, Zhang Yun-Bo. Mobility edges of bosonic pairs in one-dimensional quasi-periodical lattices. Acta Physica Sinica, 2019, 68(8): 087201. doi: 10.7498/aps.68.20182218
[8]	Wang Zi, Zhang Dan-Mei, Ren Jie. Topological and non-reciprocal phenomena in elastic waves and heat transport of phononic systems. Acta Physica Sinica, 2019, 68(22): 220302. doi: 10.7498/aps.68.20191463
[9]	Liu Tong, Gao Xian-Long. Identifying the mobility edges in a one-dimensional incommensurate model with p-wave superfluid. Acta Physica Sinica, 2016, 65(11): 117101. doi: 10.7498/aps.65.117101
[10]	Ding Rui, Jin Ya-Qiu, Ogura Hisanao. Stochastic functional analysis of propagation and localization of cylindrical wave in a two-dimensional random medium. Acta Physica Sinica, 2010, 59(6): 3674-3685. doi: 10.7498/aps.59.3674
[11]	Cao Yong-Jun, Yang Xu, Jiang Zi-Lei. Transmission property of elastic wave through one-dimensional compound materials. Acta Physica Sinica, 2009, 58(11): 7735-7740. doi: 10.7498/aps.58.7735
[12]	Wang Hui-Qin, Liu Zheng-Dong, Wang Bing. The spatial distribution of optical field in random media with different filling densities of the same material particles. Acta Physica Sinica, 2008, 57(4): 2186-2191. doi: 10.7498/aps.57.2186
[13]	Wang Hui-Qin, Liu Zheng-Dong, Wang Bing. The spatial energy distribution and the spectrum characteristics in a two-dimensional random medium. Acta Physica Sinica, 2008, 57(9): 5550-5557. doi: 10.7498/aps.57.5550
[14]	Cao Yong-Jun, Yang Xu. Transmission properties of the generalized Fibonacci quasi-periodical phononic crystal. Acta Physica Sinica, 2008, 57(6): 3620-3624. doi: 10.7498/aps.57.3620
[15]	Zhou Wen-Zheng, Lin Tie, Shang Li-Yan, Huang Zhi-Ming, Cui Li-Jie, Li Dong-Lin, Gao Hong-Ling, Zeng Yi-Ping, Guo Shao-Ling, Gui Yong-Sheng, Chu Jun-Hao. Weak anti-localization in InAlAs/InGaAs/InAlAs high mobility two-dimensional electron gas systems. Acta Physica Sinica, 2007, 56(7): 4099-4104. doi: 10.7498/aps.56.4099
[16]	Cao Yong-Jun, Dong Chun-Hong, Zhou Pei-Qin. Transmission properties of one-dimensional qusi-periodical phononic crystal. Acta Physica Sinica, 2006, 55(12): 6470-6475. doi: 10.7498/aps.55.6470
[17]	WANG CHUAN-KUI, SUN JIN-ZUO, WANG JI-SUO, WANG WEN-ZHENG. MOBILITY EDGE IN THE AUBRY MODEL OF ONE-DIMENSIONAL INCOMMENSURATE SYSTEMS. Acta Physica Sinica, 1993, 42(1): 95-100. doi: 10.7498/aps.42.95
[18]	PANG GEN-DI, CAI JIAN-HUA. PHONON LOCALIZATION IN INHOMOGENEOUS DISORDERED SYSTEMS. Acta Physica Sinica, 1988, 37(4): 688-690. doi: 10.7498/aps.37.688
[19]	LIU YOU-YAN, ZHOU YI-CHANG. ON THE MOBILITY EDGES IN A ONE-DIMENSIONAL INCOMMENSURATE SYSTEMS. Acta Physica Sinica, 1988, 37(11): 1807-1813. doi: 10.7498/aps.37.1807
[20]	XIONG SHI-JIE, CAI JIAN-HUA. SCALING THEORY OF ANDERSON LOCALIZATION IN DISORDERED SYSTEMS WITH SPACE MODULATIONS A REAL SPACE RENORMALIZATION GROUP APPROACH. Acta Physica Sinica, 1985, 34(12): 1530-1538. doi: 10.7498/aps.34.1530

Catalog

Vol. 74, Issue 9 - 2025-05-05

Metrics

Abstract views: 479
PDF Downloads: 33
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板