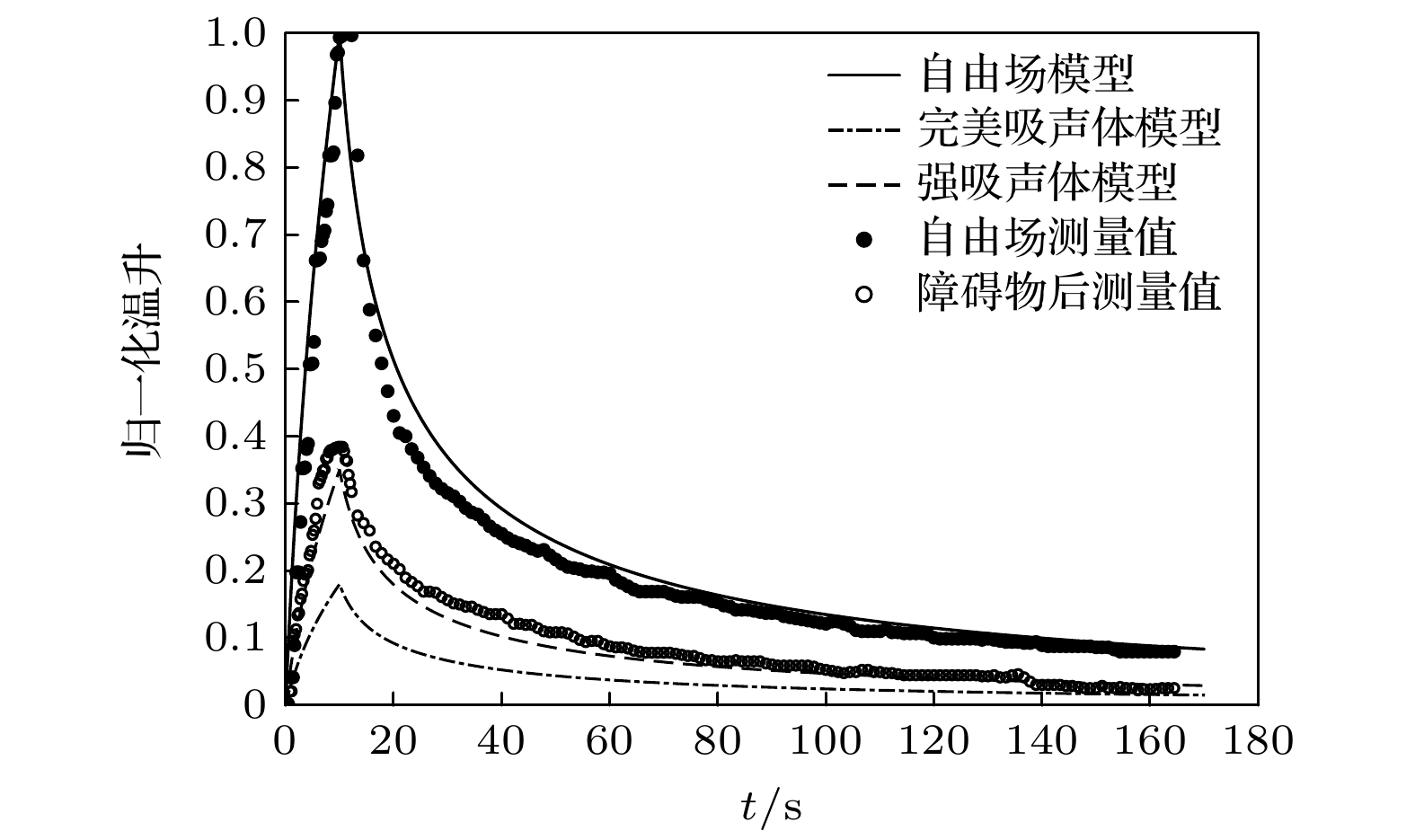
-
During the treatment of subcostal lesions with high intensity focused ultrasound (HIFU), the obstruction by the ribs significantly affects the therapeutic effect, which can be assessed through numerical calculations. In existing studies, ribs are typically regarded as perfect acoustic absorbers, and even though this assumption could reveal the influence of the ribs on the acoustic field to some extent, it may still underestimate the energy behind the rib cage. In order to address the shortcomings of current work, an innovative numerical calculation method that avoids treating ribs as perfect sound absorbers is proposed in this work. Subsequently, experiments are conducted using ABS plastic rib cage mimic to compare the effectiveness between the two methods, demonstrating that the method proposed in this paper, which avoids the assumption of considering ribs as perfect acoustic absorbers, could better reveal the influence caused by ribs, and further studies are carried out on the influence of ribs in a multi-layered medium model. In response to the numerical oscillation issues encountered in existing work when dealing with media with high acoustic attenuation coefficients, the operator splitting method to enhance the stability of numerical calculations is adopted in this work. Furthermore, to tackle the challenges posed by asymmetric acoustic fields in numerical computations, in this paper matrix vectorization technique is introduced and stable solutions for the acoustic field under the backward implicit difference scheme are obtained. Additionally, when considering nonlinear effects, an asymptotic maximum number of harmonics is employed to reduce the computational load. These improvements in both the numerical calculation model and the corresponding algorithm not only enhance the precision of numerical computations, but also reveal the underestimation of energy behind the ribs due to the assumption of perfect acoustic absorbers, which is significant for optimizing HIFU treatment strategies.
-
Keywords:
- high intensity focused ultrasound /
- operator separation method /
- asymmetry /
- matrix vectorization
[1] Guang Z L P, Kristensen G, Røder A, Brasso K 2024 Clin. Genitourin. Cancer 22 102101
Google Scholar
[2] Schaudinn A, Michaelis J, Franz T, et al. 2021 Eur. J. Radiol. 144 109957
Google Scholar
[3] 蔡忠林, 刘强照, 王朝阳, 李慧, 周逢海 2017 现代肿瘤医学 25 2011
Google Scholar
Cai Z L, Liu Q Z, Wang C Y, Li H, Zhou F H 2017 Mod. Oncol. 25 2011
Google Scholar
[4] Zhang P, Xie L, Chen J, Zhan P, Xing H R, Yuan Y 2024 Ultrasound Med. Biol. 50 1381
Google Scholar
[5] Fan H J, Cun J P, Zhao W, Huang J Q, Yi G F, Yao R H, Gao B L, Li X H 2018 Int. J. Hyperthermia 35 534
Google Scholar
[6] 姚一静, 姜立新 2021 声学技术 40 376
Google Scholar
Yao Y J, Jiang L X 2021 Tech. Acoust. 40 376
Google Scholar
[7] Imankulov S B, Fedotovskikh G V, Shaimardanova G M, Yerlan M, Zhampeisov N K 2015 Ultrason. Sonochem. 27 712
Google Scholar
[8] Dupré A, Melodelima D, Cilleros C, De Crignis L, Peyrat P, Vincenot J, Rivoire M 2023 IRBM 44 100738
Google Scholar
[9] Li J L, Liu X Z, Zhang D, Gong X F 2007 Ultrasound Med. Biol. 33 1413
Google Scholar
[10] Yuldashev P V, Shmeleva S M, Ilyin S A, Sapozhnikov O A, Gavrilov L R, Khokhlova V A 2013 Phys. Med. Biol. 58 2537
Google Scholar
[11] Lin J X, Liu X Z, Gong X F, Ping Z H, Wu J R 2013 J. Acoust. Soc. Am. 134 1702
Google Scholar
[12] Kamakura T, Ishiwata T, Matsuda K 2000 J. Acoust. Soc. Am. 107 3035
Google Scholar
[13] Wang X, Lin J, Liu X J, Liu J Z, Gong X F 2016 Chin. Phys. B 25 044301
Google Scholar
[14] Zhao Y S, Gan Y, Long Y P, Sun F J, Fan X H 2024 Appl. Acoust. 216 109740
Google Scholar
[15] de Greef M, Schubert G, Wijlemans J W, Koskela J, Bartels L W, Moonen C T W, Ries M 2015 Med. Phys. 42 4685
Google Scholar
[16] Liu H L, Chang H, Chen W S, Shih T C, Hsiao J K, Lin W L 2007 Med. Phys. 34 3436
Google Scholar
[17] Liu H L, Hsu C L, Huang S M, Hsi Y W 2010 Med. Phys. 37 848
Google Scholar
[18] Sapareto S A, Dewey W C 1984 Int. J. Radiat. Oncol. Biol. Phys. 10 787
Google Scholar
[19] Cao R, Huang Z, Nabi G, Melzer A 2020 J. Ultrasound Med. 39 883
Google Scholar
[20] Khokhlova V A, Souchon R, Tavakkoli J, Sapozhnikov O A, Cathignol D 2001 J. Acoust. Soc. Am. 110 95
Google Scholar
[21] Fan T B, Liu Z B, Zhang Z, Zhang D, Gong X F 2009 Chin. Phys. Lett. 26 084302
Google Scholar
[22] Wear K A 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 67 454
Google Scholar
[23] 钱盛友, 王鸿樟 2001 50 501
Google Scholar
Qian S Y, Wang H Z 2001 Acta Phys. Sin. 50 501
Google Scholar
[24] Khokhlova V, Shmeleva S, Gavrilov L 2010 Acoust. Phys. 56 665
Google Scholar
-
图 1 椭球系示意图
Figure 1. Illustration of the oblate spheroidal coordinate system.
图 2 球面波区域与平面波区域划分示意图
Figure 2. Illustration of the division between spherical wave region and plane wave region.
图 3 生物组织模型示意图 (a) 三维视图; (b) $ xz $截面图
Figure 3. Schematic diagram of the biological tissue model: (a) 3D-view; (b) $ xz $-section.
图 4 实验环境示意图 (a) 声场扫描系统; (b) ABS塑料仿肋模型
Figure 4. Schematic diagram of the experimental environment: (a) Ultrasonic scanning system; (b) rib mimic made of ABS plastic.
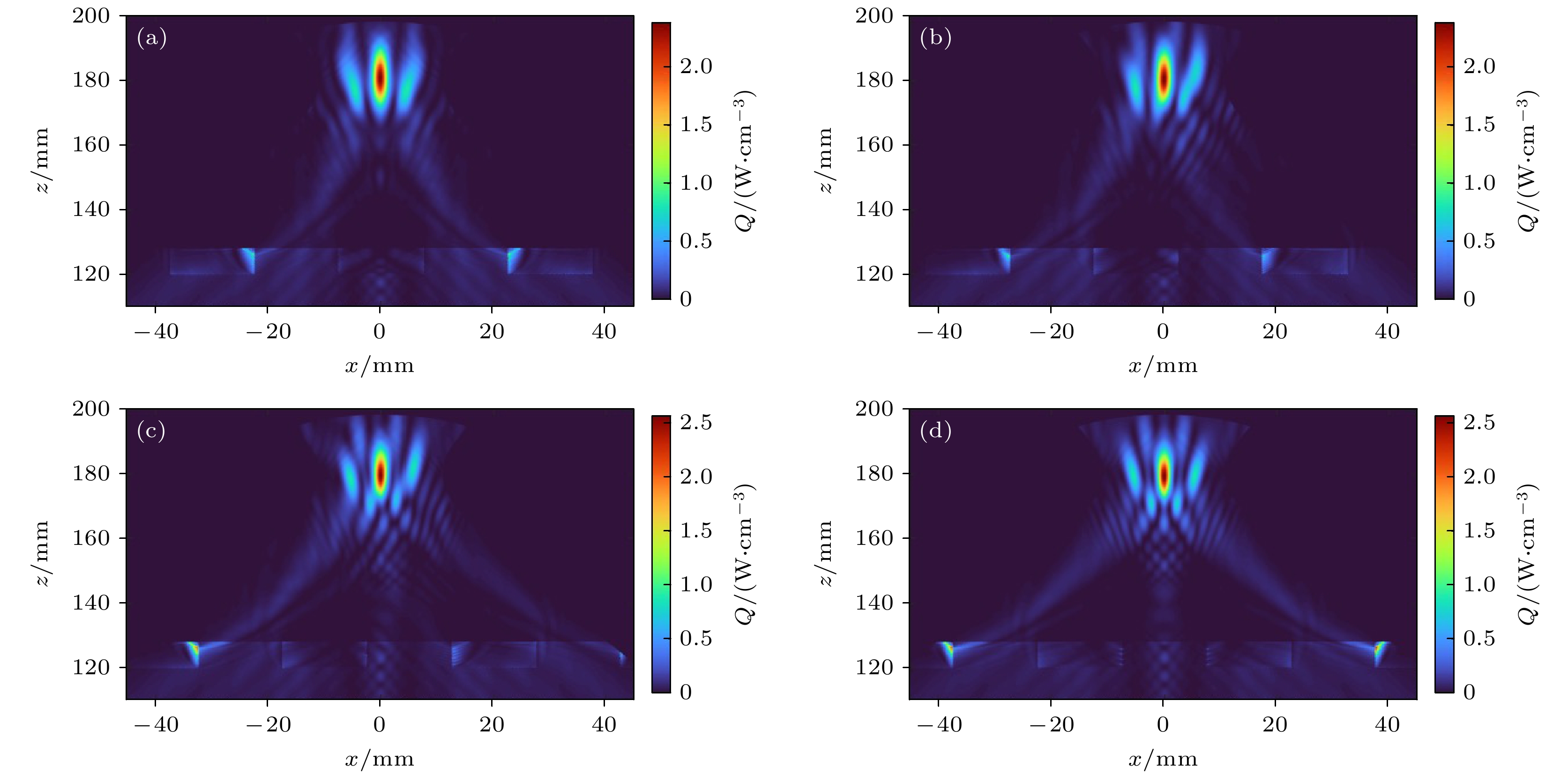
图 5 焦平面内声压峰峰值分布图 (a) $H\mathrm{_{FS}}= 64\; {\mathrm{mm}} $; (b) $H\mathrm{_{FS}}= 74\; {\mathrm{mm}} $; (c) $H\mathrm{_{FS}}= 84\; {\mathrm{mm}} $; (d) $H\mathrm{_{FS}}= 94\; {\mathrm{mm}} $
Figure 5. Distribution of peak-to-peak pressure in the focal plane: (a) $H\mathrm{_{FS}}= 64\; {\mathrm{mm}} $; (b) $H\mathrm{_{FS}}= 74\; {\mathrm{mm}} $; (c) $H\mathrm{_{FS}}= 84\; {\mathrm{mm}} $; (d) $H\mathrm{_{FS}}= 94\; {\mathrm{mm}} $
图 6 $ H\mathrm{_{FS} = 70\; mm} $时基波振幅沿z轴的分布
Figure 6. Distribution of fundamental amplitude with $ H\mathrm{_{FS} = 70\; mm} $ along the z-axis.
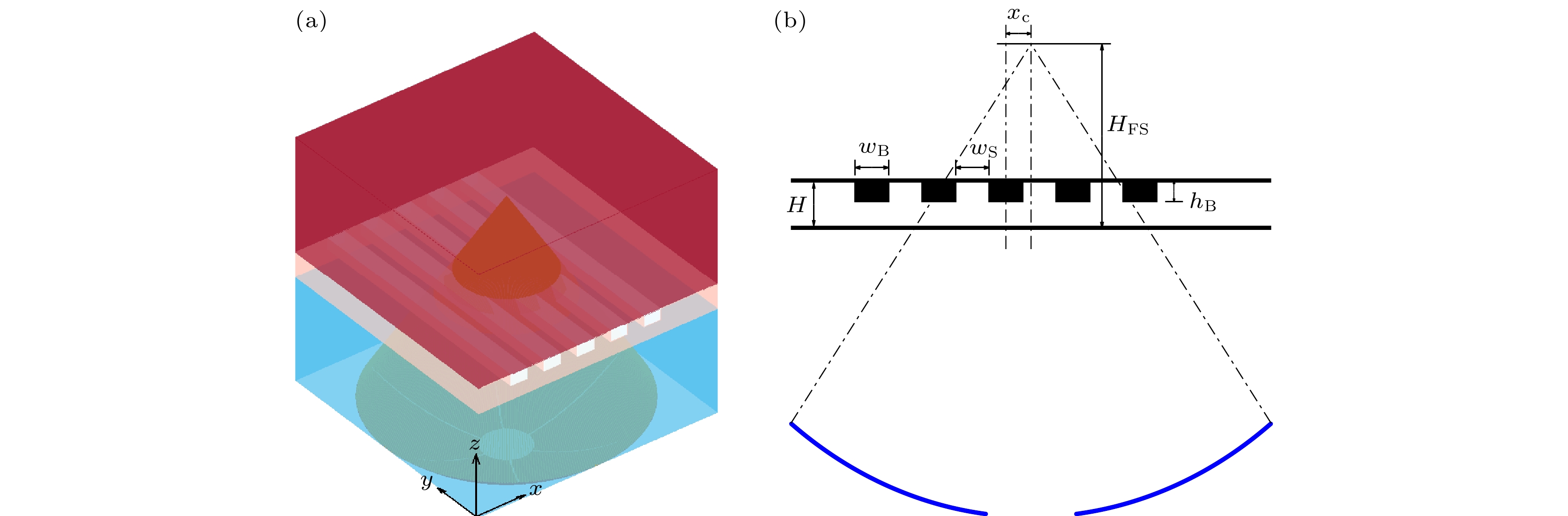
图 7 $ H\mathrm{_{FS} = 70 \;mm} $时热沉积速率在截面$ \varphi = 0 $内的分布 (a) $ x\mathrm{_c = 0\; mm} $; (b) $ x\mathrm{_c = 5 \;mm} $; (c) $ x\mathrm{_c = 10\; mm} $; (d) $ x\mathrm{_c = 15 \;mm} $
Figure 7. Distribution of heat deposition rate in the $ \varphi = 0 $ plane with $ H\mathrm{_{FS} = 70 \;mm} $: (a) $ x\mathrm{_c = 0 \;mm} $; (b) $ x\mathrm{_c = 5 \;mm} $; (c) $ x\mathrm{_c = 10\; mm} $; (d) $ x\mathrm{_c = 15\; mm} $.
图 8 $ H\mathrm{_{FS}} $对最大热沉积速率的影响 (a) 完美吸声体模型; (b) 强吸声体模型
Figure 8. Maximum heat deposition rate corresponding to different $ H\mathrm{_{FS}} $: (a) Perfect acoustic absorber model; (b) strong acoustic absorber model.
图 9 测温组织模型 (a) 定制容器; (b) 模型实物
Figure 9. Temperature measurement tissue model: (a) Customized container; (b) actual model.
图 10 焦域的归一化温升随时间的变化情况
Figure 10. Normalized temperature variation in the focal region over time.
表 1 数值计算中使用的介质声参数
Table 1. Acoustic parameters of the medium used in numerical computation.
$ \rho/\left(\mathrm{kg \cdot m^{-3}} \right) $ $ c/\left(\mathrm{m\cdot s^{-1}} \right) $ $ \alpha/\left(\mathrm{Np\cdot {MHz}^{-\mu} \cdot m^{-1}} \right) $ μ β 水 1000 1500 0.025 2 3.5 脂肪 910 1430 9 1.15 10.5 肋骨 1450 2350 90 1 0 肝脏 1050 1596 4.5 1.13 6 
DownLoad: CSV
表 2 $ H \mathrm{_{FS} = 70 \;mm} $时z轴上的声场参数
Table 2. Acoustic field’s parameters along the z-axis with $ H\mathrm{_{FS} = 70 \;mm} $.
$ x\mathrm{_c/mm} $ Field Ref 0 5 10 15 $ A\mathrm{_1}/p_0 $ 49.09 27.43 27.75 29.07 29.73 $ A\mathrm{_2}/p_0 $ 26.02 10.21 10.13 10.22 10.26 $ A\mathrm{_3}/p_0 $ 14.96 3.82 3.71 3.59 3.53 $ z\mathrm{_1/mm} $ 179.60 180.99 180.90 179.42 179.37 $ z\mathrm{_2/mm} $ 180.09 180.54 180.36 179.78 179.46 $ z\mathrm{_3/mm} $ 180.32 180.90 180.68 179.96 179.60
DownLoad: CSV
表 3 $ H \mathrm{_{FS} = 70 \;mm} $时平面$ \sigma = 0 $内的声场参数
Table 3. Acoustic field’s parameters in the $ \sigma = 0 $ plane with $ H \mathrm{_{FS} = 70 \;mm} $.
$ x\mathrm{_c/mm} $ Field Ref 0 5 10 15 $ \mathrm{WX_{1, -6\;dB}/mm} $ 3.22 3.39 3.21 2.86 2.71 $ \mathrm{WX_{2, -6\;dB}/mm} $ 1.90 1.91 1.83 1.62 1.53 $ \mathrm{WX_{3, -6\; dB}/mm} $ 1.46 1.40 1.36 1.23 1.18 $ \mathrm{WY_{1, -6\; dB}/mm} $ 3.22 3.12 3.13 3.23 3.27 $ \mathrm{WY_{2, -6\; dB}/mm} $ 1.90 1.80 1.80 1.84 1.86 $ \mathrm{WY_{3, -6\; dB}/mm} $ 1.46 1.39 1.39 1.39 1.39
DownLoad: CSV
-
[1] Guang Z L P, Kristensen G, Røder A, Brasso K 2024 Clin. Genitourin. Cancer 22 102101
Google Scholar
[2] Schaudinn A, Michaelis J, Franz T, et al. 2021 Eur. J. Radiol. 144 109957
Google Scholar
[3] 蔡忠林, 刘强照, 王朝阳, 李慧, 周逢海 2017 现代肿瘤医学 25 2011
Google Scholar
Cai Z L, Liu Q Z, Wang C Y, Li H, Zhou F H 2017 Mod. Oncol. 25 2011
Google Scholar
[4] Zhang P, Xie L, Chen J, Zhan P, Xing H R, Yuan Y 2024 Ultrasound Med. Biol. 50 1381
Google Scholar
[5] Fan H J, Cun J P, Zhao W, Huang J Q, Yi G F, Yao R H, Gao B L, Li X H 2018 Int. J. Hyperthermia 35 534
Google Scholar
[6] 姚一静, 姜立新 2021 声学技术 40 376
Google Scholar
Yao Y J, Jiang L X 2021 Tech. Acoust. 40 376
Google Scholar
[7] Imankulov S B, Fedotovskikh G V, Shaimardanova G M, Yerlan M, Zhampeisov N K 2015 Ultrason. Sonochem. 27 712
Google Scholar
[8] Dupré A, Melodelima D, Cilleros C, De Crignis L, Peyrat P, Vincenot J, Rivoire M 2023 IRBM 44 100738
Google Scholar
[9] Li J L, Liu X Z, Zhang D, Gong X F 2007 Ultrasound Med. Biol. 33 1413
Google Scholar
[10] Yuldashev P V, Shmeleva S M, Ilyin S A, Sapozhnikov O A, Gavrilov L R, Khokhlova V A 2013 Phys. Med. Biol. 58 2537
Google Scholar
[11] Lin J X, Liu X Z, Gong X F, Ping Z H, Wu J R 2013 J. Acoust. Soc. Am. 134 1702
Google Scholar
[12] Kamakura T, Ishiwata T, Matsuda K 2000 J. Acoust. Soc. Am. 107 3035
Google Scholar
[13] Wang X, Lin J, Liu X J, Liu J Z, Gong X F 2016 Chin. Phys. B 25 044301
Google Scholar
[14] Zhao Y S, Gan Y, Long Y P, Sun F J, Fan X H 2024 Appl. Acoust. 216 109740
Google Scholar
[15] de Greef M, Schubert G, Wijlemans J W, Koskela J, Bartels L W, Moonen C T W, Ries M 2015 Med. Phys. 42 4685
Google Scholar
[16] Liu H L, Chang H, Chen W S, Shih T C, Hsiao J K, Lin W L 2007 Med. Phys. 34 3436
Google Scholar
[17] Liu H L, Hsu C L, Huang S M, Hsi Y W 2010 Med. Phys. 37 848
Google Scholar
[18] Sapareto S A, Dewey W C 1984 Int. J. Radiat. Oncol. Biol. Phys. 10 787
Google Scholar
[19] Cao R, Huang Z, Nabi G, Melzer A 2020 J. Ultrasound Med. 39 883
Google Scholar
[20] Khokhlova V A, Souchon R, Tavakkoli J, Sapozhnikov O A, Cathignol D 2001 J. Acoust. Soc. Am. 110 95
Google Scholar
[21] Fan T B, Liu Z B, Zhang Z, Zhang D, Gong X F 2009 Chin. Phys. Lett. 26 084302
Google Scholar
[22] Wear K A 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 67 454
Google Scholar
[23] 钱盛友, 王鸿樟 2001 50 501
Google Scholar
Qian S Y, Wang H Z 2001 Acta Phys. Sin. 50 501
Google Scholar
[24] Khokhlova V, Shmeleva S, Gavrilov L 2010 Acoust. Phys. 56 665
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 511
- PDF Downloads: 3
- Cited By: 0
