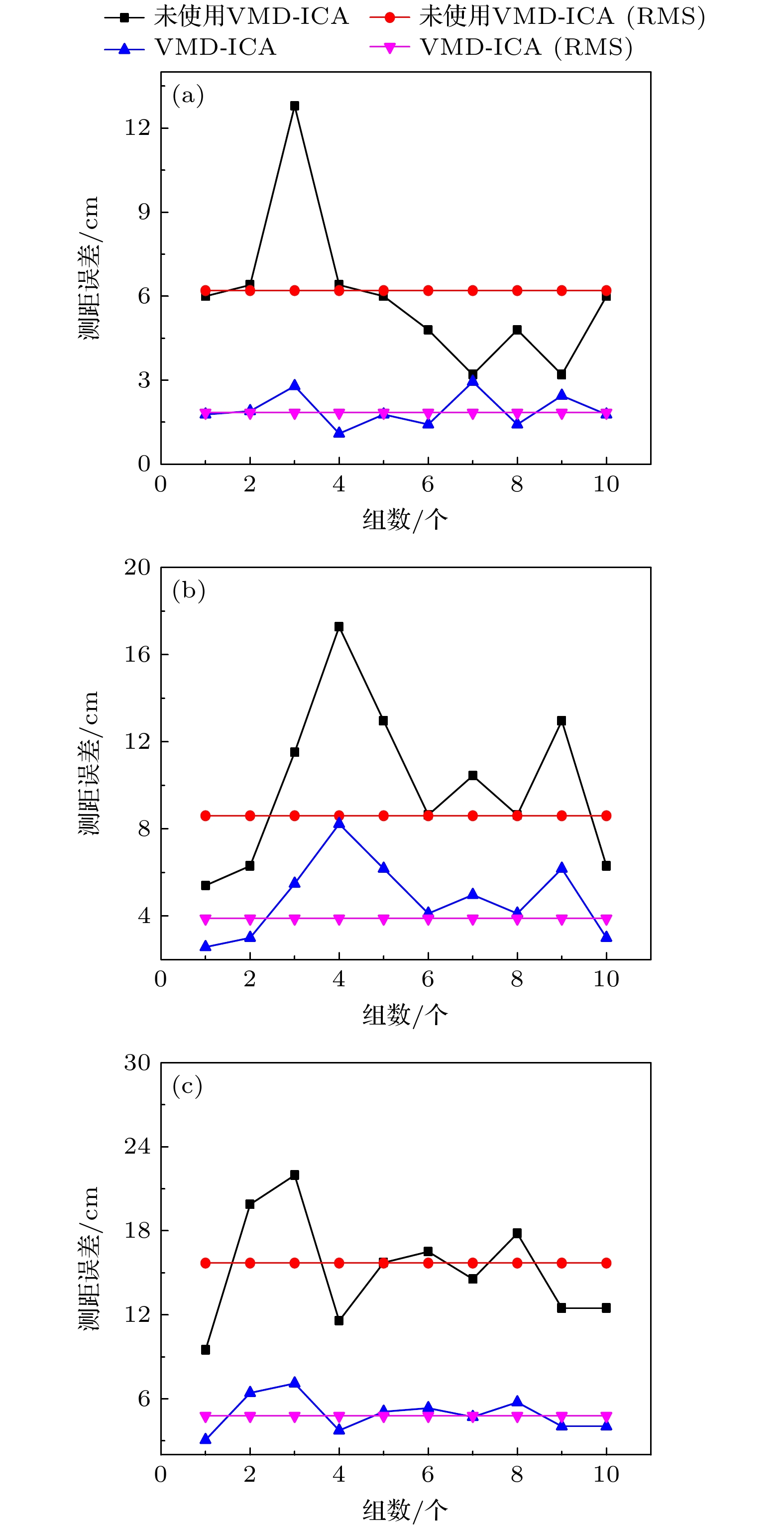
-
This paper proposes an underwater signal processing method based on the combination of variational mode decomposition (VMD) and independent component analysis (ICA). In this method, the VMD is used to decompose a set of echo signals into groups of eigenmodal signals arranged according to the order of their frequencies. These modal signals are then used as an observation matrix for ICA to ensure the integrity of the separated target echo signals. In this method, the correlation between the decomposed modal signals and the original signal is used to select the signals which are used as input matrix rows for ICA. The signal-to-clutter ratio is used to determine the number of decomposed layers. The ICA is used to separate the scattering and target echoes, so as to recover the weak target echoes in the strongly scattered water and greatly improve the ranging accuracy. A 532 nm intensity-modulated continuous wave (CW) laser is used in the underwater ranging experiments. The attenuation coefficient of the water is changed by adding Mg(OH)2, ranging experiments are carried out at different attenuation lengths. The experimental results show that the signal processing method can be used to successfully measure the target within 9 AL (attenuation length) when the laser output power is 2.3 W, and the ranging accuracy is improved from 16 cm to less than 5 cm by using the algorithm. The proposed method can be applied to underwater lidar application in turbid water and long distance ranging underwater where the scattering dominates the echoes.
[1] 梁喆, 彭苏萍, 郑晶 2014 计算机工程与应用 50 7
Google Scholar
Liang J, Peng S P, Zheng J 2014 Comput. Eng. Appl. 50 7
Google Scholar
[2] Battista B M, Knapp C C, McGee T, Goebel V 2007 Geophysics 72 29
Google Scholar
[3] 贾瑞生, 赵同彬, 孙红梅 2015 地球 58 1013
Google Scholar
Jia R S, Zhao T P, Sun H H 2015 Chin. J. Geophys. 58 1013
Google Scholar
[4] 李月, 彭蛟龙, 马海涛 2013 地球 56 626
Google Scholar
Li Y, Peng J L, Ma H T 2013 Chin. J. Geophys. 56 626
Google Scholar
[5] Huang N E, Shen Z, Long S R, Wu M L, Shi H H, Zheng Q N, Yen N C, Tung C C, Liu H H 1998 Roy. Soc. A-Math. Phy. 454 903
Google Scholar
[6] 郑祖光, 刘莉红 2010 经验模态与小波分析及应用 (北京: 气象出版社) 第83页
Zheng Z G, Liu L H 2010 Empirical Modal Analysis and Wavelet Analysis and their Applications (Beijing: Meteorological Publishing House) p83
[7] 胡爱军, 孙敬敬, 向玲 2011 振动、测试与诊断 31 429
Google Scholar
Hu A J, Sun J J, Xiang L 2011 Vib. Test. Diag. 31 429
Google Scholar
[8] Dragomiretskiy K, Zosso D 2014 IEEE Trans. Signal Process. 62 531
Google Scholar
[9] Dey P, Satija U, Ramkumar B 2015 Annual IEEE India Conference (INDICON) New Delhi, India, December 17–20, 2015 p1
[10] 高艳丰, 朱永利, 闫红艳 2016 电工技术学报 31 24
Google Scholar
Gao Y F, Zhu Y L, Yan H 2016 Trans. China Electrotech. Soc. 31 24
Google Scholar
[11] 马增强, 李亚超, 刘政 2016 振动与冲击 35 134
Google Scholar
Ma Z Q, Li Y C, Liu Z 2016 Vib. Shock 35 134
Google Scholar
[12] 谢平, 杨芳梅, 李欣欣 2016 65 118701
Google Scholar
Xie P, Yang F M, Li X X 2016 Acta Phys. Sin. 65 118701
Google Scholar
[13] 唐贵基, 王晓龙 2015 西安交通大学学报 49 73
Google Scholar
Tang G J, Wang X L 2015 J. Xi'an Jiaotong Univ. 49 73
Google Scholar
[14] Comon P 1994 Signal Process. 36 287
Google Scholar
[15] Laubach M, Shuler M, Nicolelis M A L 1999 Nicolelis Neurosci. Meth. 94 141
Google Scholar
[16] Bell A J, Sejnowski T J 1995 Neurosci. Methods 7 1129
Google Scholar
[17] Hyvarnen A, Oja E 2000 Neural Networks 13 411
Google Scholar
[18] Hyvarnen A, Oja E 1997 Neural Comput. 9 1483
Google Scholar
[19] Vrabie V D, Mars I J, Lacoume J L 2004 Signal Process. 84 645
Google Scholar
[20] Nian R, Liu F, Bo B 2013 Sensors 13 9104
Google Scholar
[21] Illig D W, Jemison W D, Mullen L J 2016 Appl. Opt. 55 C25
Google Scholar
[22] Prasad R, Deo C R, Li Y 2018 Geoderma 330 136
Google Scholar
[23] Colominas M A, Schlotthauer G, Torres M E 2014 Biomed. Signal Proces. 14 19
Google Scholar
[24] Yu Y, D J Yu, Cheng J S 2006 Sound Vib. 294 269
Google Scholar
[25] Kim D, Kim S K 2012 Behev. Res. Methods 44 1239
Google Scholar
[26] Li K, Yang S H, Liao Y Q, Lin X T, Wang X, Zhang J Y, Li Z 2020 IEEE Photonics J. 12 1503811
Google Scholar
-
图 1 VMD分解过程
Figure 1. VMD decomposition process.
图 2 激光水下探测光学系统
Figure 2. Laser underwater detection optical system.
图 3 镜面目标回波信号波形对应频谱能量分布及VMD-ICA方法处理结果 (a), (b)入射窗回波信号(基准)及其对应的FFT频谱; (c), (d) 1.5 m处镜面目标回波信号及其对应的FFT频谱; (e)—(l)回波信号的变分模态分解结果及其逆傅里叶变换时域波形; (m), (n) ICA处理结果(信号部分)及对应FFT频谱
Figure 3. Spectral energy distribution corresponding to the waveform of mirror target echo signal and the processing results of VMD-ICA method: (a), (b) The reference signal and the corresponding FFT spectrum; (c), (d) mirror target echo and the corresponding FFT spectrum at 1.5 m; (e)–(l) spectra of IMFs of VMD and the corresponding inverse Fourier transform in time domain; (m), (n) the result of ICA (target echo) and the corresponding FFT spectrum.
图 4 对于衰减长度不同的水体, VMD-ICA方法测量的测量误差以及均方根 (a) 衰减长度为3; (b) 衰减长度为6; (c)衰减长度为9
Figure 4. Measurement errors and root mean square of the distance measurement method for water bodies with attenuation lengths of (a) 3, (b) 6, (c) 9 based on VMD-ICA ranging results.
-
[1] 梁喆, 彭苏萍, 郑晶 2014 计算机工程与应用 50 7
Google Scholar
Liang J, Peng S P, Zheng J 2014 Comput. Eng. Appl. 50 7
Google Scholar
[2] Battista B M, Knapp C C, McGee T, Goebel V 2007 Geophysics 72 29
Google Scholar
[3] 贾瑞生, 赵同彬, 孙红梅 2015 地球 58 1013
Google Scholar
Jia R S, Zhao T P, Sun H H 2015 Chin. J. Geophys. 58 1013
Google Scholar
[4] 李月, 彭蛟龙, 马海涛 2013 地球 56 626
Google Scholar
Li Y, Peng J L, Ma H T 2013 Chin. J. Geophys. 56 626
Google Scholar
[5] Huang N E, Shen Z, Long S R, Wu M L, Shi H H, Zheng Q N, Yen N C, Tung C C, Liu H H 1998 Roy. Soc. A-Math. Phy. 454 903
Google Scholar
[6] 郑祖光, 刘莉红 2010 经验模态与小波分析及应用 (北京: 气象出版社) 第83页
Zheng Z G, Liu L H 2010 Empirical Modal Analysis and Wavelet Analysis and their Applications (Beijing: Meteorological Publishing House) p83
[7] 胡爱军, 孙敬敬, 向玲 2011 振动、测试与诊断 31 429
Google Scholar
Hu A J, Sun J J, Xiang L 2011 Vib. Test. Diag. 31 429
Google Scholar
[8] Dragomiretskiy K, Zosso D 2014 IEEE Trans. Signal Process. 62 531
Google Scholar
[9] Dey P, Satija U, Ramkumar B 2015 Annual IEEE India Conference (INDICON) New Delhi, India, December 17–20, 2015 p1
[10] 高艳丰, 朱永利, 闫红艳 2016 电工技术学报 31 24
Google Scholar
Gao Y F, Zhu Y L, Yan H 2016 Trans. China Electrotech. Soc. 31 24
Google Scholar
[11] 马增强, 李亚超, 刘政 2016 振动与冲击 35 134
Google Scholar
Ma Z Q, Li Y C, Liu Z 2016 Vib. Shock 35 134
Google Scholar
[12] 谢平, 杨芳梅, 李欣欣 2016 65 118701
Google Scholar
Xie P, Yang F M, Li X X 2016 Acta Phys. Sin. 65 118701
Google Scholar
[13] 唐贵基, 王晓龙 2015 西安交通大学学报 49 73
Google Scholar
Tang G J, Wang X L 2015 J. Xi'an Jiaotong Univ. 49 73
Google Scholar
[14] Comon P 1994 Signal Process. 36 287
Google Scholar
[15] Laubach M, Shuler M, Nicolelis M A L 1999 Nicolelis Neurosci. Meth. 94 141
Google Scholar
[16] Bell A J, Sejnowski T J 1995 Neurosci. Methods 7 1129
Google Scholar
[17] Hyvarnen A, Oja E 2000 Neural Networks 13 411
Google Scholar
[18] Hyvarnen A, Oja E 1997 Neural Comput. 9 1483
Google Scholar
[19] Vrabie V D, Mars I J, Lacoume J L 2004 Signal Process. 84 645
Google Scholar
[20] Nian R, Liu F, Bo B 2013 Sensors 13 9104
Google Scholar
[21] Illig D W, Jemison W D, Mullen L J 2016 Appl. Opt. 55 C25
Google Scholar
[22] Prasad R, Deo C R, Li Y 2018 Geoderma 330 136
Google Scholar
[23] Colominas M A, Schlotthauer G, Torres M E 2014 Biomed. Signal Proces. 14 19
Google Scholar
[24] Yu Y, D J Yu, Cheng J S 2006 Sound Vib. 294 269
Google Scholar
[25] Kim D, Kim S K 2012 Behev. Res. Methods 44 1239
Google Scholar
[26] Li K, Yang S H, Liao Y Q, Lin X T, Wang X, Zhang J Y, Li Z 2020 IEEE Photonics J. 12 1503811
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 2243
- PDF Downloads: 22
- Cited By: 0
