Stability analysis of ring-like cavitation bubble cluster structure in standing wave field

Lei Zhao-Kang; Wu Yao-Rong; Huang Chen-Yang; Mo Run-Yang; Shen Zhuang-Zhi; Wang Cheng-Hui; Guo Jian-Zhong; Lin Shu-Yu

doi:10.7498/aps.73.20231956

Abstract

Multi-cavitation bubble system can easily produce cavitation clouds with various structure types, including ring-like cavitation structures. Nonetheless, the evolutionary behavior of the structure and the physical mechanism of its formation are less investigated. In this work, high-speed photography and image analysis techniques are used to study the evolution of ring-like cavitation bubble aggregation structure in an ultrasonic cleaning tank with a frequency of 40 kHz. The ring-like structure usually appears near the pressure nodule, and its radius is less than a one-eighth wavelength. The structure involves establishment, stability and disappearance during an envelope wave period, and its morphology is stable. The ring-like cavitation structure exists as a bubble transport phenomenon, and the formed small bubble clusters flow to the outside of the ring and become discrete cavitation bubbles, or the bubble nuclei rejoin the cycle of bubble transport in the main accumulation area of the bubble. The size of the ring structure and the bubble accumulation area oscillate slightly, and there exists the whole structure rotation phenomenon, which depends on the interaction of the main sound field and the secondary radiation field with the bubbles. Furthermore, in this work, a mathematical model of two bubbles is used to investigate the physical mechanism behind the formation of a ring. It is found that the sound field is a key factor in ring formation. The ring chain model is used to analyze the structural stability by taking into account the time delay caused by the secondary acoustic radiation of the bubble. The numerical results show that the equivalent potential energy distribution of a ring bubble chain with a one-eighth wavelength in radius can stabilize each bubble in the potential well, and the radial distribution presents a ring-like barrier structure. The higher the sound pressure, the greater the equivalent potential, and the more the bubbles are clustered. The higher the driving sound field, the more complete the ring chain structure is. However, high sound pressure may cause the agglomeration of bubbles with high number density to disintegrate the stability of the ring aggregation of bubbles and evolve into other types of bubble aggregation structures. The theoretical results are in good consistence with the experimental phenomena.

Keywords:

Authors and contacts

Institute of Shaanxi Key Laboratory of Ultrasonics, Shaanxi Normal University, Xi’an 710062, China

Corresponding author: Wang Cheng-Hui, wangld001@snnu.edu.cn ; Guo Jian-Zhong, guojz@snnu.edu.cn ;

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12374441, 11974232).

[1]	陈伟中 2014 声空化物理 (北京: 科技出版社) 第140页 Chen W Z 2014 Acoustic Cavitation Physics (Beijing: Science press) p140
[2]	Behnia S, Jafari A, Soltanpoor W, Jahanbakhsh O 2009 Chaos Soliton. Fract. 41 818 Google Scholar
[3]	Chahine G L, Kapahi A, Choi J K, Hsiao C T 2016 Ultrason. Sonochem. 29 528 Google Scholar
[4]	Zhang Y, Wu W H, Wang J Y, Zhai W 2022 Acta Phys. Sin. 71 244303 [张颖, 吴文华, 王建元, 翟薇 2022 71 244303]Google Scholar Zhang Y, Wu W H, Wang J Y, Zhai W 2022 Acta Phys. Sin. 71 244303 Google Scholar
[5]	Mettin R 2005 Mod. Trends Appl. Res. Signpost 1
[6]	Mettin R, Luther S, Ohl C D, Lauterborn W 1999 Ultrason. Sonochem. 6 25 Google Scholar
[7]	Lauterborn W, Kurz T, Mettin R, Ohl C D 1999 Adv. Chem. Phys. 110 295 Google Scholar
[8]	Hatanaka S I, Yasui K, Kozuka T, Tuziuti T, Mitome H 2002 Ultrasonics 40 655 Google Scholar
[9]	Fatjó G G A, Torres Pérez A, Hadfield M 2010 Ultrason. Sonochem. 17 73 Google Scholar
[10]	Yamashita T, Ando K 2019 Ultrason. Sonochem. 52 268 Google Scholar
[11]	Alloul M, Dollet B, Stephan O, Bossy E, Quilliet C, Marmottant P 2022 Phys. Rev. Lett 129 134501 Google Scholar
[12]	Zhang A M, Li S M, Cui P, Li S, Liu Y L 2023 Phys. Fluids 35 033323 Google Scholar
[13]	Zhang A M, Li S M, Cui P, Li S, Liu Y L 2023 Theor. Appl. Mech. Lett. 13 100438 Google Scholar
[14]	Wu P F, Bai L X, Lin W J, Yan J C 2017 Ultrason. Sonochem. 38 75 Google Scholar
[15]	白立新, 吴鹏飞, 李超, 邓京军, 曾志杰 2018 应用声学 37 614 Google Scholar Bai L X, Wu P F, Li C, Deng J J, Zeng Z J 2018 Appl. Acoust. 37 614 Google Scholar
[16]	李凡, 张先梅, 田华, 胡静, 陈时, 王成会, 郭建中, 莫润阳 2022 71 084303 Google Scholar Li F, Zhang X M, Tian H, Hu J, Chen S, Wang C H, Guo J Z, Mo R Y 2022 Acta Phys. Sin. 71 084303 Google Scholar
[17]	Bai L C, Sun J G, Gao Y D, Xu W L, Zeng Z J, Ma Y H, Bai L X 2021 Ultrason. Sonochem. 74 105577 Google Scholar
[18]	白立春, 孙劲光, 高艳东 2021 70 054701 Google Scholar Bai L C, Sun J G, Gao Y D 2021 Acta Phys. Sin 70 054701 Google Scholar
[19]	许龙, 汪尧 2023 72 024303 Google Scholar Xu L, Wang Y 2023 Acta Phys. Sin. 72 024303 Google Scholar
[20]	Zhang L L, Chen W Z, Shen Y, Wu Y R, Zhao G Y, Kou S Y 2022 Chin. Phys. B 31 044303 Google Scholar
[21]	Wu W H, Yang P F, Zhai W, Wei B B 2019 Chin. Phys. Lett. 36 084302 Google Scholar
[22]	Avvaru B, Pandit A B 2009 Ultrason. Sonochem. 16 105 Google Scholar
[23]	李希胜王绍纯 2014 自动检测技术 (北京: 冶金工业出版社) 第226页 Li X S, Wang S Z 2014 Automatic Measurement Technique (Beijing: Metallurgical Industry Press) p226
[24]	Li F, Zhang X M, Tian H, Hu J, Chen S, Mo R Y, Wang C H, Guo J Z 2022 Ultrason. Sonochem. 87 106057 Google Scholar
[25]	Li F, Huang C Y, Zhang X M, Wang C H, Guo J Z, Lin S Y, Shen Z Z, Tian H 2023 Ultrasonics 132 106992 Google Scholar
[26]	Li F, Huang C Y, Zhang X M, Wang C H, Hu J, Chen S, Tian H, Shen Z Z, Guo J Z, Lin S Y 2023 Ultrason. Sonochem. 98 106500 Google Scholar
[27]	An Y 2011 Phys. Rev. E 83 066313 Google Scholar
[28]	Zhang W J, An Y 2013 Phy. Rev. E 87 053023 Google Scholar
[29]	Mettin R, Ohl C D, Lauterborn W 1999 Ultrasonics 524 139 Google Scholar
[30]	沈壮志 2015 64 124702 Google Scholar Shen Z Z 2015 Acta Phys. Sin 64 124702 Google Scholar

Cited By

图 1 实验装置示意图

Figure 1. Schematic diagram of experimental apparatus.

DownLoad: Full-Size Img PowerPoint

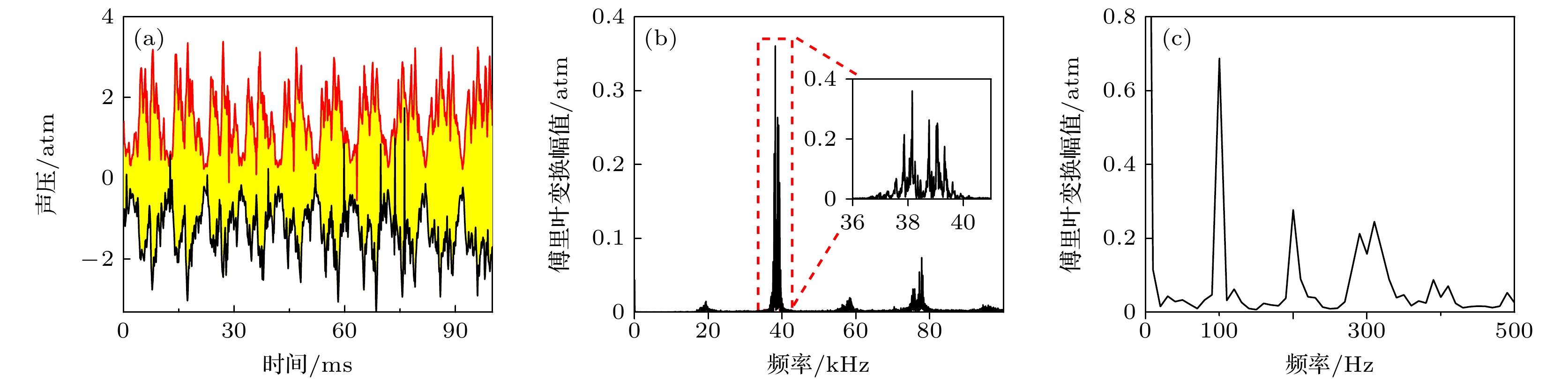
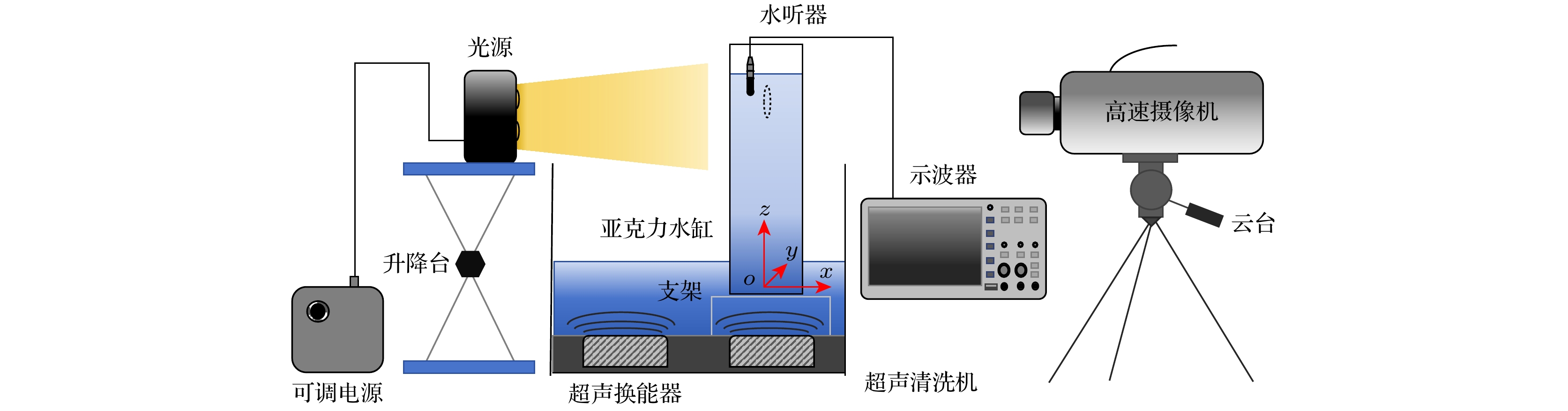
图 2 液体的空化信号　(a) 波形图(红色和黑色曲线分别为空化场信号的上下包络线); (b) 空化信号幅度谱; (c) 空化信号上包络线的幅度谱

Figure 2. Cavitation signal of liquid: (a) Waveform diagram (the red and black curves are the upper and lower envelope lines of the cavitation field signal, respectively); (b) amplitude spectrum of cavitation signal; (c) amplitude spectrum of envelope on cavitation signal.

DownLoad: Full-Size Img PowerPoint

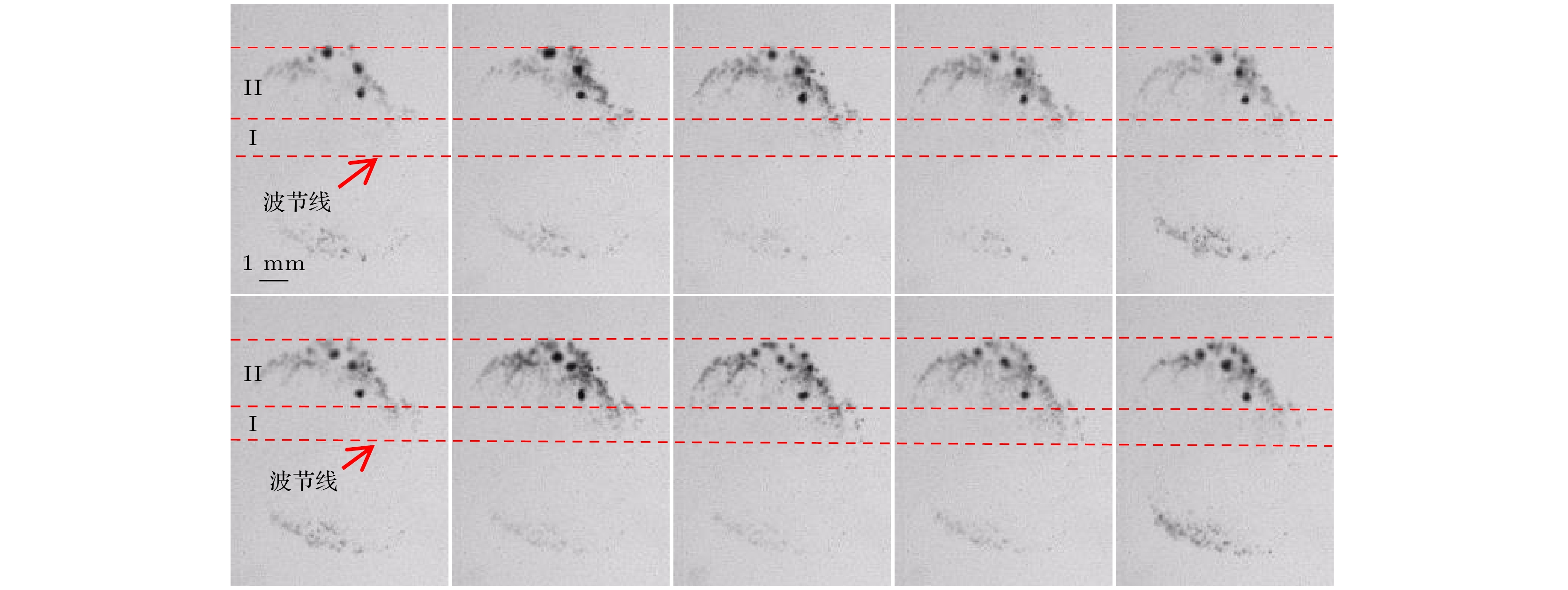
图 3 环状空化结构演化时间序列(代表帧选自补充材料视频1(//m.suprmerch.com/article/doi/10.7498/aps.73.20231956)), 图中红色虚线用于区分上环状结构分布的不同区域形貌

Figure 3. Ring-like cavitation structure evolution time series (representative frame selected from supplementary video 1 (//m.suprmerch.com/article/doi/10.7498/aps.73.20231956)), the red dotted line in the figure is used to distinguish the topography of different regions of the upper ring-like structure distribution.

DownLoad: Full-Size Img PowerPoint

图 4 包络波周期对环形结构演化行为的影响(虚线椭圆用于标注气泡聚集区, 实线圆圈用于标注气泡聚集区内的小泡团, 代表帧选自补充材料视频1 (//m.suprmerch.com/article/doi/10.7498/aps.73.20231956))

Figure 4. Influence of envelope wave period on evolution behavior of ring structure (the dotted ellipse is used to mark the bubble aggregation area, and the solid circle is used to mark the bubble clusters in the bubble aggregation area, representative frame selected from Supplementary Video 1 (//m.suprmerch.com/article/doi/10.7498/aps.73.20231956)).

DownLoad: Full-Size Img PowerPoint

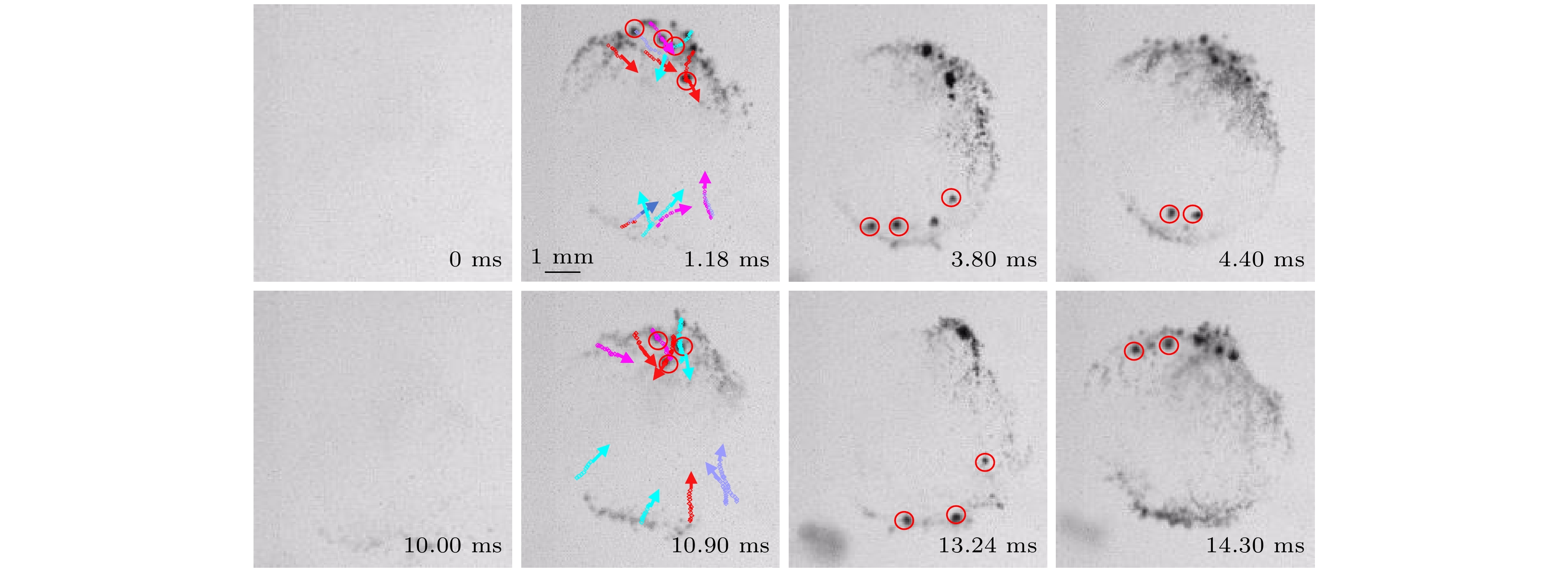
图 5 小气泡团在环状空化结构中的运动轨迹追踪(代表帧选自补充材料视频1 (//m.suprmerch.com/article/doi/10.7498/aps.73.20231956)), 红色圆圈标注被追踪的小泡团, 其他带箭头的标志线代表各气泡团的运动方向和轨迹

Figure 5. Trajectory tracking of small bubble clusters in ring-like cavitation structures (representative frame selected from Supplementary Video 1 (//m.suprmerch.com/article/doi/10.7498/aps.73.20231956)). The red circle marks the tracked bubble clusters, and other marked lines with arrows represent the movement direction and trajectory of each bubble cluster.

DownLoad: Full-Size Img PowerPoint

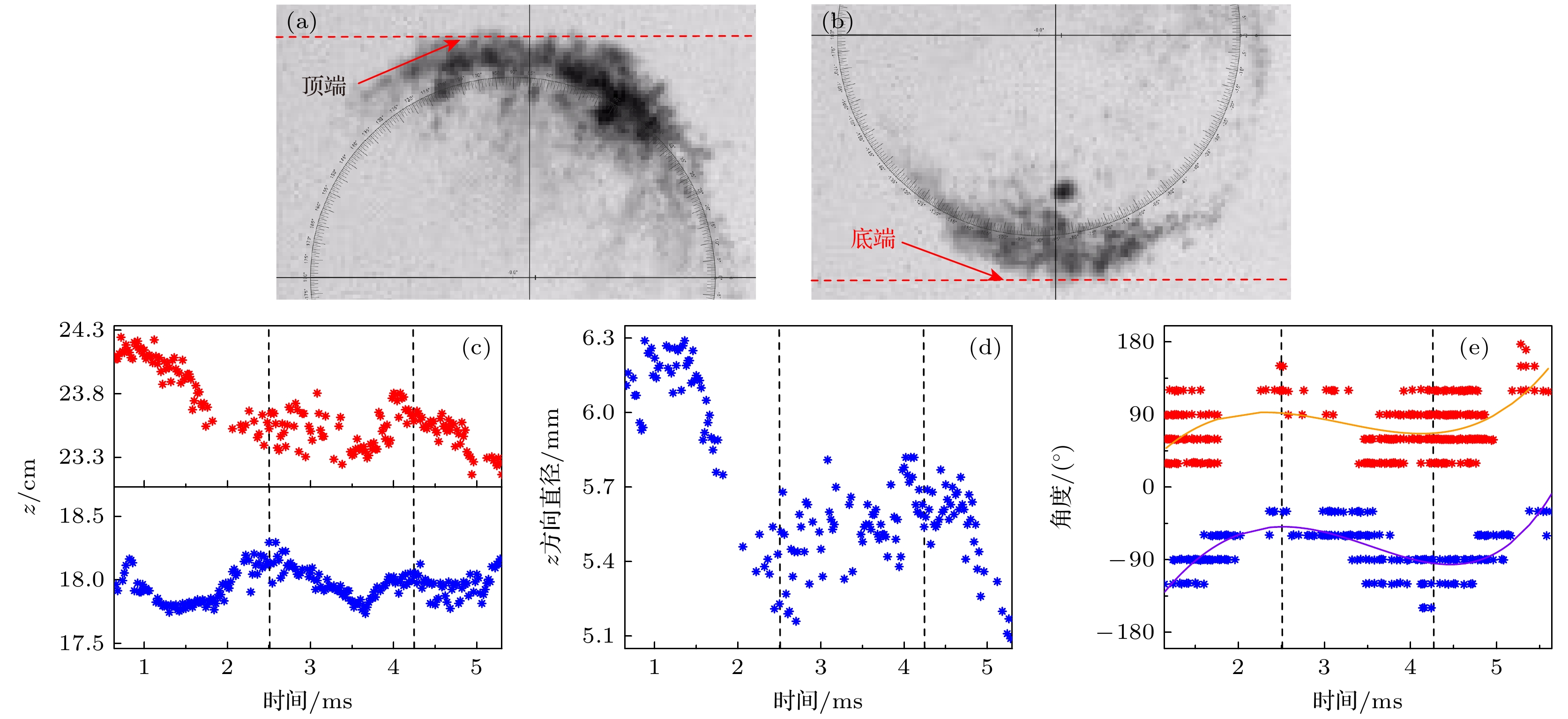
图 6 环状空化结构的演化规律, 追踪时间段为补充材料视频1 (//m.suprmerch.com/article/doi/10.7498/aps.73.20231956)的前6 ms (a), (b)环状结构气泡追踪示意图; (c)环状结构弧形顶端和底端位置变化; (d)结构直径变化(左右垂直虚线用于标注直径相对稳定变化时间段); (e)小泡团聚集位置所在的环结构角度分布

Figure 6. The evolution law of the ring-like cavitation structure and the tracking period are in the first 6 ms of Supplementary Video 1 (//m.suprmerch.com/article/doi/10.7498/aps.73.20231956): (a), (b) Schematic diagram of ring-like structure bubble tracking; (c) changes in the position of the top and bottom of the arc of the ring structure; (d) changes in structural diameter (the left and right vertical dashed lines are used to mark the period of relatively stable change in diameter.); (e) angle distribution of a ring-like structure where small bubble clusters gather.

DownLoad: Full-Size Img PowerPoint

图 7 波节两侧双泡相互作用示意图

Figure 7. Diagram of two bubbles located on both sides of the wave node.

DownLoad: Full-Size Img PowerPoint

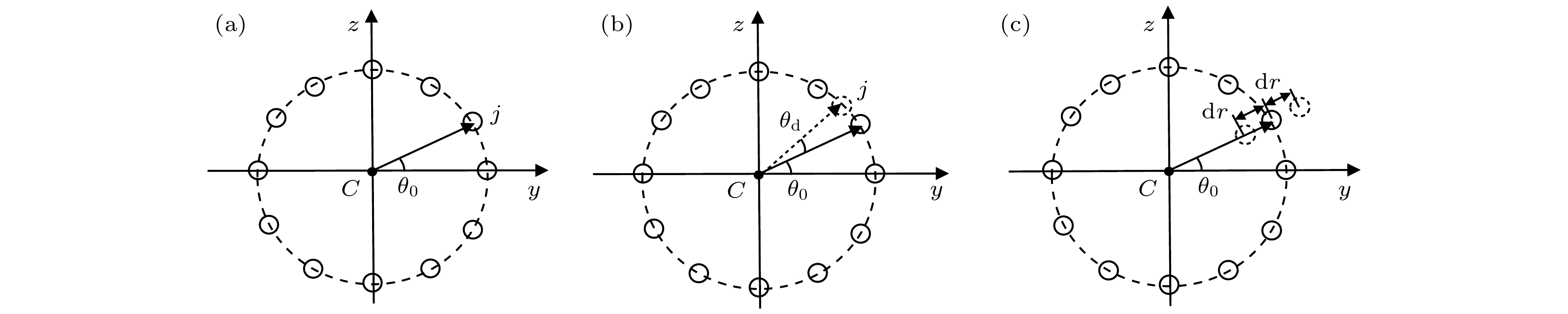
图 8 环形气泡链的结构示意图　(a)气泡在环形气泡链上的分布示意图; (b)计算等效势能θ方向分布的示意图; (c)计算等效势能径向分布的示意图

Figure 8. Structural diagram of the ring bubble chain: (a) Distribution diagram of the bubble on the ring bubble chain; (b) schematic diagram for calculating the distribution of the equivalent potential in the direction of θ; (c) schematic diagram for calculating the radial distribution of the equivalent potential.

DownLoad: Full-Size Img PowerPoint

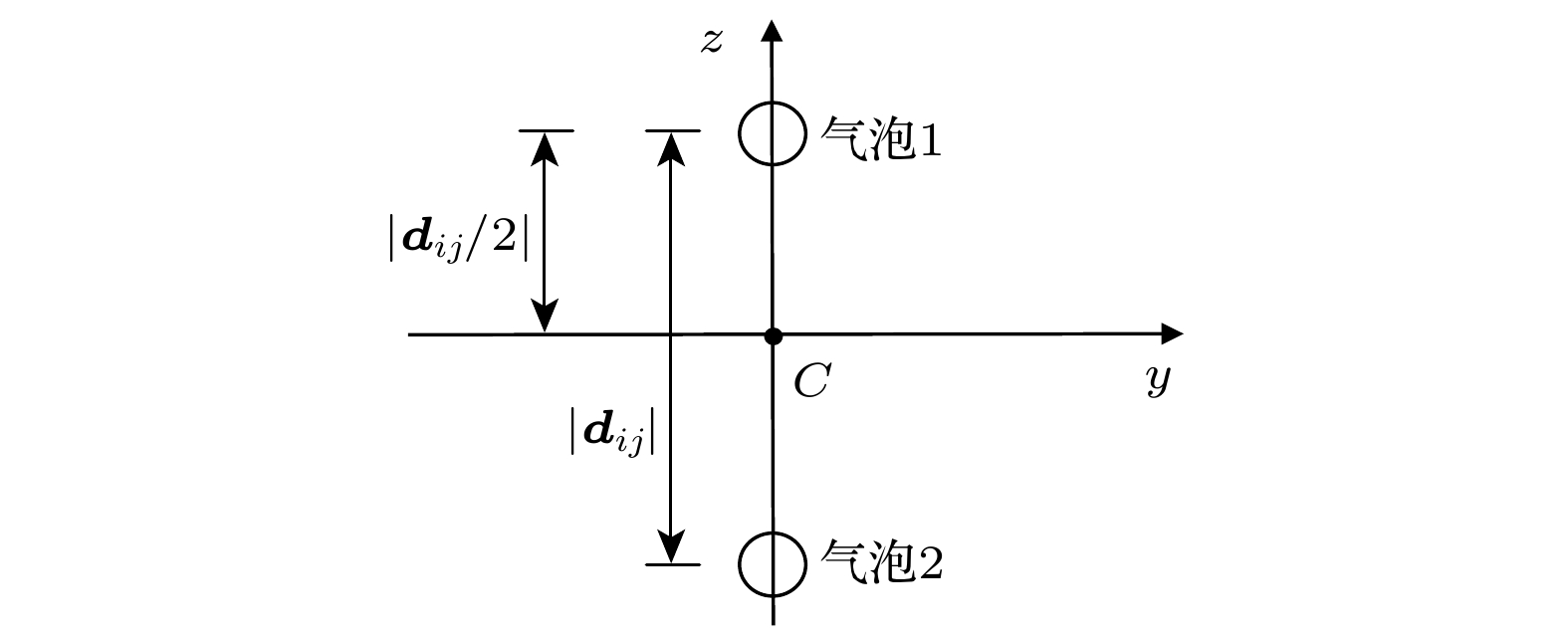
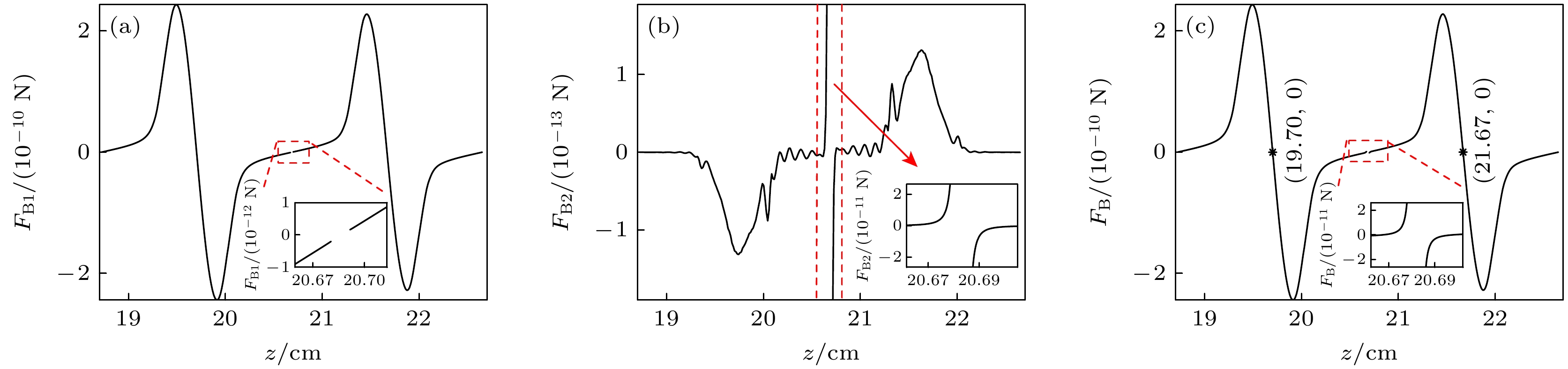
图 9 波节两侧对称耦合双泡在驻波场中的受力情况　(a) 所受的主Bjerknes力; (b)次Bjerknes力; (c)主Bjerknes力与次Bjerknes力的合力

Figure 9. The force of a symmetrically coupled double bubble on both sides of the node in a standing wave field: (a) The primary Bjerknes force; (b) the secondary Bjerknes force; (c) the resultant of the primary Bjerknes force and the secondary Bjerknes force.

DownLoad: Full-Size Img PowerPoint

图 10 等效势在环形气泡链圆周上θ方向的分布　(a) 圆周上的分布; (b) 90°附近的分布; (c) 270°附近的分布

Figure 10. The distribution of the equivalent potential in the θ direction along the circumference of the ring bubble chain: (a) The distribution on the circumference; (b) distribution near 90°; (c) distribution near 270°

DownLoad: Full-Size Img PowerPoint

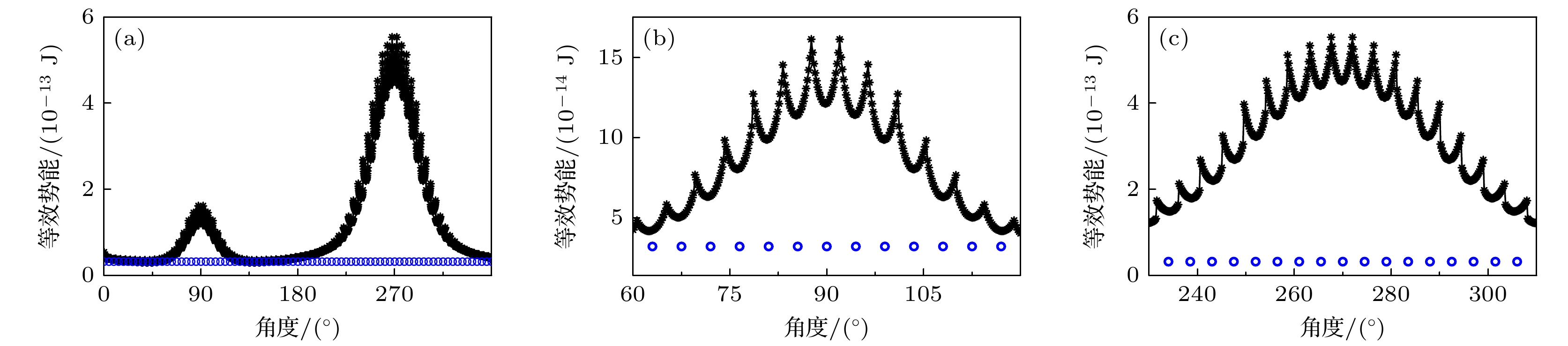
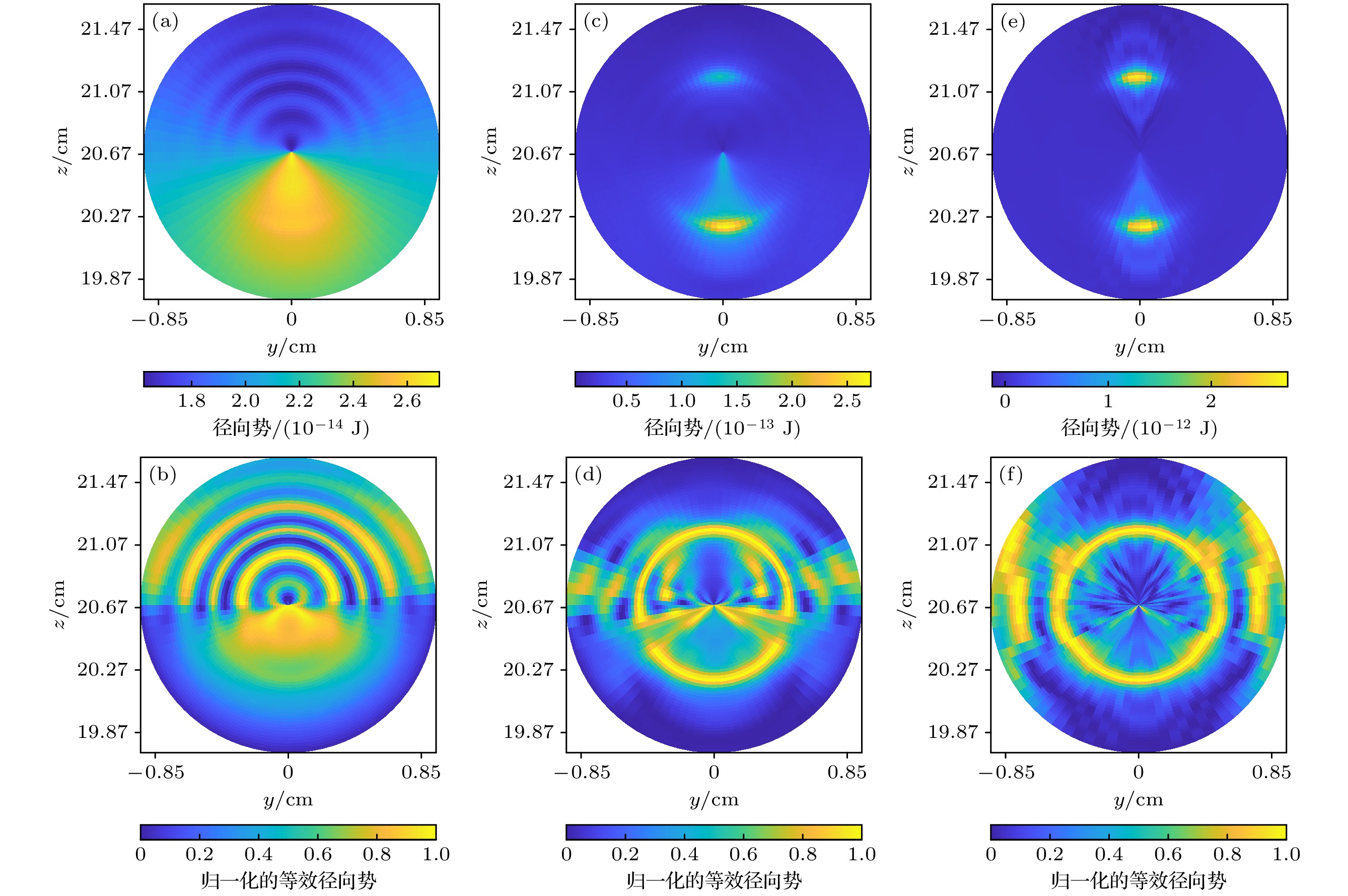
图 11 环形气泡链上等效势的径向分布　(a), (b), (c) 等效势的径向分布; (d), (e), (f)归一化等效势的径向分布; (a), (d) p_A = 0.217 atm; (b), (e) p_A = 0.655 atm; (c), (f) p_A = 0.755 atm

Figure 11. The radial distribution of the equivalent potential on the ring bubble chain: (a), (b), (c) The radial distribution of the equivalent potential; (d), (e), (f) the radial distribution of the normalized equivalent potential; (a), (d) p_A = 0.217 atm; (b), (e) p_A = 0.655 atm; (c), (f) p_A = 0.755 atm.

DownLoad: Full-Size Img PowerPoint

map

[1]	陈伟中 2014 声空化物理 (北京: 科技出版社) 第140页 Chen W Z 2014 Acoustic Cavitation Physics (Beijing: Science press) p140
[2]	Behnia S, Jafari A, Soltanpoor W, Jahanbakhsh O 2009 Chaos Soliton. Fract. 41 818 Google Scholar
[3]	Chahine G L, Kapahi A, Choi J K, Hsiao C T 2016 Ultrason. Sonochem. 29 528 Google Scholar
[4]	Zhang Y, Wu W H, Wang J Y, Zhai W 2022 Acta Phys. Sin. 71 244303 [张颖, 吴文华, 王建元, 翟薇 2022 71 244303]Google Scholar Zhang Y, Wu W H, Wang J Y, Zhai W 2022 Acta Phys. Sin. 71 244303 Google Scholar
[5]	Mettin R 2005 Mod. Trends Appl. Res. Signpost 1
[6]	Mettin R, Luther S, Ohl C D, Lauterborn W 1999 Ultrason. Sonochem. 6 25 Google Scholar
[7]	Lauterborn W, Kurz T, Mettin R, Ohl C D 1999 Adv. Chem. Phys. 110 295 Google Scholar
[8]	Hatanaka S I, Yasui K, Kozuka T, Tuziuti T, Mitome H 2002 Ultrasonics 40 655 Google Scholar
[9]	Fatjó G G A, Torres Pérez A, Hadfield M 2010 Ultrason. Sonochem. 17 73 Google Scholar
[10]	Yamashita T, Ando K 2019 Ultrason. Sonochem. 52 268 Google Scholar
[11]	Alloul M, Dollet B, Stephan O, Bossy E, Quilliet C, Marmottant P 2022 Phys. Rev. Lett 129 134501 Google Scholar
[12]	Zhang A M, Li S M, Cui P, Li S, Liu Y L 2023 Phys. Fluids 35 033323 Google Scholar
[13]	Zhang A M, Li S M, Cui P, Li S, Liu Y L 2023 Theor. Appl. Mech. Lett. 13 100438 Google Scholar
[14]	Wu P F, Bai L X, Lin W J, Yan J C 2017 Ultrason. Sonochem. 38 75 Google Scholar
[15]	白立新, 吴鹏飞, 李超, 邓京军, 曾志杰 2018 应用声学 37 614 Google Scholar Bai L X, Wu P F, Li C, Deng J J, Zeng Z J 2018 Appl. Acoust. 37 614 Google Scholar
[16]	李凡, 张先梅, 田华, 胡静, 陈时, 王成会, 郭建中, 莫润阳 2022 71 084303 Google Scholar Li F, Zhang X M, Tian H, Hu J, Chen S, Wang C H, Guo J Z, Mo R Y 2022 Acta Phys. Sin. 71 084303 Google Scholar
[17]	Bai L C, Sun J G, Gao Y D, Xu W L, Zeng Z J, Ma Y H, Bai L X 2021 Ultrason. Sonochem. 74 105577 Google Scholar
[18]	白立春, 孙劲光, 高艳东 2021 70 054701 Google Scholar Bai L C, Sun J G, Gao Y D 2021 Acta Phys. Sin 70 054701 Google Scholar
[19]	许龙, 汪尧 2023 72 024303 Google Scholar Xu L, Wang Y 2023 Acta Phys. Sin. 72 024303 Google Scholar
[20]	Zhang L L, Chen W Z, Shen Y, Wu Y R, Zhao G Y, Kou S Y 2022 Chin. Phys. B 31 044303 Google Scholar
[21]	Wu W H, Yang P F, Zhai W, Wei B B 2019 Chin. Phys. Lett. 36 084302 Google Scholar
[22]	Avvaru B, Pandit A B 2009 Ultrason. Sonochem. 16 105 Google Scholar
[23]	李希胜王绍纯 2014 自动检测技术 (北京: 冶金工业出版社) 第226页 Li X S, Wang S Z 2014 Automatic Measurement Technique (Beijing: Metallurgical Industry Press) p226
[24]	Li F, Zhang X M, Tian H, Hu J, Chen S, Mo R Y, Wang C H, Guo J Z 2022 Ultrason. Sonochem. 87 106057 Google Scholar
[25]	Li F, Huang C Y, Zhang X M, Wang C H, Guo J Z, Lin S Y, Shen Z Z, Tian H 2023 Ultrasonics 132 106992 Google Scholar
[26]	Li F, Huang C Y, Zhang X M, Wang C H, Hu J, Chen S, Tian H, Shen Z Z, Guo J Z, Lin S Y 2023 Ultrason. Sonochem. 98 106500 Google Scholar
[27]	An Y 2011 Phys. Rev. E 83 066313 Google Scholar
[28]	Zhang W J, An Y 2013 Phy. Rev. E 87 053023 Google Scholar
[29]	Mettin R, Ohl C D, Lauterborn W 1999 Ultrasonics 524 139 Google Scholar
[30]	沈壮志 2015 64 124702 Google Scholar Shen Z Z 2015 Acta Phys. Sin 64 124702 Google Scholar

[1]	Liu Rui, Huang Chen-Yang, Wu Yao-Rong, Hu Jing, Mo Run-Yang, Wang Cheng-Hui. Structural stability analysis of spherical bubble clusters in acoustic cavitation fields. Acta Physica Sinica, 2024, 73(8): 084303. doi: 10.7498/aps.73.20232008
[2]	Xu Long, Wang Yao. Simulation of dynamic process of double bubble coupled acoustic cavitation. Acta Physica Sinica, 2023, 72(2): 024303. doi: 10.7498/aps.72.20221571
[3]	Li Fan, Zhang Xian-Mei, Tian Hua, Hu Jing, Chen Shi, Wang Cheng-Hui, Guo Jian-Zhong, Mo Run-Yang. Structure stability of cyclic chain-like cavitation cloud in thin liquid layer. Acta Physica Sinica, 2022, 71(8): 084303. doi: 10.7498/aps.71.20212257
[4]	Yang Xue, Ding Da-Jun, Hu Zhan, Zhao Guo-Ming. Theoretical study on the structure and stability of neutral and cationic butanone clusters. Acta Physica Sinica, 2018, 67(3): 033601. doi: 10.7498/aps.67.20171862
[5]	Wang Zhuan-Yu, Kang Wei-Li, Jia Jian-Feng, Wu Hai-Shun. Structure and stability of Ti2Bn (n=1-10) clusters: an ab initio investigation. Acta Physica Sinica, 2014, 63(23): 233102. doi: 10.7498/aps.63.233102
[6]	Lü Jin, Yang Li-Jun, Wang Yan-Fang, Ma Wen-Jin. Density functional theory study of structure characteristics and stabilities of Al2Sn(n=2-10) clusters. Acta Physica Sinica, 2014, 63(16): 163601. doi: 10.7498/aps.63.163601
[7]	Hu Jing, Lin Shu-Yu, Wang Ceng-Hui, Li Jin. Study of resonance sound response for bubble cluster in ultrasonic field. Acta Physica Sinica, 2013, 62(13): 134303. doi: 10.7498/aps.62.134303
[8]	Wang Yong, Lin Shu-Yu, Mo Run-Yang, Zhang Xiao-Li. Vibration of the bubble in bubbly liquids. Acta Physica Sinica, 2013, 62(13): 134304. doi: 10.7498/aps.62.134304
[9]	Wang Can-Jun, Li Jiang-Cheng, Mei Dong-Cheng. Effect of noises on the stability of a metapopulation. Acta Physica Sinica, 2012, 61(12): 120506. doi: 10.7498/aps.61.120506
[10]	Xu Bo, Wang Shu-Lin, Li Sheng-Juan, Li Lai-qiang. Study of synthesis and mechanism of MgFe2O4 nanoparticles by ultrasonic Intensify. Acta Physica Sinica, 2012, 61(3): 030703. doi: 10.7498/aps.61.030703
[11]	Song Jian, Li Feng, Deng Kai-Ming, Xiao Chuan-Yun, Kan Er-Jun, Lu Rui-Feng, Wu Hai-Ping. Density functional study on the stability and electronic structure of single layer Si6H4Ph2. Acta Physica Sinica, 2012, 61(24): 246801. doi: 10.7498/aps.61.246801
[12]	Zhang Xi, Bao Bo-Cheng, Wang Jin-Ping, Ma Zheng-Hua, Xu Jian-Ping. Stability analysis of equivalent series resistance of output capacitor in fixed off-time controlled Buck converter. Acta Physica Sinica, 2012, 61(16): 160503. doi: 10.7498/aps.61.160503
[13]	Jin Rong, Chen Xiao-Hong. Structures and stabilities of VOxH2O (x= 15) clusters. Acta Physica Sinica, 2012, 61(9): 093103. doi: 10.7498/aps.61.093103
[14]	Tang Hui-Shuai, Zhang Xiu-Rong, Kang Zhang-Li, Wu Li-Qing. Theoretical study of geometry structures and stability of OsnN0,±(n=1—6) clusters. Acta Physica Sinica, 2011, 60(5): 053601. doi: 10.7498/aps.60.053601
[15]	Lu Yi-Gang, Wu Xiong-Hui. Computational analysis of double-bubble ultrasonic cavitation. Acta Physica Sinica, 2011, 60(4): 046202. doi: 10.7498/aps.60.046202
[16]	Cui Jian, Luo Ji-Run, Zhu Min, Guo Wei. Analyses for the stability of multi-gap Hughes-type coupled cavity. Acta Physica Sinica, 2011, 60(6): 061101. doi: 10.7498/aps.60.061101
[17]	Ouyang Yu, Peng Jing-Cui, Wang　Hui, Yi Shuang-Ping. Study on the stability of carbon nanotubes. Acta Physica Sinica, 2008, 57(1): 615-620. doi: 10.7498/aps.57.615
[18]	Wang Yan, Han Xiao-Yan, Ren Hui-Zhi, Hou Guo-Fu, Guo Qun-Chao, Zhu Feng, Zhang De-Kun, Sun Jian, Xue Jun-Ming, Zhao Ying, Geng Xin-Hua. Stability of mixed phase silicon thin film material under light soaking. Acta Physica Sinica, 2006, 55(2): 947-951. doi: 10.7498/aps.55.947
[19]	Yang Jian-Song, Li Bao-Xing. Study of the stability of gallium-arsenic ion clusters. Acta Physica Sinica, 2006, 55(12): 6562-6569. doi: 10.7498/aps.55.6562
[20]	Chen Qian, Zou Xin-Ye, Cheng Jian-Chun. Investigation of bubble dynamics in ultrasonic sonoporation. Acta Physica Sinica, 2006, 55(12): 6476-6481. doi: 10.7498/aps.55.6476

8-20231956-Suppl video1.mp4

8-20231956-Suppl video2.mp4

Catalog

Vol. 73, Issue 8 - 2024-04-20

Metrics

Abstract views: 2431
PDF Downloads: 46
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板