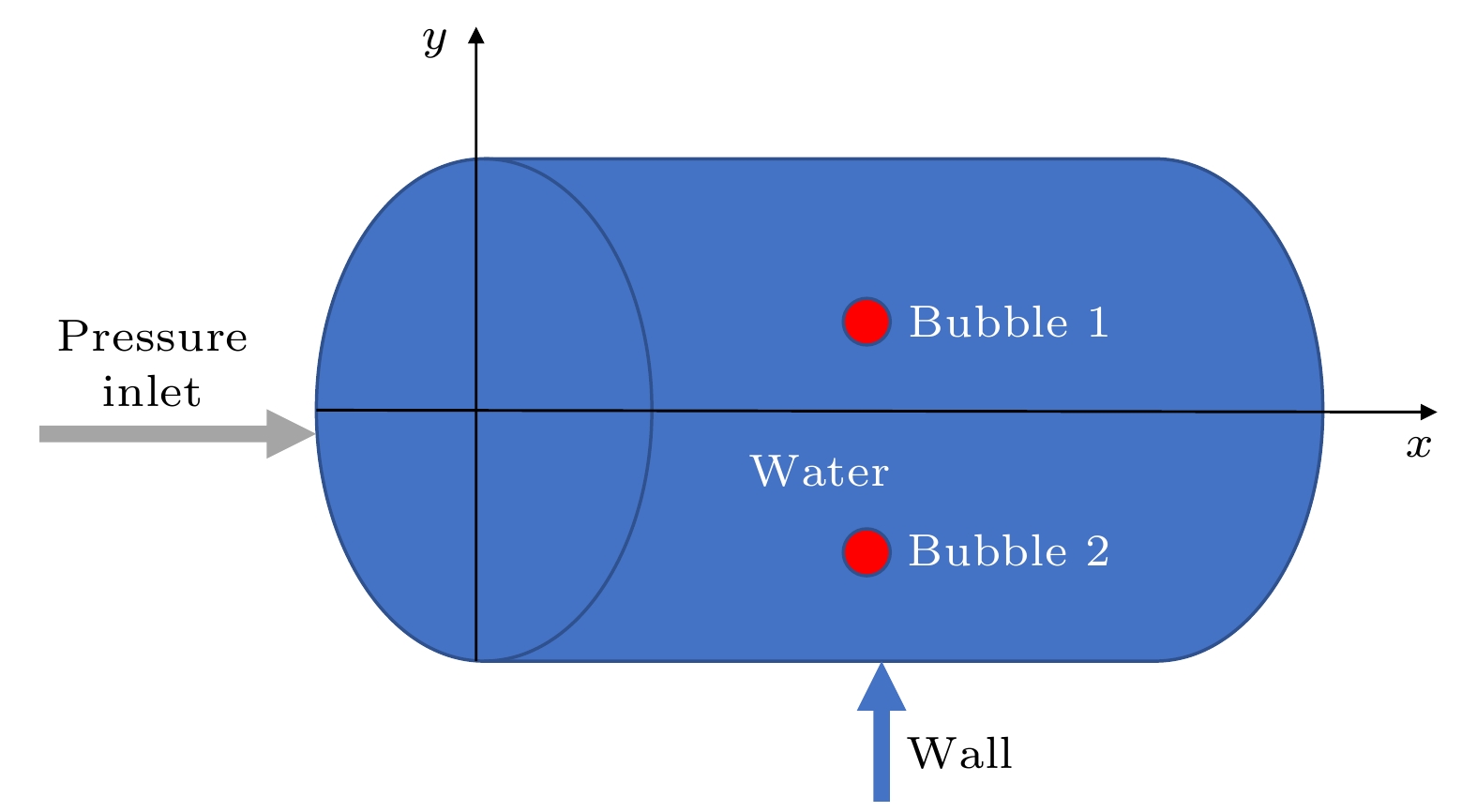
-
为了对双泡耦合的声空化过程进行模拟, 本文从流体动力学控制方程和流体体积分数模型出发, 在Fluent软件中构建双泡耦合超声空化三维有限元仿真模型, 对超声波驱动下流体中双泡耦合声空化动力学过程进行数值模拟, 并通过对空化气泡周围声场的变化进行分析研究双泡耦合声空化的非线性动力学特性. 结果显示: 在超声波驱动下, 球形气泡先缓慢扩张, 扩张到最大半径后迅速收缩直至溃灭; 耦合双气泡间存在相互作用力, 使得空化气泡的扩张受到抑制、气泡收缩时间增长; 空化气泡在收缩阶段的能量转换能力增强, 相比单气泡声空化, 耦合双气泡溃灭时气泡内部的压强更大. 本文分析结果将为超声空化泡群的动力学过程模拟提供参考.In order to simulate the acoustic cavitation process of double-bubble coupling, based on the fluid dynamics governing equation and fluid volume fraction model, this paper proposes a three-dimensional finite element simulation model of double-bubble coupled ultrasonic cavitation in Fluent software, and numerically simulates the dynamic process of double-bubble coupled acoustic cavitation in fluid driven by ultrasonic wave. The nonlinear dynamic characteristics of coupled acoustic cavitation with double bubbles are studied by evaluating the variation of the acoustic field around the cavitation bubble. The results indicate that under the ultrasonic drive, the spherical bubbles gradually widen first to the maximal radius, then shrink swiftly, and finally collapse. There is an interaction force between the paired double bubbles, which inhibits the expansion of cavitation bubbles and increases the bubble contraction time. The ability of the cavitation bubble to converse the energy is strengthened in the contraction stage. Compared with the acoustic cavitation of the single bubble, the pressure inside the bubble is expanded when the coupled double bubble collapses. The analysis results in this paper will provide the reference for the dynamic process simulation of the ultrasonic cavitation bubbles.
-
Keywords:
- ultrasonic cavitation /
- finite element simulation /
- two bubbles /
- coupled oscillation
[1] 徐柯, 许龙 2021 应用声学 40 343
Google Scholar
Xu K, Xu L 2021 Appl. Acoust. 40 343
Google Scholar
[2] 莫润阳, 林书玉, 王成会 2009 应用声学 8 389
Google Scholar
Mo R Y, Lin S Y, Wang C H 2009 Appl. Acoust. 8 389
Google Scholar
[3] 王成会, 林书玉 2011 声学学报 36 325
Wang C H, Lin S Y 2011 Acta Acust. 36 325
[4] 胡静, 林书玉, 王成会, 李锦 2013 62 134303
Google Scholar
Hu J, Lin S Y, Wang C H, Li J 2013 Acta Phys. Sin. 62 134303
Google Scholar
[5] 王成会, 莫润阳, 胡静, 陈时 2015 64 234301
Google Scholar
Wang C H, Mo R Y, Hu J, Chen S 2015 Acta Phys. Sin. 64 234301
Google Scholar
[6] 王成会, 莫润阳, 胡静, 张明铎 2017 中国科学: 物理学 力学 天文学 47 59
Wang C H, Mo R Y, Hu J, Zhang M D 2017 Sci. China, Ser. A Math. Phys. Astron. Technol. Sci. 47 59
[7] 莫润阳, 王成会, 胡静, 陈时 2020 陕西师范大学学报 (自然科学版) 48 39
Mo R Y, Wang C H, Hu J, Chen S 2020 J. Shaanxi Norm. Univ., (Nat. Sci. Ed.) 48 39
[8] 罗经, 李健, 董光能 2007 摩擦学学报 6 562
Google Scholar
Luo J, Li J, Dong G N 2007 Tribology 6 562
Google Scholar
[9] 吴晓霞, 张华余, 马空军 2012 应用声学 31 416
Google Scholar
Wu X X, Zhang H Y, Ma K J 2012 Acta Acust. 31 416
Google Scholar
[10] An Y, Lu T, Yang B 2005 Phys. Rev. E 71 026310
Google Scholar
[11] Liang J F, Chen W Z, Shao W H, Qi S B 2012 Chin. Phys. Lett. 29 074701
Google Scholar
[12] Liang J F, Wang X, Yang J, Gong L X 2017 Ultrasonics 75 58
Google Scholar
[13] Liang J F, Wu X Y, Qiao Y P 2021 Ultrason. Sonochem. 80 105837
Google Scholar
[14] 马艳, 林书玉, 徐洁 2018 67 034301
Google Scholar
Ma Y, Lin S Y, Xu J 2018 Acta Phys. Sin. 67 034301
Google Scholar
[15] Wu W H, Eskin D G, Priyadarshi A, Subroto T, Tzanakis I, Zhai W 2021 Ultrason. Sonochem. 73 105501
Google Scholar
[16] 陈伟中, 姜李安 2011 声学学报 36 197
Chen W Z, Jiang L A 2011 Acta Acust. 36 197
[17] 王福军 2004 计算流体动力学分析 (北京: 清华大学出版社) 第15页
Wang F J 2004 Computational Fluid Dynamics Analysis (Beijing: Tsinghua University Press) p15 (in Chinese)
[18] 李疆, 陈皓生 2008 摩擦学学报 28 311
Google Scholar
Li J, Chen H S 2008 Tribology 28 311
Google Scholar
[19] 刘兰, 张凌新 2015 机电工程 32 447
Liu L, Zhang L X 2015 J. Mech. Electr. Eng. 32 447
[20] 黄诗彬, 郭钟宁, 黄志刚, 印四华 2016 机床与液压 44 14
Google Scholar
Huang S B, Guo Z N, Huang Z G, YIN S H 2016 Machine Tool Hydraulics 44 14
Google Scholar
[21] 杨日福, 洪旭烨 2018 应用声学 37 455
Google Scholar
Yang R F, Hong X Y 2018 Appl. Acoust. 37 455
Google Scholar
[22] 王舰航, 陈韬厚, 包福兵, 王月兵 2020 应用声学 39 329
Google Scholar
Wang J H, Chen T H, Bao F B, Wang Y B 2020 Appl. Acoust. 39 329
Google Scholar
[23] 江帆, 黄鹏 2008 FLUENT高级应用与实例分析(北京: 清华大学出版社) 第140页
Jiang F, Huang P 2008 Fluent Advanced Application and Case Analysis (Beijing: Tsinghua University Press) p140 (in Chinese)
[24] Prosperetti A 1977 Q. Appl. Math 34 339
Google Scholar
[25] 谢崇国, 安宇 2003 52 102
Google Scholar
Xie C G, An Y 2003 Acta Phys. Sin. 52 102
Google Scholar
[26] Voronin D 2005 J. Appl. Mech. Tech. Phys. 46 677
Google Scholar
[27] Wang W J, Chen W Z, Wei R J 2001 Youth Academic Conference of Chinese Acoustic Society Shanghai, China, June 30th, 2001 p60
[28] 马艳, 申学鹏, 徐洁 2019 云南大学学报 (自然科学版) 41 718
Ma Y, Shen X P, Xu J 2019 J. Yunnan Univ., (Nat. Sci. Ed) 41 718
-
图 2 不同时刻气泡的形态变化情况 (a) 0 µs; (b) 4 µs; (c) 8 µs; (d) 10 µs; (e) 12 µs; (f) 14 µs; (g) 16 µs; (h) 18.80 µs; (i) 20 µs; (j) 22 µs; (k) 22.2 µs; (l) 22.4 µs
Fig. 2. The shape change of the bubble at different time: (a) 0 µs; (b) 4 µs; (c) 8 µs; (d) 10 µs; (e) 12 µs; (f) 14 µs; (g) 16 µs; (h) 18.80 µs; (i) 20 µs; (j) 22 µs; (k) 22.2 µs; (l) 22.4 µs.
图 4 监测点压强变化 (a) 声波激励方向; (b) 垂直声波激励方向
Fig. 4. Pressure change of monitoring points: (a) Acoustic excitation direction; (b) vertical acoustic excitation direction.
图 5 垂直声波激励方向监测点压强变化
Fig. 5. Pressure changes at monitoring points in the direction of vertical acoustic excitation.
图 6 塌缩阶段监测点压强变化 (a) 单气泡; (b) 耦合气泡
Fig. 6. Monitor pressure changes at the point during collapse: (a) Single bubble; (b) coupled bubble.
表 1 Fluent参数设置
Table 1. Parameter setting of Fluent.
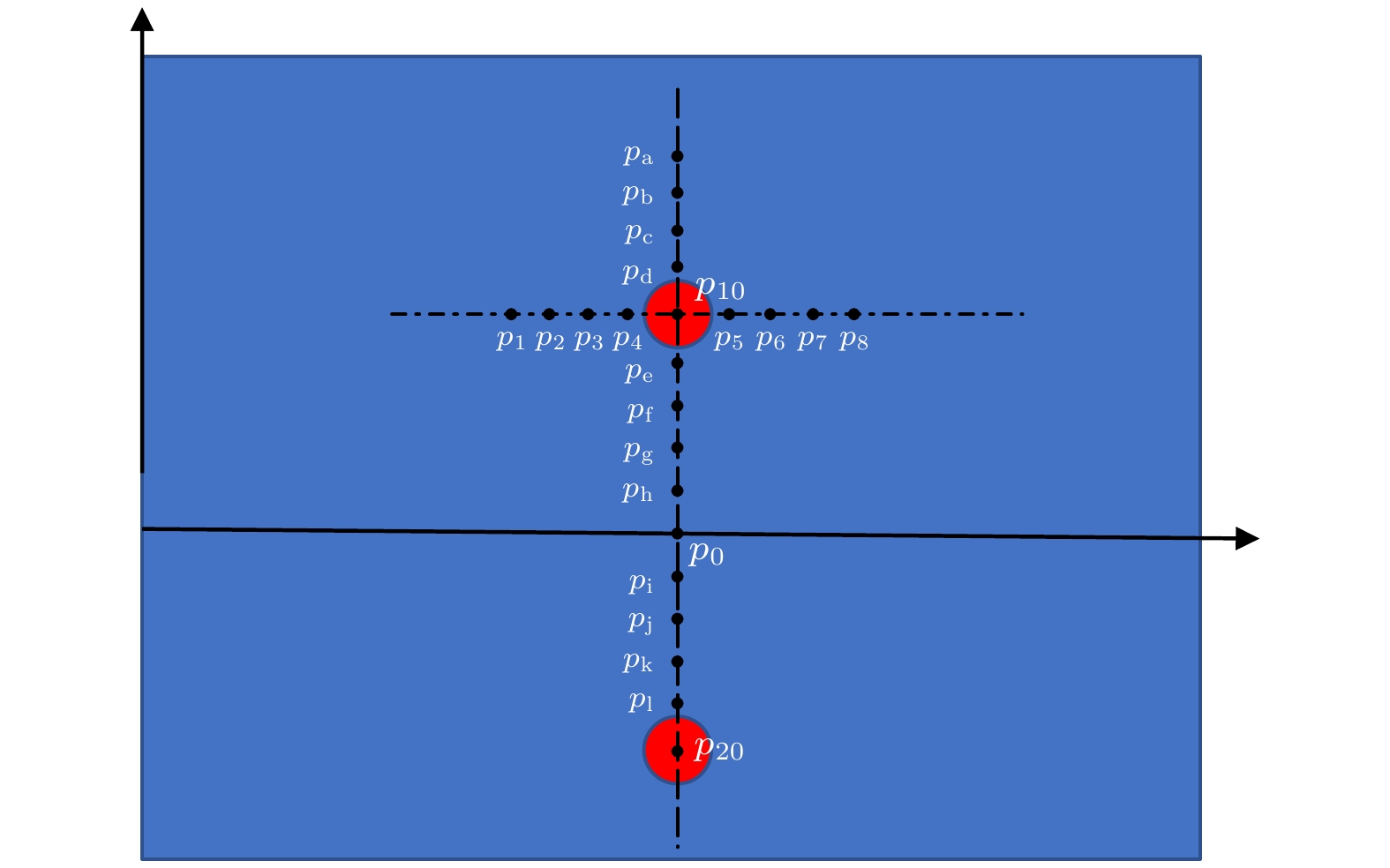
Setup Tasks Solver Pressure-based Absolute Transient Methods PISO First order implicit Second order upwind Model Multiphase-on Viscous-realizable k-epsilon Standard wall function Materials Fluid water-liquid Fluid water-vapor Density 1000 $ \rm{k}\rm{g}/{\rm{m}}^{3} $ 0.02558$ \rm{k}\rm{g}/{\rm{m}}^{3} $ Viscosity $0.001~{\rm kg/(m \cdot s)}$ $1.26\times 1{0}^{-6}~\rm{k}\rm{g}/(\rm{m}{\cdot} \rm{s})$ 
下载: 导出CSV
表 2 空化泡两侧位置压强变化起始时刻差
Table 2. Initial time difference between pressure changes on both sides of cavitation bubble.
距气泡中心距离/µm 压强变化起始时间差/µs 单泡 双泡耦合 10 0.02 0.13 20 0.04 0.41 30 0.04 0.53 40 0.06 1.02
下载: 导出CSV
表 3 不同空化气泡最大半径和气泡内部最大压强(
$ {P}_{\rm{a}} $ = 1.29 atm, f = 25 kHz,${R}_{10}=3.09~\text{μm},~ {R}_{20}=3.09~\text{μm}$ )Table 3. The maximum of radius and inside pressure of two kinds of bubbles (
$ {P}_{\rm{a}} $ = 1.29 atm, f = 25 kHz,${R}_{10}=3.09~ \text{μm},\; {R}_{20}=3.09 ~\text{μm}$ ).气泡类型 $ {R}_{\rm{m}\rm{a}\rm{x}} $/出现时刻
t/(μm·μs–1)$ {p}_{\rm{m}\rm{a}\rm{x}} $/出现时刻
t/(MPa·μs–1)双泡耦合 26.64/18.80 81.81/22.55 单泡 30.75/19.20 77.21/22.01
下载: 导出CSV
-
[1] 徐柯, 许龙 2021 应用声学 40 343
Google Scholar
Xu K, Xu L 2021 Appl. Acoust. 40 343
Google Scholar
[2] 莫润阳, 林书玉, 王成会 2009 应用声学 8 389
Google Scholar
Mo R Y, Lin S Y, Wang C H 2009 Appl. Acoust. 8 389
Google Scholar
[3] 王成会, 林书玉 2011 声学学报 36 325
Wang C H, Lin S Y 2011 Acta Acust. 36 325
[4] 胡静, 林书玉, 王成会, 李锦 2013 62 134303
Google Scholar
Hu J, Lin S Y, Wang C H, Li J 2013 Acta Phys. Sin. 62 134303
Google Scholar
[5] 王成会, 莫润阳, 胡静, 陈时 2015 64 234301
Google Scholar
Wang C H, Mo R Y, Hu J, Chen S 2015 Acta Phys. Sin. 64 234301
Google Scholar
[6] 王成会, 莫润阳, 胡静, 张明铎 2017 中国科学: 物理学 力学 天文学 47 59
Wang C H, Mo R Y, Hu J, Zhang M D 2017 Sci. China, Ser. A Math. Phys. Astron. Technol. Sci. 47 59
[7] 莫润阳, 王成会, 胡静, 陈时 2020 陕西师范大学学报 (自然科学版) 48 39
Mo R Y, Wang C H, Hu J, Chen S 2020 J. Shaanxi Norm. Univ., (Nat. Sci. Ed.) 48 39
[8] 罗经, 李健, 董光能 2007 摩擦学学报 6 562
Google Scholar
Luo J, Li J, Dong G N 2007 Tribology 6 562
Google Scholar
[9] 吴晓霞, 张华余, 马空军 2012 应用声学 31 416
Google Scholar
Wu X X, Zhang H Y, Ma K J 2012 Acta Acust. 31 416
Google Scholar
[10] An Y, Lu T, Yang B 2005 Phys. Rev. E 71 026310
Google Scholar
[11] Liang J F, Chen W Z, Shao W H, Qi S B 2012 Chin. Phys. Lett. 29 074701
Google Scholar
[12] Liang J F, Wang X, Yang J, Gong L X 2017 Ultrasonics 75 58
Google Scholar
[13] Liang J F, Wu X Y, Qiao Y P 2021 Ultrason. Sonochem. 80 105837
Google Scholar
[14] 马艳, 林书玉, 徐洁 2018 67 034301
Google Scholar
Ma Y, Lin S Y, Xu J 2018 Acta Phys. Sin. 67 034301
Google Scholar
[15] Wu W H, Eskin D G, Priyadarshi A, Subroto T, Tzanakis I, Zhai W 2021 Ultrason. Sonochem. 73 105501
Google Scholar
[16] 陈伟中, 姜李安 2011 声学学报 36 197
Chen W Z, Jiang L A 2011 Acta Acust. 36 197
[17] 王福军 2004 计算流体动力学分析 (北京: 清华大学出版社) 第15页
Wang F J 2004 Computational Fluid Dynamics Analysis (Beijing: Tsinghua University Press) p15 (in Chinese)
[18] 李疆, 陈皓生 2008 摩擦学学报 28 311
Google Scholar
Li J, Chen H S 2008 Tribology 28 311
Google Scholar
[19] 刘兰, 张凌新 2015 机电工程 32 447
Liu L, Zhang L X 2015 J. Mech. Electr. Eng. 32 447
[20] 黄诗彬, 郭钟宁, 黄志刚, 印四华 2016 机床与液压 44 14
Google Scholar
Huang S B, Guo Z N, Huang Z G, YIN S H 2016 Machine Tool Hydraulics 44 14
Google Scholar
[21] 杨日福, 洪旭烨 2018 应用声学 37 455
Google Scholar
Yang R F, Hong X Y 2018 Appl. Acoust. 37 455
Google Scholar
[22] 王舰航, 陈韬厚, 包福兵, 王月兵 2020 应用声学 39 329
Google Scholar
Wang J H, Chen T H, Bao F B, Wang Y B 2020 Appl. Acoust. 39 329
Google Scholar
[23] 江帆, 黄鹏 2008 FLUENT高级应用与实例分析(北京: 清华大学出版社) 第140页
Jiang F, Huang P 2008 Fluent Advanced Application and Case Analysis (Beijing: Tsinghua University Press) p140 (in Chinese)
[24] Prosperetti A 1977 Q. Appl. Math 34 339
Google Scholar
[25] 谢崇国, 安宇 2003 52 102
Google Scholar
Xie C G, An Y 2003 Acta Phys. Sin. 52 102
Google Scholar
[26] Voronin D 2005 J. Appl. Mech. Tech. Phys. 46 677
Google Scholar
[27] Wang W J, Chen W Z, Wei R J 2001 Youth Academic Conference of Chinese Acoustic Society Shanghai, China, June 30th, 2001 p60
[28] 马艳, 申学鹏, 徐洁 2019 云南大学学报 (自然科学版) 41 718
Ma Y, Shen X P, Xu J 2019 J. Yunnan Univ., (Nat. Sci. Ed) 41 718
下载:
计量
- 文章访问数: 4672
- PDF下载量: 106
- 被引次数: 0
