-
为分析超声空化的薄层液体中稳定的环状气泡链结构, 本文考虑气泡间次级声辐射影响, 得到了表征气泡间相互作用的气泡基本动力学方程以及次Bjerknes力的表达式, 数值分析了气泡平衡半径、声波频率和声压对纯液体区可能出现的单气泡所受的次Bjerknes力, 发现环形泡链能够吸引液体区内的新生的半径小于2 μm的气泡, 这可能是一定条件下环形气泡链能够稳定存在的原因. 随着驱动声波压力增加, 气泡数密度增加, 气泡间的耦合作用增强, 液体区内的环形泡链结构可能被液体区内出现的大气泡或者气泡团破坏, 进而导致环形结构演变成柱状、雾状乃至整个液体区均充满空化泡的情况发生. 通过高速摄影机观察了强声场作用下换能器辐射面外侧液体薄层内空化初生至形成空化云团簇的整个过程, 在空化云团簇中发现了局部同步崩溃并形成类纯液体薄层的现象, 该液体薄层边界随时间振荡持续约4个声周期后被空化云团簇吞没, 局部类纯液体区出现的位置具有随机性. 实验观察结果和理论预测具有很好的一致性.
-
关键词:
- 超声空化结构 /
- 气泡群 /
- 次Bjerknes力 /
- 稳定性
In this paper, the evolution of the cavitation bubbles is investigated. A model is developed to describe the cyclic chain-like cavitation cloud and analyze its structure stability in a thin liquid layer. By considering the effect of secondary acoustic radiation of bubbles, the dynamic equations of the bubbles in three zones of the cyclic chain are obtained. The secondary Bjerknes force is selected and used to explore the interaction between the bubbles in different regions. Numerical results show that the newborn bubbles inside the pure liquid zone of the thin layer can be attracted by the bubbles at the cyclic chain-like bubble chain. The bubble number density can affect the coupling strength between bubbles, and it is closely related to the driving pressure. Therefore, the structure stability of cyclic chain-like cavitation cloud can be disrupted by the perturbations of the acoustic pressure. To verify our analysis, we observe the structure of cavitation cloud in a thin liquid layer in a strong acoustic field by using a high speed camera. It is observed that the simultaneous collapse of local bubbles occurs, and pure liquid-like thin layers are distributed in the bubble cloud randomly. The boundary of the pure liquid-like thin layers oscillates with the acoustic field, and these liquid zones sustain about 4 acoustic cycles. The experimental results accord well with theoretical results.-
Keywords:
- ultrasonic cavitation structure /
- bubble group /
- secondary Bjerknes force /
- stability
[1] 应崇福 2007 中国科学: 物理学 力学 天文学 37 129
Ying C F 2007 Sci. Sin. Phys. Mech. Astron. 37 129
[2] 程效锐, 张舒研, 房宁 2018 应用化工 47 1753
Google Scholar
Cheng X R, Zhang S Y, Fang N 2018 Appl. Chem. Ind. 47 1753
Google Scholar
[3] 朱新建, 朱雪茹, 吴若愚, 吴宝明 2017 北京生物医学工程 36 106
Google Scholar
Zhu X J, Zhu X R, Wu R Y, Wu B M 2017 Beijing Biomed. Eng. 36 106
Google Scholar
[4] 陈伟中 2014 声空化物理 (北京: 科学出版社) 第236页
Chen W Z 2014 Acoustic Cavitation Physics (Beijing: Science Press) p236 (in Chinese)
[5] Bai L X, Xu W L, Zhang Y C, Li Y F, Huang D F 2008 IEEE Ultrasonics Symposium Beijing, China, November 2–5, 2008 p942
[6] Parlitz U, Mettin R, Luther S, Akhatov I, Voss M, Lauterborn W 1999 Philos. Trans. R. Soc. A 357 313
Google Scholar
[7] An Y 2011 Phys. Rev. E 83 066313
Google Scholar
[8] Zhang W J, An Y 2013 Phys. Rev. E 87 053023
Google Scholar
[9] 王成会, 莫润阳, 胡静 2016 65 149
Wang C H, Mo R Y, Hu J 2016 Acta Phys. Sin. 65 149
[10] 王成会 2007 陕西师范大学学报 (自然科学版) 35 45
Wang C H 2007 J. Shaanxi Norm. Univ. (Nat. Sci. Ed. ) 35 45
[11] Mettin R, Akhatov I, Parlitz U, Ohl C D, Lauterborn W 1997 Phys. Rev. E 56 2924
Google Scholar
[12] Wu P F, Bai L X, Lin W J, Yan J C 2017 Ultrason. Sonochem. 38 75
Google Scholar
[13] Moussatov A, Granger C, Dubus B 2005 Ultrason. Sonochem. 12 415
Google Scholar
[14] Gallego-juarez J A, Riera E, Acosta V, Rodriguez G, Blanco A 2010 Ultrason. Sonochem. 17 234
Google Scholar
[15] Soyama H, Saito K, Saka M 2002 J. Eng. Mater. Technol. 124 135
Google Scholar
[16] Soyama H, Park J D, Saka M 2000 J. Manuf. Sci. Eng. 122 83
Google Scholar
[17] Gao Y B, Wu B X, Liu Z, Zhou Y, Shen N G, Ding H T 2014 J. Manuf. Sci. Eng. 136 014502
Google Scholar
[18] Bai L X, Chen X G, Zhu G, Xu W L, Lin W J, Wu P F, Li C, Xu D L, Yan J C 2017 Ultrason. Sonochem. 35 405
Google Scholar
[19] Keller J B, Kolodner I I 1956 J. Appl. Phys. 27 1152
Google Scholar
[20] Keller J B, Miksis M 1980 J. Acoust. Soc. Am. 68 628
Google Scholar
[21] Yasui K, Iida Y, Tuziuti T, Kozuka T, Towata A 2008 Phys. Rev. E 77 016609
Google Scholar
[22] Wang C H, Cheng J C 2013 Chin. Phys. B 22 014304
Google Scholar
[23] Weninger K R, Camara C G, Putterman S J 2000 Phys. Rev. E 63 016310
Google Scholar
[24] 白立新, 吴鹏飞, 李超, 邓京军, 曾志杰 2018 应用声学 37 614
Google Scholar
Bai L X, Wu P F, Li C, Deng J J, Zeng Z J 2018 Appl. Acoust. 37 614
Google Scholar
[25] Vanhille C, Campos-Pozuelo C 2012 Ultrason. Sonochem. 19 217
Google Scholar
-
图 2 声波频率
$ f{\text{ = 2}}0{\text{ kHz}} $ , 液体区域内单气泡所受的次Bjerknes力随气泡半径的变化 (a) Pa = 100 kPa; (b) Pa = 120 kPa; (c) Pa = 140 kPaFig. 2. Secondary Bjerknes force of a single bubble in the liquid area varies with the bubble radius,
$ f{\text{ = 2}}0{\text{ kHz}} $ : (a) Pa = 100 kPa; (b) Pa = 120 kPa; (c) Pa = 140 kPa.
图 3 驱动声压
$ {P_{\text{a}}} = 100{\text{ kPa}} $ , 驱动声波频率对单气泡所受的次Bjerknes力的影响 (a)$ f{\text{ = 4}}0{\text{ kHz}} $ ; (b)$ f = 80{\text{ kHz}} $ Fig. 3. Driving amplitude
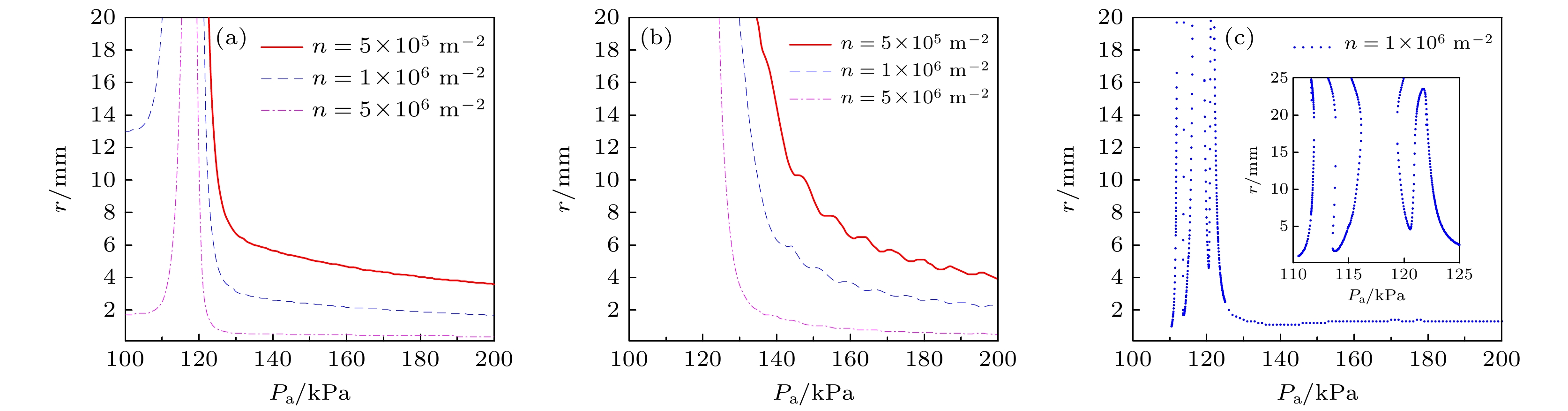
$ {P_{\text{a}}} = 100{\text{ kPa}}, $ influence of driving acoustic frequency on the secondary Bjerknes force applied to a single bubble: (a)$ f{\text{ = 4}}0{\text{ kHz}} $ ; (b)$ f = 80{\text{ kHz}} $ .
图 4 不同气泡半径时, 气泡数密度对气泡链半径随声压演变规律的影响, 声波频率
$ f{\text{ = }}20{\text{kHz}} $ (a)${R_{10}} = {R_{20}} = 3\;{{ \mu {\rm{m}}}}$ , (b)${R_{10}} = 5\;{{ \mu {\rm{m}}}},\; {R_{20}} = 3\;{{ \mu {\rm{m}}}}$ , (c)${R_{10}} = 3\;{{ \mu {\rm{m}}, }}\;{R_{20}} = 5\;{{ \mu {\rm{m}}}}$ Fig. 4. Effect of bubble number density on the evolution of bubble chain radius with acoustic pressure for different bubble radius,
$f{\text{ = }}20\;{\text{kHz}}$ : (a)${R_{10}} = {R_{20}} = 3\;{{ \mu {\rm{m}}}}$ , (b)${R_{10}} = 5\;{{ \mu {\rm{m}}}},\; {R_{20}} = 3\;{{ \mu {\rm{m}}}}$ , (c)${R_{10}} = 3\;{{ \mu {\rm{m}}, }}\;{R_{20}} = 5\;{{ \mu {\rm{m}}}}$ .
图 7 薄层液体中的空化结构随时间的变化
Fig. 7. Variation of cavitation structure in thin layer liquid with time.
-
[1] 应崇福 2007 中国科学: 物理学 力学 天文学 37 129
Ying C F 2007 Sci. Sin. Phys. Mech. Astron. 37 129
[2] 程效锐, 张舒研, 房宁 2018 应用化工 47 1753
Google Scholar
Cheng X R, Zhang S Y, Fang N 2018 Appl. Chem. Ind. 47 1753
Google Scholar
[3] 朱新建, 朱雪茹, 吴若愚, 吴宝明 2017 北京生物医学工程 36 106
Google Scholar
Zhu X J, Zhu X R, Wu R Y, Wu B M 2017 Beijing Biomed. Eng. 36 106
Google Scholar
[4] 陈伟中 2014 声空化物理 (北京: 科学出版社) 第236页
Chen W Z 2014 Acoustic Cavitation Physics (Beijing: Science Press) p236 (in Chinese)
[5] Bai L X, Xu W L, Zhang Y C, Li Y F, Huang D F 2008 IEEE Ultrasonics Symposium Beijing, China, November 2–5, 2008 p942
[6] Parlitz U, Mettin R, Luther S, Akhatov I, Voss M, Lauterborn W 1999 Philos. Trans. R. Soc. A 357 313
Google Scholar
[7] An Y 2011 Phys. Rev. E 83 066313
Google Scholar
[8] Zhang W J, An Y 2013 Phys. Rev. E 87 053023
Google Scholar
[9] 王成会, 莫润阳, 胡静 2016 65 149
Wang C H, Mo R Y, Hu J 2016 Acta Phys. Sin. 65 149
[10] 王成会 2007 陕西师范大学学报 (自然科学版) 35 45
Wang C H 2007 J. Shaanxi Norm. Univ. (Nat. Sci. Ed. ) 35 45
[11] Mettin R, Akhatov I, Parlitz U, Ohl C D, Lauterborn W 1997 Phys. Rev. E 56 2924
Google Scholar
[12] Wu P F, Bai L X, Lin W J, Yan J C 2017 Ultrason. Sonochem. 38 75
Google Scholar
[13] Moussatov A, Granger C, Dubus B 2005 Ultrason. Sonochem. 12 415
Google Scholar
[14] Gallego-juarez J A, Riera E, Acosta V, Rodriguez G, Blanco A 2010 Ultrason. Sonochem. 17 234
Google Scholar
[15] Soyama H, Saito K, Saka M 2002 J. Eng. Mater. Technol. 124 135
Google Scholar
[16] Soyama H, Park J D, Saka M 2000 J. Manuf. Sci. Eng. 122 83
Google Scholar
[17] Gao Y B, Wu B X, Liu Z, Zhou Y, Shen N G, Ding H T 2014 J. Manuf. Sci. Eng. 136 014502
Google Scholar
[18] Bai L X, Chen X G, Zhu G, Xu W L, Lin W J, Wu P F, Li C, Xu D L, Yan J C 2017 Ultrason. Sonochem. 35 405
Google Scholar
[19] Keller J B, Kolodner I I 1956 J. Appl. Phys. 27 1152
Google Scholar
[20] Keller J B, Miksis M 1980 J. Acoust. Soc. Am. 68 628
Google Scholar
[21] Yasui K, Iida Y, Tuziuti T, Kozuka T, Towata A 2008 Phys. Rev. E 77 016609
Google Scholar
[22] Wang C H, Cheng J C 2013 Chin. Phys. B 22 014304
Google Scholar
[23] Weninger K R, Camara C G, Putterman S J 2000 Phys. Rev. E 63 016310
Google Scholar
[24] 白立新, 吴鹏飞, 李超, 邓京军, 曾志杰 2018 应用声学 37 614
Google Scholar
Bai L X, Wu P F, Li C, Deng J J, Zeng Z J 2018 Appl. Acoust. 37 614
Google Scholar
[25] Vanhille C, Campos-Pozuelo C 2012 Ultrason. Sonochem. 19 217
Google Scholar
下载:
计量
- 文章访问数: 3806
- PDF下载量: 72
- 被引次数: 0
