-
The propagation of electromagnetic wave in plasma is one of the long-standing concerns in the field of laser plasma, and it is closely related to the researches of radiation source generation, particle acceleration, and inertial confinement fusion. Recently, the proposal of various schemes for generating intense vortex beams has led to an increasing number of researchers focusing on the interaction between intense vortex beams and plasmas, resulting in significant research progress in various areas, such as particle acceleration, high-order harmonic generation, quasi-static self-generated magnetic fields, and parametric instability. Compared with traditional Gaussian beams, vortex beams, featuring their hollow amplitudes and helical phases, can exhibit novel phenomena during propagating through plasma. In this work, we primarily focus on studying the influence of the propagation process on the wave structure of vortex beams before filamentation occurs. The three-dimensional particle-in-cell simulations show that weakly relativistic vortex beams exhibit wavefront distortion during their propagation in plasma. The distortion degree is closely related to the intensity of the electromagnetic wave and the propagation distance for a given plasma density. This phenomenon is theoretically explained by using a phase correction model that considers the relativistic mass correction of electrons. Additionally, we demonstrate that the wavefront distortion can be compensated for and suppressed by appropriately modulating the initial plasma density, as confirmed by three-dimensional particle simulations. The results of decomposing the wavefront into Laguerre-Gaussian (LG) mode components indicate that the wavefront distortion is primarily caused by high-order p LG modes, and it is independent of other l LG modes. Additionally, we extend the present investigation to the propagation of vortex beams in axially magnetized plasma, where the phase correction model can also effectively explain the occurrence of wavefront distortion. Our work can deepen the understanding of the interaction between plasma and strong vortex beams, and provide some valuable references for designing plasma devices serving as the manipulation of intense vortex beams in future research.
[1] Beth R A 1936 Phys. Rev. 50 115
Google Scholar
[2] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[3] He H, Friese M E J, Heckenberg N R, Rubinsztein-Dunlop H 1995 Phys. Rev. Lett. 75 826
Google Scholar
[4] Wang J, Yang J Y, Fazal I M, Ahmed N, Yan Y, Huang H, Ren Y, Yue Y, Dolinar S, Tur M, Willner A E 2012 Nat. Photonics 6 488
Google Scholar
[5] Bozinovic N, Yue Y, Ren Y, Tur M, Kristensen P, Huang H, Willner A E, Ramachandran S 2013 Science 340 1545
Google Scholar
[6] Tamburini F, Anzolin G, Umbriaco G, Bianchini A, Barbieri C 2006 Phys. Rev. Lett. 97 163903
Google Scholar
[7] Harwit M 2003 The Astrophysical Journal 597 1266
Google Scholar
[8] Tamburini F, Thidé B, Molina-Terriza G, Anzolin G 2011 Nat. Phys. 7 195
Google Scholar
[9] Tamburini F, Thidé B, Della Valle M 2019 Mon. Not. R. Astron. Soc. 492 L22
[10] Shi Y, Shen B, Zhang L, Zhang X, Wang W, Xu Z 2014 Phys. Rev. Lett. 112 235001
Google Scholar
[11] Vieira J, Trines R M G M, Alves E P, Fonseca R A, Mendonca J T, Bingham R, Norreys P, Silva L O 2016 Nat. Commun. 7 10371
Google Scholar
[12] Leblanc A, Denoeud A, Chopineau L, Mennerat G, Martin P, Quéré F 2017 Nat. Phys. 13 440
Google Scholar
[13] Qu K, Jia Q, Fisch N J 2017 Phys. Rev. E 96 053207
Google Scholar
[14] Shi Y, Blackman D, Stutman D, Arefiev A 2021 Phys. Rev. Lett. 126 234801
Google Scholar
[15] Vieira J, Mendonca J T 2014 Phys. Rev. Lett. 112 215001
Google Scholar
[16] Brabetz C, Busold S, Cowan T, Deppert O, Jahn D, Kester O, Roth M, Schumacher D, Bagnoud V 2015 Phys. Plasmas 22 013105
Google Scholar
[17] Zhang X, Shen B, Zhang L, Xu J, Wang X, Wang W, Yi L, Shi Y 2014 New J. Phys. 16 123051
Google Scholar
[18] Willim C, Vieira J, Malka V, Silva L O 2023 Phys. Rev. Res. 5 023083
Google Scholar
[19] Zhang X, Shen B, Shi Y, Wang X, Zhang L, Wang W, Xu J, Yi L, Xu Z 2015 Phys. Rev. Lett. 114 173901
Google Scholar
[20] Denoeud A, Chopineau L, Leblanc A, Quéré F 2017 Phys. Rev. Lett. 118 033902
Google Scholar
[21] Wang J W, Zepf M, Rykovanov S G 2019 Nat. Commun. 10 5554
Google Scholar
[22] Li S, Zhang X, Gong W, Bu Z, Shen B 2020 New J. Phys. 22 013054
Google Scholar
[23] Chen Y Y, Li J X, Hatsagortsyan K Z, Keitel C H 2018 Phys. Rev. Lett. 121 074801
Google Scholar
[24] Zhu X L, Chen M, Yu T P, Weng S M, Hu L X, McKenna P, Sheng Z M 2018 Appl. Phys. Lett. 112 174102
Google Scholar
[25] Ali S, Davies J R, Mendonca J T 2010 Phys. Rev. Lett. 105 035001
Google Scholar
[26] Shi Y, Vieira J, Trines R M G M, Bingham R, Shen B F, Kingham R J 2018 Phys. Rev. Lett. 121 145002
Google Scholar
[27] Longman A, Fedosejevs R 2021 Phys. Rev. Res. 3 043180
Google Scholar
[28] Liu W, Jia Q, Zheng J 2023 Matter Radiat. Extremes 8 014405
Google Scholar
[29] Wang W P, Jiang C, Shen B F, Yuan F, Gan Z M, Zhang H, Zhai S H, Xu Z Z 2019 Phys. Rev. Lett. 122 024801
Google Scholar
[30] Baumann C, Pukhov A 2018 Phys. Plasmas 25 083114
Google Scholar
[31] Mendonca J T, Thidé B, Then H 2009 Phys. Rev. Lett. 102 185005
Google Scholar
[32] Nuter R, Korneev P, Tikhonchuk V T 2022 Phys. Plasmas 29 062101
Google Scholar
[33] Ji Y, Lian C W, Shi Y, Yan R, Cao S, Ren C, Zheng J 2023 Phys. Rev. Res. 5 L022025
Google Scholar
[34] Ju L B, Huang T W, Xiao K D, Wu G Z, Yang S L, Li R, Yang Y C, Long T Y, Zhang H, Wu S Z, Qiao B, Ruan S C, Zhou C T 2016 Phys. Rev. E 94 033202
Google Scholar
[35] 范海玲, 郭志坚, 李明强, 卓红斌 2023 72 014206
Google Scholar
Fan H L, Guo Z J, Li M Q, Zhuo H B 2023 Acta Phys. Sin. 72 014206
Google Scholar
[36] Arber T D, Bennett K, Brady C S, Lawrence-Douglas A, Ramsay M G, Sircombe N J, Gillies P, Evans R G, Schmitz H, Bell A R, Ridgers C P 2015 Plasma Phys. Control. Fusion 57 113001
Google Scholar
[37] Gibbon P 2005 Short Pulse Laser Interactions With Matter: an Introduction (London: Imperial College Press) pp31–36, 84, 92, 99, 106
[38] Esarey E, Ting A, Sprangle P, Umstadter D, Liu X 1993 IEEE Trans. Plasma Sci. 21 95
Google Scholar
[39] 盛政明 2014 强场激光物理研究前沿(上海: 上海交通大学出版社) 第21页
Sheng Z M 2014 Advances in High Field Laser Physics (Shanghai: Shanghai Jiao Tong University Press) pp21
[40] Liu C, Tripathi V, Eliasson B 2019 High-Power Laser-Plasma Interaction (Cambridge: Cambridge University Press) p89
-
图 1 三维粒子模拟中, 在不同光强(a)—(d) $ a = 0.01 $, (e)—(h) $ a = 0.1 $, (i)—(l) $ a = 0.2 $下, 模式为$ l = 1,\; {\sigma_x} = 1 $的圆偏振涡旋光在等离子中传播至不同距离(a)(e)(i) $ x = 5\; {\text{μm}} $, (b)(f)(j) $ x = 10\; {\text{μm}} $, (c)(g)(k) $ x = 15\; {\text{μm}} $, (d)(h)(l) $ x = 20\; {\text{μm}} $处, 对应的电场$ E_y $分布
Figure 1. Transverse distributions of electric fields $ E_y $ obtained by three-dimensional (3D) particle-in-cell (PIC) simulations for different laser intensities (a)–(d) $ a = 0.01 $, (e)–(h) $ a = 0.1 $, (i)–(l) $ a = 0.2 $, when a circularly polarized vortex light beam with $ l = 1,\; {\sigma_x} = 1 $ propagates in the plasma over different distances (a)(e)(i) $ x = 5\; {\text{μm}} $, (b)(f)(j) $ x = 10\; {\text{μm}} $, (c)(g)(k) $ x = 15\; {\text{μm}} $, (d)(h)(l) $ x = 20\; {\text{μm}} $
图 2 在粒子模拟中, 强度为$ a = 0.2 $的$ l = 1,\; {\sigma_x} = 1 $模式的圆偏振涡旋光在密度为$ {{n}_{{\mathrm{e}}}} = 0.56{{n}_{{\mathrm{c}}}} $的等离子体中传播时, 电场$ E_y $在沿传播方向上k空间的频谱分布
Figure 2. Distribution of electric field $ E_y $ in k-space along the propagation direction obtained by 3D PIC simulations when a $ l = 1,\; {\sigma_x} = 1 $ circularly polarized vortex light beam with $ a = 0.2 $ propagates in a plasma with a density of $ {n_{\mathrm{e}}} = 0.56 {n_{\mathrm{c}}} $
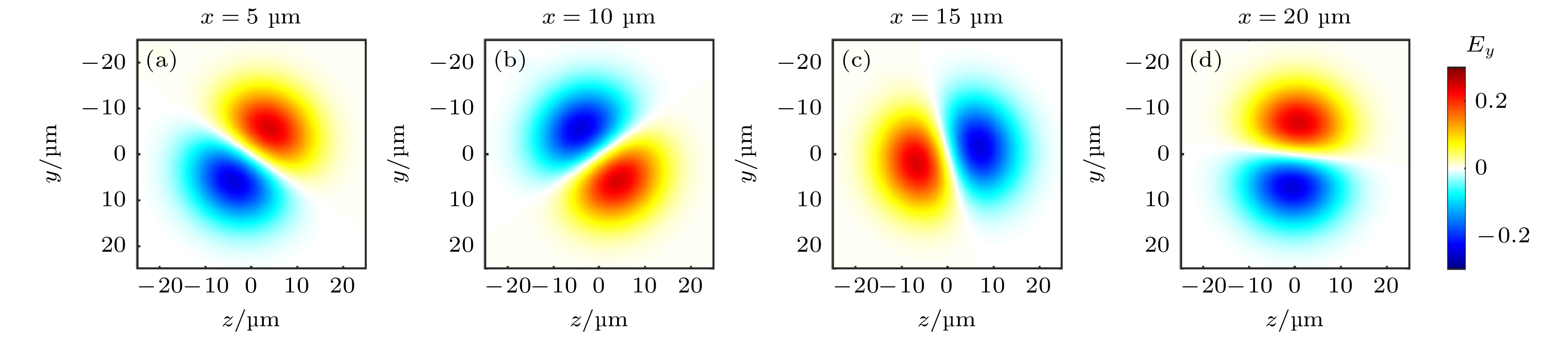
图 3 基于相位修正模型, 考虑相对论电子质量修正效应时, 强度为$ a = 0.2 $的$ l = 1,\; {\sigma_x} = 1 $模式的圆偏振涡旋光在等离子体中传播至不同距离处的电场$ E_y $分布 (a) $ x = 5\; {\text{μm}} $; (b) $ x = 10\; {\text{μm}} $; (c) $ x = 15\; {\text{μm}} $; (d) $ x = 20\; {\text{μm}} $
Figure 3. Transverse distributions of electric fields $ E_y $ obtained by the phase-correction model taking into account the relativistic electron mass effect when a $ l = 1,\; {\sigma_x} = 1 $ circularly polarized vortex light beam with $ a = 0.2 $ propagating in the plasma over different distances: (a) $ x = 5\; {\text{μm}} $; (b) $ x = 10\; {\text{μm}} $; (c) $ x = 15\; {\text{μm}} $; (d) $ x = 20\; {\text{μm}} $
图 4 基于相位修正模型, 考虑激光有质动力效应时, 强度为$ a = 0.2 $的$ l = 1,\; {\sigma_x} = 1 $模式的圆偏振涡旋光在等离子体中传播至不同距离处的电场$ E_y $分布 (a) $ x = 5\; {\text{μm}} $; (b) $ x = 10\; {\text{μm}} $; (c) $ x = 15\; {\text{μm}} $; (d) $ x = 20\; {\text{μm}} $
Figure 4. Transverse distributions of electric fields $ E_y $ obtained by the phase-correction model considering the laser ponderomotive force effect when a $ l = 1,\; {\sigma_x} = 1 $ circularly polarized vortex light beam with $ a = 0.2 $ propagating in the plasma over different distances: (a) $ x = 5\; {\text{μm}} $; (b) $ x = 10\; {\text{μm}} $; (c) $ x = 15\; {\text{μm}} $; (d) $ x = 20\; {\text{μm}} $
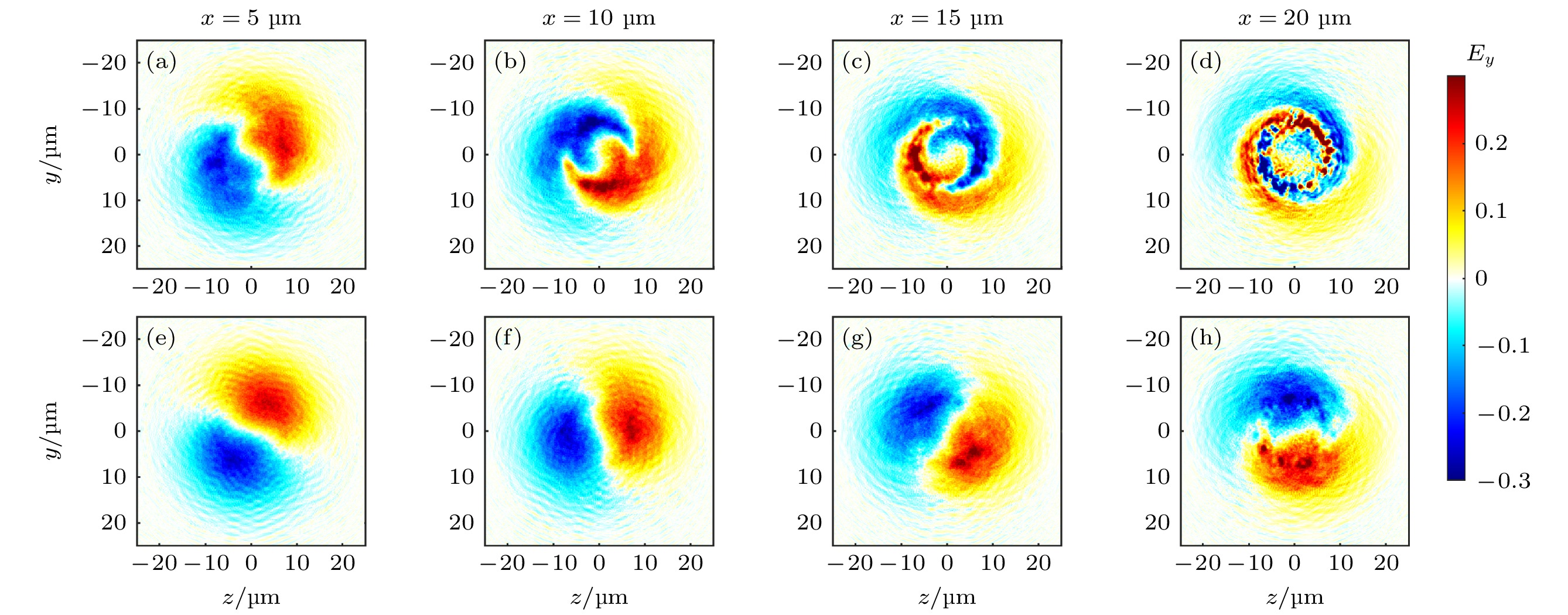
图 5 在三维粒子模拟中, 强度为$ a = 0.2 $的$ l = 1,\; {\sigma_x} = 1 $的圆偏振涡旋光在预设密度分布为$ n(r) = {n_{{\mathrm{ini}}}}\sqrt{1+{{{| \boldsymbol{E}|}^{2}}}/{2{{E}_{0}^{2}}}} $ ($ n_{{\mathrm{ini}}} = 0.56 {n_{\mathrm{c}}} $)的等离子体中传播至不同距离处, 电场$ E_y $分布 (a) $ x = 5\; {\text{μm}} $; (b) $ x = 10\; {\text{μm}} $; (c) $ x = 15\; {\text{μm}} $; (d) $ x = 20\; {\text{μm}} $
Figure 5. Transverse distributions of electric fields $ E_y $ obtained by 3D PIC simulation when a $ l = 1,\; {\sigma_x} = 1 $ circularly polarized vortex light beam with $ a = 0.2 $ propagating in a plasma with a predetermined density distribution of $ n(r) = {n_{{\mathrm{ini}}}}\sqrt{1+{{{| \boldsymbol{E}|}^{2}}}/{2{{E}_{0}^{2}}}} $ over different distances: (a) $ x = 5\; {\text{μm}} $; (b) $ x = 10\; {\text{μm}} $; (c) $ x = 15\; {\text{μm}} $; (d) $ x = 20\; {\text{μm}} $, where $ n_{{\mathrm{ini}}} = 0.56 {n_{\mathrm{c}}} $
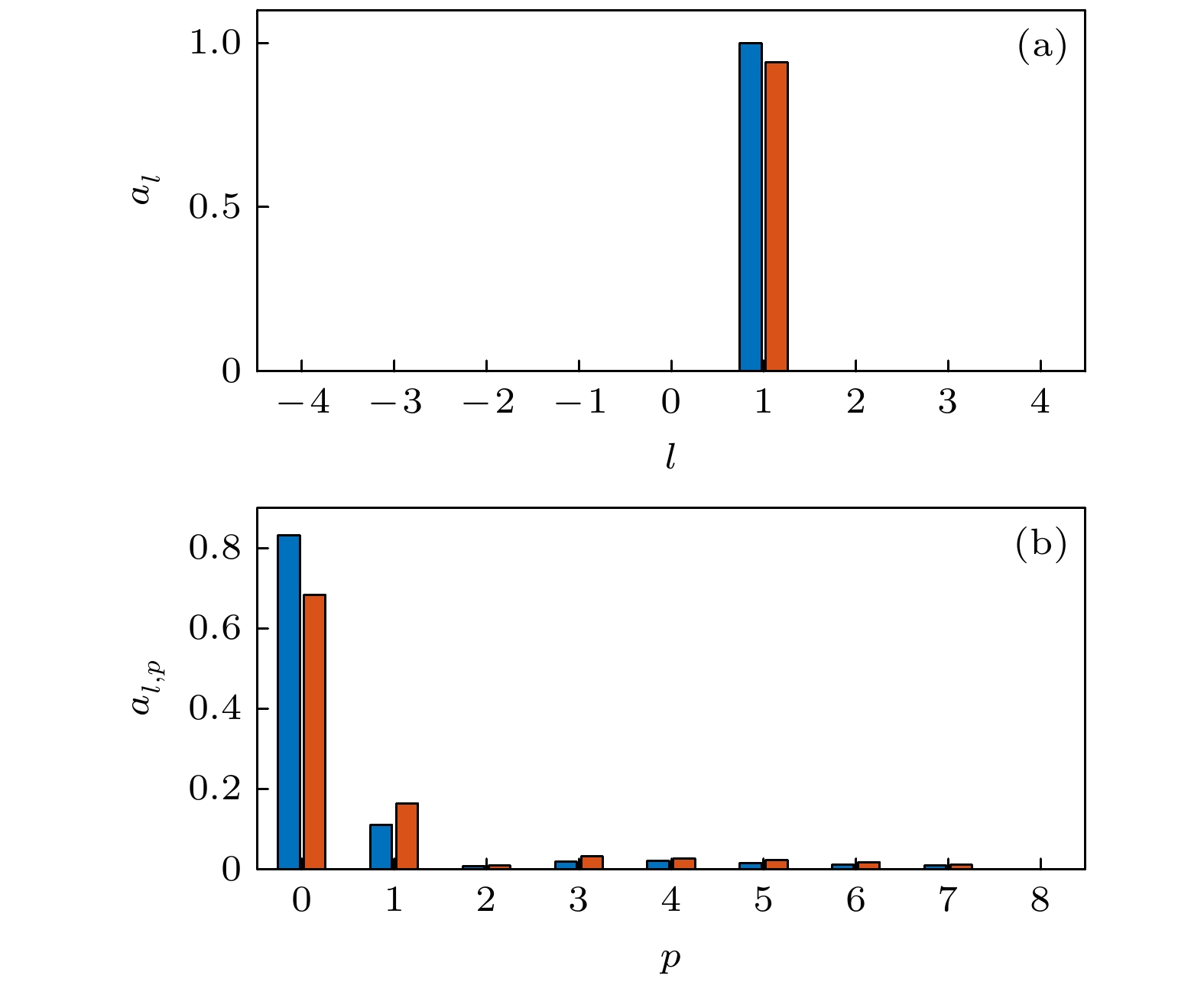
图 6 强度为$ a = 0.2 $的$ l = 1,\; {\sigma_x} = 1 $的圆偏振涡旋光在等离子体中传播至$ x = 20\; {\text{μm}} $处, 对波前复振幅进行LG模式分解的结果 (a)不同l模式$ ({a}_{l} = \displaystyle\sum\nolimits_{p}{a}_{l, p}) $的能量占比分布; (b) $ l = 1 $的不同p模式的能量占比分布. 图中橙色表示与图1(l)对应的粒子模拟结果, 蓝色表示与图3(d)对应的理论结果
Figure 6. The results of LG mode decomposition of wavefront for a $ l = 1,\; {\sigma_x} = 1 $ circularly polarized vortex light beam with $ a = 0.2 $ propagating in a plasma to a distance of $ x = 20\; {\text{μm}} $: (a) Dstribution of $ {a}_{l} = \displaystyle\sum\nolimits_{p}{a}_{l, p} $; (b) distribution of different p modes for $ l = 1 $. The orange and blue colors in the figure indicate the results corresponding to Fig. 1(l) and Fig. 3(d), respectively
图 7 在三维粒子模拟中, 强度为$ a/\sqrt{2} = 0.2 $的$ l = 1,\; p = 0 $的线偏振涡旋光在(a)—(d)均匀密度等离子体及(e)—(h)预设密度分布$ n(r) $等离子中传播至不同距离处的电场$ E_y $分布 (a)(e) $ x = 5\; {\text{μm}} $; (b)(f) $ x = 10\; {\text{μm}} $; (c)(g) $ x = 15\; {\text{μm}} $; (d)(h) $ x = 20\; {\text{μm}} $
Figure 7. Transverse distributions of electric fields $ E_y $ obtained by 3D PIC simulations when a $ l = 1,\; p = 0 $ linearly polarized vortex light beam with $ a/\sqrt{2} = 0.2 $ propagating in (a)–(d) uniform plasmas and (e)–(h) plasmas with a predetermined density distribution $ n(r) $ over different distances: (a)(e) $ x = 5\; {\text{μm}} $; (b)(f) $ x = 10\; {\text{μm}} $; (c)(g) $ x = 15\; {\text{μm}} $; (d)(h) $ x = 20\; {\text{μm}} $
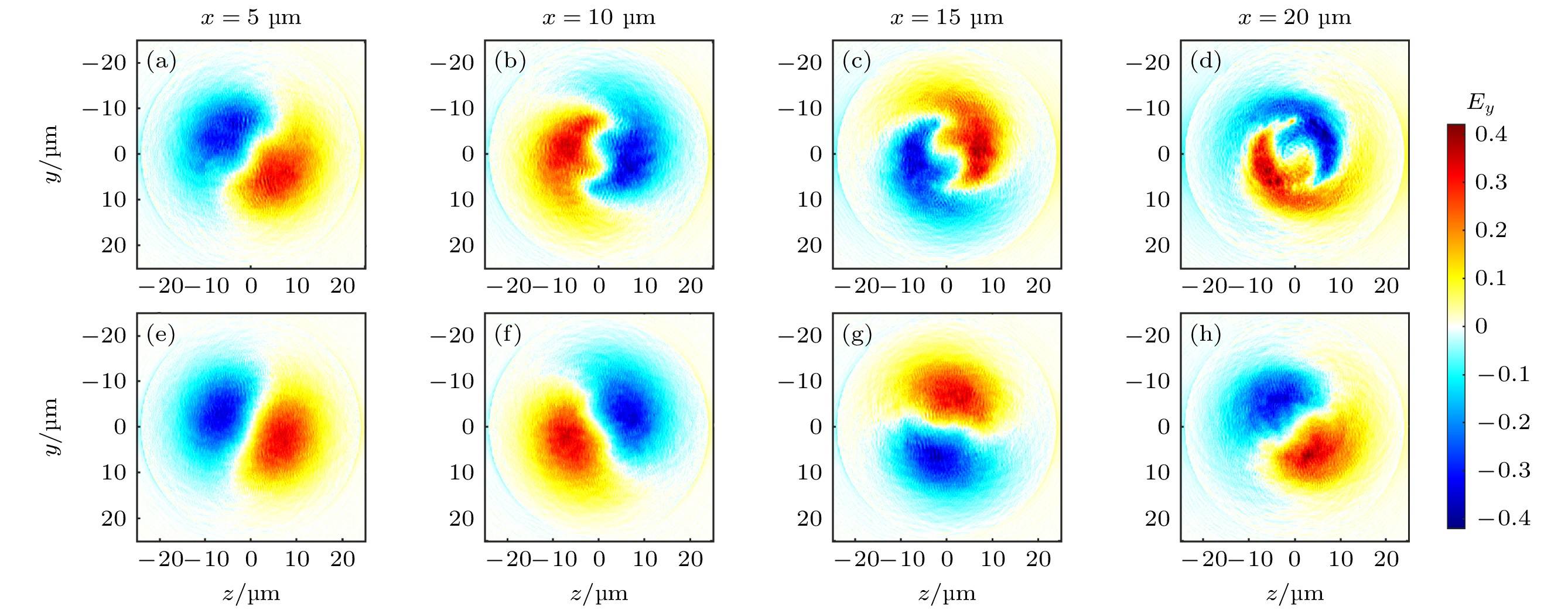
图 8 在三维粒子模拟中, 强度为$ a = 0.2 $的$ l = 1,\; {\sigma_x} = 1 $的圆偏振涡旋光在(a)—(d)均匀轴向磁化等离子体及(e)—(h)预设密度及轴向磁化的等离子中传播至不同距离处的电场$ E_y $分布 (a)(e) $ x = 5\; {\text{μm}} $; (b)(f) $ x = 10\; {\text{μm}} $; (c)(g) $ x = 15\; {\text{μm}} $; (d)(h) $ x = 20\; {\text{μm}} $
Figure 8. Transverse distributions of electric fields $ E_y $ obtained by 3D PIC simulations when a $ l = 1,\; {\sigma_x} = 1 $ circularly polarized vortex light beam with $ a = 0.2 $ propagating in (a)–(d) uniformly axially magnetized plasmas and (e)–(h) plasmas with a predetermined density and axial magnetization over different distances: (a)(e) $ x = 5\; {\text{μm}} $; (b)(f) $ x = 10\; {\text{μm}} $, (c)(g) $ x = 15\; {\text{μm}} $; (d)(h) $ x = 20\; {\text{μm}} $
图 A1 三维粒子模拟中, 在不同密度(a)—(d) $ n_{\mathrm{e}} = 0.36 n_{\mathrm{c}} $, (e)—(h) $ n_{\mathrm{e}} = 0.56 n_{\mathrm{c}} $, (i)—(l) $ n_{\mathrm{e}} = 0.64 n_{\mathrm{c}} $下, 强度为$ a = 0.2 $, 模式为$ l = 1, {\sigma_x} = 1 $的圆偏振涡旋光在等离子中传播至不同距离(a)(e)(i) $ x = 5\; {\text{μm}} $, (b)(f)(j) $ x = 10\; {\text{μm}} $, (c)(g)(k) $ x = 15\; {\text{μm}} $, (d)(h)(l) $ x = 20\; {\text{μm}} $处, 对应的电场$ E_y $分布
Figure A1. Transverse distributions of electric fields $ E_y $ obtained by 3D PIC simulations for different plasma densities (a)–(d) $ n_{\mathrm{e}} = 0.36 n_{\mathrm{c}} $, (e)–(h) $ n_{\mathrm{e}} = 0.56 n_{\mathrm{c}} $, (i)–(l) $ n_{\mathrm{e}} = 0.64 n_{\mathrm{c}} $, when a $ a = 0.2 $ circularly polarized vortex light beam with $ l = 1, {\sigma_x} = 1 $ propagates in the plasma over different distances (a)(e)(i) $ x = 5\; {\text{μm}} $, (b)(f)(j) $ x = 10\; {\text{μm}} $, (c)(g)(k) $ x = 15\; {\text{μm}} $, (d)(h)(l) $ x = 20\; {\text{μm}} $
-
[1] Beth R A 1936 Phys. Rev. 50 115
Google Scholar
[2] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[3] He H, Friese M E J, Heckenberg N R, Rubinsztein-Dunlop H 1995 Phys. Rev. Lett. 75 826
Google Scholar
[4] Wang J, Yang J Y, Fazal I M, Ahmed N, Yan Y, Huang H, Ren Y, Yue Y, Dolinar S, Tur M, Willner A E 2012 Nat. Photonics 6 488
Google Scholar
[5] Bozinovic N, Yue Y, Ren Y, Tur M, Kristensen P, Huang H, Willner A E, Ramachandran S 2013 Science 340 1545
Google Scholar
[6] Tamburini F, Anzolin G, Umbriaco G, Bianchini A, Barbieri C 2006 Phys. Rev. Lett. 97 163903
Google Scholar
[7] Harwit M 2003 The Astrophysical Journal 597 1266
Google Scholar
[8] Tamburini F, Thidé B, Molina-Terriza G, Anzolin G 2011 Nat. Phys. 7 195
Google Scholar
[9] Tamburini F, Thidé B, Della Valle M 2019 Mon. Not. R. Astron. Soc. 492 L22
[10] Shi Y, Shen B, Zhang L, Zhang X, Wang W, Xu Z 2014 Phys. Rev. Lett. 112 235001
Google Scholar
[11] Vieira J, Trines R M G M, Alves E P, Fonseca R A, Mendonca J T, Bingham R, Norreys P, Silva L O 2016 Nat. Commun. 7 10371
Google Scholar
[12] Leblanc A, Denoeud A, Chopineau L, Mennerat G, Martin P, Quéré F 2017 Nat. Phys. 13 440
Google Scholar
[13] Qu K, Jia Q, Fisch N J 2017 Phys. Rev. E 96 053207
Google Scholar
[14] Shi Y, Blackman D, Stutman D, Arefiev A 2021 Phys. Rev. Lett. 126 234801
Google Scholar
[15] Vieira J, Mendonca J T 2014 Phys. Rev. Lett. 112 215001
Google Scholar
[16] Brabetz C, Busold S, Cowan T, Deppert O, Jahn D, Kester O, Roth M, Schumacher D, Bagnoud V 2015 Phys. Plasmas 22 013105
Google Scholar
[17] Zhang X, Shen B, Zhang L, Xu J, Wang X, Wang W, Yi L, Shi Y 2014 New J. Phys. 16 123051
Google Scholar
[18] Willim C, Vieira J, Malka V, Silva L O 2023 Phys. Rev. Res. 5 023083
Google Scholar
[19] Zhang X, Shen B, Shi Y, Wang X, Zhang L, Wang W, Xu J, Yi L, Xu Z 2015 Phys. Rev. Lett. 114 173901
Google Scholar
[20] Denoeud A, Chopineau L, Leblanc A, Quéré F 2017 Phys. Rev. Lett. 118 033902
Google Scholar
[21] Wang J W, Zepf M, Rykovanov S G 2019 Nat. Commun. 10 5554
Google Scholar
[22] Li S, Zhang X, Gong W, Bu Z, Shen B 2020 New J. Phys. 22 013054
Google Scholar
[23] Chen Y Y, Li J X, Hatsagortsyan K Z, Keitel C H 2018 Phys. Rev. Lett. 121 074801
Google Scholar
[24] Zhu X L, Chen M, Yu T P, Weng S M, Hu L X, McKenna P, Sheng Z M 2018 Appl. Phys. Lett. 112 174102
Google Scholar
[25] Ali S, Davies J R, Mendonca J T 2010 Phys. Rev. Lett. 105 035001
Google Scholar
[26] Shi Y, Vieira J, Trines R M G M, Bingham R, Shen B F, Kingham R J 2018 Phys. Rev. Lett. 121 145002
Google Scholar
[27] Longman A, Fedosejevs R 2021 Phys. Rev. Res. 3 043180
Google Scholar
[28] Liu W, Jia Q, Zheng J 2023 Matter Radiat. Extremes 8 014405
Google Scholar
[29] Wang W P, Jiang C, Shen B F, Yuan F, Gan Z M, Zhang H, Zhai S H, Xu Z Z 2019 Phys. Rev. Lett. 122 024801
Google Scholar
[30] Baumann C, Pukhov A 2018 Phys. Plasmas 25 083114
Google Scholar
[31] Mendonca J T, Thidé B, Then H 2009 Phys. Rev. Lett. 102 185005
Google Scholar
[32] Nuter R, Korneev P, Tikhonchuk V T 2022 Phys. Plasmas 29 062101
Google Scholar
[33] Ji Y, Lian C W, Shi Y, Yan R, Cao S, Ren C, Zheng J 2023 Phys. Rev. Res. 5 L022025
Google Scholar
[34] Ju L B, Huang T W, Xiao K D, Wu G Z, Yang S L, Li R, Yang Y C, Long T Y, Zhang H, Wu S Z, Qiao B, Ruan S C, Zhou C T 2016 Phys. Rev. E 94 033202
Google Scholar
[35] 范海玲, 郭志坚, 李明强, 卓红斌 2023 72 014206
Google Scholar
Fan H L, Guo Z J, Li M Q, Zhuo H B 2023 Acta Phys. Sin. 72 014206
Google Scholar
[36] Arber T D, Bennett K, Brady C S, Lawrence-Douglas A, Ramsay M G, Sircombe N J, Gillies P, Evans R G, Schmitz H, Bell A R, Ridgers C P 2015 Plasma Phys. Control. Fusion 57 113001
Google Scholar
[37] Gibbon P 2005 Short Pulse Laser Interactions With Matter: an Introduction (London: Imperial College Press) pp31–36, 84, 92, 99, 106
[38] Esarey E, Ting A, Sprangle P, Umstadter D, Liu X 1993 IEEE Trans. Plasma Sci. 21 95
Google Scholar
[39] 盛政明 2014 强场激光物理研究前沿(上海: 上海交通大学出版社) 第21页
Sheng Z M 2014 Advances in High Field Laser Physics (Shanghai: Shanghai Jiao Tong University Press) pp21
[40] Liu C, Tripathi V, Eliasson B 2019 High-Power Laser-Plasma Interaction (Cambridge: Cambridge University Press) p89
DownLoad:
Catalog
Metrics
- Abstract views: 2258
- PDF Downloads: 127
- Cited By: 0
