-
Oxide interfaces manifest many fascinating phenomena with synergetic correlations among multiple degrees of freedom, including the interplay of broken symmetry, lattice mismatch, charge transfer, spatial confinement. In particular, the interface superconductivity in oxide heterostructure has attracted extensive attention due to the rich underlying physical connotations. The interfaces not only provide alternative research platforms with respect to the bulk material counterpart for exploring new superconductors and investigating superconducting mechanisms, but also create new opportunities for applying superconductors to future electronic devices. In recent years, owing to the rapid development of heteroepitaxial techniques and accurate characterization methods, researchers have found quasi-two-dimensional interface superconductivity in various oxide heterostructures and revealed numerous novel quantum phenomena associated with interface superconductivity, which not only promotes the development of condensed matter physics, but also lays important foundation for the practical application of interface superconductivity. In this brief review, we mainly focus on the quasi-two-dimensional superconductivity at oxide interface. Taking the typical quasi-two-dimensional superconductivity at the LaAlO3/SrTiO3 interface and copper oxides such as La2CuO4/La1.56Sr0.44CuO4 for example, we summarize and examine some novel physical phenomena with interface superconductivity in complex oxide heterostructures. Then we address the related problems that remain to be solved, and finally we prospect the possible future development of the interface superconductivity.
-
Keywords:
- oxide /
- interface superconductivity /
- cuprate superconductor /
- two-dimensional electron gas
[1] Onnes H K 1911 Comm. Phys. Lab. Univ. Leiden 120b 122
[2] Bardeen J, Cooper L N, Schrieffer J R 1957 Phys. Rev. 106 162
Google Scholar
[3] Bednorz J G, Müller K A 1986 Z. Phys. B:Condens. Matter 64 189
Google Scholar
[4] Kamihara Y, Watanabe T, Hirano M, Hosono H 2008 J. Am. Chem. Soc. 130 3296
Google Scholar
[5] McMillan W L 1968 Phys. Rev. 167 331
Google Scholar
[6] Strongin M, Kammerer O F, Crow J E, Parks R D, Douglass D H, Jensen M A 1968 Phys. Rev. Lett. 21 1320
[7] Kroemer H 2001 Rev. Mod. Phys. 73 783
Google Scholar
[8] Reyren N, Thiel S, Caviglia A D, Kourkoutis L F, Hammerl G, Richter C, Schneider C W, Kopp T, Rüetschi A S, Jaccard D, Gabay M, Muller D A, Triscone J M, Mannhart J 2007 Science 317 1196
Google Scholar
[9] Gozar A, Logvenov G, Kourkoutis L F, Bollinger A T, Giannuzzi L A, Muller D A, Bozovic I 2008 Nature 455 782
Google Scholar
[10] Bozovic I 2001 IEEE Trans. Appl. Supercond. 11 2686
Google Scholar
[11] Wang Q Y, Li Z, Zhang W H, Zhang Z C, Zhang J S, Li W, Ding H, Ou Y B, Deng P, Chang K, Wen J, Song C L, He K, Jia J F, Ji S H, Wang Y Y, Wang L L, Chen X, Ma X C, Xue Q K 2012 Chin. Phys. Lett. 29 037402
Google Scholar
[12] Saito Y, Nojima T, Iwasa Y 2016 Nat. Rev. Mater. 2 16094
Google Scholar
[13] Uchihashi T 2017 Supercond. Sci. Technol. 30 013002
Google Scholar
[14] Xing Y, Zhang H M, Fu H L, Liu H, Sun Y, Peng J P, Wang F, Lin X, Ma X C, Xue Q K, Wang J, Xie X C 2015 Science 350 542
Google Scholar
[15] Ohtomo A, Muller D A, Grazul J L, Hwang H Y 2002 Nature 419 378
Google Scholar
[16] Ohtomo A, Hwang H Y 2004 Nature 427 423
Google Scholar
[17] Cantoni C, Gazquez J, Miletto Granozio F, Oxley M P, Varela M, Lupini A R, Pennycook S J, Aruta C, di Uccio U S, Perna P, Maccariello D 2012 Adv. Mater. 24 3952
Google Scholar
[18] Cen C, Thiel S, Hammerl G, Schneider C W, Andersen K E, Hellberg C S, Mannhart J, Levy J 2008 Nat. Mater. 7 298
Google Scholar
[19] Nakagawa N, Hwang H Y, Muller D A 2006 Nat. Mater. 5 204
Google Scholar
[20] Lee H, Campbell N, Lee J, Asel T J, Paudel T R, Zhou H, Lee J W, Noesges B, Seo J, Park B, Brillson L J, Oh S H, Tsymbal E Y, Rzchowski M S, Eom C B 2018 Nat. Mater. 17 231
Google Scholar
[21] Chen Y Z, Bovet N, Trier F, Christensen D V, Qu F M, Andersen N H, Kasama T, Zhang W, Giraud R, Dufouleur J, Jespersen T S, Sun J R, Smith A, Nygård J, Lu L, Büchner B, Shen B G, Linderoth S, Pryds N 2013 Nat. Commun. 4 1371
Google Scholar
[22] Lee S W, Liu Y, Heo J, Gordon R G 2012 Nano Lett. 12 4775
Google Scholar
[23] Liu Z Q, Li C J, Lü W M, Huang X H, Huang Z, Zeng S W, Qiu X P, Huang L S, Annadi A, Chen J S, Coey J M D, Venkatesan T, Ariando 2013 Phys. Rev. X 3 021010
[24] Zhang M, Chen Z, Mao B, Li Q, Bo H, Ren T, He P, Liu Z, Xie Y 2018 Phys. Rev. Mater. 2 065002
Google Scholar
[25] Chen Z, Chen X, Mao B, Li Q, Zhang M, Bo H, Liu Z, Tian H, Zhang Z, Xie Y 2018 Adv. Mater. Interfaces 5 1801216
Google Scholar
[26] Chen Z, Zhang M, Ren T, Xie Y 2019 J. Phys. Condens. Matter 31 505002
Google Scholar
[27] Yu L, Zunger A 2014 Nat. Commun. 5 5118
Google Scholar
[28] Caviglia A D, Gariglio S, Reyren N, Jaccard D, Schneider T, Gabay M, Thiel S, Hammerl G, Mannhart J, Triscone J M 2008 Nature 456 624
Google Scholar
[29] Parendo K A, Tan K H S B, Bhattacharya A, Eblen Zayas M, Staley N E, Goldman A M 2005 Phys. Rev. Lett. 94 197004
Google Scholar
[30] Aubin H, Marrache Kikuchi C A, Pourret A, Behnia K, Bergé L, Dumoulin L, Lesueur J 2006 Phys. Rev. B 73 094521
Google Scholar
[31] Sachdev S 2011 Quantum Phase Transitions (2nd Ed.) (Cambridge: Cambridge University Press) pp293–331
[32] Biscaras J, Bergeal N, Kushwaha A, Wolf T, Rastogi A, Budhani R C, Lesueur J 2010 Nat. Commun. 1 89
Google Scholar
[33] Biscaras J, Bergeal N, Hurand S, Feuillet-Palma C, Rastogi A, Budhani R C, Grilli M, Caprara S, Lesueur J 2013 Nat. Mater. 12 542
Google Scholar
[34] Herranz G, Singh G, Bergeal N, Jouan A, Lesueur J, Gázquez J, Varela M, Scigaj M, Dix N, Sánchez F, Fontcuberta J 2015 Nat. Commun. 6 6028
Google Scholar
[35] Monteiro A M R V L, Groenendijk D J, Groen I, de Bruijckere J, Gaudenzi R, van der Zant H S J, Caviglia A D 2017 Phys. Rev. B 96 020504
Google Scholar
[36] Caviglia A D, Gabay M, Gariglio S, Reyren N, Cancellieri C, Triscone J M 2010 Phys. Rev. Lett. 104 126803
Google Scholar
[37] Rout P K, Maniv E, Dagan Y 2017 Phys. Rev. Lett. 119 237002
Google Scholar
[38] Zhong Z, Tóth A, Held K 2013 Phys. Rev. B 87 161102
Google Scholar
[39] Michaeli K, Potter A C, Lee P A 2012 Phys. Rev. Lett. 108 117003
Google Scholar
[40] Abrahams E, Anderson P W, Licciardello D C, Ramakrishnan T V 1979 Phys. Rev. Lett. 42 673
Google Scholar
[41] Jaeger H M, Haviland D B, Orr B G, Goldman A M 1989 Phys. Rev. B 40 182
Google Scholar
[42] Bøttcher C G L, Nichele F, Kjaergaard M, Suominen H J, Shabani J, Palmstrøm C J, Marcus C M 2018 Nat. Phys. 14 1138
Google Scholar
[43] Chakravarty S, Yin L, Abrahams E 1998 Phys. Rev. B 58 R559
Google Scholar
[44] Phillips P, Dalidovich D 2003 Science 302 243
Google Scholar
[45] Spivak B, Kravchenko S V, Kivelson S A, Gao X P A 2010 Rev. Mod. Phys. 82 1743
Google Scholar
[46] Chen Z, Swartz A G, Yoon H, Inoue H, Merz T A, Lu D, Xie Y, Yuan H, Hikita Y, Raghu S, Hwang H Y 2018 Nat. Commun. 9 4008
Google Scholar
[47] Richter C, Boschker H, Dietsche W, Fillis Tsirakis E, Jany R, Loder F, Kourkoutis L F, Muller D A, Kirtley J R, Schneider C W, Mannhart J 2013 Nature 502 528
Google Scholar
[48] Zhang H, Yun Y, Zhang X, Zhang H, Ma Y, Yan X, Wang F, Li G, Li R, Khan T, Chen Y, Liu W, Hu F, Liu B, Shen B, Han W, Sun J 2018 Phys. Rev. Lett. 121 116803
Google Scholar
[49] Liu C, Yan X, Jin D, Ma Y, Hsiao H W, Lin Y, Bretz Sullivan T M, Zhou X, Pearson J, Fisher B, Jiang J S, Han W, Zuo J M, Wen J, Fong D D, Sun J, Zhou H, Bhattacharya A 2021 Science 371 716
Google Scholar
[50] Chen Z, Liu Z, Sun Y, Chen X, Liu Y, Zhang H, Li H, Zhang M, Hong S, Ren T, Zhang C, Tian H, Zhou Y, Sun J, Xie Y 2021 Phys. Rev. Lett. 126 026802
Google Scholar
[51] Sun Y, Liu Y, Hong S, Chen Z, Zhang M, Xie Y 2021 Phys. Rev. Lett. 127 086804
Google Scholar
[52] Chen Z, Liu Y, Zhang H, Liu Z, Tian H, Sun Y, Zhang M, Zhou Y, Sun J, Xie Y 2021 Science 372 721
Google Scholar
[53] Christiansen C, Hernandez L M, Goldman A M 2002 Phys. Rev. Lett. 88 037004
Google Scholar
[54] Liu C, Zhou X, Hong D, Fisher B, Zheng H, Pearson J, Jiang J S, Jin D, Norman M R, Bhattacharya A 2023 Nat. Commun. 14 951
Google Scholar
[55] Schooley J F, Hosler W R, Cohen M L 1964 Phys. Rev. Lett. 12 474
Google Scholar
[56] Ueno K, Nakamura S, Shimotani H, Ohtomo A, Kimura N, Nojima T, Aoki H, Iwasa Y, Kawasaki M 2008 Nat. Mater. 7 855
Google Scholar
[57] Ueno K, Nakamura S, Shimotani H, Yuan H T, Kimura N, Nojima T, Aoki H, Iwasa Y, Kawasaki M 2011 Nat. Nanotech. 6 408
Google Scholar
[58] Ren T, Li M, Sun X, Ju L, Liu Y, Hong S, Sun Y, Tao Q, Zhou Y, Xu Z A, Xie Y 2022 Sci. Adv. 8 eabn4273
Google Scholar
[59] Imada M, Fujimori A, Tokura Y 1998 Rev. Mod. Phys. 70 1039
Google Scholar
[60] Di Stasio M, Müller K A, Pietronero L 1990 Phys. Rev. Lett. 64 2827
Google Scholar
[61] Shen Z X, Dessau D S, Wells B O, King D M, Spicer W E, Arko A J, Marshall D, Lombardo L W, Kapitulnik A, Dickinson P, Doniach S, DiCarlo J, Loeser T, Park C H 1993 Phys. Rev. Lett. 70 1553
Google Scholar
[62] Norton D P, Chakoumakos B C, Budai J D, Lowndes D H, Sales B C, Thompson J R, Christen D K 1994 Science 265 2074
Google Scholar
[63] Balestrino G, Martellucci S, Medaglia P G, Paoletti A, Petrocelli G, Varlamov A A 1998 Phys. Rev. B 58 R8925
Google Scholar
[64] Di Castro D, Salvato M, Tebano A, Innocenti D, Aruta C, Prellier W, Lebedev O I, Ottaviani I, Brookes N B, Minola M, Moretti Sala M, Mazzoli C, Medaglia P G, Ghiringhelli G, Braicovich L, Cirillo M, Balestrino G 2012 Phys. Rev. B 86 134524
Google Scholar
[65] Aruta C, Schlueter C, Lee T L, Di Castro D, Innocenti D, Tebano A, Zegenhagen J, Balestrino G 2013 Phys. Rev. B 87 155145
Google Scholar
[66] Salvato M, Tieri G, Balestrino G, Di Castro D 2015 Supercond. Sci. Technol. 28 095012
Google Scholar
[67] Salvato M, Ottaviani I, Lucci M, Cirillo M, Di Castro D, Innocenti D, Tebano A, Balestrino G 2013 J. Phys. Condens. Matter 25 335702
Google Scholar
[68] Kitazawa K, Sakai M, Uchida S i, Takagi H, Kishio K, Kanbe S, Tanaka S, Fueki K 1987 Jpn. J. Appl. Phys. 26 L342
Google Scholar
[69] Logvenov G, Gozar A, Bozovic I 2009 Science 326 699
Google Scholar
[70] Smadici S, Lee J C T, Wang S, Abbamonte P, Logvenov G, Gozar A, Cavellin C D, Bozovic I 2009 Phys. Rev. Lett. 102 107004
Google Scholar
[71] Ju L, Ren T, Li Z, Liu Z, Shi C, Liu Y, Hong S, Wu J, Tian H, Zhou Y, Xie Y 2022 Phys. Rev. B 105 024516
Google Scholar
[72] Bert J A, Kalisky B, Bell C, Kim M, Hikita Y, Hwang H Y, Moler K A 2011 Nat. Phys. 7 767
Google Scholar
[73] Li L, Richter C, Mannhart J, Ashoori R C 2011 Nat. Phys. 7 762
Google Scholar
[74] Dikin D A, Mehta M, Bark C W, Folkman C M, Eom C B, Chandrasekhar V 2011 Phys. Rev. Lett. 107 056802
Google Scholar
[75] Nayak C, Simon S H, Stern A, Freedman M, Das Sarma S 2008 Rev. Mod. Phys. 80 1083
Google Scholar
[76] Zhang Y, Gu Y, Li P, Hu J, Jiang K 2022 Phys. Rev. X 12 041013
-
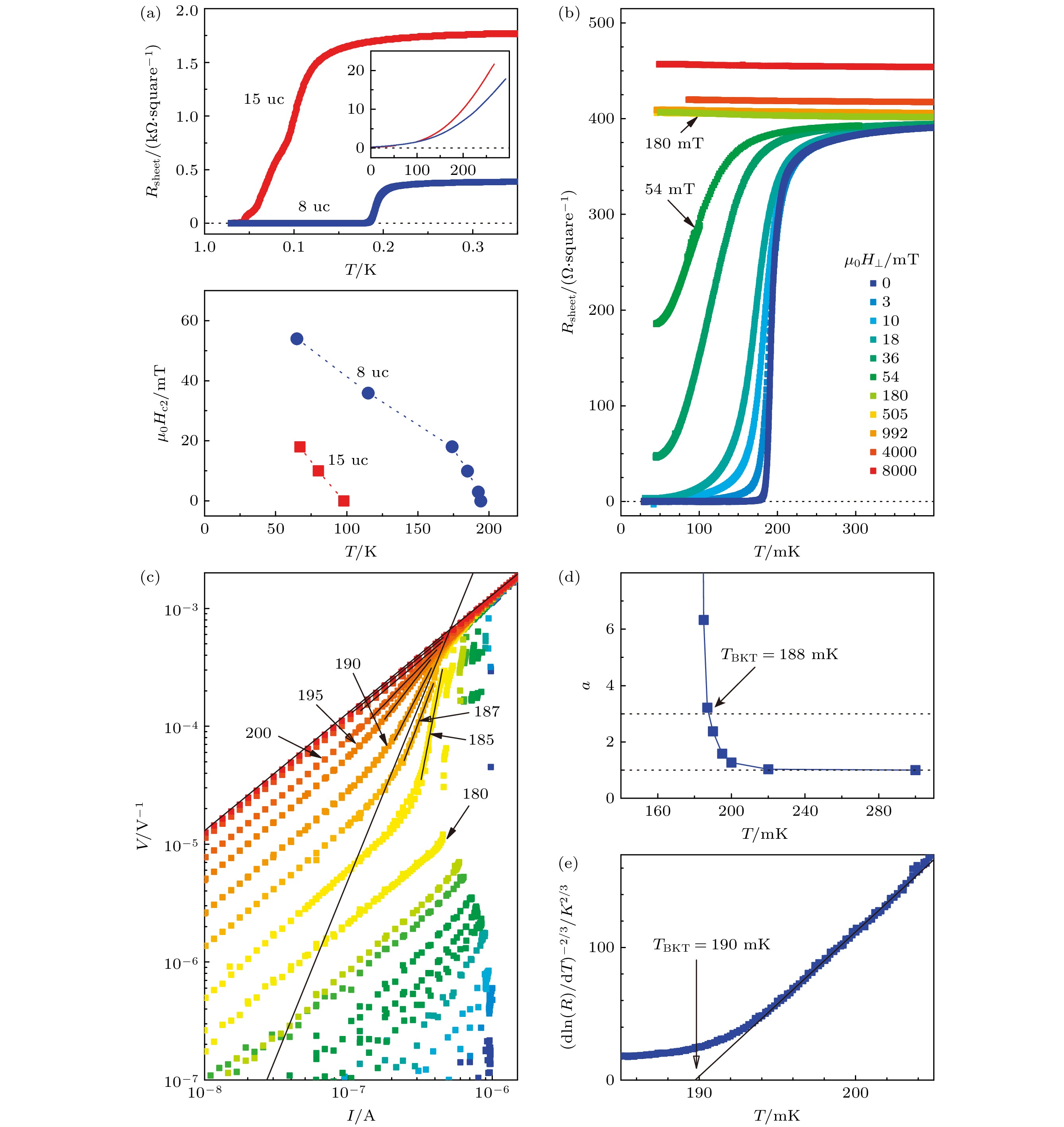
图 1 LAO/STO界面超导[8] (a) 8 uc (unit cells, uc)与15 uc厚度样品的电阻-温度依赖关系(上), 两样品施加垂直界面磁场下的电阻-温度依赖关系(下). (b) 8 uc厚度样品施加垂直界面磁场时的电阻与温度依赖关系. (c) 对数坐标下的V-I关系曲线. 两条长黑线对应V = RI与V
$ \sim $ I 3, 数字代表V-I曲线测量的温度(mK), 可以得到 187 mK < TBKT < 190 mK, 其中TBKT为BKT相变温度. (d) 由图(c)得到的幂次指数a的温度依赖关系, 其中a为V$ \sim $ Ia的幂次指数. (e) [dln(R)/dT ]–2/3坐标下8 uc样品的R-T曲线, 实线为拟合的BKT相变温度, TBKT = 190 mKFigure 1. LAO/STO interface superconductivity[8]. (a) The resistance-temperature dependence of 8 uc (unit cells, uc) and 15 uc thickness samples (upper), and the resistance-temperature dependence of the two samples under the vertical interface magnetic field (lower). (b) The resistance of 8 uc thick samples is temperature-dependent when the vertical interface magnetic field is applied. (c) V-I relation curve in logarithmic coordinates. The two long black lines correspond to V = RI and V
$ \sim $ I 3. The number represents the temperature measured by the V-I curve, and the unit is mK. We can obtain 187 mK < TBKT < 190 mK, Where TBKT is the BKT phase transition temperature. (d) The temperature dependence of the power exponent a obtained from the (c) diagram temperature, where a is the power exponent of V$ \sim $ Ia. (e) The R-T curve of the 8 uc sample under the [dln(R)/dT ]–2/3 coordinate, the solid line is the fitted BKT phase transition temperature, TBKT = 190 mK.
图 2 LAO/ATO界面绝缘-超导相变[28] (a) 蓝色曲线为1.5 K测得的不同电容的电介质可调性曲线, 电容C-V曲线施加了1 V的直流电压; 红色曲线为栅极电压调控流子浓度曲线. 在虚线区域发现量子临界行为, 所以在此区域应满足 δn2D∝δV. (b) 场效应器件示意图. (c) 左图为半对数坐标下施加不同栅极电压的Rsheet-T依赖关系曲线, 其中虚线是电阻量子RQ; 右图为线性坐标下施加不同栅极电压的Rsheet-T依赖关系曲线. (d) LAO/STO界面超导随电压调控相图. 右边坐标轴和蓝点是BKT相变温度TBKT随栅极电压的变化, 揭示了超导区域的相图. 左边坐标轴和红三角是400 mK的正常态随栅极电压变化曲线, 实线是利用标度关系
${T}_{{\rm{B}}{\rm{K}}{\rm{T}}}\propto {(V-{V}_{{\rm{c}}})}^{z\bar{\nu }}$ 得到的量子临界点, 其中$z\bar{\nu }=2/3$ Figure 2. LAO/ATO interface insulation-superconducting phase transition[28]. (a) Blue curve: the adjustable curve of dielectric with different capacitance measured at 1.5 K, and the capacitance C-V curve applies 1 V DC voltage. Red curve: grid voltage regulated streamer concentration curve. The dashed lines indicate the region where the quantum critical behavior is observed. Note that in this region δn2D∝δV. (b) Schematic diagram of field effect device. (c) Left: Rsheet-T dependence curve of different grid voltage applied in semi-logarithmic coordinates. The dotted line is the resistance quantum RQ. Right: Rsheet-T dependence curve of different grid voltage applied in linear coordinates. (d) The phase diagram of LAO/STO interface superconductivity is regulated by voltage. The right coordinate axis and blue point are the changes of BKT phase transition temperature TBKT with grid voltage, revealing the phase diagram of superconducting region. The left coordinate axis and the red triangle are the normal state curve of 400 mK versus grid voltage, and the solid line is based on the scale relationship
${T}_{{\rm{B}}{\rm{K}}{\rm{T}}}\propto {(V-{V}_{{\rm{c}}})}^{z\bar{\nu }}$ . The obtained quantum critical point, where$z\bar{\nu }=2/3$ .
图 3 LTO/STO界面准二维超导-绝缘体相变[33] (a)不同磁场下电阻RS与温度的依赖关系, 其中插图为Tc, GS = 1/RS与调控电压VG的依赖关系; (b) BC, BX (左坐标), Tc (右坐标)与调控电压关系图; (c)在两个转变时zν与VG关系, BC是低温区域, BX是高温区域, 其中BX随调控电压VG单调变化, 最后趋于饱和, 其饱和值为Bd
Figure 3. LTO/STO interface quasi-two-dimensional superconduction-insulator phase transition[33]: (a) Sheet resistance RS as a function of temperature for different magnetic fields, where the inset shows Tc and GS = 1/RS as a function of the gate voltage VG; (b) relation diagram of BC, BX (left coordinate), Tc (right coordinate) and regulated voltage; (c) at two transitions, zν in relation to VG, BC is the low temperature region and BX is the high temperature region, where BX increases with VG and then saturates to a value Bd
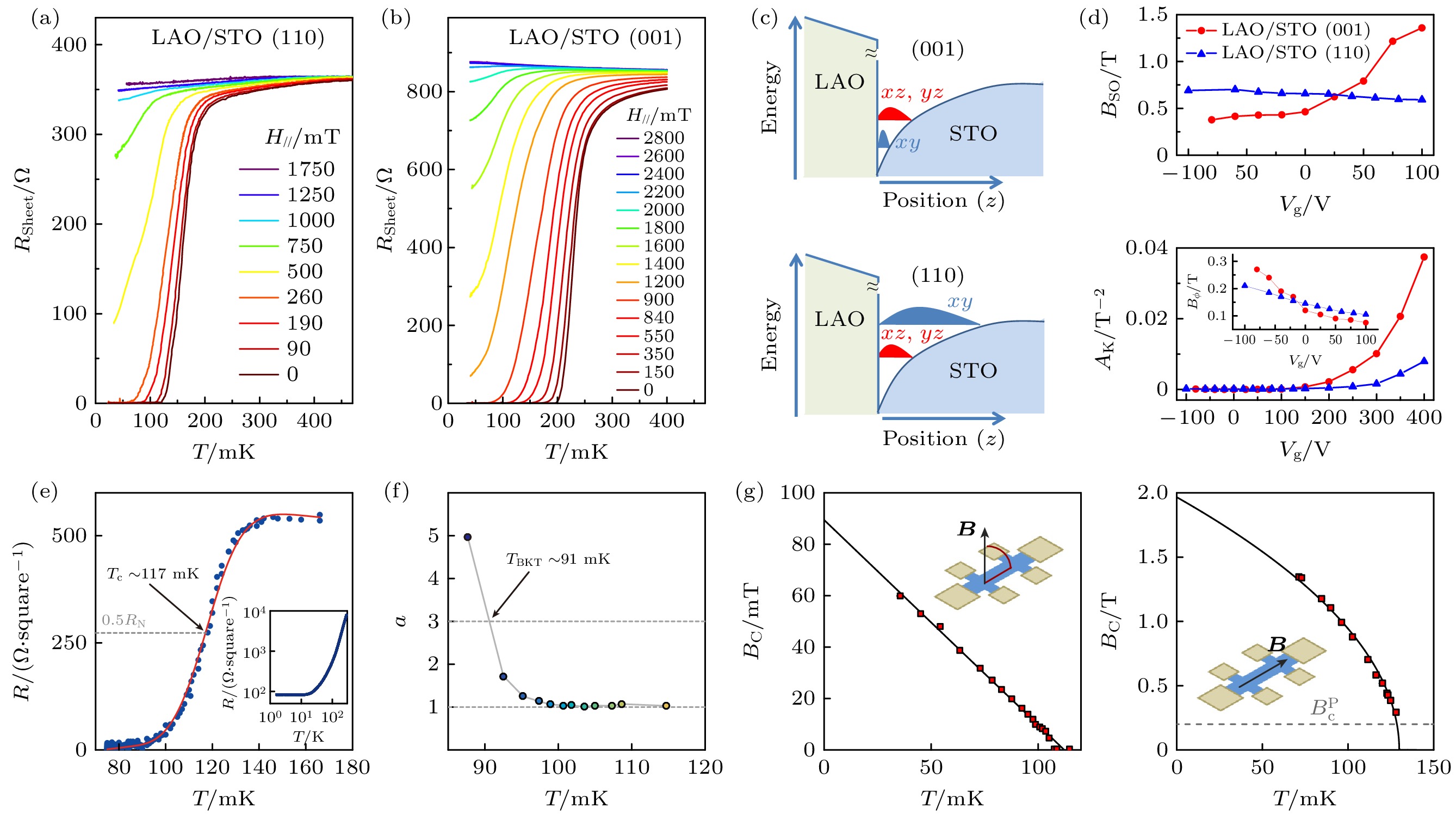
图 4 不同取向STO上构筑的LAO/STO异质界面的输运测试 (a) (110)取向STO上界面超导受平行于界面磁场调控的电阻-温度依赖关系, 样品厚度为14 MLs (monolayers, MLs), 约为3.8 nm[34]. (b) (001)取向STO界面超导受平行于界面磁场调控的电阻-温度依赖关系, 样品厚度为10 MLs, 约为3.8 nm[34]. (c) 不同取向STO上LAO/STO异质界面能带示意图. 上: 对于(001)取向的STO, dxz/dyz轨道能量高于dxy轨道能量; 下: 对于(110)取向的STO, dxz/dyz轨道能量低于dxy轨道能量[34]. (d) 上: SOC项与调控电压的依赖关系; 下: Kohler AK 和非弹性的BΦ(插图)与调控电压依赖关系[34]. (e) LAO/STO(111)异质界面施加30 V栅极电压下的电阻-温度依赖关系, 插图为R-T曲线[35]. (f) LAO/STO(111)异质界面根据V
$ \sim $ Ia拟合V-I关系得到的参数a随温度的变化曲线[35]. (g) LAO/STO(111)异质界面的面外和面内上临界场-温度依赖关系, 虚线对应Pauli极限磁场大小[35]Figure 4. Transport test of LAO/STO heterointerface constructed on different orientation STO. (a) The interface superconductivity on (110) oriented STO is controlled by a resistance-temperature dependence parallel to the interface magnetic field, and the sample thickness is 14 MLs (monolayers, MLs), corresponding to 3.8 nm[34]. (b) (001) oriented STO interface superconductivity is controlled by a resistance-temperature dependence parallel to the interface magnetic field, and the sample thickness is 10 MLs, corresponding to 3.8 nm[34]. (c) Schematic diagram of LAO/STO heterointerface energy band on different orientation STO. Above: for STO with (001) orientation, dxz/dyz orbital energy is higher than dxy orbital energy; Below: for the (110) oriented STO, the dxz/dyz orbital energy is lower than the dxy orbital energy[34]. (d) Above: the dependence of SOC term on regulated voltage; Below: Kohler AK and inelastic BΦ (Illustration) dependence on regulated voltage[34]. (e) The resistance-temperature dependence under 30 V grid voltage of LAO/STO (111) heterointerface. Illustrated: R-T curve[35]. (f) Curve of parameter a with temperature of LAO/STO (111) heterointerface obtained by fitting V-I relationship with V
$ \sim $ Ia[35]. (g) The out-of-plane and in-plane upper critical field-temperature dependence of LAO/STO(111) heterointerface, and the dotted line corresponds to the Pauli limit magnetic field size[35].
图 5 LAO/STO(111)异质界面上不同参数在门电压调控下的变化[37] (a)不同栅极电压下的电阻-温度依赖关系; (b)Tc, RS (350 mK)与逆霍尔系数1/|eRH|随栅极电压变化曲线; (c)上图为εSO随栅极电压变化曲线, 下图为拟合弱反局域化得到的非弹性场参数Hi、自旋-轨道场参数HSO 随栅极电压变化曲线
Figure 5. Changes of different parameters on LAO/STO(111) heterostructure under gate voltage regulation[37]: (a) Resistance-temperature dependence at different grid voltages; (b) Tc, RS (350 mK), inverse Hall coefficient 1/|eRH| change curve with grid voltage; (c) the figure above shows εSO versus grid voltage, below shows the curve of inelastic fields Hi and d spin-orbit fields HSO with grid voltage obtained by fitting weak anti-localization.
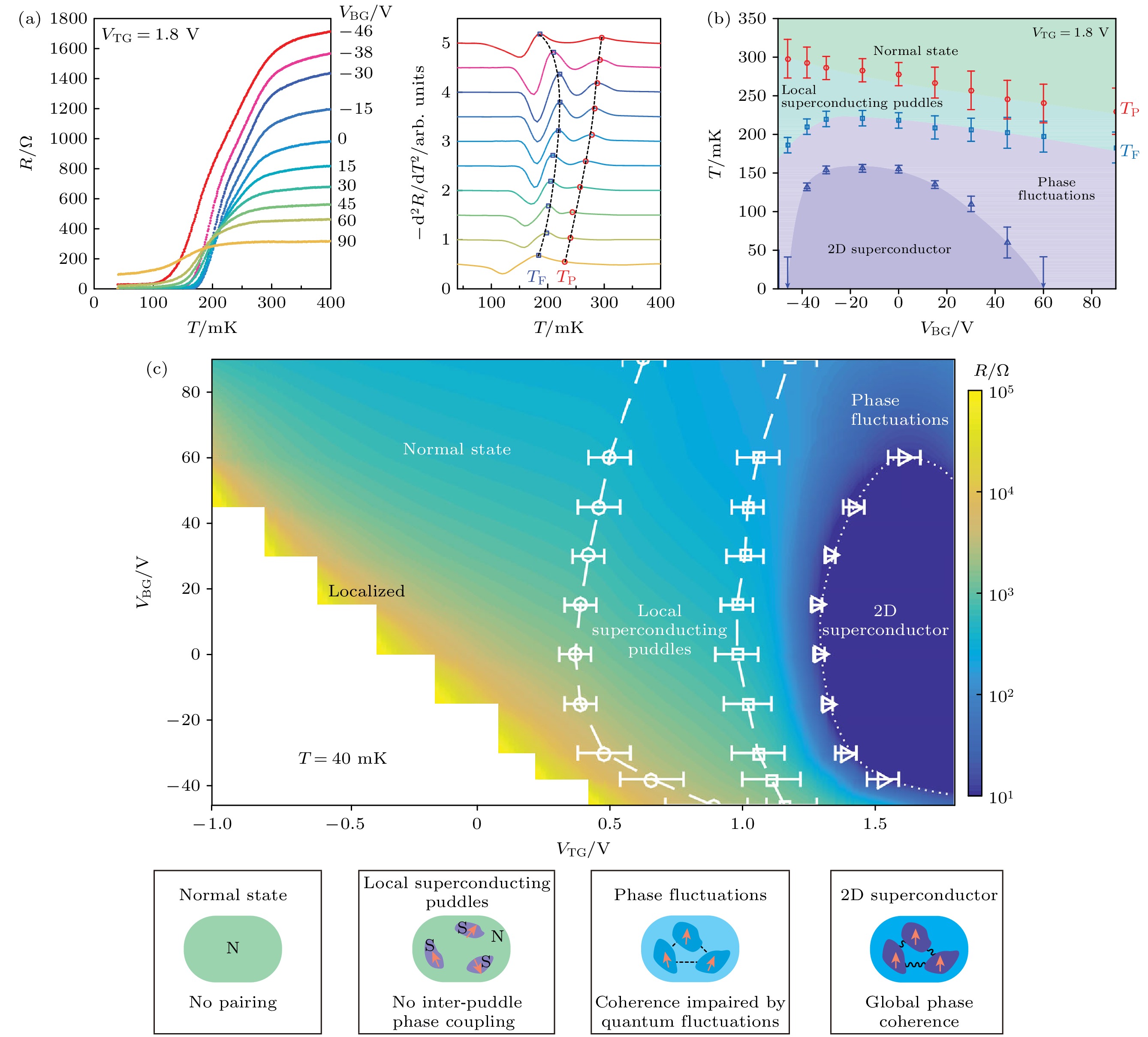
图 6 顶栅调控下LAO/STO异质界面输运测试[46] (a) 8 uc厚度的LAO/STO异质界面在不同顶栅电压下电阻-温度依赖关系; (b)对图(a)曲线进行二阶微分, 两个峰位分别定义为温度TP和TF; (c)根据图(a)和图(b)得到的顶栅调控的相图
Figure 6. Transport test of LAO/STO heterointerface under top gate control[46]: (a) Resistance-temperature dependence of LAO/STO heterostructure with 8 uc thickness at different top gate voltages; (b) second order differential is performed on the curve of panel (a), and the two peak positions are defined as temperature TP and TF, respectively; (c) the phase diagram of top grid control obtained according to panel (a), (b).
图 7 背栅调控下LAO/STO异质界面输运测试与得到的相图[46] (a)左图为不同背栅电压下的电阻-温度依赖关系, 右图为对左图曲线进行二阶微分, 两个峰位分别定义为温度TP和TF; (b) 根据图(a)得到的背栅调控的相图; (c)综合顶栅极、背栅极调控得到的相图以及相图中不同基态的示意图
Figure 7. Transport test and phase diagram of LAO/STO heterointerface under back gate control[46]: (a) Left figure shows resistance-temperature dependence under different back-gate voltages; right shows that the second order differential is performed on the curve of left figure, and the two peak positions are defined as temperature TP and TF respectively; (b) the phase diagram of back gate regulation obtained according to panel (a); (c) the phase diagram of top gate and back gate regulation and the schematic diagram of different ground states in the phase diagram.
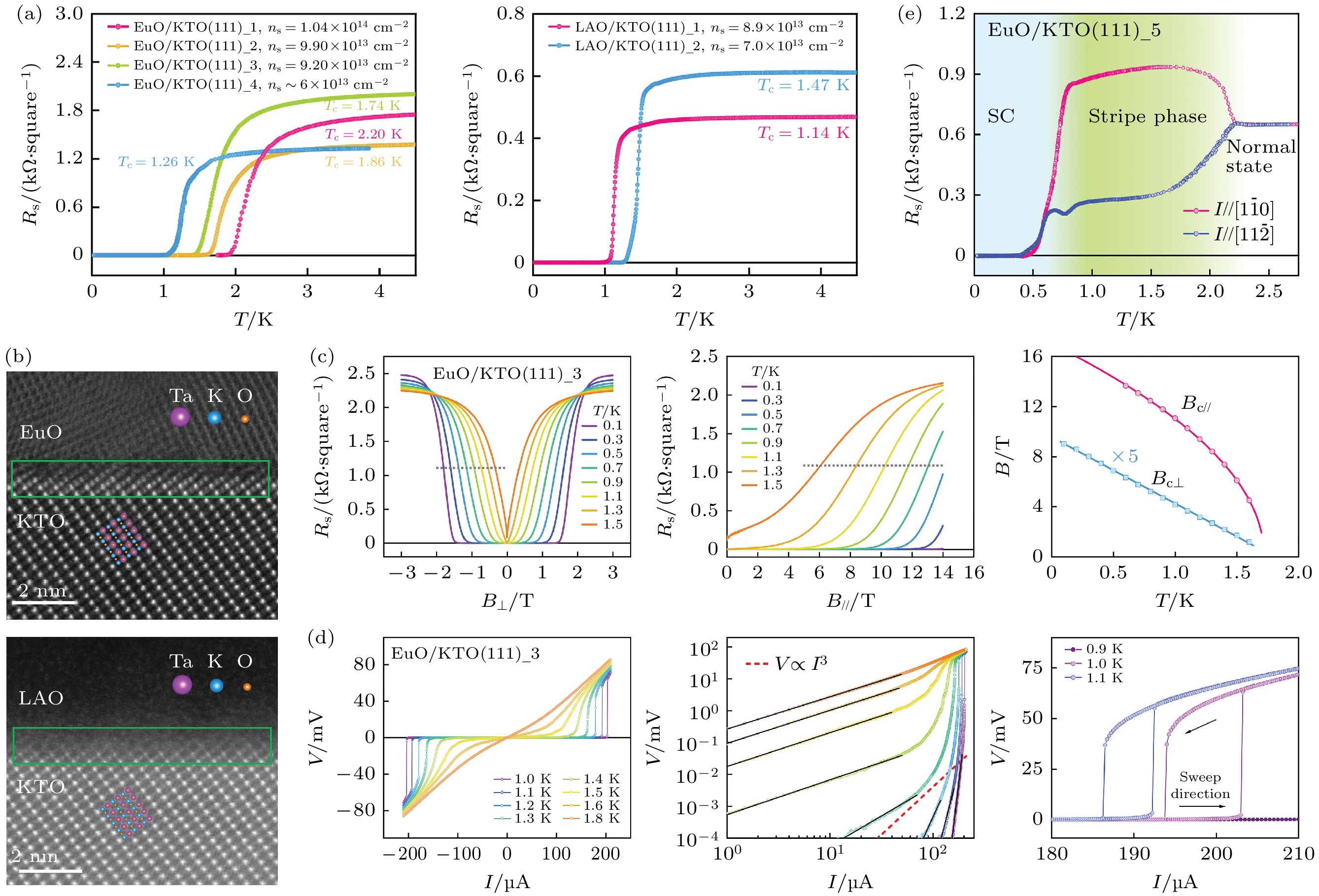
图 8 EuO/KTO(111)异质界面超导[49] (a) KTO(111)衬底上多个样品的Rs-T曲线, 不同材料和载流子浓度都表现出超导. (b) KTO(111)衬底上生长EuO (上)和LAO (下)的STEM图, 绿线框区域为界面附近. (c) 左: EuO/KTO样品施加垂直于界面的磁场下的Rsheet-T依赖关系; 中: EuO/KTO样品施加平行于界面的磁场下的Rsheet-T依赖关系; 右: 根据前两图得到的面内面外上临界场-温度依赖关系. (d) 左: EuO/KTO样品I-V曲线; 中: 对数坐标下EuO/KTO样品I-V曲线; 右: 在零电阻温度Tc0以下, I-V在临界电流附近出现的回滞. (e)电流沿不同方向的Rs-T依赖关系
Figure 8. EuO/KTO (111) heterostructure superconductivity[49]. (a) Rs-T curves of several samples on the KTO (111) substrate, different materials and carrier concentrations show superconductivity. (b) STEM diagrams of EuO (upper) and LAO (lower) are grown on the KTO (111) substrate. The area shown by the green line is near the interface. (c) Left: the Rs-T dependence of EuO/KTO samples under a magnetic field perpendicular to the interface; Middle: the Rs-T dependence of EuO/KTO samples under the magnetic field parallel to the interface; Right: the in-plane and out-of-plane critical field-temperature dependence obtained from the first two figures. (d) Left: EuO/KTO sample I-V curve; Middle: I-V curve of EuO/KTO sample in logarithmic coordinates; Right: below zero resistance temperature Tc0, I-V hysteresis occurs near the critical current. (e) Rs-T dependence of current in different directions.
图 9 LAO/KTO(110) 异质界面超导[50] (a) 不同厚度样品低温下的Rsheet-T依赖关系; (b) 指数坐标下的V-I曲线, 黑色长直线对应V
$ \sim $ I 3依赖关系, 得到0.87 K < TBKT < 0.88 K; (c) 对于20 nm厚度的样品施加垂直于界面磁场的Rsheet-T依赖关系; (d) 对于20 nm厚度的样品施加平行于界面磁场的Rsheet-T依赖关系Figure 9. LAO/KTO (110) heterostructure superconductivity[50]: (a) Rsheet-T dependence of samples with different thickness at low temperature; (b) black long line of the V-I curve in exponential coordinates corresponds to the dependence of V
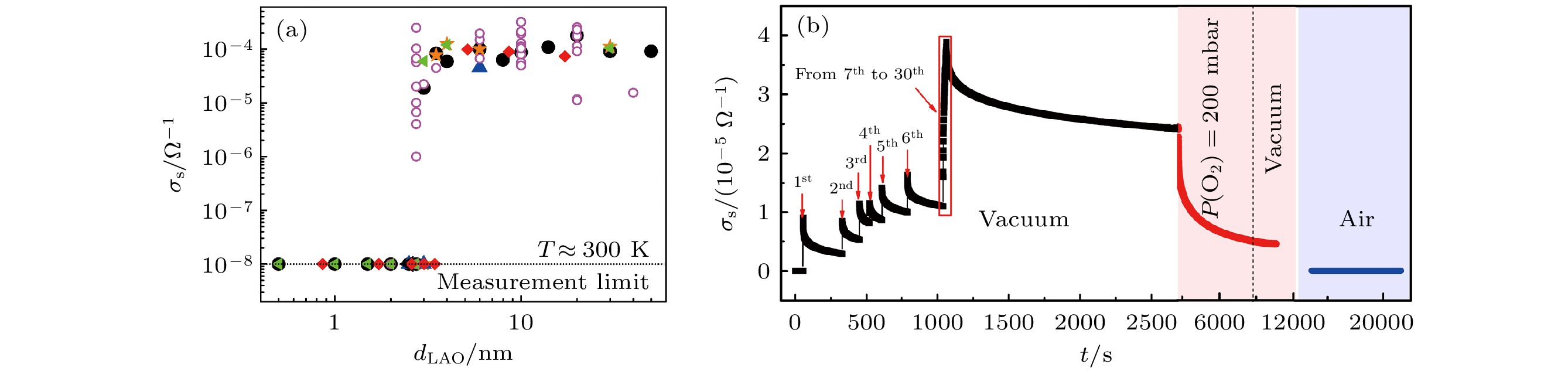
$ \sim $ I 3, and 0.87 K < TBKT < 0.88 K can be obtained; (c) Rsheet-T dependence of the magnetic field perpendicular to the interface is applied to the sample with a thickness of 20 nm; (d) Rsheet-T dependence of the magnetic field parallel to the interface is applied to the sample with a thickness of 20 nm.
图 10 LAO/KTO(111)异质界面电导率与临界厚度[51] (a) LAO/KTO(111)异质界面中LAO厚度与界面电导率关系图, 数据是在室温下(约300 K)测量得到; (b) 室温下对1 nm厚度的样品上沉积LAO的原位输运测试结果, 红色箭头指示激光脉冲数
Figure 10. Conductivity and critical thickness of LAO/KTO (111) heterointerface[51]: (a) The relationship between LAO thickness and interface conductivity in LAO/KTO (111) heterointerface is measured at room temperature (
$ \sim $ 300 K); (b) in situ transport test results of LAO deposited on samples with thickness of 1 nm at room temperature. The number of laser pulses is indicated by the red arrow.
图 11 电场调控的LAO/KTO(111)异质界面超导 (a) 不同调控电压下LAO/KTO(111)异质界面的电阻-温度依赖关系[52]; (b) 不同直流电流驱动下, Tc与调控电压关系图[52]; (c) 调控电压与载流子浓度和迁移率关系图[52]. (d) EuO/KTO(111)异质界面Tc与载流子浓度关系图[54]; (e) 电场调控下EuO/KTO(111) Tc与载流子浓度关系图, 插图为载流子浓度与调控电压关系曲线[54]
Figure 11. LAO/KTO (111) heterostructure superconductivity regulated by electric field. (a) Resistance-temperature dependence of LAO/KTO (111) heterostructure at different control voltages[52]; (b) the relationship between Tc and gate voltage under different DC current drives[52]; (c) diagram of the relationship between gate voltage and carrier concentration and mobility[52]. (d) EuO/KTO (111) heterointerface Tc versus carrier concentration[54]; (e) the relationship between EuO/KTO (111) Tc and carrier concentration under the control of electric field; the illustration shows relation curve between carrier concentration and regulated voltage[54].
图 12 (BCO)2/(CCO)m超晶格超导 (a) (BCO)2/(CCO)m超晶格中CCO层数m与Tc依赖关系, 虚线为m > 3时单个CuO2上有效载流子掺杂减少导致的Tc降低[63]. (b) CCO-STO超晶格不同生长条件下的温度-电阻依赖关系. 点划线表示低氧压生长; 点线表示低氧压生长, 高氧压淬火; 红线表示高氧压生长, 高氧压淬火; 插图为超导转变区间放大图[64]. (c) (CCO)n/(STO)2超晶格中Tcm与CCO厚度依赖关系, 其中Tcm为电阻转变的中点. 插图为不同CCO厚度的(CCO)n/(STO)2超晶格归一化的电阻-温度依赖关系[64]
Figure 12. (BCO)2/(CCO)m superlattice superconductivity. (a) The number of CCO layers m in the (BCO)2/(CCO)m superlattice is dependent on Tc. When the dotted line is m > 3, the effective carrier doping on a single CuO2 decreases, resulting in the decrease of Tc[63]. (b) The temperature-resistance dependence of CCO-STO superlattice under different growth conditions. Dash line shows low oxygen pressure growth; dot line shows low oxygen pressure growth, high oxygen pressure quenching; red line shows high oxygen pressure growth, high oxygen pressure quenching. Illustration shows enlarged view of superconducting transition region[64]. (c) Tcm in (CCO)n/(STO)2 superlattice depends on the thickness of CCO, where Tcm defined as the midpoint of the resistive transitions. Illustration shows normalized resistance-temperature dependence of (CCO)n/(STO)2 superlattices with different CCO thicknesses[64].
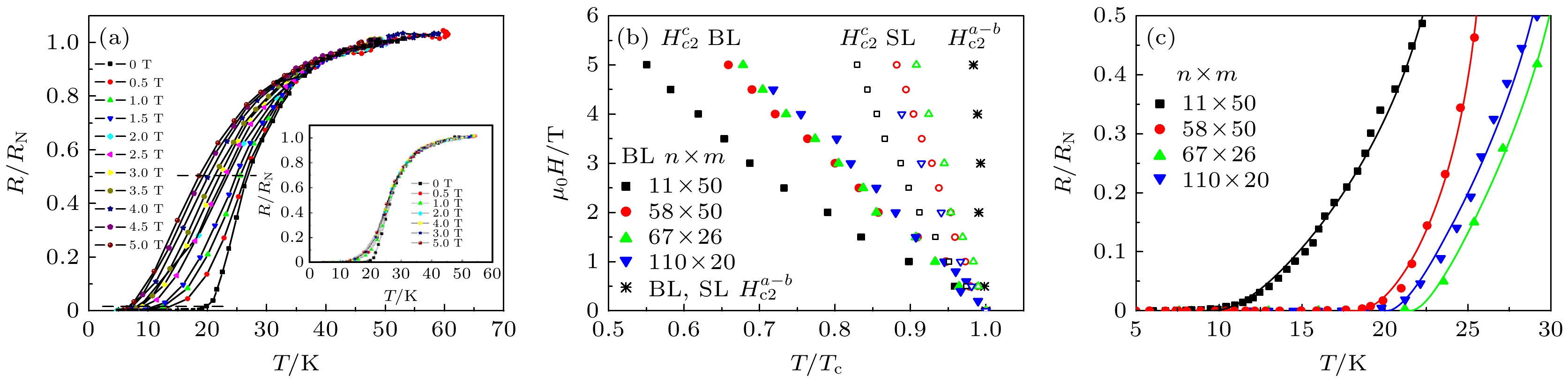
图 13 (CCO)n/(STO)m异质界面输运测试[66] (a)对于n = 58, m = 50的(CCO)n/(STO)m样品施加垂直于界面方向磁场的归一化电阻-温度依赖关系, 插图为施加平行于界面方向磁场的归一化电阻-温度依赖关系. (b)所有样品垂直(闭合符号)和平行(星号)界面的上临界场-温度依赖关系. (c)不同构成的样品的归一化电阻-温度依赖关系, 实线是拟合BKT相变表达式:
$R\left(T\right)= $ $ {R}_{{\rm{N}}}{{\rm{e}}}^{-A/\sqrt{X\left(T\right)-1}}$ , 其中RN为60 K时异质界面的电阻, X(T) = (Tc–TBKT)T/(Tc–T)TBKT, A和TBKT为拟合参量Figure 13. (CCO)n/(STO)m heterointerface transport test[66]. (a) For (CCO)n/(STO)m samples with n = 58 and m = 50, the normalized resistance-temperature dependence of the magnetic field perpendicular to the interface direction is applied. Illustration shows the normalized resistance-temperature dependence of magnetic field applied parallel to the interface direction. (b) The upper critical field-temperature dependence of the vertical (closed symbol) and parallel (asterisk) interfaces of all samples. (c) The normalized resistance-temperature dependence of samples with different compositions, the solid line is the fitting BKT phase transformation expression:
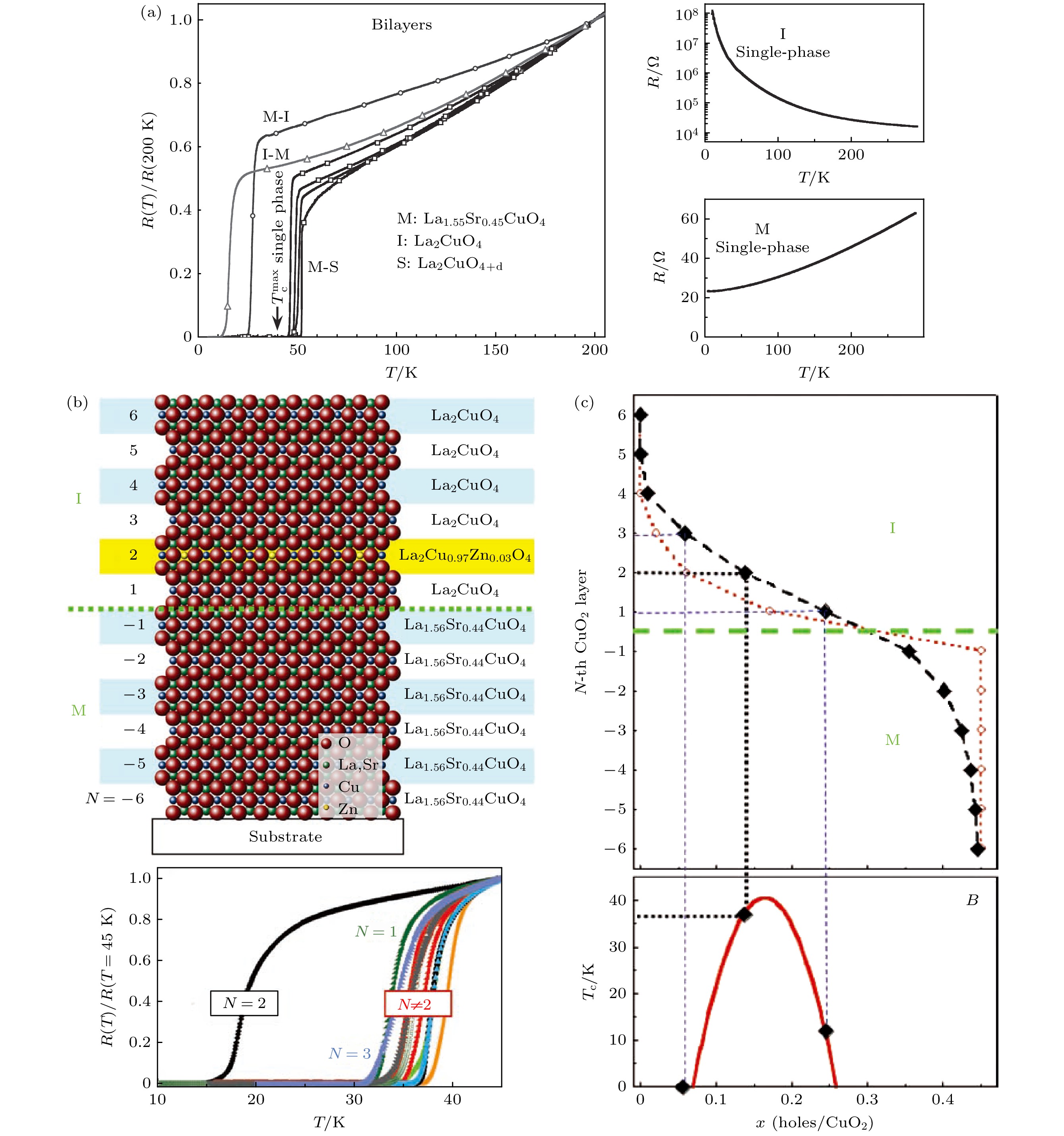
$R\left(T\right)={R}_{{\rm{N}}}{\rm e}^{-A/\sqrt{X\left(T\right)-1}}$ Where RN is the resistance of the heterointerface at 60 K, X(T) = (Tc–TBKT)T/(Tc–T)TBKT, A and TBKT are the fitting parameters
图 14 LCO-LSCO异质界面超导 (a) LCO与La1.56Sr0.44CuO4的电阻-温度依赖关系以及不同异质界面构成的归一化电阻-温度依赖关系. 对于I-M异质界面, Tc
$ \sim $ 15 K; M-I异质界面, Tc$ \sim $ 30 K; M-S异质界面, Tc$ \sim $ 50 K[9]. (b)上: 对于6 uc厚的M-S异质界面进行δ-掺杂Zn原子示意图. 下: δ-掺杂Zn原子不同掺杂位置对超导转变的影响, 超导转变温度只有掺杂Zn在第2层时会发生急剧减小. (c)上: 在6 uc厚度的M-I异质界面中不同位置Sr原子浓度(空心圆)和载流子浓度(实心方块). 下: LCO超导相图, 可以看出第2层的载流子浓度在异质界面中对应的Tc 最高[69]Figure 14. LCO-LSCO heterostructure superconductivity. (a) The resistance-temperature dependence of LCO and La1.56Sr0.44CuO4 and the normalized resistance-temperature dependence of different heterointerface. For I-M heterointerface, Tc
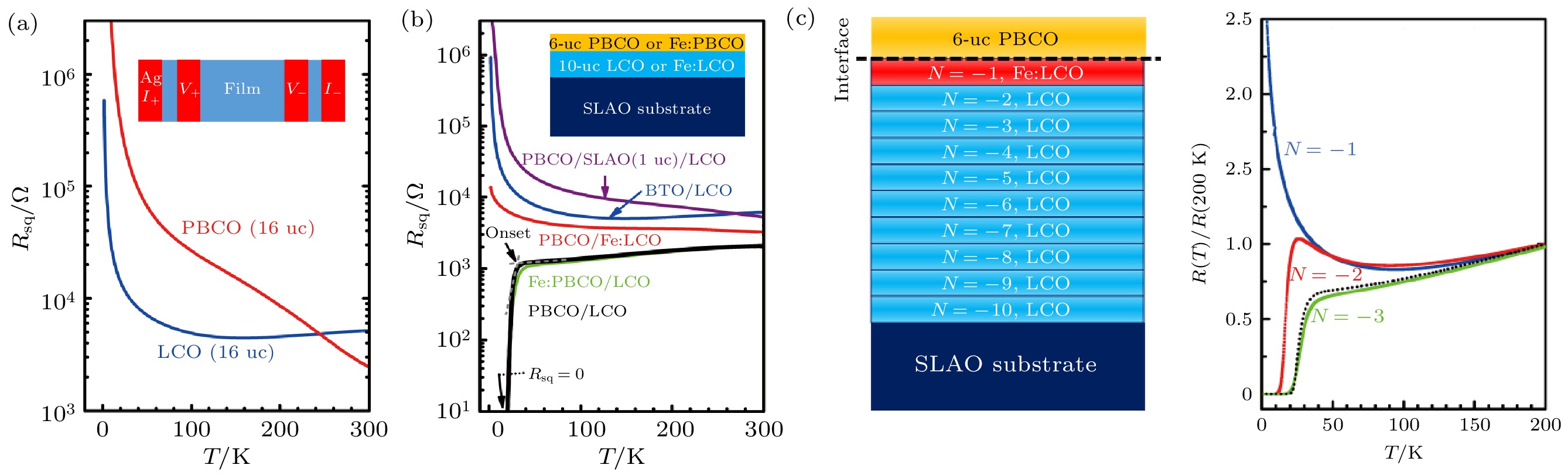
$ \sim $ 15 K, M-I heterointerface, Tc$ \sim $ 30 K, M-S heterointerface, Tc$ \sim $ 50 K[9]. (b) For the M-S heterointerface with a thickness of 6 uc, the schematic diagram of δ-doped Zn atoms (above). The effect of different δ-doping positions of doped Zn atoms on the superconductivity transition (below). The superconductivity transition temperature will decrease sharply only when doped Zn is in the second layer. (c) Above: Sr atom concentration (hollow circle) and carrier concentration (solid block) at different positions in the M-I heterostructure with a thickness of 6 uc. Below: LCO superconducting phase diagram, it can be seen that the carrier concentration of the second layer corresponds to the highest Tc in the heterointerface[69].
图 15 LCO/PBCO界面超导[71] (a)单一组分的LCO与PBCO的电阻-温度依赖关系. (b)不同构成异质界面的电阻-温度依赖关系, 分别为PBCO/LCO异质界面、PBCO掺杂Fe的Fe∶PBCO/LCO异质界面、LCO掺杂Fe的PBCO/Fe∶LCO异质界面以及PBCO/SLAO/LCO异质界面和BTO/LCO异质界面. (c)左: Fe掺杂LCO/PBCO异质界面中不同位置的LCO示意图. 右: Fe掺杂不同位置LCO后异质界面的电阻-温度依赖关系
Figure 15. LCO/PBCO interface superconductivity[71]. (a) The resistance-temperature dependence of single component LCO and PBCO. (b) The resistance-temperature dependence of different components of heterointerface are PBCO/LCO heterointerface, PBCO doped Fe∶PBCO/LCO heterointerface LCO doped Fe PBCO/Fe∶LCO heterointerface, PBCO/SLAO/LCO heterointerface and BTO/LCO heterointerface. (c) Left: Schematic diagram of LCO at different positions in Fe-doped LCO/PBCO heterointerface. Right: The resistance-temperature dependence of the heterointerface after Fe doping with LCO at different positions.
-
[1] Onnes H K 1911 Comm. Phys. Lab. Univ. Leiden 120b 122
[2] Bardeen J, Cooper L N, Schrieffer J R 1957 Phys. Rev. 106 162
Google Scholar
[3] Bednorz J G, Müller K A 1986 Z. Phys. B:Condens. Matter 64 189
Google Scholar
[4] Kamihara Y, Watanabe T, Hirano M, Hosono H 2008 J. Am. Chem. Soc. 130 3296
Google Scholar
[5] McMillan W L 1968 Phys. Rev. 167 331
Google Scholar
[6] Strongin M, Kammerer O F, Crow J E, Parks R D, Douglass D H, Jensen M A 1968 Phys. Rev. Lett. 21 1320
[7] Kroemer H 2001 Rev. Mod. Phys. 73 783
Google Scholar
[8] Reyren N, Thiel S, Caviglia A D, Kourkoutis L F, Hammerl G, Richter C, Schneider C W, Kopp T, Rüetschi A S, Jaccard D, Gabay M, Muller D A, Triscone J M, Mannhart J 2007 Science 317 1196
Google Scholar
[9] Gozar A, Logvenov G, Kourkoutis L F, Bollinger A T, Giannuzzi L A, Muller D A, Bozovic I 2008 Nature 455 782
Google Scholar
[10] Bozovic I 2001 IEEE Trans. Appl. Supercond. 11 2686
Google Scholar
[11] Wang Q Y, Li Z, Zhang W H, Zhang Z C, Zhang J S, Li W, Ding H, Ou Y B, Deng P, Chang K, Wen J, Song C L, He K, Jia J F, Ji S H, Wang Y Y, Wang L L, Chen X, Ma X C, Xue Q K 2012 Chin. Phys. Lett. 29 037402
Google Scholar
[12] Saito Y, Nojima T, Iwasa Y 2016 Nat. Rev. Mater. 2 16094
Google Scholar
[13] Uchihashi T 2017 Supercond. Sci. Technol. 30 013002
Google Scholar
[14] Xing Y, Zhang H M, Fu H L, Liu H, Sun Y, Peng J P, Wang F, Lin X, Ma X C, Xue Q K, Wang J, Xie X C 2015 Science 350 542
Google Scholar
[15] Ohtomo A, Muller D A, Grazul J L, Hwang H Y 2002 Nature 419 378
Google Scholar
[16] Ohtomo A, Hwang H Y 2004 Nature 427 423
Google Scholar
[17] Cantoni C, Gazquez J, Miletto Granozio F, Oxley M P, Varela M, Lupini A R, Pennycook S J, Aruta C, di Uccio U S, Perna P, Maccariello D 2012 Adv. Mater. 24 3952
Google Scholar
[18] Cen C, Thiel S, Hammerl G, Schneider C W, Andersen K E, Hellberg C S, Mannhart J, Levy J 2008 Nat. Mater. 7 298
Google Scholar
[19] Nakagawa N, Hwang H Y, Muller D A 2006 Nat. Mater. 5 204
Google Scholar
[20] Lee H, Campbell N, Lee J, Asel T J, Paudel T R, Zhou H, Lee J W, Noesges B, Seo J, Park B, Brillson L J, Oh S H, Tsymbal E Y, Rzchowski M S, Eom C B 2018 Nat. Mater. 17 231
Google Scholar
[21] Chen Y Z, Bovet N, Trier F, Christensen D V, Qu F M, Andersen N H, Kasama T, Zhang W, Giraud R, Dufouleur J, Jespersen T S, Sun J R, Smith A, Nygård J, Lu L, Büchner B, Shen B G, Linderoth S, Pryds N 2013 Nat. Commun. 4 1371
Google Scholar
[22] Lee S W, Liu Y, Heo J, Gordon R G 2012 Nano Lett. 12 4775
Google Scholar
[23] Liu Z Q, Li C J, Lü W M, Huang X H, Huang Z, Zeng S W, Qiu X P, Huang L S, Annadi A, Chen J S, Coey J M D, Venkatesan T, Ariando 2013 Phys. Rev. X 3 021010
[24] Zhang M, Chen Z, Mao B, Li Q, Bo H, Ren T, He P, Liu Z, Xie Y 2018 Phys. Rev. Mater. 2 065002
Google Scholar
[25] Chen Z, Chen X, Mao B, Li Q, Zhang M, Bo H, Liu Z, Tian H, Zhang Z, Xie Y 2018 Adv. Mater. Interfaces 5 1801216
Google Scholar
[26] Chen Z, Zhang M, Ren T, Xie Y 2019 J. Phys. Condens. Matter 31 505002
Google Scholar
[27] Yu L, Zunger A 2014 Nat. Commun. 5 5118
Google Scholar
[28] Caviglia A D, Gariglio S, Reyren N, Jaccard D, Schneider T, Gabay M, Thiel S, Hammerl G, Mannhart J, Triscone J M 2008 Nature 456 624
Google Scholar
[29] Parendo K A, Tan K H S B, Bhattacharya A, Eblen Zayas M, Staley N E, Goldman A M 2005 Phys. Rev. Lett. 94 197004
Google Scholar
[30] Aubin H, Marrache Kikuchi C A, Pourret A, Behnia K, Bergé L, Dumoulin L, Lesueur J 2006 Phys. Rev. B 73 094521
Google Scholar
[31] Sachdev S 2011 Quantum Phase Transitions (2nd Ed.) (Cambridge: Cambridge University Press) pp293–331
[32] Biscaras J, Bergeal N, Kushwaha A, Wolf T, Rastogi A, Budhani R C, Lesueur J 2010 Nat. Commun. 1 89
Google Scholar
[33] Biscaras J, Bergeal N, Hurand S, Feuillet-Palma C, Rastogi A, Budhani R C, Grilli M, Caprara S, Lesueur J 2013 Nat. Mater. 12 542
Google Scholar
[34] Herranz G, Singh G, Bergeal N, Jouan A, Lesueur J, Gázquez J, Varela M, Scigaj M, Dix N, Sánchez F, Fontcuberta J 2015 Nat. Commun. 6 6028
Google Scholar
[35] Monteiro A M R V L, Groenendijk D J, Groen I, de Bruijckere J, Gaudenzi R, van der Zant H S J, Caviglia A D 2017 Phys. Rev. B 96 020504
Google Scholar
[36] Caviglia A D, Gabay M, Gariglio S, Reyren N, Cancellieri C, Triscone J M 2010 Phys. Rev. Lett. 104 126803
Google Scholar
[37] Rout P K, Maniv E, Dagan Y 2017 Phys. Rev. Lett. 119 237002
Google Scholar
[38] Zhong Z, Tóth A, Held K 2013 Phys. Rev. B 87 161102
Google Scholar
[39] Michaeli K, Potter A C, Lee P A 2012 Phys. Rev. Lett. 108 117003
Google Scholar
[40] Abrahams E, Anderson P W, Licciardello D C, Ramakrishnan T V 1979 Phys. Rev. Lett. 42 673
Google Scholar
[41] Jaeger H M, Haviland D B, Orr B G, Goldman A M 1989 Phys. Rev. B 40 182
Google Scholar
[42] Bøttcher C G L, Nichele F, Kjaergaard M, Suominen H J, Shabani J, Palmstrøm C J, Marcus C M 2018 Nat. Phys. 14 1138
Google Scholar
[43] Chakravarty S, Yin L, Abrahams E 1998 Phys. Rev. B 58 R559
Google Scholar
[44] Phillips P, Dalidovich D 2003 Science 302 243
Google Scholar
[45] Spivak B, Kravchenko S V, Kivelson S A, Gao X P A 2010 Rev. Mod. Phys. 82 1743
Google Scholar
[46] Chen Z, Swartz A G, Yoon H, Inoue H, Merz T A, Lu D, Xie Y, Yuan H, Hikita Y, Raghu S, Hwang H Y 2018 Nat. Commun. 9 4008
Google Scholar
[47] Richter C, Boschker H, Dietsche W, Fillis Tsirakis E, Jany R, Loder F, Kourkoutis L F, Muller D A, Kirtley J R, Schneider C W, Mannhart J 2013 Nature 502 528
Google Scholar
[48] Zhang H, Yun Y, Zhang X, Zhang H, Ma Y, Yan X, Wang F, Li G, Li R, Khan T, Chen Y, Liu W, Hu F, Liu B, Shen B, Han W, Sun J 2018 Phys. Rev. Lett. 121 116803
Google Scholar
[49] Liu C, Yan X, Jin D, Ma Y, Hsiao H W, Lin Y, Bretz Sullivan T M, Zhou X, Pearson J, Fisher B, Jiang J S, Han W, Zuo J M, Wen J, Fong D D, Sun J, Zhou H, Bhattacharya A 2021 Science 371 716
Google Scholar
[50] Chen Z, Liu Z, Sun Y, Chen X, Liu Y, Zhang H, Li H, Zhang M, Hong S, Ren T, Zhang C, Tian H, Zhou Y, Sun J, Xie Y 2021 Phys. Rev. Lett. 126 026802
Google Scholar
[51] Sun Y, Liu Y, Hong S, Chen Z, Zhang M, Xie Y 2021 Phys. Rev. Lett. 127 086804
Google Scholar
[52] Chen Z, Liu Y, Zhang H, Liu Z, Tian H, Sun Y, Zhang M, Zhou Y, Sun J, Xie Y 2021 Science 372 721
Google Scholar
[53] Christiansen C, Hernandez L M, Goldman A M 2002 Phys. Rev. Lett. 88 037004
Google Scholar
[54] Liu C, Zhou X, Hong D, Fisher B, Zheng H, Pearson J, Jiang J S, Jin D, Norman M R, Bhattacharya A 2023 Nat. Commun. 14 951
Google Scholar
[55] Schooley J F, Hosler W R, Cohen M L 1964 Phys. Rev. Lett. 12 474
Google Scholar
[56] Ueno K, Nakamura S, Shimotani H, Ohtomo A, Kimura N, Nojima T, Aoki H, Iwasa Y, Kawasaki M 2008 Nat. Mater. 7 855
Google Scholar
[57] Ueno K, Nakamura S, Shimotani H, Yuan H T, Kimura N, Nojima T, Aoki H, Iwasa Y, Kawasaki M 2011 Nat. Nanotech. 6 408
Google Scholar
[58] Ren T, Li M, Sun X, Ju L, Liu Y, Hong S, Sun Y, Tao Q, Zhou Y, Xu Z A, Xie Y 2022 Sci. Adv. 8 eabn4273
Google Scholar
[59] Imada M, Fujimori A, Tokura Y 1998 Rev. Mod. Phys. 70 1039
Google Scholar
[60] Di Stasio M, Müller K A, Pietronero L 1990 Phys. Rev. Lett. 64 2827
Google Scholar
[61] Shen Z X, Dessau D S, Wells B O, King D M, Spicer W E, Arko A J, Marshall D, Lombardo L W, Kapitulnik A, Dickinson P, Doniach S, DiCarlo J, Loeser T, Park C H 1993 Phys. Rev. Lett. 70 1553
Google Scholar
[62] Norton D P, Chakoumakos B C, Budai J D, Lowndes D H, Sales B C, Thompson J R, Christen D K 1994 Science 265 2074
Google Scholar
[63] Balestrino G, Martellucci S, Medaglia P G, Paoletti A, Petrocelli G, Varlamov A A 1998 Phys. Rev. B 58 R8925
Google Scholar
[64] Di Castro D, Salvato M, Tebano A, Innocenti D, Aruta C, Prellier W, Lebedev O I, Ottaviani I, Brookes N B, Minola M, Moretti Sala M, Mazzoli C, Medaglia P G, Ghiringhelli G, Braicovich L, Cirillo M, Balestrino G 2012 Phys. Rev. B 86 134524
Google Scholar
[65] Aruta C, Schlueter C, Lee T L, Di Castro D, Innocenti D, Tebano A, Zegenhagen J, Balestrino G 2013 Phys. Rev. B 87 155145
Google Scholar
[66] Salvato M, Tieri G, Balestrino G, Di Castro D 2015 Supercond. Sci. Technol. 28 095012
Google Scholar
[67] Salvato M, Ottaviani I, Lucci M, Cirillo M, Di Castro D, Innocenti D, Tebano A, Balestrino G 2013 J. Phys. Condens. Matter 25 335702
Google Scholar
[68] Kitazawa K, Sakai M, Uchida S i, Takagi H, Kishio K, Kanbe S, Tanaka S, Fueki K 1987 Jpn. J. Appl. Phys. 26 L342
Google Scholar
[69] Logvenov G, Gozar A, Bozovic I 2009 Science 326 699
Google Scholar
[70] Smadici S, Lee J C T, Wang S, Abbamonte P, Logvenov G, Gozar A, Cavellin C D, Bozovic I 2009 Phys. Rev. Lett. 102 107004
Google Scholar
[71] Ju L, Ren T, Li Z, Liu Z, Shi C, Liu Y, Hong S, Wu J, Tian H, Zhou Y, Xie Y 2022 Phys. Rev. B 105 024516
Google Scholar
[72] Bert J A, Kalisky B, Bell C, Kim M, Hikita Y, Hwang H Y, Moler K A 2011 Nat. Phys. 7 767
Google Scholar
[73] Li L, Richter C, Mannhart J, Ashoori R C 2011 Nat. Phys. 7 762
Google Scholar
[74] Dikin D A, Mehta M, Bark C W, Folkman C M, Eom C B, Chandrasekhar V 2011 Phys. Rev. Lett. 107 056802
Google Scholar
[75] Nayak C, Simon S H, Stern A, Freedman M, Das Sarma S 2008 Rev. Mod. Phys. 80 1083
Google Scholar
[76] Zhang Y, Gu Y, Li P, Hu J, Jiang K 2022 Phys. Rev. X 12 041013
DownLoad:
Catalog
Metrics
- Abstract views: 6467
- PDF Downloads: 270
- Cited By: 0
