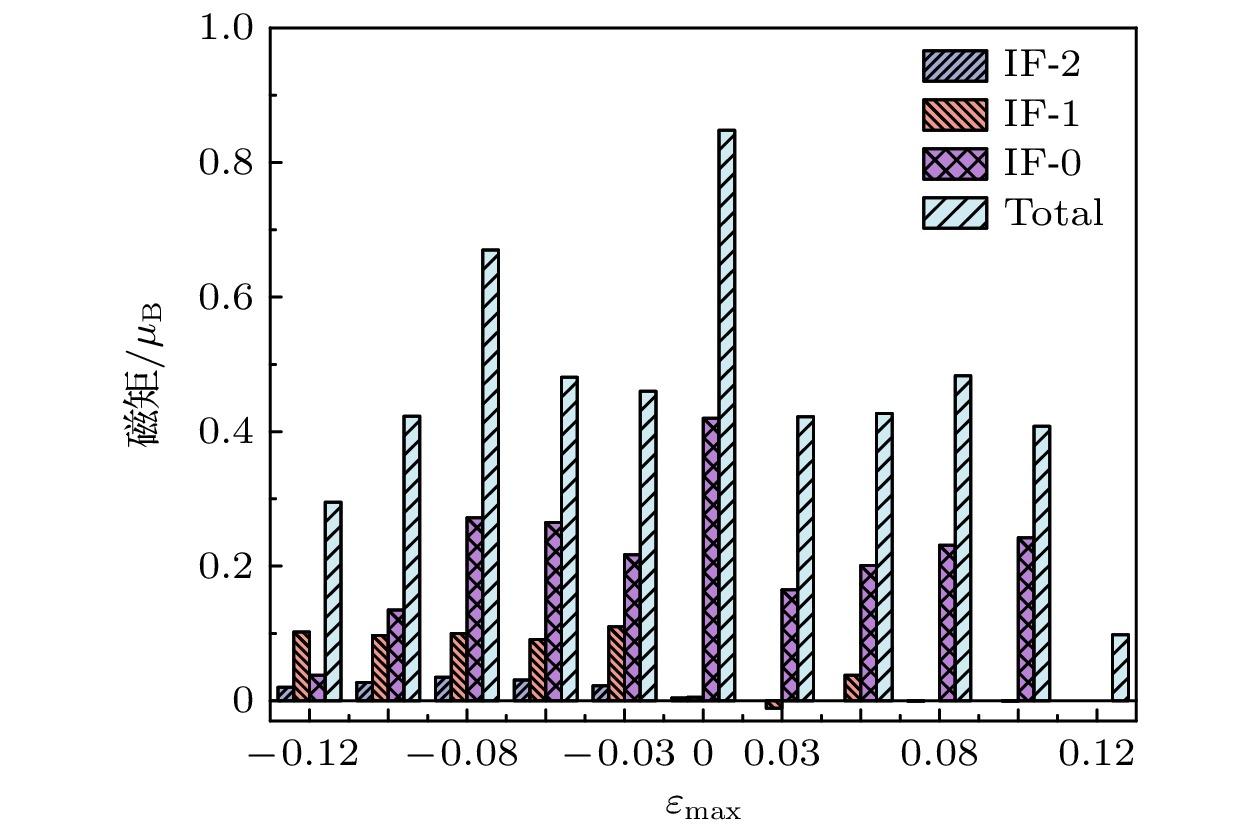
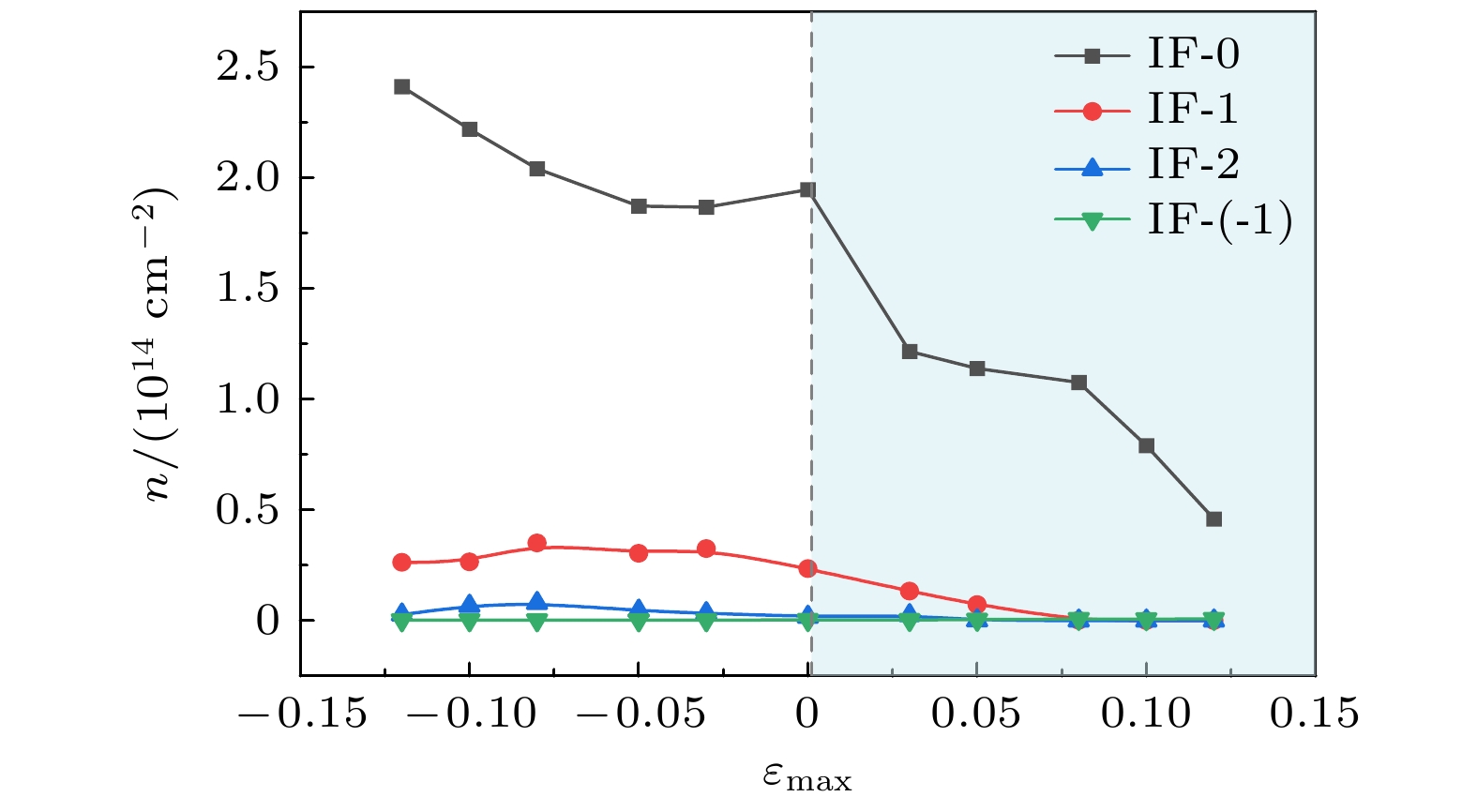
-
钙钛矿超晶格材料界面的独特电子性质, 在纳米器件、新型超敏传感器器件等方面具有广阔的应用前景, 探索其界面独特的电子性质对理解关联电子系统中多自由度耦合和传感器器件的设计等方面具有重要的意义. 本文通过构建LaAlO3/KNbO3超晶格材料, 施加非均匀应变对其界面的载流子浓度等性质进行调控. 计算结果表明, 施加拉伸和压缩的应变梯度都可以调节界面处二维电子气性质. 其中, 当最大压缩应变梯度系数为12%时, 界面处二维电子气浓度减小了76.4%, 并且界面磁矩消失, 材料的总磁矩减小约88.44%, 向无磁性材料转变; 当最大拉伸型应变梯度系数为12%时, 界面处电子气浓度增大约23.9%, 界面磁矩明显减小, 而且界面临近层出现明显的磁矩. 该理论计算结果表明, 应变梯度是一种新的钙钛矿界面载流子的有效调控手段, 对LaAlO3/KNbO3钙钛矿超晶格材料界面电子性质的研究提升了对此类氧化物界面电子特性的认识, 为探索调控氧化物界面处的高性能自旋极化载流子气开辟了新的途径.The superlattices composed of polar/polar perovskites have two-dimensional electron gas (2DEG) at the interface, which has broad applications in nano devices, super sensitive sensor devices, high electron mobility transistor, etc. Tuning the electronic properties of the 2DEG at the interface perovskite superlattice, such as the coupling between strain gradient and the electronic properties of the 2DEG in correlated electronic systems, is of great significance. In this paper, the properties of (LaAlO3)4.5/(KNbO3)8.5 superlattice, which is composed of KNbO3 and LaAlO3, are systematically investigated through first-principles density functional theory calculations. The band structure of (LaAlO3)4.5/(KNbO3)8.5 superlattice exhibits 2DEG at the interface, which is consistent with the result in the literature. The band structure, density of states, magnetic moments and carrier concentration at the interface are fully investigated by using compressive gradient and tensile strain gradient, respectively. The results show that compressive strain gradient can effectively reduce the 2DEG concentration at the interface. When the compressive strain gradient coefficient reaches 12%, the 2DEG concentration decreases by 76.4%, and the interface magnetic moment disappears. The total magnetic moment of the superlattice decreases by 88.44%. When the tensile strain gradient is 12%, the electron gas concentration at the interface is increased by about 23.9%, and the interface magnetic moment is reduced by about 90.7%. At the same time, an obvious magnetic moment appears in the layer near the interface. Hence, the strain gradient can be a new approach to regulating the electron gas concentration at interface of perovskite superlattice. The tensile strain gradient increases the electron gas concentration at the interface, while the compressive strain gradient reduces the electron gas concentration. Therefore, it opens up a new way to exploring the regulation of high-performance spin polarized carrier gas at the oxide interface.
-
Keywords:
- strain gradient /
- two-dimensional electron gas /
- superlattice /
- first principles
[1] Tra V T, Chen J W, Huang P C, et al. 2013 Adv. Mater. 25 3357
Google Scholar
[2] Thiel S, Hammerl G, Schmehl A, Schneider C W, Mannhart J 2006 Science 313 1942
Google Scholar
[3] Reyren N, Thiel S, Caviglia A D, et al. 2007 Science 317 1196
Google Scholar
[4] Caviglia A D, Gariglio S, Reyren N, et al. 2008 Nature 456 624
Google Scholar
[5] Bi F, Huang M, Ryu S, Lee H, Bark C W, Eom C B, Irvin P, Levy J 2014 Nat. Commun. 5 5019
Google Scholar
[6] 李敏, 时鑫娜, 张泽霖, 吉彦达, 樊济宇, 杨浩 2019 68 087302
Google Scholar
Li M, Shi X N, Zhang Z L, Ji Y D, Fan J Y, Yang H 2019 Acta Phys. Sin. 68 087302
Google Scholar
[7] Bert J A, Kalisky B, Bell C, Kim M, Hikita Y, Hwang H Y, Moler K A 2011 Nat. Phys. 7 767
Google Scholar
[8] 朱立峰, 潘文远, 谢燕, 张波萍, 尹阳, 赵高磊 2019 68 217701
Google Scholar
Zhu L F, Pan W Y, Xie Y, Zhang B P, Yin Y, Zhao G L 2019 Acta Phys. Sin. 68 217701
Google Scholar
[9] Sharma P, Huang Z, Li M, Li C, Hu S, Lee H, Lee J W, Eom C B, Pennycook S J, Seidel J, Ariando, Gruverman A 2018 Adv. Funct. Mater. 28 1707159
Google Scholar
[10] Baikie T, Fang Y, Kadro J M, Schreyer M, Wei F, Mhaisalkar S G, Graetzel M, White T J 2013 J. Mater. Chem. A 1 5628
Google Scholar
[11] Phillips L J, Rashed A M, Treharne R E, et al. 2016 Sol. Energy Mater. Sol. Cells 147 327
Google Scholar
[12] Stranks S D, Eperon G E, Grancini G, et al. 2013 Science 342 341
Google Scholar
[13] Zhao Y, Nardes A M, Zhu K 2014 J. Phys. Chem. Lett. 5 490
Google Scholar
[14] Ponseca C S, Savenije T J, Abdellah M, et al. 2014 J. Am. Chem. Soc. 136 5189
Google Scholar
[15] Brinkman A, Huijben M, Van Zalk M, et al. 2007 Nat. Mater. 6 493
Google Scholar
[16] Kalisky B, Bert J A, Bell C, Xie Y, Sato H K, Hosoda M, Hikita Y, Hwang H Y, Moler K A 2012 Nano Lett. 12 4055
Google Scholar
[17] Dikin D A, Mehta M, Bark C W, Folkman C M, Eom C B, Chandrasekhar V 2011 Phys. Rev. Lett. 107 056802
Google Scholar
[18] Guduru V K, del Aguila A G, Wenderich S, et al. 2013 Appl. Phys. Lett. 102 051604
Google Scholar
[19] Lei Y, Li Y, Chen Y Z, Xie Y W, Chen Y S, Wang S H, Wang J, Shen B G, Pryds N, Hwang H Y, Sun J R 2014 Nat. Commun. 5 5554
Google Scholar
[20] Au K, Li D F, Chan N Y, Dai J Y 2012 Adv. Mater. 24 2598
Google Scholar
[21] Bark C W, Felker D A, Wang Y, et al. 2011 Proc. Natl. Acad. Sci. U.S.A. 108 4720
Google Scholar
[22] Salluzzo M, Gariglio S, Stornaiuolo D, et al. 2013 Phys. Rev. Lett. 111 087204
Google Scholar
[23] Schoofs F, Carpenter M A, Vickers M E, et al. 2013 J. Phys. Condes. Matter 25 175005
Google Scholar
[24] Huang Z, Liu Z Q, Yang M, et al. 2014 Phys. Rev. B 90 125156
Google Scholar
[25] Du Y, Wang C, Li J, Zhang X, Wang F, Zhu Y, Yin N, Mei L 2015 Comput. Mater. Sci. 99 57
Google Scholar
[26] Meevasana W, King P D C, He R H, Mo S K, Hashimoto M, Tamai A, Songsiriritthigul P, Baumberger F, Shen Z X 2011 Nat. Mater. 10 114
Google Scholar
[27] Fang L, Chen C, Yang Y, Wu Y, Hu T, Zhao G, Zhu Q, Ren W 2019 Phys. Chem. Chem. Phys. 21 8046
Google Scholar
[28] Zhang Z, Jiang W, Liu K, Liu M, Meng J, Wu L, Shao T, Ling J, Yao C, Xiong C, Dou R, Nie J 2020 Ann. Phys. Berlin 532 2000155
Google Scholar
[29] Cooper V R 2012 Phys. Rev. B 85 235109
Google Scholar
[30] Pentcheva R, Pickett W E 2008 Phys. Rev. B 78 205106
Google Scholar
[31] Shu L, Wei X, Jin L, Li Y, Wang H, Yao X 2013 Appl. Phys. Lett. 102 152904
Google Scholar
[32] Nystrom M J, Wessels B W, Chen J, Marks T J 1996 Appl. Phys. Lett. 68 761
Google Scholar
-
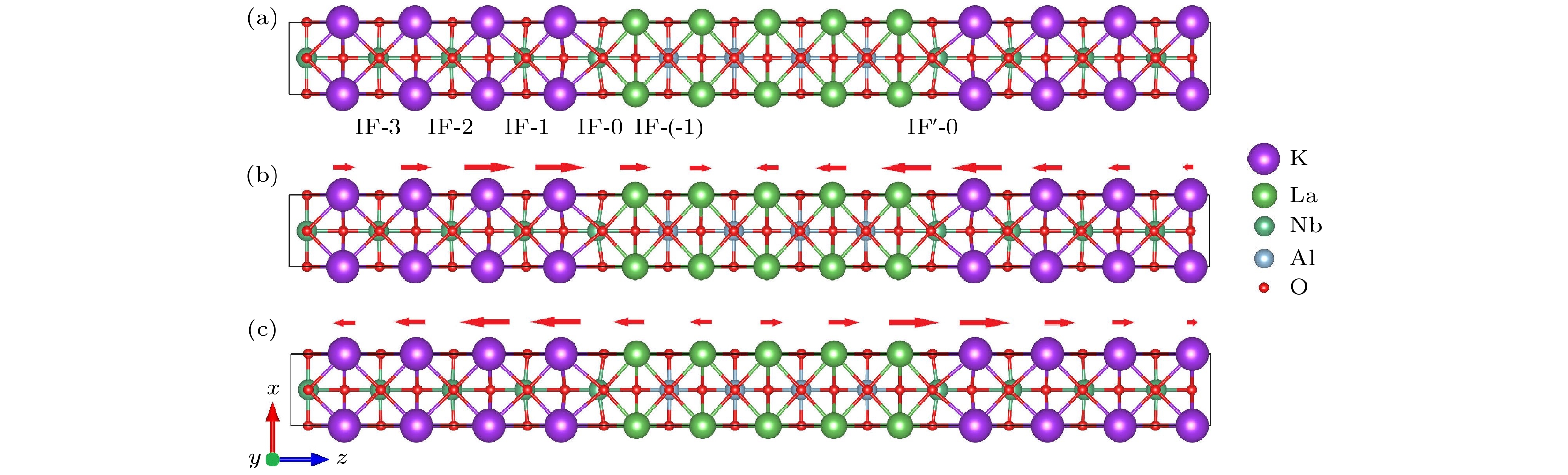
图 1 (a) (LaAlO3)4.5/(KNbO3)8.5晶胞模型; (b)压缩非均匀应变原子偏移示意图; (c)拉伸非均匀应变原子偏移示意图, 箭头大小表示偏离平衡位置的程度
Fig. 1. (a) The (LAO)4.5/(KNO)8.5 superlattice model; (b) schematic diagram of atomic shift in compressed non-uniform strain; (c) schematic diagram of atomic migration under non-uniform tensile strain. Arrow size indicates the degree of deviation from the balance position.
图 2 LAO/KNO的界面附近各层的投影态密度及界面附近的电荷差分密度图
Fig. 2. The layer resolved DOS LAO/KNO with the Fermi level is set to zero, and the charge difference density diagram near the interface.
图 3 不同最大压缩应变梯度系数下NbO2/LaO界面层及其附近的态密度 (a)
$\varepsilon_{\max} $ = 0%; (b)$\varepsilon_{\max} $ = 3%; (c)$\varepsilon_{\max} $ = 5%; (d)$\varepsilon_{\max} $ = 8%; (e)$\varepsilon_{\max} $ = 10%; (f)$\varepsilon_{\max} $ = 12%Fig. 3. Density of state of NbO2/LaO interface layer and its adjacent layer under different maximum compressive strain gradients coefficient: (a)
$\varepsilon_{\max} $ = 0%; (b)$\varepsilon_{\max} $ = 3%; (c)$\varepsilon_{\max} $ = 5%; (d)$\varepsilon_{\max} $ = 8%; (e)$\varepsilon_{\max} $ = 10%; (f)$\varepsilon_{\max} $ = 12%.
图 4 不同最大压缩应变梯度系数下LAO/KNO的能带图及界面Nb元素dxy的轨道投影能带 (a)
$\varepsilon_{\max} $ = 0%; (b)$\varepsilon_{\max} $ = 3%; (c)$\varepsilon_{\max} $ = 5%; (d)$\varepsilon_{\max} $ = 8%; (e)$\varepsilon_{\max} $ = 10%; (f)$\varepsilon_{\max} $ = 12%Fig. 4. The band structures of LAO/KNO and projected band structures of interface with orbit of Nb element dxy under different maximum compressive strain gradient coefficient: (a)
$\varepsilon_{\max} $ = 0%; (b)$ \varepsilon_{\max}$ = 3%; (c)$\varepsilon_{\max} $ = 5%; (d)$\varepsilon_{\max} $ = 8%; (e)$\varepsilon_{\max} $ = 10%; (f)$\varepsilon_{\max} $ = 12%.
图 5 不同最大拉伸应变梯度系数下NbO2/LaO界面层及其附近的态密度 (a)
$\varepsilon_{\max} $ = 0%; (b)$\varepsilon_{\max} $ =3%; (c)$\varepsilon_{\max} $ = 5%; (d)$\varepsilon_{\max} $ = 8%; (e)$\varepsilon_{\max} $ = 10%; (f)$\varepsilon_{\max} $ =12%Fig. 5. Density of state of NbO2/LaO interface layer and its adjacent layer under different maximum tensile strain gradient coefficient: (a)
$\varepsilon_{\max} $ = 0%; (b)$\varepsilon_{\max} $ = 3%; (c)$\varepsilon_{\max} $ = 5%; (d)$\varepsilon_{\max} $ = 8%; (e)$\varepsilon_{\max} $ = 10%; (f)$\varepsilon_{\max} $ = 12%.
图 6 不同最大拉伸应变梯度系数下LAO/KNO的能带图及界面Nb元素的dxy轨道的投影能带 (a)
$\varepsilon_{\max} $ = 3%; (b)$\varepsilon_{\max} $ = 5%; (c)$\varepsilon_{\max} $ = 8%; (d)$\varepsilon_{\max} $ = 10%Fig. 6. The band structures of LAO/KNO and projected band structures of interface with orbit of Nb element dxy under different maximum tensile strain gradient coefficient: (a)
$\varepsilon_{\max} $ = 3 %; (b)$\varepsilon_{\max} $ = 5%; (c)$\varepsilon_{\max} $ = 8%; (d)$\varepsilon_{\max} $ = 10%.
图 7 施加拉伸和压缩应变梯度时不同界面的磁矩和体系总磁矩
Fig. 7. The change of interface magnetic moment with the strength of strain gradient when the compressive and tensile strain gradients are applied.
图 8 施加压缩(深色区域)和拉伸应变梯度时, 界面二维电子气密度随应变梯度强度的变化
Fig. 8. The 2 DEG density of the interface changes with the strength of the strain gradient when the compressive (shadow region) and tensile strain gradients are applied.
-
[1] Tra V T, Chen J W, Huang P C, et al. 2013 Adv. Mater. 25 3357
Google Scholar
[2] Thiel S, Hammerl G, Schmehl A, Schneider C W, Mannhart J 2006 Science 313 1942
Google Scholar
[3] Reyren N, Thiel S, Caviglia A D, et al. 2007 Science 317 1196
Google Scholar
[4] Caviglia A D, Gariglio S, Reyren N, et al. 2008 Nature 456 624
Google Scholar
[5] Bi F, Huang M, Ryu S, Lee H, Bark C W, Eom C B, Irvin P, Levy J 2014 Nat. Commun. 5 5019
Google Scholar
[6] 李敏, 时鑫娜, 张泽霖, 吉彦达, 樊济宇, 杨浩 2019 68 087302
Google Scholar
Li M, Shi X N, Zhang Z L, Ji Y D, Fan J Y, Yang H 2019 Acta Phys. Sin. 68 087302
Google Scholar
[7] Bert J A, Kalisky B, Bell C, Kim M, Hikita Y, Hwang H Y, Moler K A 2011 Nat. Phys. 7 767
Google Scholar
[8] 朱立峰, 潘文远, 谢燕, 张波萍, 尹阳, 赵高磊 2019 68 217701
Google Scholar
Zhu L F, Pan W Y, Xie Y, Zhang B P, Yin Y, Zhao G L 2019 Acta Phys. Sin. 68 217701
Google Scholar
[9] Sharma P, Huang Z, Li M, Li C, Hu S, Lee H, Lee J W, Eom C B, Pennycook S J, Seidel J, Ariando, Gruverman A 2018 Adv. Funct. Mater. 28 1707159
Google Scholar
[10] Baikie T, Fang Y, Kadro J M, Schreyer M, Wei F, Mhaisalkar S G, Graetzel M, White T J 2013 J. Mater. Chem. A 1 5628
Google Scholar
[11] Phillips L J, Rashed A M, Treharne R E, et al. 2016 Sol. Energy Mater. Sol. Cells 147 327
Google Scholar
[12] Stranks S D, Eperon G E, Grancini G, et al. 2013 Science 342 341
Google Scholar
[13] Zhao Y, Nardes A M, Zhu K 2014 J. Phys. Chem. Lett. 5 490
Google Scholar
[14] Ponseca C S, Savenije T J, Abdellah M, et al. 2014 J. Am. Chem. Soc. 136 5189
Google Scholar
[15] Brinkman A, Huijben M, Van Zalk M, et al. 2007 Nat. Mater. 6 493
Google Scholar
[16] Kalisky B, Bert J A, Bell C, Xie Y, Sato H K, Hosoda M, Hikita Y, Hwang H Y, Moler K A 2012 Nano Lett. 12 4055
Google Scholar
[17] Dikin D A, Mehta M, Bark C W, Folkman C M, Eom C B, Chandrasekhar V 2011 Phys. Rev. Lett. 107 056802
Google Scholar
[18] Guduru V K, del Aguila A G, Wenderich S, et al. 2013 Appl. Phys. Lett. 102 051604
Google Scholar
[19] Lei Y, Li Y, Chen Y Z, Xie Y W, Chen Y S, Wang S H, Wang J, Shen B G, Pryds N, Hwang H Y, Sun J R 2014 Nat. Commun. 5 5554
Google Scholar
[20] Au K, Li D F, Chan N Y, Dai J Y 2012 Adv. Mater. 24 2598
Google Scholar
[21] Bark C W, Felker D A, Wang Y, et al. 2011 Proc. Natl. Acad. Sci. U.S.A. 108 4720
Google Scholar
[22] Salluzzo M, Gariglio S, Stornaiuolo D, et al. 2013 Phys. Rev. Lett. 111 087204
Google Scholar
[23] Schoofs F, Carpenter M A, Vickers M E, et al. 2013 J. Phys. Condes. Matter 25 175005
Google Scholar
[24] Huang Z, Liu Z Q, Yang M, et al. 2014 Phys. Rev. B 90 125156
Google Scholar
[25] Du Y, Wang C, Li J, Zhang X, Wang F, Zhu Y, Yin N, Mei L 2015 Comput. Mater. Sci. 99 57
Google Scholar
[26] Meevasana W, King P D C, He R H, Mo S K, Hashimoto M, Tamai A, Songsiriritthigul P, Baumberger F, Shen Z X 2011 Nat. Mater. 10 114
Google Scholar
[27] Fang L, Chen C, Yang Y, Wu Y, Hu T, Zhao G, Zhu Q, Ren W 2019 Phys. Chem. Chem. Phys. 21 8046
Google Scholar
[28] Zhang Z, Jiang W, Liu K, Liu M, Meng J, Wu L, Shao T, Ling J, Yao C, Xiong C, Dou R, Nie J 2020 Ann. Phys. Berlin 532 2000155
Google Scholar
[29] Cooper V R 2012 Phys. Rev. B 85 235109
Google Scholar
[30] Pentcheva R, Pickett W E 2008 Phys. Rev. B 78 205106
Google Scholar
[31] Shu L, Wei X, Jin L, Li Y, Wang H, Yao X 2013 Appl. Phys. Lett. 102 152904
Google Scholar
[32] Nystrom M J, Wessels B W, Chen J, Marks T J 1996 Appl. Phys. Lett. 68 761
Google Scholar
下载:
计量
- 文章访问数: 3059
- PDF下载量: 100
- 被引次数: 0
