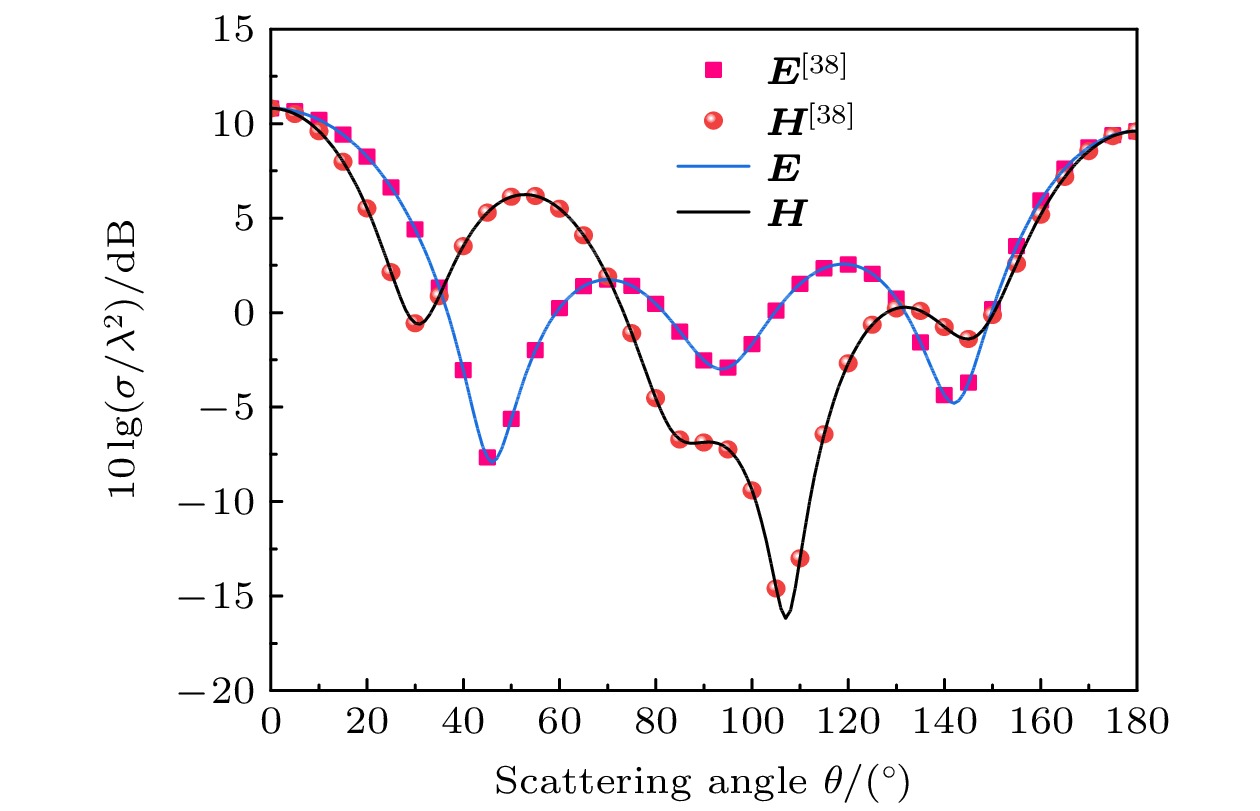
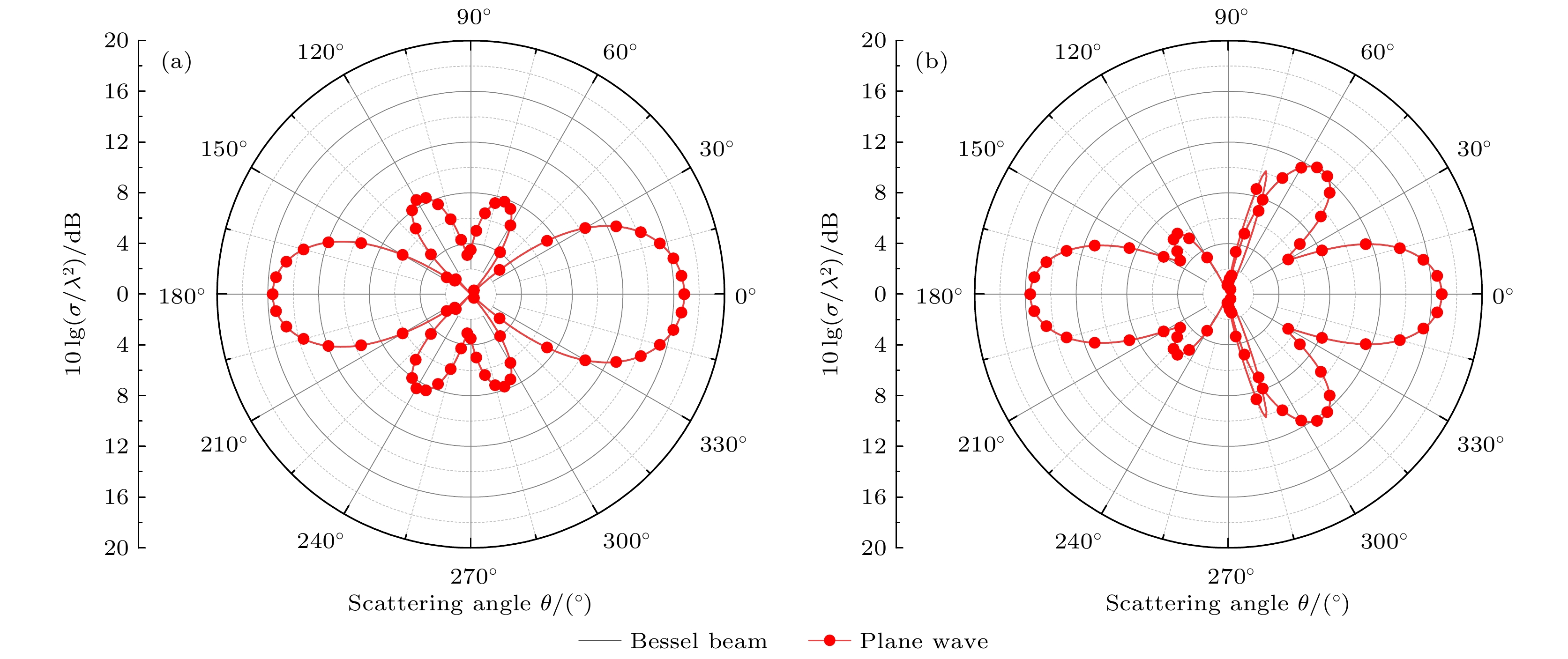
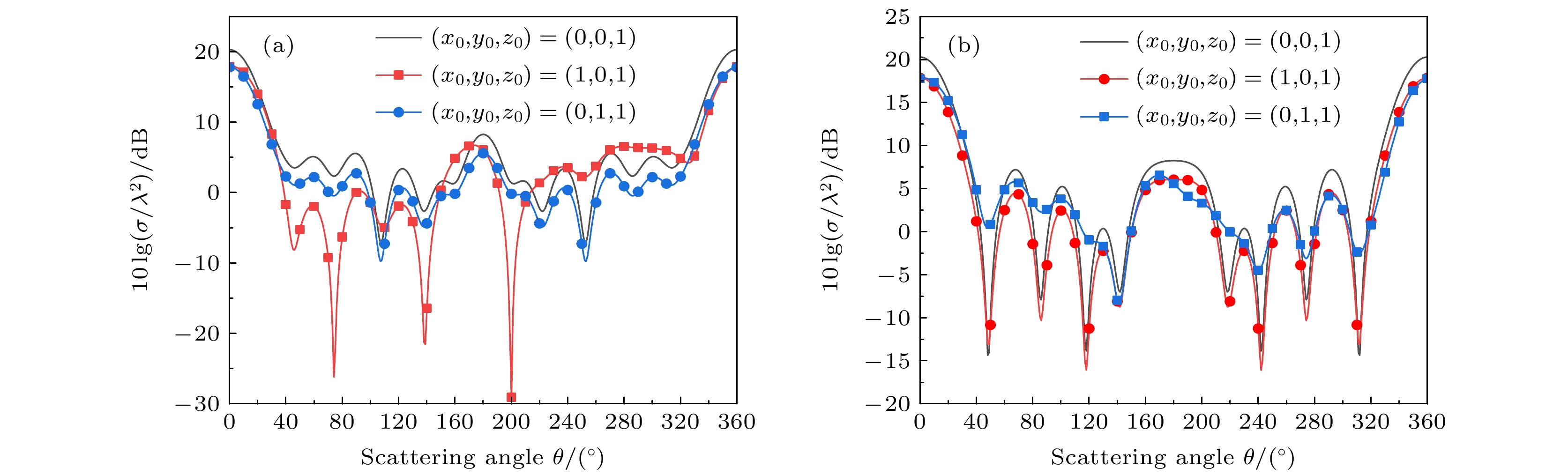
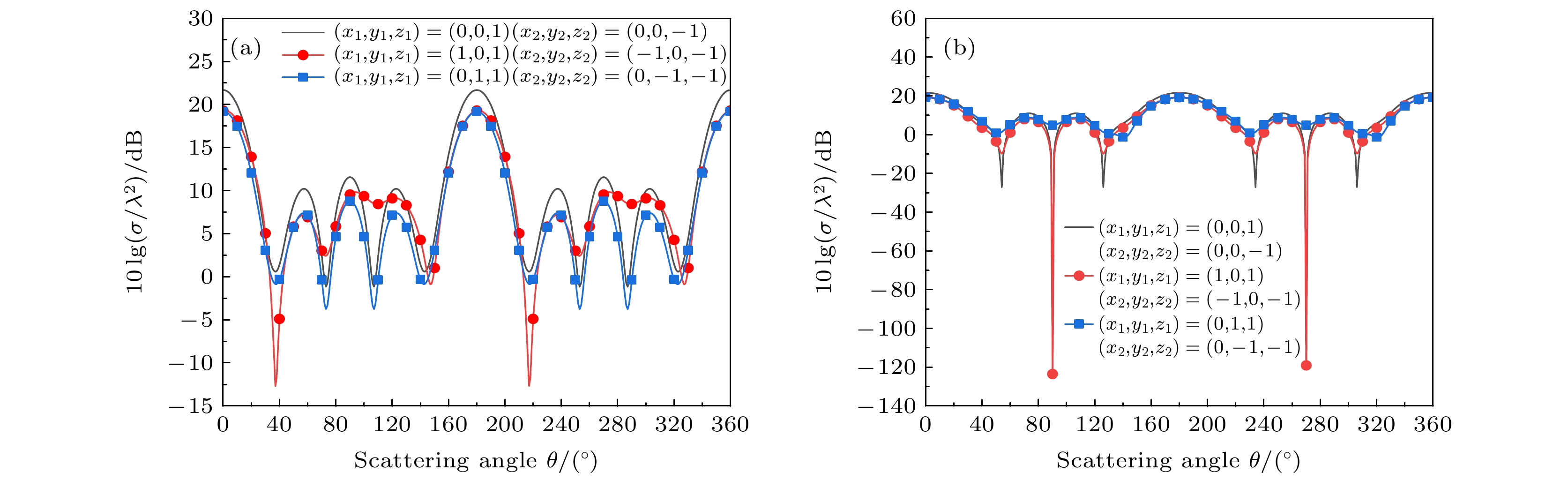
-
Based on the generalized Lorenz Mie theory, the propagation and scattering properties of a uniaxial anisotropic spherical particle illuminated separately by double zero-order Bessel beam with arbitrary propagation direction and polarization direction are studied. The propagation and scattering characteristics are compared with those of a uniaxial anisotropic spherical particle illuminated by a single zero-order Bessel beam. Using the orthogonal relation of the spherical vector wave function and coordinate rotation theorem, the expanded forms of double zero-order Bessel beams with arbitrary propagation direction and polarization direction are derived. The analytical expressions of the expansion coefficients are derived by the integral method. The expansion coefficients of total incident field are obtained through the vector superposition principle. Based on the Fourier transform and tangentially continuous boundary conditions, the internal electromagnetic field of the uniaxial anisotropic sphere is expanded in terms of the spherical vector wave function and the scattering coefficients are derived. By comparing the angular distribution of the radar cross section of the particle illuminated by single and double zero-order Bessel beam when degenerating into plane waves with those results given by the literature, the correctness of the theory and the program in this paper are both verified. The effects of the incidence angle, conic angle and polarization angle on angle distribution of the radar cross section are numerically analyzed. The theoretical and numerical results in this paper are expected to be used to study the scattering properties, particle size analysis and optical trapping for anisotropic particles, biological cells and other particles illuminated by multi-beams.
-
Keywords:
- scattering /
- anisotropic particles /
- double beams /
- Bessel beam
[1] Durnin J 1987 J. Opt. Soc. 4 651
Google Scholar
[2] Garces-Chavez V, Roskey D, Summers M D, Melville H, Mcgloin D, Wright E M, Dholakia K 2004 Appl. Phys. Lett. 85 4001
Google Scholar
[3] Marston P L 2006 J. Acoust. Soc. Am. 120 3518
Google Scholar
[4] Zhang L, Marston P L 2012 J. Acoust. Soc. Am. 131 329
Google Scholar
[5] Milne G, Dholakia K, Mcgloin D 2007 Opt. Express 15 13972
Google Scholar
[6] Vahimaa P, Kettunen V, Kuittnen M 1997 J. Opt. Soc. Am. A 14 1817
Google Scholar
[7] Ding D, Liu X 1999 J. Opt. Soc. Am. A 16 1286
Google Scholar
[8] Cimár T, Kollárová V, Bouchal Z, Zemánek P 2006 New J. Phys. 8 43
Google Scholar
[9] Taylor J M, Love G D 2009 J. Opt. Soc. Am. A 26 278
Google Scholar
[10] Ambrosio L A, Herández-Figueroa H E 2011 Biomed. Opt. Express 2 1893
Google Scholar
[11] Mishra S R 1991 Opt. Commun. 85 159
Google Scholar
[12] Marton P L 2007 J. Acoust. Soc. Am. 121 753
Google Scholar
[13] Ma X B, Li E 2010 Chin. Opt. Lett. 8 1195
Google Scholar
[14] Gouesbet G, Maheu B, Gréhan G 1988 J. Opt. Soc. Am. A 5 1427
Google Scholar
[15] Li R X, Guo L X, Ding C 2013 Opt. Commun. 307 25
Google Scholar
[16] Mitri F G 2011 Opt. Lett. 36 766
Google Scholar
[17] Cui Z W, Han Y P, Han L 2013 J. Opt. Soc. Am. A 30 1913
Google Scholar
[18] Klimov V 2020 Opt. Lett. 45 4300
Google Scholar
[19] Wang X, Wang L, Lin P 2021 J. Innovative. Opt. Health Sci. 14 2150008
Google Scholar
[20] Stout B, Nevière M, Popov E 2006 J. Opt. Soc. Am. A 23 1111
Google Scholar
[21] Wong K L, Chen H T 1992 IEE Proc. H 139 314
Google Scholar
[22] Qiu C W, Li L W, Yeo T S 2007 Phys. Rev. E 75 026609
Google Scholar
[23] Geng Y L, Wu X B, Li L W, Guan B R 2004 Phys. Rev. E 70 056609
Google Scholar
[24] Wang M J, Zhang H Y, Liu G S, Li Y L 2012 J. Opt. Soc. Am. A 29 2376
Google Scholar
[25] Yuan Q K, Wu Z S, Li Z J 2010 J. Opt. Soc. Am. A 27 1457
Google Scholar
[26] Wu Z S, Yuan Q K, Peng Y, Li Z J 2009 J. Opt. Soc. Am. A 26 1778
Google Scholar
[27] Wang J J, Chen A T, Han Y P 2015 J. Quant. Spectrosc. Radiat. Transfer. 167 135
Google Scholar
[28] Qu T, Wu Z S, Shang Q C, Li Z J, Bai L 2013 J. Opt. Soc. Am. A 30 1661
Google Scholar
[29] Zemánek P, Alexandr J, Liska M 2002 J. Opt. Soc. Am. A 19 1025
Google Scholar
[30] Li Z J, Wu Z S, Qu T, Li H Y, Bai L, Gong L 2015 J. Quant. Spectrosc. Radiat. Transfer. 162 56
Google Scholar
[31] Hulst H 1957 Phys. Today 10 28
Google Scholar
[32] Barton J P, Alexander D R, Schaub S A 1988 J. Appl. Phys. 64 1632
Google Scholar
[33] Li Z J, Wu Z S, Qu T, Shang Q C, Bai L 2016 J. Quant. Spectrosc. Radiat. Transfer. 169 1
Google Scholar
[34] Bolton H C 1959 Mathematical Gazette 43 157
Google Scholar
[35] Li Z J, Wu Z S, Huan L, Li H Y 2011 Chin. Phys. B 20 081101
Google Scholar
[36] Li M M, Yin S H, Yao B L, Lei M, Yang Y L, Min J W 2015 J. Opt. Soc. Am. B 32 468
Google Scholar
[37] Wu Z S, Li Z J, Li H Y, Yuan Q K, Li H Y 2011 IEEE Trans. Antennas Propag. 59 4740
Google Scholar
[38] 李正军, 吴振森, 屈檀, 白璐, 曹运华 2014 电波科学学报 29 668
Google Scholar
Li Z J, Wu Z S, Qu T, Bai L, Cao Y H 2014 Chin. J. Radio 29 668
Google Scholar
[39] Li Z J, Wu Z S, Shang Q C 2015 Procedia Eng. 102 89
Google Scholar
-
图 1 双零阶贝塞尔波束离轴斜入射UA介质球的示意图
Figure 1. Schematic diagram of a uniaxial anisotropic sphere illuminated by off-axis obliquely incident double zero-order Bessel beams.
图 2 两直角坐标的旋转关系示意图
Figure 2. Schematic diagram of rotation relationship between two rectangular coordinate systems.
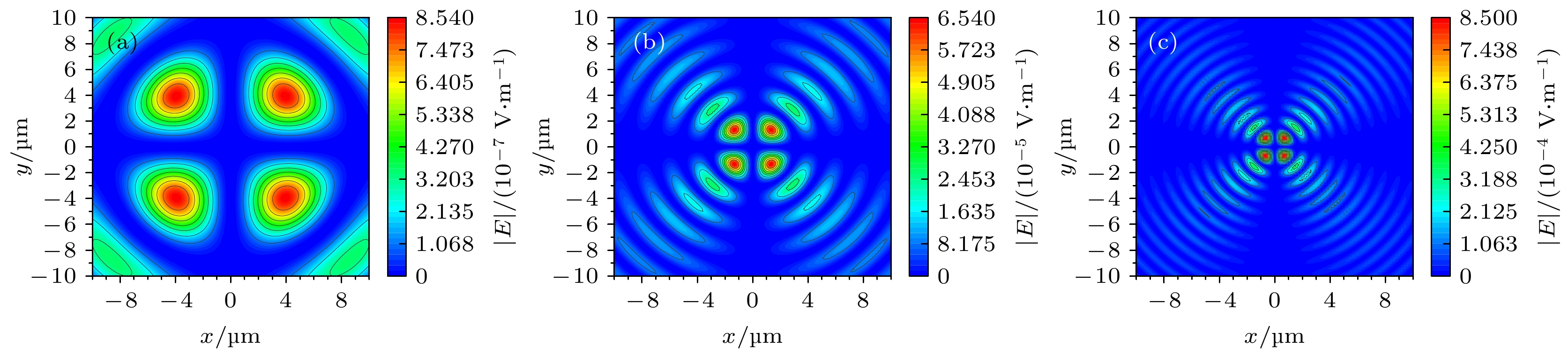
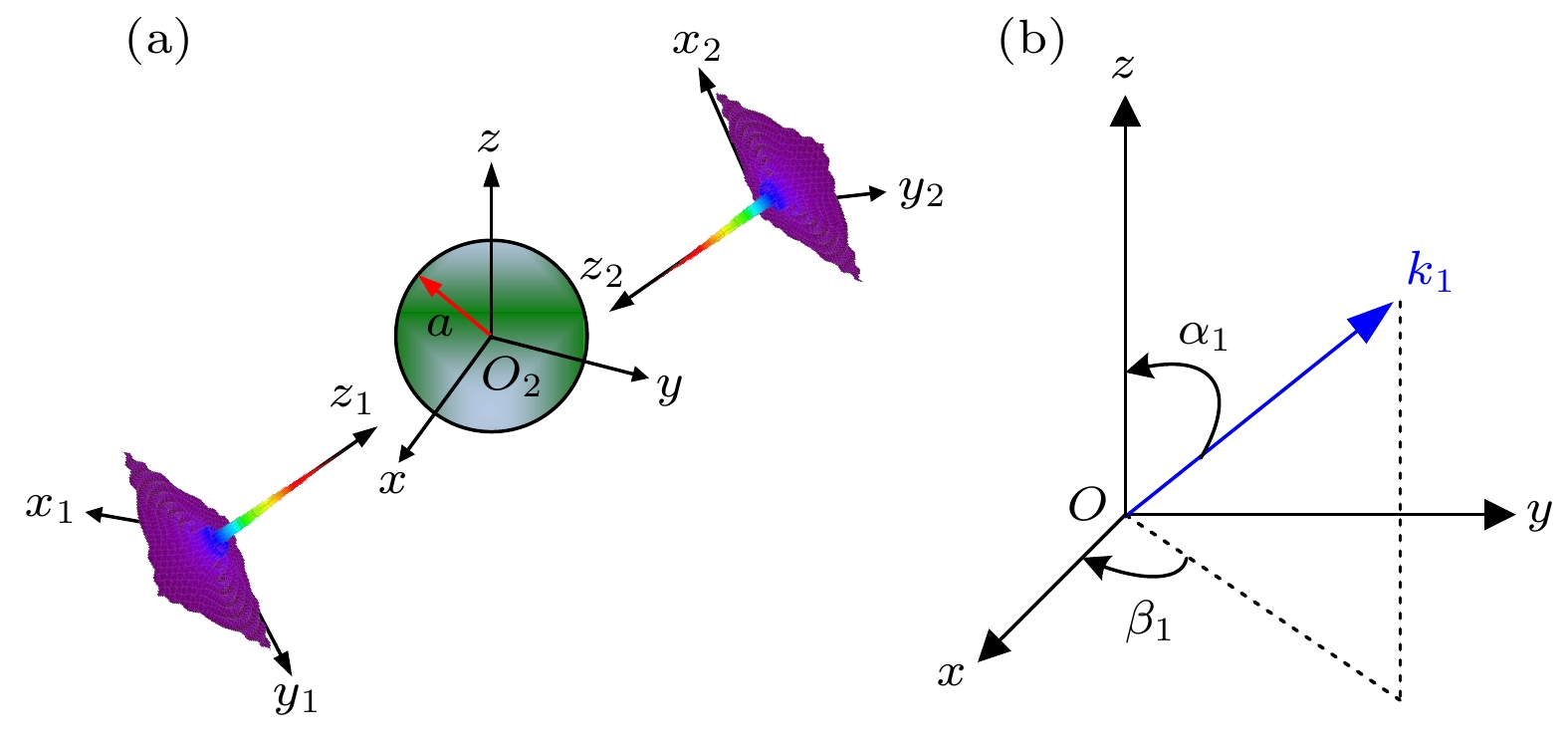
图 3 相同极化角下反向传播双零阶贝塞尔波束的电场强度分布 (a)
${C_1} = {C_2} = 5^\circ $ ; (b)${C_1} = {C_2} = 15^\circ $ ; (c)${C_1} = {C_2} = 30^\circ $ Figure 3. Electric intensity distribution of back propagating double zero-order Bessel beams with identical polarization angles: (a)
${C_1} = $ $ {C_2} = 5^\circ $ ; (b)${C_1} = {C_2} = 15^\circ $ ; (c)${C_1} = {C_2} = 30^\circ $ .
图 4 不同极化角下反向传播双零阶贝塞尔波束的电场强度分布 (a)
${C_1} = {C_2} = 5^\circ $ ; (b)${C_1} = {C_2} = 15^\circ $ ; (c)${C_1} = {C_2} = 30^\circ $ Figure 4. Electric intensity distribution of back propagating double zero-order Bessel beams with different polarization angles: (a)
${C_1} = {C_2} = 5^\circ $ ; (b)${C_1} = {C_2} = 15^\circ $ ; (c)${C_1} = {C_2} = 30^\circ $ .
图 5 斜入射时反向传播双零阶贝塞尔波束的电场强度分布 (a)
${C_1} = {C_2} = 5^\circ $ ; (b)${C_1} = {C_2} = 15^\circ $ ; (c)${C_1} = {C_2} = 30^\circ $ Figure 5. Electric intensity distribution of back propagating double zero-order Bessel beams with oblique incidence: (a)
${C_1} = {C_2} = 5^\circ $ ; (b)${C_1} = {C_2} = 15^\circ $ ; (c)${C_1} = {C_2} = 30^\circ $ .
图 6 斜入射时非反向传播双零阶贝塞尔波束的电场强度分布 (a)
${C_1} = {C_2} = 5^\circ $ ; (b)${C_1} = {C_2} = 15^\circ $ ; (c)${C_1} = $ $ {C_2} = 30^\circ $ Figure 6. Electric intensity distribution of non-back propagating double zero-order Bessel beams with oblique incidence: (a)
${C_1} = $ $ {C_2} = 5^\circ $ ; (b)${C_1} = {C_2} = 15^\circ $ ; (c)${C_1} = {C_2} = 30^\circ $ .
图 7 UA介质球对单零阶贝塞尔波束的RCS角分布
Figure 7. Normalized RCS of a UA dielectric sphere illuminated by single zero-order Bessel beam versus the scattering angle.
图 8 双零阶贝塞尔波束退化成平面波入射UA介质球的RCS角分布 (a) E-plane; (b) H-plane
Figure 8. Angular distribution of the RCS of a UA dielectric sphere by double zero-order Bessel beams when degenerating into plane waves: (a) E-plane; (b) H-plane.
图 9 UA介质球对不同离轴情形入射的单零阶贝塞尔波束的RCS角分布 (a) E-plane; (b) H-plane
Figure 9. Angular distribution of the RCS of a UA dielectric sphere illuminated by different off-axis incident zero-order Bessel beams: (a) E-plane; (b) H-plane.
图 10 UA介质球对离轴入射的双零阶贝塞尔波束的RCS角分布 (a) E-plane; (b) H-plane
Figure 10. Angular distribution of the RCS of a UA dielectric sphere illuminated by different off-axis incident double zero-order Bessel beams: (a) E-plane; (b) H-plane.
图 11 UA介质球对不同入射角下双零阶贝塞尔波束的RCS角分布 (a) E-plane; (b) H-plane
Figure 11. Angular distribution of the RCS of a UA dielectric sphere illuminated by double zero-order Bessel beams with different incident angles: (a) E-plane; (b) H-plane.
图 12 双反向传输的零阶贝塞尔波束在不同锥角入射的情况下UA介质球的RCS角分布 (a) E-plane; (b) H-plane
Figure 12. Angular distribution of the RCS of a UA dielectric sphere illuminated by back propagating double zero-order Bessel beams with different conic angles: (a) E-plane; (b) H-plane.
-
[1] Durnin J 1987 J. Opt. Soc. 4 651
Google Scholar
[2] Garces-Chavez V, Roskey D, Summers M D, Melville H, Mcgloin D, Wright E M, Dholakia K 2004 Appl. Phys. Lett. 85 4001
Google Scholar
[3] Marston P L 2006 J. Acoust. Soc. Am. 120 3518
Google Scholar
[4] Zhang L, Marston P L 2012 J. Acoust. Soc. Am. 131 329
Google Scholar
[5] Milne G, Dholakia K, Mcgloin D 2007 Opt. Express 15 13972
Google Scholar
[6] Vahimaa P, Kettunen V, Kuittnen M 1997 J. Opt. Soc. Am. A 14 1817
Google Scholar
[7] Ding D, Liu X 1999 J. Opt. Soc. Am. A 16 1286
Google Scholar
[8] Cimár T, Kollárová V, Bouchal Z, Zemánek P 2006 New J. Phys. 8 43
Google Scholar
[9] Taylor J M, Love G D 2009 J. Opt. Soc. Am. A 26 278
Google Scholar
[10] Ambrosio L A, Herández-Figueroa H E 2011 Biomed. Opt. Express 2 1893
Google Scholar
[11] Mishra S R 1991 Opt. Commun. 85 159
Google Scholar
[12] Marton P L 2007 J. Acoust. Soc. Am. 121 753
Google Scholar
[13] Ma X B, Li E 2010 Chin. Opt. Lett. 8 1195
Google Scholar
[14] Gouesbet G, Maheu B, Gréhan G 1988 J. Opt. Soc. Am. A 5 1427
Google Scholar
[15] Li R X, Guo L X, Ding C 2013 Opt. Commun. 307 25
Google Scholar
[16] Mitri F G 2011 Opt. Lett. 36 766
Google Scholar
[17] Cui Z W, Han Y P, Han L 2013 J. Opt. Soc. Am. A 30 1913
Google Scholar
[18] Klimov V 2020 Opt. Lett. 45 4300
Google Scholar
[19] Wang X, Wang L, Lin P 2021 J. Innovative. Opt. Health Sci. 14 2150008
Google Scholar
[20] Stout B, Nevière M, Popov E 2006 J. Opt. Soc. Am. A 23 1111
Google Scholar
[21] Wong K L, Chen H T 1992 IEE Proc. H 139 314
Google Scholar
[22] Qiu C W, Li L W, Yeo T S 2007 Phys. Rev. E 75 026609
Google Scholar
[23] Geng Y L, Wu X B, Li L W, Guan B R 2004 Phys. Rev. E 70 056609
Google Scholar
[24] Wang M J, Zhang H Y, Liu G S, Li Y L 2012 J. Opt. Soc. Am. A 29 2376
Google Scholar
[25] Yuan Q K, Wu Z S, Li Z J 2010 J. Opt. Soc. Am. A 27 1457
Google Scholar
[26] Wu Z S, Yuan Q K, Peng Y, Li Z J 2009 J. Opt. Soc. Am. A 26 1778
Google Scholar
[27] Wang J J, Chen A T, Han Y P 2015 J. Quant. Spectrosc. Radiat. Transfer. 167 135
Google Scholar
[28] Qu T, Wu Z S, Shang Q C, Li Z J, Bai L 2013 J. Opt. Soc. Am. A 30 1661
Google Scholar
[29] Zemánek P, Alexandr J, Liska M 2002 J. Opt. Soc. Am. A 19 1025
Google Scholar
[30] Li Z J, Wu Z S, Qu T, Li H Y, Bai L, Gong L 2015 J. Quant. Spectrosc. Radiat. Transfer. 162 56
Google Scholar
[31] Hulst H 1957 Phys. Today 10 28
Google Scholar
[32] Barton J P, Alexander D R, Schaub S A 1988 J. Appl. Phys. 64 1632
Google Scholar
[33] Li Z J, Wu Z S, Qu T, Shang Q C, Bai L 2016 J. Quant. Spectrosc. Radiat. Transfer. 169 1
Google Scholar
[34] Bolton H C 1959 Mathematical Gazette 43 157
Google Scholar
[35] Li Z J, Wu Z S, Huan L, Li H Y 2011 Chin. Phys. B 20 081101
Google Scholar
[36] Li M M, Yin S H, Yao B L, Lei M, Yang Y L, Min J W 2015 J. Opt. Soc. Am. B 32 468
Google Scholar
[37] Wu Z S, Li Z J, Li H Y, Yuan Q K, Li H Y 2011 IEEE Trans. Antennas Propag. 59 4740
Google Scholar
[38] 李正军, 吴振森, 屈檀, 白璐, 曹运华 2014 电波科学学报 29 668
Google Scholar
Li Z J, Wu Z S, Qu T, Bai L, Cao Y H 2014 Chin. J. Radio 29 668
Google Scholar
[39] Li Z J, Wu Z S, Shang Q C 2015 Procedia Eng. 102 89
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4328
- PDF Downloads: 61
- Cited By: 0
