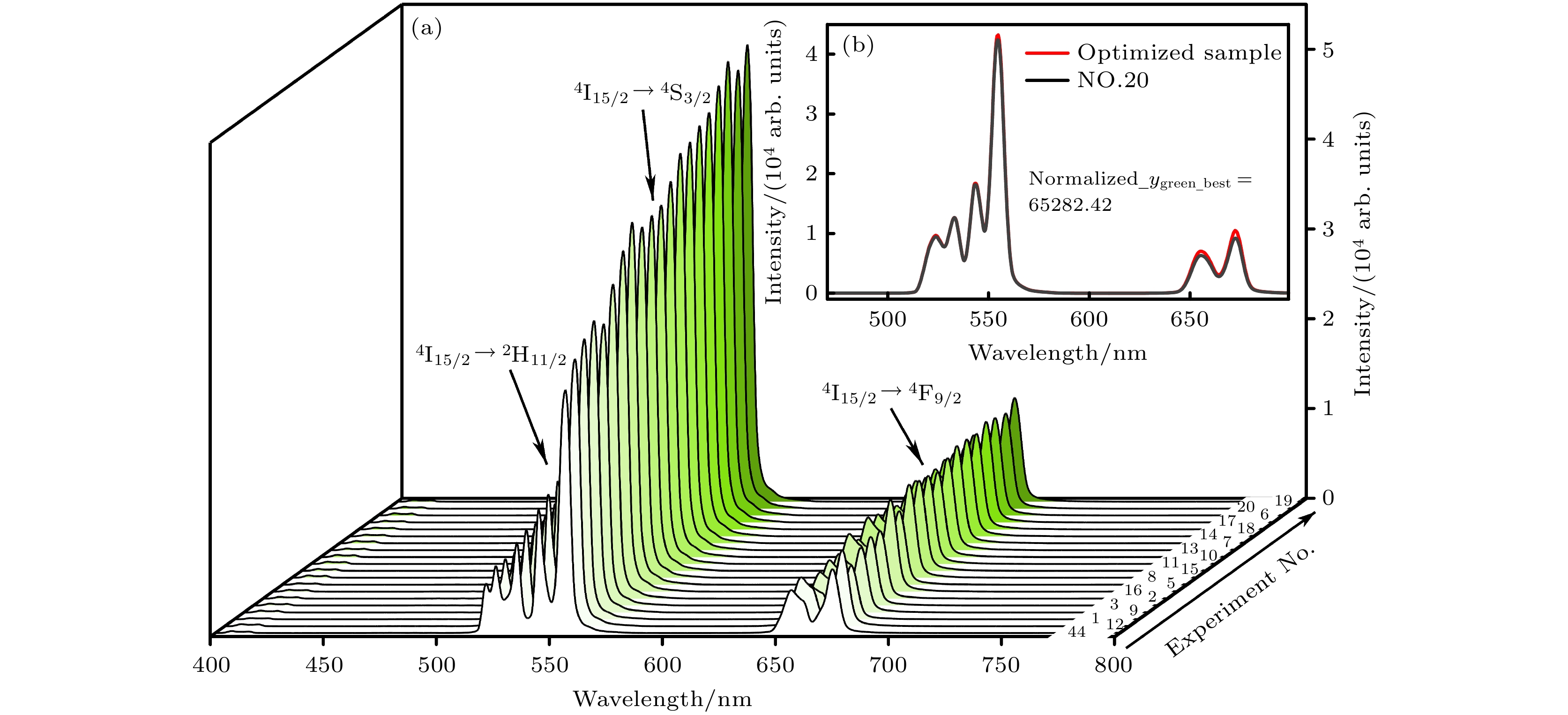
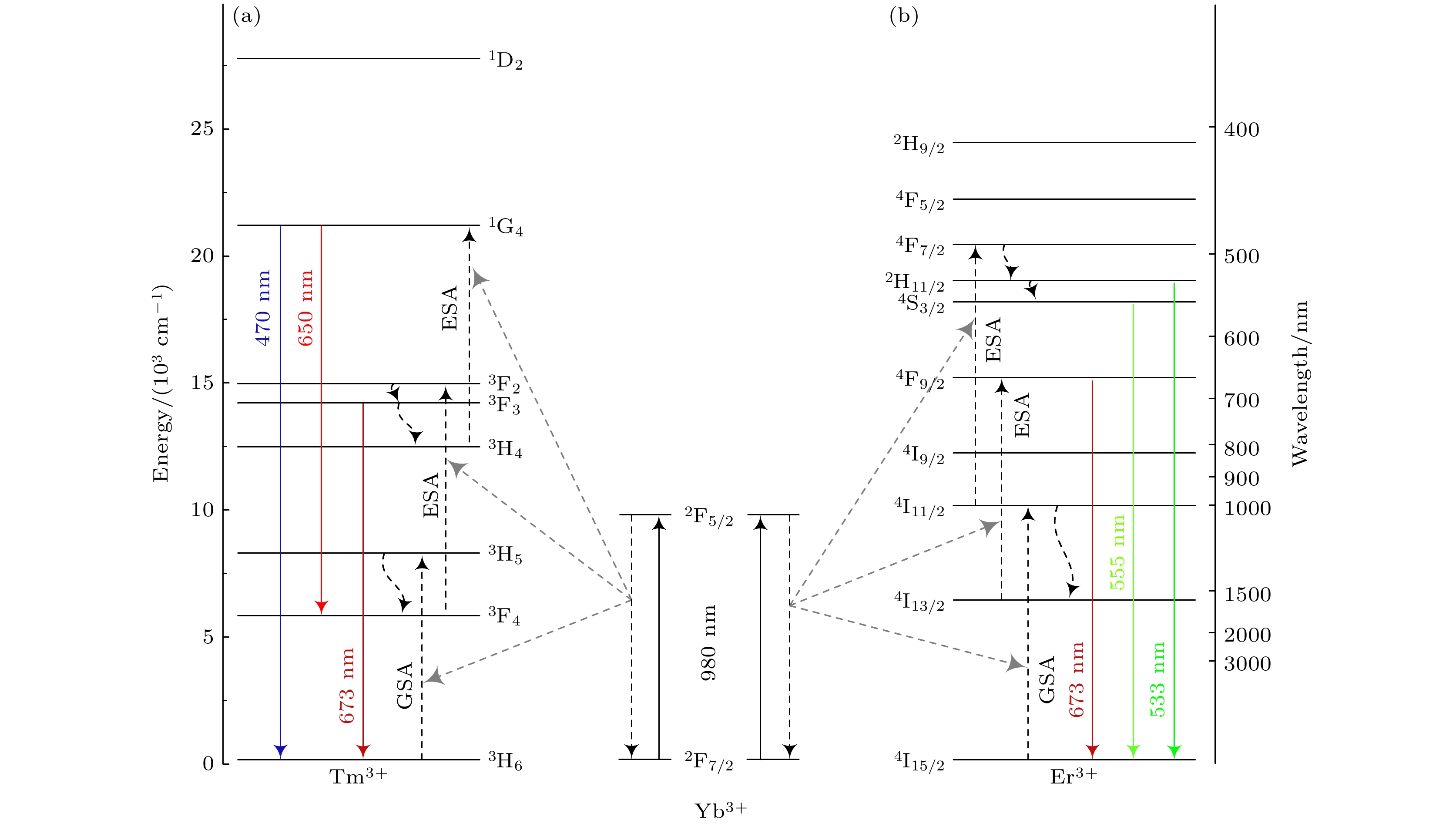
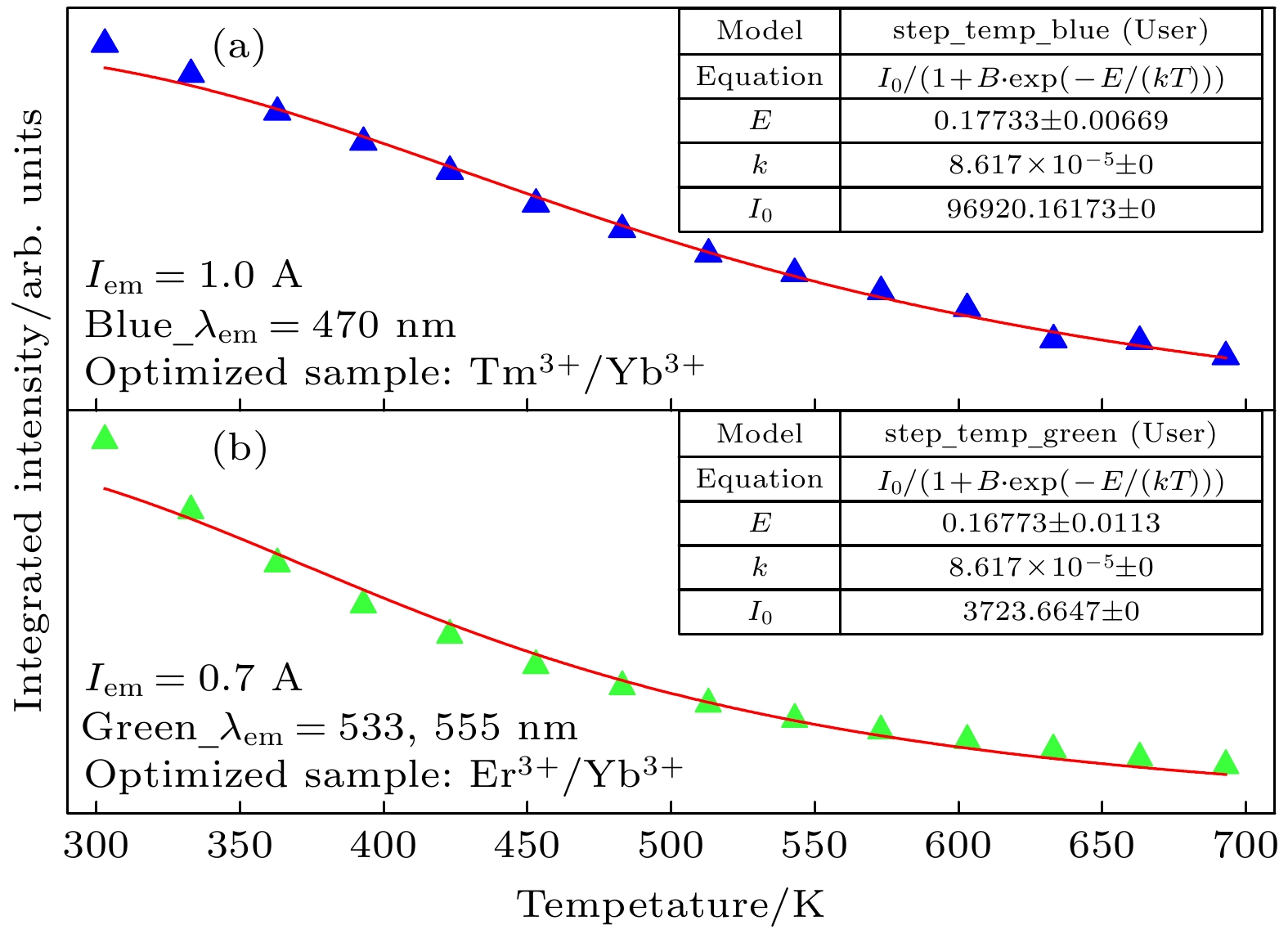
-
In order to obtain the maximum characteristic intensities of the up-conversion luminescence in GdTaO4:RE/Yb(RE = Tm, Er) series, we establish the regression equation between the luminescent intensity of the phosphors and the rare earth doping concentration upon the 980 nm laser excitation based on the experimental optimization design. The Tm3+/Yb3+ doping samples are combined with the uniform design and quadratic general rotation combination design, meanwhile the Er3+/Yb3+ doping samples are optimized by the uniform design and cubic orthogonal phosphor step by step. The relationship between concentration and luminous intensity is analyzed. The results show that the changes of concentration of RE3+ (RE = Tm, Er) and Yb3+ can exert a significant effect on luminous intensity, and there exist extreme points of luminescent intensity in the test space. By solving the regression equation, we obtain the optimal doping concentration. The optimal samples are also prepared by the high-temperature solid state method. The XRD diffraction patterns of the optimal samples are analyzed. The results show that the samples are of pure phase, the doping of Li+ flux will inhibit the generation of reaction impurity phase, and the doping of rare earth will shift the diffraction peak to a high angle, with the peak shape remaining unchanged. The relationship between excitation power and luminescent intensity is analyzed. The results show that the blue light emission of Tm3+/Yb3+ co-doped phosphor is a three-photon process, and the green light emission of Er3+/Yb3+ co-coped phosphor is a two-photon process. The relationship between sample temperature and luminescent intensity is analyzed. The luminescent intensity of the sample decreases with the increase of the temperature, indicating temperature quenching. Finally, the quenching activated energy of the sample is calculated.
-
Keywords:
- up-conversion /
- quadratic general rotary unitized design /
- orthogonal polynomial regression design /
- GdTaO4:RE/Yb(RE = Tm /
- Er)
[1] Zhang W S, Gao Q, Zhou S, Li L J, Ma X Z 2021 Opt. Laser Technol. 114 107368
[2] Chen Y Z, Peng F, Zhang Q L, Liu W P, Dou R Q, Ding S J, Luo J Q, Sun D L, Sun G H, Wang X F 2017 J. Lumin. 192 555
Google Scholar
[3] Dai T Y, Guo S X, Duan X M, Dou R Q, Zhang Q L 2019 Opt. Express 27 34205
[4] Issler S L, Torardi C C 1995 J. Alloy Compd. 229 54
Google Scholar
[5] Li B, Gu Z N, Lin J H, Su M Z 2000 Mater. Res. Bull. 35 1921
Google Scholar
[6] Siqueira K P F, Carmo A P, Bell M J V, Dias A 2013 J. Lumin. 138 133
Google Scholar
[7] Brixner L H, Chen H 1983 J. Electrochem. Soc. 130 12
Google Scholar
[8] Roy A, Dwivedi A, Kumar D, Mishra H, Rai S B 2020 Ceram. Int. 46 24893
Google Scholar
[9] Roy A, Dwivedi A, Mishra H, Kumar D, Rai S B 2020 J. Alloy Compd. 821 2020
[10] Sun G H, Zhang Q L, Luo J Q, Liu W P, Han S, Zheng L L, Li W M 2019 J. Lumin. 217 116831
[11] 任露泉 2009 试验优化设计与分析 (北京: 科学出版社) 第1页
Ren L Q 2009 Design of Experiment and Optimization (Beijing: Science Press) p1 (in Chinese)
[12] Sun J S, Shi L L, Li S W, Li J J, Li X P, Zhang J S, Cheng L H, Chen B J 2016 Mater. Res. Bull. 80 102
Google Scholar
[13] 刘盛意, 张金苏, 孙佳石, 陈宝玖, 李香萍, 徐赛, 程丽红 2019 68 053301
Google Scholar
Liu S Y, Zhang J S, Sun J S, Chen B J, Li X P, Xu S, Cheng L H 2019 Acta Phys. Sin. 68 053301
Google Scholar
[14] 赵越, 杨帆, 孙佳石, 李香萍, 张金苏, 张希珍, 徐赛, 程丽红, 陈宝玖 2019 68 213301
Google Scholar
Zhao Y, Yang F, Sun J S, Li X P, Zhang J S, Zhang X Z, Xu S, Cheng L H, Chen B J 2019 Acta Phys. Sin. 68 213301
Google Scholar
[15] 孙佳石, 李香萍, 吴金磊, 李树伟, 石琳琳, 徐赛, 张金苏, 程丽红, 陈宝玖 2017 66 100201
Google Scholar
Sun J S, Li X P, Wu J L, Li S W, Shi L L, Xu S, Zhang J S, Cheng L H, Chen B J 2017 Acta Phys. Sin. 66 100201
Google Scholar
[16] 何为, 薛卫东, 唐斌 2012 优化试验设计方法及数据分析 (北京: 化学工业出版社) 第164—170页
He W, Xue W D, Tang B 2012 The Method of Opti-mal Design of Experiment and Data Analysis (Beijing: Chemical Industry Press) pp164–170 (in Chinese)
[17] 杨帆 2020 硕士学位论文 (大连: 大连海事大学)
Yang F 2020 M. S. Thesis (Dalian: Dalian Maritime University) (in Chinese)
[18] 任露泉 2009 回归设计及其优化 (北京: 科学出版社) 第12页
Ren L Q 2009 Regression Design and Optimization (Beijing: Science Press) p12 (in Chinese)
[19] He C, Yang K S, Liu L, Si Z J 2013 J. Rare Earths 31 790
Google Scholar
[20] Van U 1967 J. Electrochem. Soc. 114 1048
Google Scholar
[21] Tian Y, Chen B J, Hua R N, Yu N S, Liu B Q, Sun J S, Cheng L H, Zhong H Y, Li X P, Zhang J S, Tian B N, Zhong H 2012 CrystEngComm 14 1760
Google Scholar
[22] Tian B N, Chen B J, Tian Y, Sun J S, Li X P, Zhang J S, Zhong H Y, Cheng L H, Hua R N 2012 J. Phys. Chem. Solids 73 1314
Google Scholar
[23] Liu X L, Han K, Gu M, Xiao L H, Ni C, Huang S M, Liu B 2007 Solid State Commun. 142 680
Google Scholar
[24] 肖莉红, 顾牡, 刘小林, 张睿, 刘冰洁, 徐昕 2007 光谱学与光谱分析 27 1054
Google Scholar
Xiao L H, Gu M, Liu X L, Zhang R, Liu B J, Xu X 2007 Spectrosc. Spectral Anal. 27 1054
Google Scholar
[25] 傅尚怡, 顾牡, 刘小林, 倪晨, 刘波, 黄世明 2010 光谱学与光谱分析 30 2317
Google Scholar
Fu S Y, Gu M, Liu X L, Ni C, Liu B, Huang S M 2010 Spectrosc. Spectral Anal. 30 2317
Google Scholar
[26] Liu W J, Zhang W J, Liu R X, Li G J 2021 New J. Chem. 45 9818
Google Scholar
[27] Yu H Q, Jiang P P, Chen B J, Sun J S, Cheng L H, Li X P, Zhang J S, Xu S 2020 Appl. Phys. A 126 690
Google Scholar
[28] Jung K Y 2020 RSC Adv. 10 16323
Google Scholar
[29] 张洪杰, 苏锵 1988 应用化学 3 28
Zhang H J, Su Q 1988 Chin. J. Appl. Chem. 3 28
-
图 1 Tm3+/Yb3+共掺浓度与蓝光发光强度关系图
Figure 1. Relationship between Tm3+/Yb3+ co-doped concentration and blue emission intensity.
图 2 (a) 旋转组合设计实验点发射光谱图(根据积分强度大小排序); (b) 序号10样品与最优样品同激发条件发射光谱图
Figure 2. (a) Emission spectra of rotary unitized design experimental point (sort by integrated results); (b) emission spectra of No. 10 and optimized sample under same excitation power.
图 3 Er3+/Yb3+共掺浓度与绿光发光强度关系图
Figure 3. Relationship between Er3+/Yb3+ co-doped concentration and green emission intensity.
图 4 (a) 正交多项式回归设计实验点发射光谱图(根据积分强度大小排序); (b) 序号20样品与最优样品同激发条件发射光谱图
Figure 4. (a) Emission spectra of orthogonal polynomial regression design experimental point (sort by integrated results). (b) emission spectra of No. 20 and optimized sample under same excitation power.
图 5 GdTaO4系列样品XRD图谱与标准JCPDS#24-0441
Figure 5. XRD patterns of GdTaO4 series samples and standard JCPDS#24-0441.
图 6 (a) Tm3+/Yb3+共掺最优样品变激发功率发射光谱图; (b) 蓝光波段和红光波段积分与工作电流拟合曲线
Figure 6. (a) Emission spectra of Tm3+/Yb3+ co-doping optimized sample for variable excitation power; (b) curves fitted between the integral of the blue, red bands and the operating current.
图 8 (a) Tm3+/Yb3+最优样品能级敏化关系图; (b) Er3+/Yb3+最优样品能级敏化关系图
Figure 8. (a) Tm3+/Yb3+ optimized sample energy level sensitization chart; (b) Er3+/Yb3+ optimized sample energy level sensitization chart.
图 7 (a) Er3+/Yb3+共掺最优样品变激发功率发射光谱图; (b) 绿波段和红光波段积分与工作电流拟合曲线
Figure 7. (a) Emission spectra of Er3+/Yb3+ co-doping optimized sample for variable excitation power; (b) curves fitted between the integral of the green, red bands and the operating current.
图 9 (a) Tm3+/Yb3+最优样品发光积分强度与温度关系; (b) Er3+/Yb3+最优样品发光积分强度与温度关系
Figure 9. (a) Tm3+/Yb3+ optimized sample dependence of integrated intensity on temperature; (b) Er3+/Yb3+ optimized sample dependence of integrated intensity on temperature.
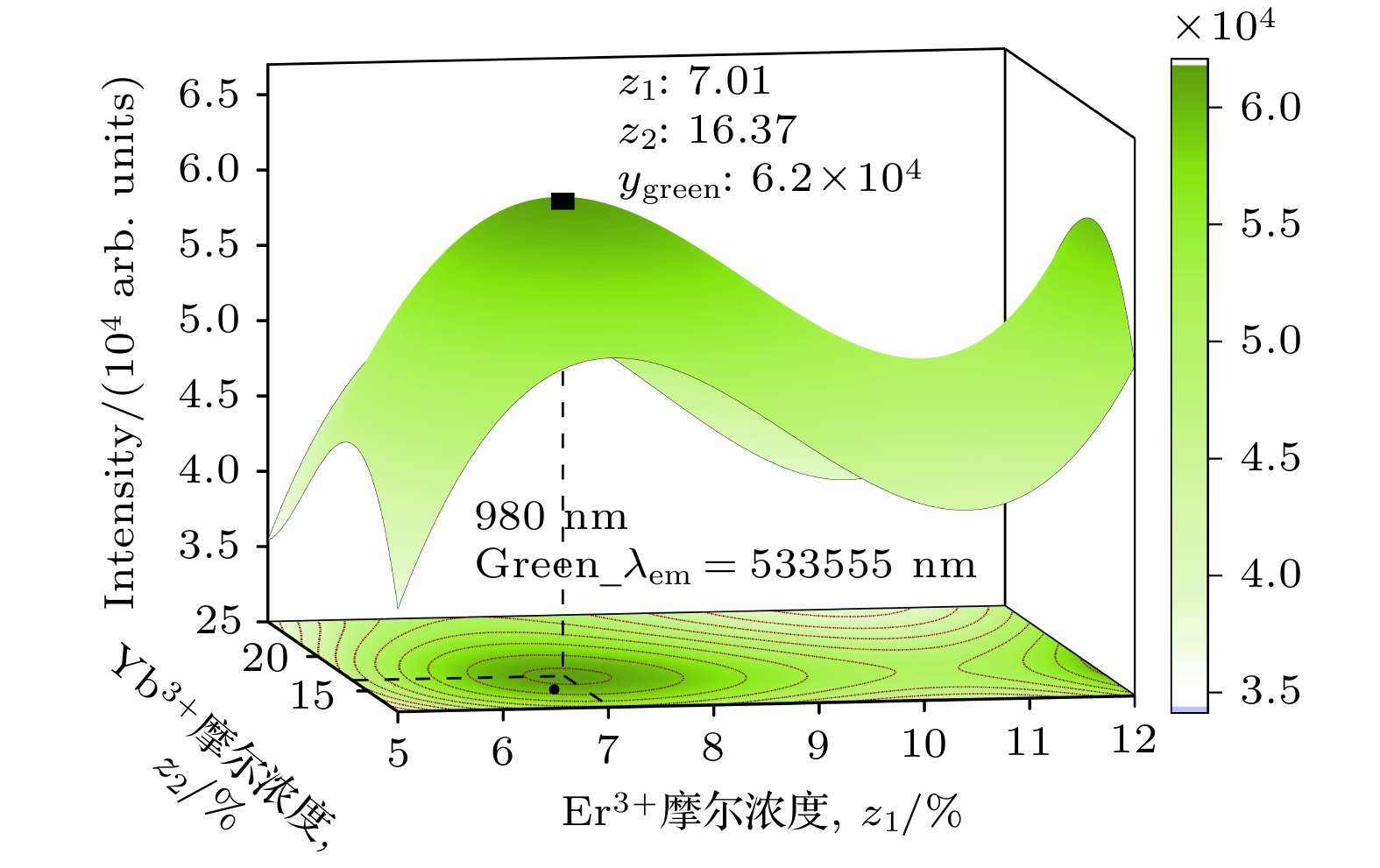
表 1 Tm3+/Yb3+ U9(92)试验方案和积分强度
Table 1. Tm3+/Yb3+ U9(92) experimental design and integrated intensity.
No. Factors ${y_{\rm{b\_int} } }$/(arb. units) Tm3+/mol% Yb3+/mol% 1 1 (0.1) 4 (6.25) 14548.3 2 2 (0.9625) 8 (13.25) 40832.1 3 3 (1.825) 3 (4.5) 16268.4 4 4 (2.6875) 7 (11.5) 27236.0 5 5 (3.55) 2 (2.75) 7918.2 6 6 (4.4125) 6 (9.75) 10844.0 7 7 (5.275) 1 (1) 2176.0 8 8 (6.1375) 5 (8) 7370.5 9 9 (7) 9 (15) 7673.2 
DownLoad: CSV
表 2 Er3+/Yb3+ U11(112)试验方案和积分强度
Table 2. Er3+/Yb3+ U11(112) experimental design and integrated intensity.
No. Factors ${y_{\rm{g\_int}}}$/(arb. units) Er3+/mol% Yb3+/mol% 1 1 (1) 7 (32) 2615.08 2 2 (3.9) 3 (14) 65415.60 3 3 (6.8) 10 (45.5) 13779.16 4 4 (9.7) 6 (27.5) 67919.49 5 5 (12.6) 2 (9.5) 53751.00 6 6 (15.5) 9 (41) 27765.73 7 7 (18.4) 5 (23) 60404.28 8 8 (21.3) 1 (5) 27232.45 9 9 (24.2) 8 (36.5) 25725.90 10 10 (27.1) 4 (18.5) 45363.83 11 11 (30) 11 (50) 5775.05
DownLoad: CSV
表 3 Tm3+/Yb3+自然因素水平及编码设计表
Table 3. Tm3+/Yb3+ natural factors level and coding table.
xj(zj) z1 z2 Tm3+/mol% Yb3+/mol% $ r({z_{2 j}}) $ 0.4 20 $ 1({z_{0 j}} + {\Delta _j}) $ 0.3444 18.5361 $ 0({z_{0 j}}) $ 0.21 15 $ - 1({z_{0 j}} - {\Delta _j}) $ 0.0756 11.4639 $ - r({z_{1 j}}) $ 0.02 10 $ {\Delta _j} = ({{{z_{2 j}} - {z_{1 j}}}})/{{2 r}} $ 0.1344 3.5361 $ {x_j} = \dfrac{{{z_j} - {z_{0 j}}}}{{{\Delta _j}}} $ $ {x_1} = \dfrac{{{z_1} - 0.21}}{{0.1344}} $ $ {x_2} = \dfrac{{{z_2} - 15}}{{3.5361}} $
DownLoad: CSV
表 4 Tm3+/Yb3+二次通用旋转组合设计试验方案及蓝光积分结果
Table 4. Tm3+/Yb3+ scheme of quadratic general rotary unitized design and blue luminescence integrated results.
No. Factors ${y_{\rm{b\_int}} }$/
(arb. units)$ {x_0} $ $ {x_1}({z_1}) $ $ {x_2}({z_2}) $ $ {x_1}{x_2} $ $ x_1^2 $ $ x_2^2 $ 1 1 1 1 1 1 1 103074.268 2 1 1 –1 –1 1 1 82246.127 3 1 –1 1 –1 1 1 52874.380 4 1 –1 –1 1 1 1 59604.598 5 1 r 0 0 r2 0 99703.531 6 1 –r 0 0 r2 0 52894.450 7 1 0 r 0 0 r2 102782.281 8 1 0 –r 0 0 r2 92052.066 9 1 0 0 0 0 0 91641.231 10 1 0 0 0 0 0 119721.420 11 1 0 0 0 0 0 107477.062 12 1 0 0 0 0 0 102883.388 13 1 0 0 0 0 0 100900.284
DownLoad: CSV
表 5 Er3+/Yb3+正交多项式回归设计试验方案及绿光积分结果
Table 5. Er3+/Yb3+ scheme of orthogonal polynomial regression design and green luminescence integrated results.
No. scheme ψ0 X1
(z1)X2
(z1)X3
(z1)X1
(z2)X2
(z2)X3
(z2)X1X1
(z1z2)${y_{\rm{g\_int}} }$/
(arb. unit)$ {z_1} $ $ {z_2} $ 1 5 12 1 –1 1 –1 –3 1 –1 3 38813.91 2 5 16.33 1 –1 1 –1 –1 –1 3 1 43767.25 3 5 20.67 1 –1 1 –1 1 –1 –3 –1 39883.01 4 5 25 1 –1 1 –1 3 1 1 –3 34969.53 5 7.33 12 1 0 –2 3 –3 1 –1 0 49578.38 6 7.33 16.33 1 0 –2 3 –1 –1 3 0 62691.10 7 7.33 20.67 1 0 –2 3 1 –1 –3 0 56730.83 8 7.33 25 1 0 –2 3 3 1 1 0 49814.24 9 9.67 12 1 1 1 –3 –3 1 –1 –3 39812.41 10 9.67 16.33 1 1 1 –3 –1 –1 3 –1 52462.51 11 9.67 20.67 1 1 1 –3 1 –1 –3 1 50272.68 12 9.67 25 1 1 1 –3 3 1 1 3 38264.82 13 12 12 1 3 1 1 –3 1 –1 3 54874.82 14 12 16.33 1 3 1 1 –1 –1 3 –9 56897.90 15 12 20.67 1 3 1 1 1 –1 –3 3 50154.07 16 12 25 1 3 1 1 3 1 1 9 48139.09 17 7.33 16.33 — — — — — — — — 61723.89 18 7.33 16.33 — — — — — — — — 58839.98 19 7.33 16.33 — — — — — — — — 64899.02 20 7.33 16.33 — — — — — — — — 63139.10
DownLoad: CSV
表 6 旋转组合设计F方差检验和显著性分析
Table 6. Rotary unitized design F-variance test and significant analysis.
计算
项目偏差平方和 自由度 $ {F}_{比} $ 显著性
α$ {S}_{回} $ 4995616451.05 5 10.72 0.01 $ {S_{\text{R}}} $ 652160821.93 7 ${S_{\rm{lf}} }$ 230690012.89 3 0.73 0.01 ${S_{\text{e} } }$ 421470809.04 4 $ {S}_{总} $ 5647777272.99 12
DownLoad: CSV
表 7 正交多项式回归设计 F 方差检验和显著性分析
Table 7. Orthogonal polynomial regression design F-variance test and significant analysis.
计算
项目偏差平方和 自由度 ${{F} }_{\text{比} }$ 显著性 $ \text{α} $ $ {S}_{回} $ 900041174.40 7 12.06 0.01 $ {S_{\text{R}}} $ 85273837.77 8 ${S_{\rm e } }$ 20165233.95 3 0.38 0.01 $ {S}_{总} $ 985315012.10 15 $ {\widehat y_0} $ 61434.84 $ {\overline y _0} $ 63027.40
DownLoad: CSV
-
[1] Zhang W S, Gao Q, Zhou S, Li L J, Ma X Z 2021 Opt. Laser Technol. 114 107368
[2] Chen Y Z, Peng F, Zhang Q L, Liu W P, Dou R Q, Ding S J, Luo J Q, Sun D L, Sun G H, Wang X F 2017 J. Lumin. 192 555
Google Scholar
[3] Dai T Y, Guo S X, Duan X M, Dou R Q, Zhang Q L 2019 Opt. Express 27 34205
[4] Issler S L, Torardi C C 1995 J. Alloy Compd. 229 54
Google Scholar
[5] Li B, Gu Z N, Lin J H, Su M Z 2000 Mater. Res. Bull. 35 1921
Google Scholar
[6] Siqueira K P F, Carmo A P, Bell M J V, Dias A 2013 J. Lumin. 138 133
Google Scholar
[7] Brixner L H, Chen H 1983 J. Electrochem. Soc. 130 12
Google Scholar
[8] Roy A, Dwivedi A, Kumar D, Mishra H, Rai S B 2020 Ceram. Int. 46 24893
Google Scholar
[9] Roy A, Dwivedi A, Mishra H, Kumar D, Rai S B 2020 J. Alloy Compd. 821 2020
[10] Sun G H, Zhang Q L, Luo J Q, Liu W P, Han S, Zheng L L, Li W M 2019 J. Lumin. 217 116831
[11] 任露泉 2009 试验优化设计与分析 (北京: 科学出版社) 第1页
Ren L Q 2009 Design of Experiment and Optimization (Beijing: Science Press) p1 (in Chinese)
[12] Sun J S, Shi L L, Li S W, Li J J, Li X P, Zhang J S, Cheng L H, Chen B J 2016 Mater. Res. Bull. 80 102
Google Scholar
[13] 刘盛意, 张金苏, 孙佳石, 陈宝玖, 李香萍, 徐赛, 程丽红 2019 68 053301
Google Scholar
Liu S Y, Zhang J S, Sun J S, Chen B J, Li X P, Xu S, Cheng L H 2019 Acta Phys. Sin. 68 053301
Google Scholar
[14] 赵越, 杨帆, 孙佳石, 李香萍, 张金苏, 张希珍, 徐赛, 程丽红, 陈宝玖 2019 68 213301
Google Scholar
Zhao Y, Yang F, Sun J S, Li X P, Zhang J S, Zhang X Z, Xu S, Cheng L H, Chen B J 2019 Acta Phys. Sin. 68 213301
Google Scholar
[15] 孙佳石, 李香萍, 吴金磊, 李树伟, 石琳琳, 徐赛, 张金苏, 程丽红, 陈宝玖 2017 66 100201
Google Scholar
Sun J S, Li X P, Wu J L, Li S W, Shi L L, Xu S, Zhang J S, Cheng L H, Chen B J 2017 Acta Phys. Sin. 66 100201
Google Scholar
[16] 何为, 薛卫东, 唐斌 2012 优化试验设计方法及数据分析 (北京: 化学工业出版社) 第164—170页
He W, Xue W D, Tang B 2012 The Method of Opti-mal Design of Experiment and Data Analysis (Beijing: Chemical Industry Press) pp164–170 (in Chinese)
[17] 杨帆 2020 硕士学位论文 (大连: 大连海事大学)
Yang F 2020 M. S. Thesis (Dalian: Dalian Maritime University) (in Chinese)
[18] 任露泉 2009 回归设计及其优化 (北京: 科学出版社) 第12页
Ren L Q 2009 Regression Design and Optimization (Beijing: Science Press) p12 (in Chinese)
[19] He C, Yang K S, Liu L, Si Z J 2013 J. Rare Earths 31 790
Google Scholar
[20] Van U 1967 J. Electrochem. Soc. 114 1048
Google Scholar
[21] Tian Y, Chen B J, Hua R N, Yu N S, Liu B Q, Sun J S, Cheng L H, Zhong H Y, Li X P, Zhang J S, Tian B N, Zhong H 2012 CrystEngComm 14 1760
Google Scholar
[22] Tian B N, Chen B J, Tian Y, Sun J S, Li X P, Zhang J S, Zhong H Y, Cheng L H, Hua R N 2012 J. Phys. Chem. Solids 73 1314
Google Scholar
[23] Liu X L, Han K, Gu M, Xiao L H, Ni C, Huang S M, Liu B 2007 Solid State Commun. 142 680
Google Scholar
[24] 肖莉红, 顾牡, 刘小林, 张睿, 刘冰洁, 徐昕 2007 光谱学与光谱分析 27 1054
Google Scholar
Xiao L H, Gu M, Liu X L, Zhang R, Liu B J, Xu X 2007 Spectrosc. Spectral Anal. 27 1054
Google Scholar
[25] 傅尚怡, 顾牡, 刘小林, 倪晨, 刘波, 黄世明 2010 光谱学与光谱分析 30 2317
Google Scholar
Fu S Y, Gu M, Liu X L, Ni C, Liu B, Huang S M 2010 Spectrosc. Spectral Anal. 30 2317
Google Scholar
[26] Liu W J, Zhang W J, Liu R X, Li G J 2021 New J. Chem. 45 9818
Google Scholar
[27] Yu H Q, Jiang P P, Chen B J, Sun J S, Cheng L H, Li X P, Zhang J S, Xu S 2020 Appl. Phys. A 126 690
Google Scholar
[28] Jung K Y 2020 RSC Adv. 10 16323
Google Scholar
[29] 张洪杰, 苏锵 1988 应用化学 3 28
Zhang H J, Su Q 1988 Chin. J. Appl. Chem. 3 28
DownLoad:
Catalog
Metrics
- Abstract views: 4860
- PDF Downloads: 56
- Cited By: 0
