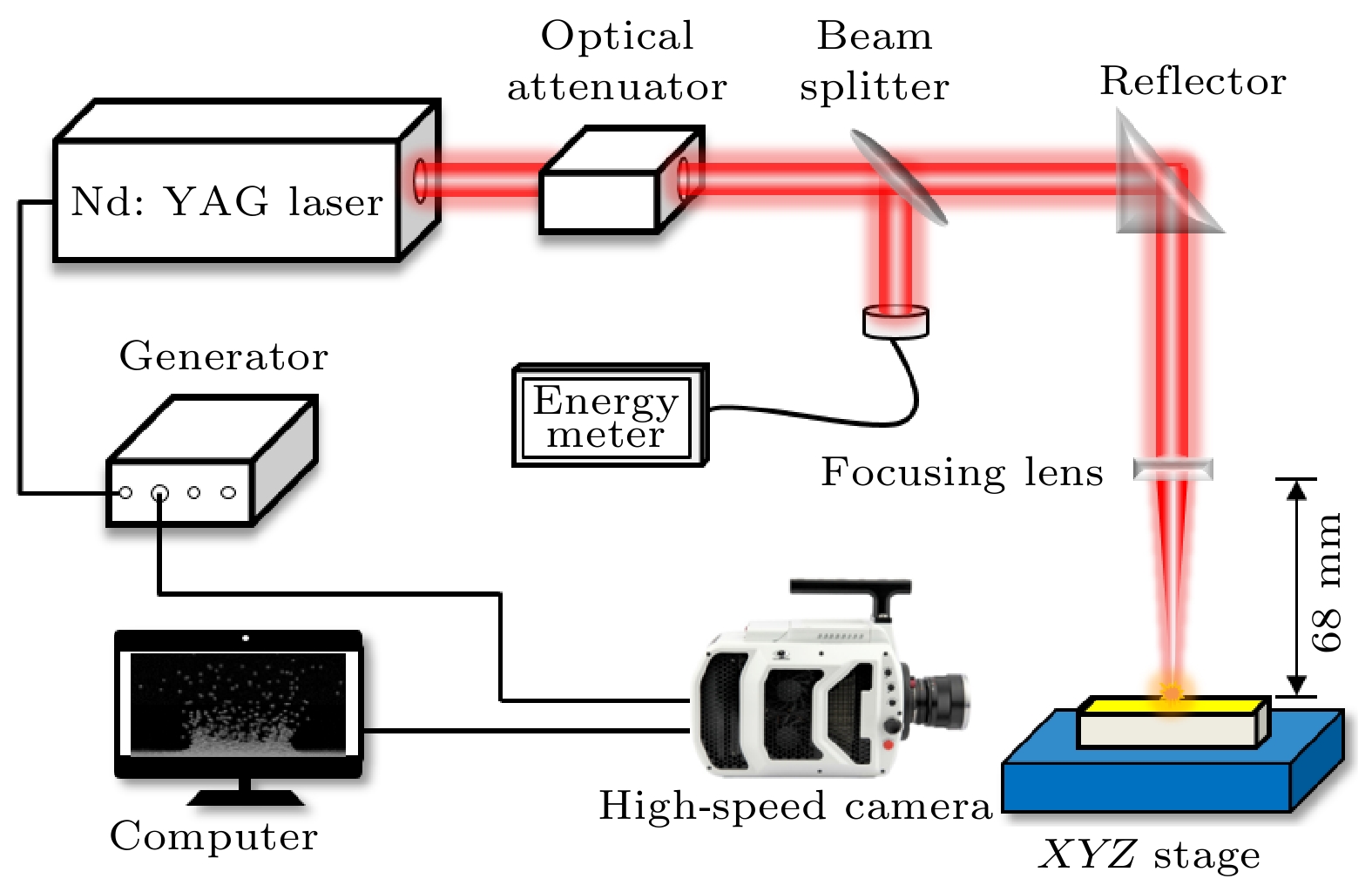
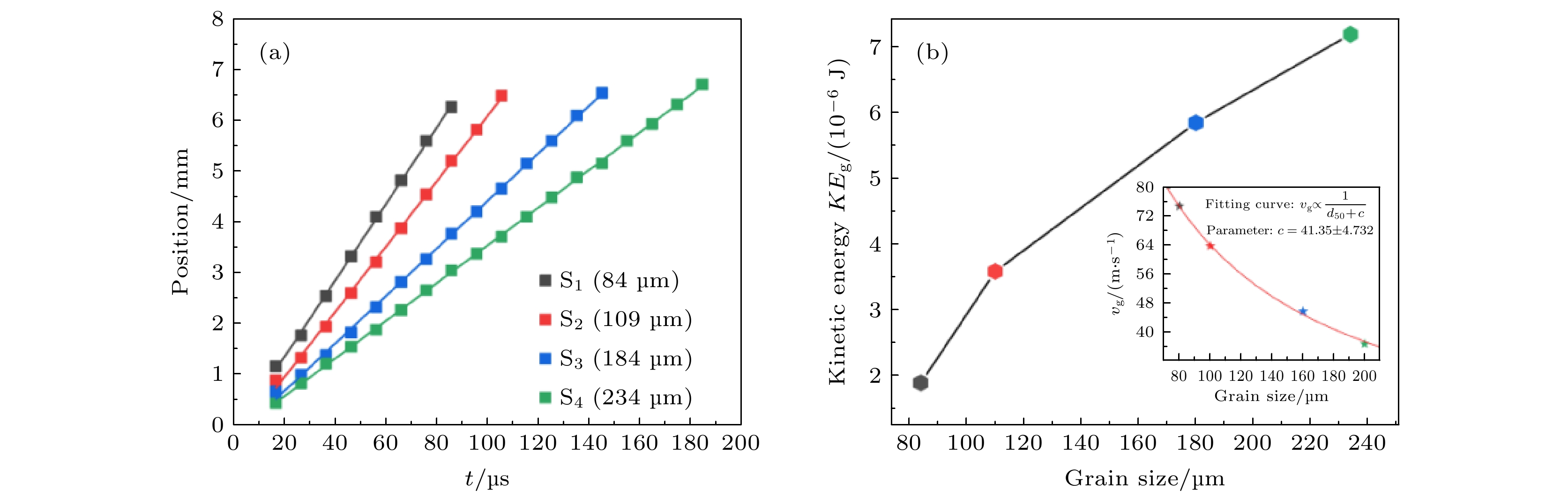
-
When intense laser pulse irradiates a target surface, the energetic processes of generation and expansion of laser-induced plasma will affect a localized pressure impulse around the irradiation zone. As a result, pulsed laser ablating granular target can drive a physical phenomenon of grain ejection. In this work, taking dry glass beads with different grain sizes as an example of granular targets and using high-speed video camera, we experimentally investigate the grain-size dependent dynamics of grain ejection driven by nanosecond laser pulses. The measured video sequences clearly exhibit that the laser-driven grain ejection process can be separated into two regimes: early-stage fast ejecting process and later-stage slow ejecting process. We find that there exists an obvious grain size effect on the kinetic energy of grains in the early-stage ejecting process. In addition, the temporal evolution of transient ejection of a curtain diameter
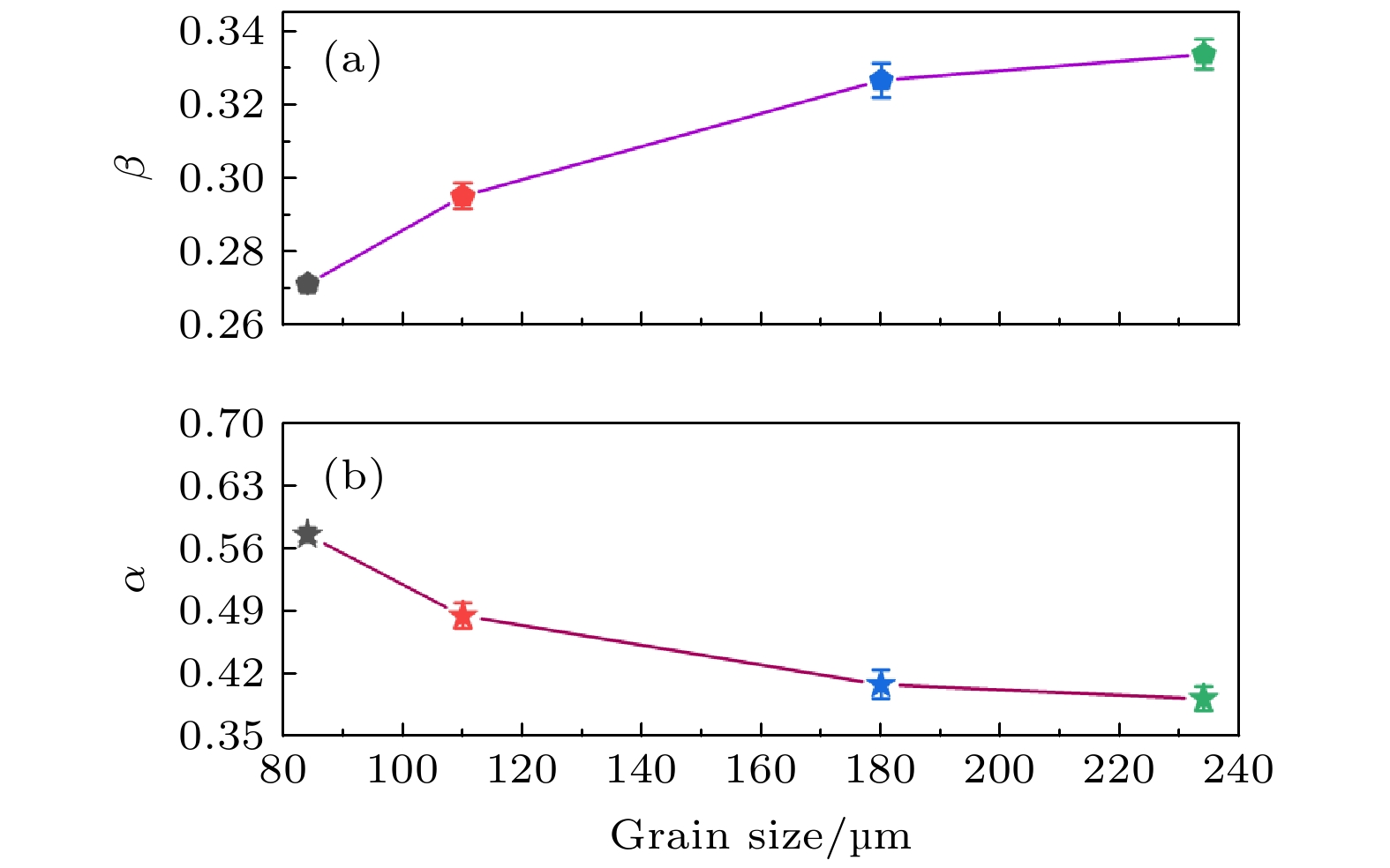
$ D\left(t\right) $ corresponding to the later-stage ejecting process obeys the well-known “point source” law,$ {D\left(t\right)=\alpha t}^{\beta } $ , where both parameters$ \alpha $ and$ \beta $ depend on grain size. The observations mentioned above can be reasonably explained by considering the grain size dependent efficiency of impulse coupling between grain and plasma flow and plasma features generated by interaction of laser pulse with granular targets. These experimental results improve the understanding of the mechanism of laser-driven grain ejection.-
Keywords:
- laser ablation /
- granular material /
- grain ejection /
- grain size
[1] Sedov L I 1946 Appl. Math. Mech. 10 241
[2] Schmidt R M, Housen K R 1987 Int. J. Impact Eng. 5 543
Google Scholar
[3] Holsapple K A, Schmidt R M 1979 Lunar Planet Sci. 10 2757
[4] Holsapple K A 1980 Lunar Planet Sci. 11 2379
[5] Gault D E, Wedekind J A 1977 Experimental Hypervelocity Impact into Quartz Sand-II, Effects of Gravitational Acceleration (New York: Impact & Explosion Cratering Planetary & Terrestrial Implications Proceedings of the Symposium on Planetary Cratering Mechanics) p1231
[6] Sedov L I 1959 Similarity and Dimensional Methods in Mechanics (4th Ed.) (New York: Academic Press) p377
[7] Holsapple K A, Schmidt R M 1987 J. Geophys. Res. 92 6350
Google Scholar
[8] Holsapple K A 1993 Annu. Rev. Earth Planet. Sci. 21 333
Google Scholar
[9] Amanda M, Walsh, Kristi E. Holloway, Piotr Habdas, John R, de Bruyn 2003 Phys. Rev. Lett. 91 104301
Google Scholar
[10] Uehara J S, Ambroso M A, Ojha R P 2003 Phys. Rev. Lett. 90 194301
Google Scholar
[11] Katsuragi H, Durian D J 2007 Nat. Phys. 3 420
Google Scholar
[12] Pacheco-Vazquez F, Ruiz-Suarez J C 2011 Phys. Rev. Lett. 107 218001
Google Scholar
[13] Ciamarra M P, Lara A H, Lee A T, Goldman D I, Vishik I, Swinney H L 2004 Phys. Rev. Lett. 92 194301
Google Scholar
[14] Lohse D, Bergmann R, Mikkelsen R, et al. 2004 Phys. Rev. Lett. 93 198003
Google Scholar
[15] Nordstrom K N, Lim E, Harrington M, Losert W 2014 Phys. Rev. Lett. 112 228002
Google Scholar
[16] Clark A H, Kondic L, Behringer R P 2012 Phys. Rev. Lett. 109 238302
Google Scholar
[17] Satoru Y, Koji W, Norihisa O, Takafumi M 2006 Icarus 183 215
Google Scholar
[18] Marston J O, Li E Q, Thoroddsen S T 2012 J. Fluid Mech. 704 5
Google Scholar
[19] Boudet J F, Amarouchene Y, Kellay H 2006 Phys. Rev. Lett. 96 158001
Google Scholar
[20] Deboeuf S, Gondret P, Rabaud M 2009 Phys. Rev. E 79 041306
Google Scholar
[21] Benusiglio A, Quéré D, Clanet C 2014 J. Fluid Mech. 752 123
Google Scholar
[22] Pacheco-Vázquez F, Tacumá A, Marston J O 2017 Phys. Rev. E 96 032904
Google Scholar
[23] Gao M, Liu X, Vanin L P, Sun T P, Cheng X 2018 AIChE J. 10 16063
Google Scholar
[24] Marston J O, Pacheco-Vázquez F 2019 Phys. Rev. E 99 030901
Google Scholar
[25] Bilger H R, Habib T 1985 Appl. Opt. 24 686
Google Scholar
[26] Goldman D I, Umbahnowar P 2008 Phys. Rev. E 77 021308
Google Scholar
[27] Li X L, Li Y J, Li S T, et al. 2021 Phys. Rev. Appl. 16 024017
Google Scholar
[28] Li Y J, Li X L, Li ST, Zhou MJ, Qian D B, Chen L W, Yang J, Zhang S F, Ma X W 2021 J. Anal. Atom. Spectrom. 36 1969
Google Scholar
[29] Yu L Y, Lu J D, Chen W, Wu G, Shen K, Feng W 2005 Plasma Sci. Technol. 7 3041
Google Scholar
-
图 1 筛分的石英砂颗粒样品S2和S4的扫描电镜图像
Figure 1. SEM images of the sieved glass beads taking S2 and S4 samples as examples.
图 2 实验装置示意图
Figure 2. Schematic of the experimental setup.
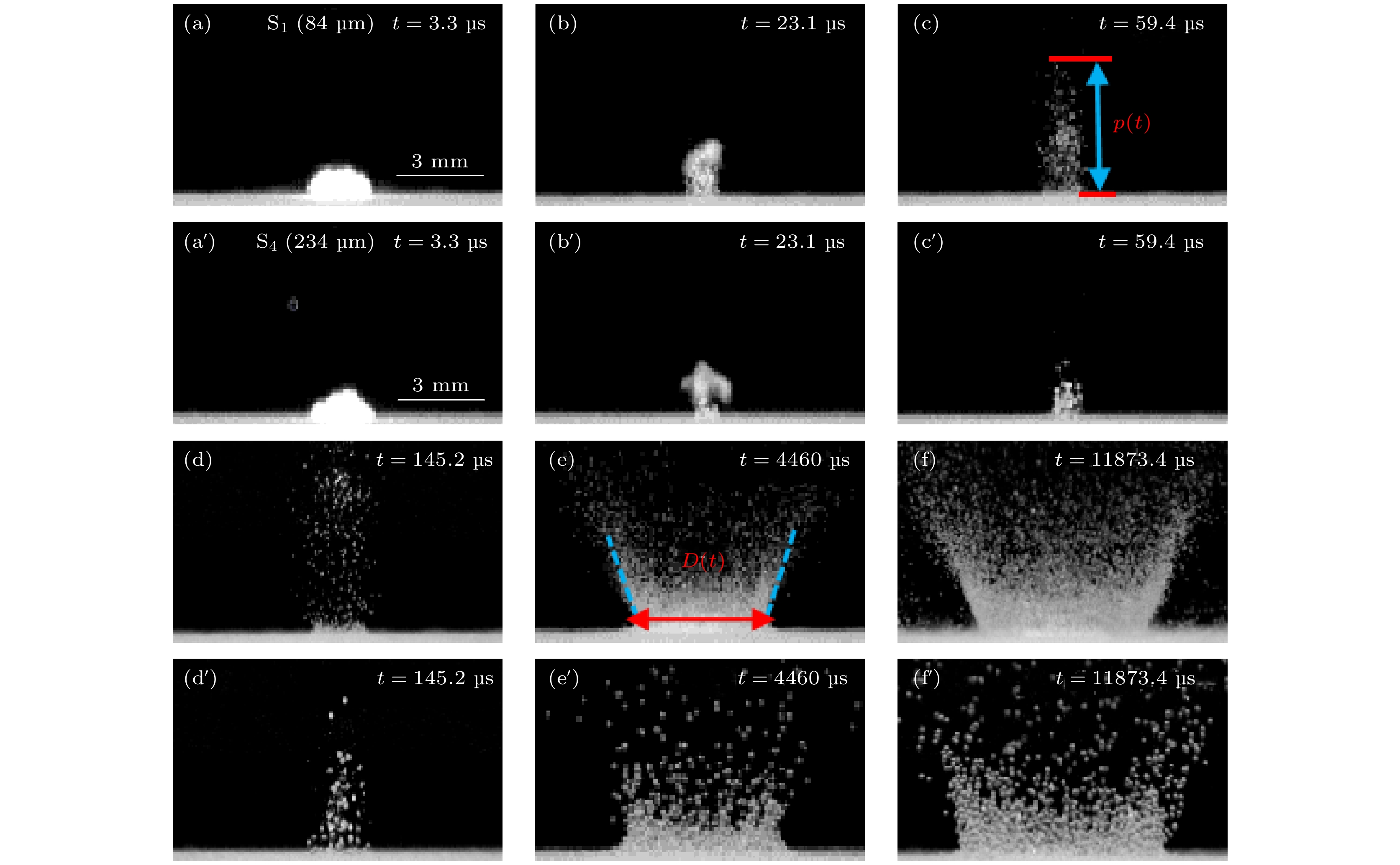
图 3 颗粒靶S1 (a)—(f)和S4 (a')—(f')对应的颗粒溅射时空影像. 图(c)中垂直于靶面的双箭头线段给出了早期颗粒溅射过程中最快颗粒位置的定义, 图(e)中平行于靶面的双箭头线段给出了对后期颗粒溅射过程中颗粒帘底直径的定义
Figure 3. Temporal and spatial images of grain ejection corresponding to granular targets S1 (a)–(f) and S4 (a')–(f'). The definitions for the fastest gain position in the early-stage ejecting process and the ejecta curtain diameter crossponding to the later-srage ejecting process are shown in panel (c) and panel (e), respectively.
图 4 (a)早期颗粒溅射过程中最快颗粒的位置随时间的依赖关系; (b)最快颗粒的动能随粒径的依赖关系
Figure 4. (a) Position of the fastest grain in the early-stage ejecting process as a function of time; (b) kinetic energy of the fastest particle as a function of grain size.
图 5 不同粒径的颗粒靶对应的后期颗粒溅射过程形成的颗粒帘底直径随时间的演化. 实线对应了采用点源模型方程
$ {D\left(t\right)=at}^{\beta } $ 拟合的结果. 插图展示了颗粒帘扩张速率随时间的演化Figure 5. Ejecta curtain diameter corresponding to the later-stage ejecting process as a function of time. The solid lines show the fitting results with the point source model. The inset exhibits the speed of expanding ejecta curtain with time.
图 6 不同粒径下的拟合参数 β 值和
$ \alpha $ 值Figure 6. Fitting parameters β and α at different grain sizes.
-
[1] Sedov L I 1946 Appl. Math. Mech. 10 241
[2] Schmidt R M, Housen K R 1987 Int. J. Impact Eng. 5 543
Google Scholar
[3] Holsapple K A, Schmidt R M 1979 Lunar Planet Sci. 10 2757
[4] Holsapple K A 1980 Lunar Planet Sci. 11 2379
[5] Gault D E, Wedekind J A 1977 Experimental Hypervelocity Impact into Quartz Sand-II, Effects of Gravitational Acceleration (New York: Impact & Explosion Cratering Planetary & Terrestrial Implications Proceedings of the Symposium on Planetary Cratering Mechanics) p1231
[6] Sedov L I 1959 Similarity and Dimensional Methods in Mechanics (4th Ed.) (New York: Academic Press) p377
[7] Holsapple K A, Schmidt R M 1987 J. Geophys. Res. 92 6350
Google Scholar
[8] Holsapple K A 1993 Annu. Rev. Earth Planet. Sci. 21 333
Google Scholar
[9] Amanda M, Walsh, Kristi E. Holloway, Piotr Habdas, John R, de Bruyn 2003 Phys. Rev. Lett. 91 104301
Google Scholar
[10] Uehara J S, Ambroso M A, Ojha R P 2003 Phys. Rev. Lett. 90 194301
Google Scholar
[11] Katsuragi H, Durian D J 2007 Nat. Phys. 3 420
Google Scholar
[12] Pacheco-Vazquez F, Ruiz-Suarez J C 2011 Phys. Rev. Lett. 107 218001
Google Scholar
[13] Ciamarra M P, Lara A H, Lee A T, Goldman D I, Vishik I, Swinney H L 2004 Phys. Rev. Lett. 92 194301
Google Scholar
[14] Lohse D, Bergmann R, Mikkelsen R, et al. 2004 Phys. Rev. Lett. 93 198003
Google Scholar
[15] Nordstrom K N, Lim E, Harrington M, Losert W 2014 Phys. Rev. Lett. 112 228002
Google Scholar
[16] Clark A H, Kondic L, Behringer R P 2012 Phys. Rev. Lett. 109 238302
Google Scholar
[17] Satoru Y, Koji W, Norihisa O, Takafumi M 2006 Icarus 183 215
Google Scholar
[18] Marston J O, Li E Q, Thoroddsen S T 2012 J. Fluid Mech. 704 5
Google Scholar
[19] Boudet J F, Amarouchene Y, Kellay H 2006 Phys. Rev. Lett. 96 158001
Google Scholar
[20] Deboeuf S, Gondret P, Rabaud M 2009 Phys. Rev. E 79 041306
Google Scholar
[21] Benusiglio A, Quéré D, Clanet C 2014 J. Fluid Mech. 752 123
Google Scholar
[22] Pacheco-Vázquez F, Tacumá A, Marston J O 2017 Phys. Rev. E 96 032904
Google Scholar
[23] Gao M, Liu X, Vanin L P, Sun T P, Cheng X 2018 AIChE J. 10 16063
Google Scholar
[24] Marston J O, Pacheco-Vázquez F 2019 Phys. Rev. E 99 030901
Google Scholar
[25] Bilger H R, Habib T 1985 Appl. Opt. 24 686
Google Scholar
[26] Goldman D I, Umbahnowar P 2008 Phys. Rev. E 77 021308
Google Scholar
[27] Li X L, Li Y J, Li S T, et al. 2021 Phys. Rev. Appl. 16 024017
Google Scholar
[28] Li Y J, Li X L, Li ST, Zhou MJ, Qian D B, Chen L W, Yang J, Zhang S F, Ma X W 2021 J. Anal. Atom. Spectrom. 36 1969
Google Scholar
[29] Yu L Y, Lu J D, Chen W, Wu G, Shen K, Feng W 2005 Plasma Sci. Technol. 7 3041
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4338
- PDF Downloads: 61
- Cited By: 0
