-
本文从双离子组分等离子体的Landau-Fokker-Planck方程出发, 通过Chapman-Enskog方法约化得到离子输运方程, 求得双离子组分等离子体的离子输运系数, 给出了计及离子扩散的完备离子流体方程; 再结合一维辐射流体力学程序Multi-1D模拟得到的烧蚀层的宏观状态, 研究了激光烧蚀固体碳氢材料时的组分分离现象. 计算结果显示, 离子组分分离对等离子体流体演化的影响小, 基本可以忽略; 但对于敏感依赖于离子组分的其他物理过程, 如汤姆孙散射, 离子组分分离的影响显著, 这意味着研究激光等离子体相互作用时, 离子组分分离必须予以考虑.Plasma usually consists of multiple ion component. Ion-component separation occurs in various conditions, and profoundly affects the plasma dynamic evolution. In this work, ion-component separation in two-ion-component plasma is investigated in the hydrodynamic condition. Starting from the Landau-Fokker-Planck equations of two-ion-component plasma, the ion transport equations are reduced through the Chapman-Enskog approach. The transport equations are then transformed into a set of linear algebraic equations and solved by expanding the perturbed ion distribution functions into the series of Sonine polynomials. The diffusive ion mass flows with inclusion of baro-diffusion, thermo-diffusion and electro-diffusion are thus obtained. With these efforts, the complete ion fluid equations are presented, which can be used to describe the processes of ion-component separation. We evaluate ion-component separation in the case of a solid CH plate target ablated with a laser pulse, by solving the ion diffusion equation with the hydro states output from the one-dimensional radiative hydro code Multi-1D. The simulation results show that ion-component separation mainly occurs around ablation front and under-dense region, and that the effect of ion-species separation on plasma hydrodynamic evolution is minor and can be neglected. For those physical processes sensitive to ion concentration such as Thomson scattering, however, the effect of ion-component separation is significant, which means that ion-component separation should be included in the study of laser plasma interaction.
-
Keywords:
- plasma transport processes /
- ion-species separation /
- laser ablation /
- radiative hydro simulation
[1] Atzeni S, Meyer-ter-Vehn J 2004 The Physics of Inertial Fusion (Vol. 125) (Oxford: Oxford University) pp38–41
[2] Zimmerman G, Kershaw D, Bailey D, Harte J 1978 J. Opt. Soc. Am. 68 549
[3] Marinak M M, Kerbel G D, Gentile N A, Jones O, Munro D, Pollaine S, Dittrich T R, Haan S W 2001 Phys. Plasmas 8 2275
Google Scholar
[4] Radha P B, Goncharov V N, Collins T J B, Delettrez J A, Elbaz Y, Glebov V Y, Keck R L, Keller D E, Knauer J P, Marozas J A, Marshall F J, McKenty P W, Meyerhofer D D, Regan S P, Sangster T C, Shvarts D, Skupsky S, Srebro Y, Town R P J, Stoeckl C 2005 Phys. Plasmas 12 032702
Google Scholar
[5] Song P, Zhai C, Li S, Yong H, Qi J, Hang X, Yang R, Cheng J, Zeng Q, Hu X, Wang S, Shi Y, Zheng W, Gu P, Zou S, Li X, Zhao Y, Zhang H, Zhang A, An H, Li J, Pei W, Zhu S 2015 High Power Laser Part. Beams 27 032007
Google Scholar
[6] Landau L D, Lifshitz E M 1987 Fluid Mechanics (Vol. 6) (Oxford: Pergamon) pp227–235
[7] Amendt P, Bellei C, Wilks S 2012 Phys. Rev. Lett. 109 075002
Google Scholar
[8] Kagan G, Tang X Z 2012 Phys. Plasmas 19 082709
Google Scholar
[9] Hicks D G, Meezan N B, Dewald E L, et al. 2012 Phys. Plasmas 19 122702
Google Scholar
[10] MacLaren S A, Schneider M B, Widmann K, Hammer J H, Yoxall B E, Moody J D, Bell P M, Benedetti L R, Bradley D K, Edwards M J, Guymer T M, Hinkel D E, Hsing W W, Kervin M L, Meezan N B, Moore A S, Ralph J E 2014 Phys. Rev. Lett. 112 105003
Google Scholar
[11] Kagan G, Tang X Z 2014 Phys. Lett. A 378 1531
Google Scholar
[12] Michel D T, Goncharov V N, Igumenshchev I V, Epstein R, Froula D H 2013 Phys. Rev. Lett. 111 245005
Google Scholar
[13] Clark D S, Weber C R, Kritcher A L, Milovich J L, Patel P K, Haan S W, Hammel B A, Koning J M, Marinak M M, Patel M V, Schroeder C R, Sepke S M, Edwards M J 2019 Nucl. Fusion 59 032008
Google Scholar
[14] Haberberger D, Shvydky A, Goncharov V N, Cao D, Carroll-Nellenback J, Hu S X, Ivancic S T, Karaseiv V V, Knauer J P, Maximov A V, Froula D H 2019 Phys. Rev. Lett. 123 235001
Google Scholar
[15] Zhang S, Hu S X 2020 Phys. Rev. Lett. 125 105001
Google Scholar
[16] Plimpton S 1995 J. Comput. Phys. 117 1
Google Scholar
[17] Ramis R, Meyer-ter-Vehn J 2016 Comput. Phys. Commun. 203 226
Google Scholar
[18] Byvank T, Langendorf S J, Thoma C, Hsu S C 2020 Phys. Plasmas 27 042302
Google Scholar
[19] Simakov A N, Keenan B D, Taitano W T, Chacon L 2017 Phys. Plasmas 24 092702
Google Scholar
[20] Chapmann S, Cowling T 1970 The Mathematical Theory of Non-Uniform Gases (Cambridge: Cambridge University)
[21] Braginskii S 1965 Rev. Plasma Phys. 1 205
[22] Molvig K, Simakov A N, Vold E L 2014 Phys. Plasmas 21 092709
Google Scholar
[23] Ross J S, Park H S, Amendt P, Divol L, Kugland N L, Rozmus W, Glenzer S H 2012 Rev. Sci. Instrum. 83 10e323
Google Scholar
[24] Stejner M, Nielsen S K, Bindslev H, Korsholm S B, Salewski M 2011 Plasma Phys. Control. Fusion 53 065020
Google Scholar
-
图 1 4种扩散系数和质量丰度c的关系 (a) CH; (b) DT
Fig. 1. Four diffusion coefficients as a function of mass concentration c for two materials: (a) CH; (b) DT.
图 2 动摩擦系数Aab随质量丰度c的函数
Fig. 2. Dynamic friction coefficient Aab as a function of mass concentration c for two materials.
图 3 (a) 1.6 ns时电子温度和离子温度随空间的分布; (b) 1.6 ns时电子压力和离子压力随空间的分布
Fig. 3. (a) Spatial distribution of electron temperature and ion temperature in 1.6 ns; (b) spatial distribution of electron pressure and ion pressure in 1.6 ns.
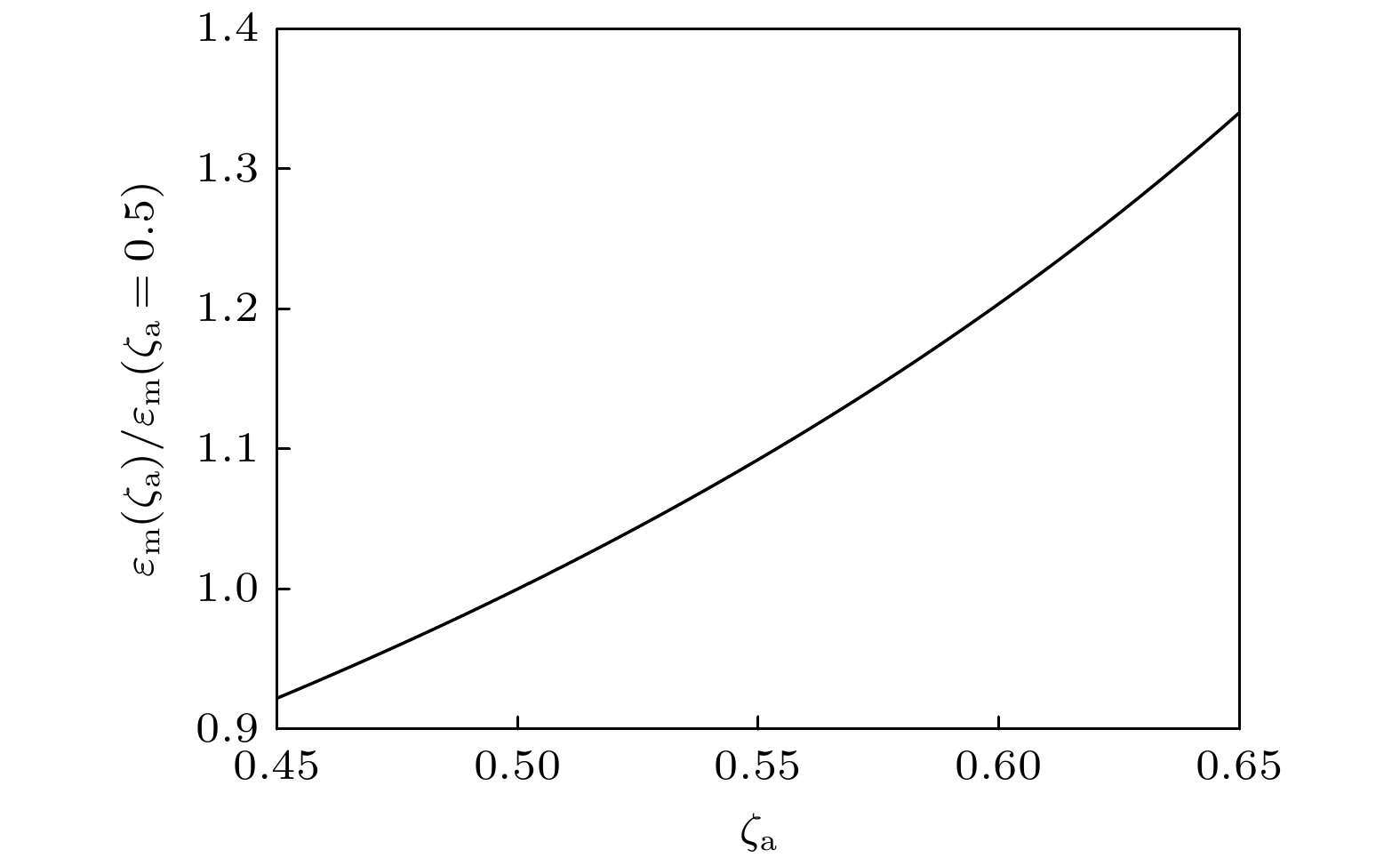
图 5 不同时刻下H离子粒子数丰度
${\zeta _a}$ 随空间的变化, 其中黑色虚线表示烧蚀面, 红色虚线表示临界密度面, 蓝色虚线表示1/4临界密度面, 黄色虚线表示1/10临界密度面 (a) 0.4 ns; (b) 0.8 ns; (c) 1.2 ns; (d) 1.6 nsFig. 5. The variation of H ion particle number abundance
${\zeta _a}$ with space at different times. Black dotted line indicates ablation front, red dotted line indicates critical density surface, blue dotted line indicates 1/4 critical density surface, yellow dotted line indicates 1/10 critical density surface: (a) 0.4 ns; (b) 0.8 ns; (c) 1.2 ns; (d) 1.6 ns.
图 6 电子-离子温度弛豫项随H离子粒子数丰度变化
Fig. 6. Variation of electron-ion thermal relaxation term with the abundance of H-ion particles.
图 7 离子比内能随H离子粒子数丰度变化图
Fig. 7. Variation of ion specific internal energy with the abundance of H-ion particles
图 8 2927 μm处离子声波在1.6 ns时对应的汤姆逊散射光谱
Fig. 8. Thomson scattering spectrum of ion acoustic wave at 2927 μm in 1.6 ns.
-
[1] Atzeni S, Meyer-ter-Vehn J 2004 The Physics of Inertial Fusion (Vol. 125) (Oxford: Oxford University) pp38–41
[2] Zimmerman G, Kershaw D, Bailey D, Harte J 1978 J. Opt. Soc. Am. 68 549
[3] Marinak M M, Kerbel G D, Gentile N A, Jones O, Munro D, Pollaine S, Dittrich T R, Haan S W 2001 Phys. Plasmas 8 2275
Google Scholar
[4] Radha P B, Goncharov V N, Collins T J B, Delettrez J A, Elbaz Y, Glebov V Y, Keck R L, Keller D E, Knauer J P, Marozas J A, Marshall F J, McKenty P W, Meyerhofer D D, Regan S P, Sangster T C, Shvarts D, Skupsky S, Srebro Y, Town R P J, Stoeckl C 2005 Phys. Plasmas 12 032702
Google Scholar
[5] Song P, Zhai C, Li S, Yong H, Qi J, Hang X, Yang R, Cheng J, Zeng Q, Hu X, Wang S, Shi Y, Zheng W, Gu P, Zou S, Li X, Zhao Y, Zhang H, Zhang A, An H, Li J, Pei W, Zhu S 2015 High Power Laser Part. Beams 27 032007
Google Scholar
[6] Landau L D, Lifshitz E M 1987 Fluid Mechanics (Vol. 6) (Oxford: Pergamon) pp227–235
[7] Amendt P, Bellei C, Wilks S 2012 Phys. Rev. Lett. 109 075002
Google Scholar
[8] Kagan G, Tang X Z 2012 Phys. Plasmas 19 082709
Google Scholar
[9] Hicks D G, Meezan N B, Dewald E L, et al. 2012 Phys. Plasmas 19 122702
Google Scholar
[10] MacLaren S A, Schneider M B, Widmann K, Hammer J H, Yoxall B E, Moody J D, Bell P M, Benedetti L R, Bradley D K, Edwards M J, Guymer T M, Hinkel D E, Hsing W W, Kervin M L, Meezan N B, Moore A S, Ralph J E 2014 Phys. Rev. Lett. 112 105003
Google Scholar
[11] Kagan G, Tang X Z 2014 Phys. Lett. A 378 1531
Google Scholar
[12] Michel D T, Goncharov V N, Igumenshchev I V, Epstein R, Froula D H 2013 Phys. Rev. Lett. 111 245005
Google Scholar
[13] Clark D S, Weber C R, Kritcher A L, Milovich J L, Patel P K, Haan S W, Hammel B A, Koning J M, Marinak M M, Patel M V, Schroeder C R, Sepke S M, Edwards M J 2019 Nucl. Fusion 59 032008
Google Scholar
[14] Haberberger D, Shvydky A, Goncharov V N, Cao D, Carroll-Nellenback J, Hu S X, Ivancic S T, Karaseiv V V, Knauer J P, Maximov A V, Froula D H 2019 Phys. Rev. Lett. 123 235001
Google Scholar
[15] Zhang S, Hu S X 2020 Phys. Rev. Lett. 125 105001
Google Scholar
[16] Plimpton S 1995 J. Comput. Phys. 117 1
Google Scholar
[17] Ramis R, Meyer-ter-Vehn J 2016 Comput. Phys. Commun. 203 226
Google Scholar
[18] Byvank T, Langendorf S J, Thoma C, Hsu S C 2020 Phys. Plasmas 27 042302
Google Scholar
[19] Simakov A N, Keenan B D, Taitano W T, Chacon L 2017 Phys. Plasmas 24 092702
Google Scholar
[20] Chapmann S, Cowling T 1970 The Mathematical Theory of Non-Uniform Gases (Cambridge: Cambridge University)
[21] Braginskii S 1965 Rev. Plasma Phys. 1 205
[22] Molvig K, Simakov A N, Vold E L 2014 Phys. Plasmas 21 092709
Google Scholar
[23] Ross J S, Park H S, Amendt P, Divol L, Kugland N L, Rozmus W, Glenzer S H 2012 Rev. Sci. Instrum. 83 10e323
Google Scholar
[24] Stejner M, Nielsen S K, Bindslev H, Korsholm S B, Salewski M 2011 Plasma Phys. Control. Fusion 53 065020
Google Scholar
下载:
计量
- 文章访问数: 3125
- PDF下载量: 68
- 被引次数: 0
