Analysis of trapping force exerted on multi-layered chiral sphere induced by laser sheet

Bai Jing; Ge Cheng-Xian; He Lang; Liu Xuan; Wu Zhen-Sen

doi:10.7498/aps.71.20212284

Abstract

Theoretical study on optical trapping of multi-layered chiral sphere has attracted more and more attention for its important applications in many frontier scientific fields such as chemical engineering, biomedicine, optical tweezers, micro/nano lithography etc. In order to trap and manipulate chiral multi-layered particles efficiently, the present paper aims at developing the theoretical research of trapping force (TF) exerted on a multi-layered chiral sphere induced by laser sheet which might have great potential to improve the light performance in optical trapping as well as capture, suspension, and high-precision delivery of chiral cells. Here, based on the Generalized Lorenz Mie theory and the completeness of spherical vector wave functions (SVWFs), the electromagnetic field of incident laser sheet are expanded in terms of SVWFs. Accordingly, by introducing the beam scattering theory and the conservation law of electromagnetic momentum (EM), the analysis of TF exerted on multi-layered chiral sphere can be analytically expressed in terms of the incident and scattering coefficients. Taking the chiral cell as an example, the TF induced by laser sheet is simulated numerically. Numerical effects of the varying chirality, polarization states, beam waist width, inner material loss and outmost size on the TF induced by laser sheet are analyzed and compared with those by circular Gaussian beam incidence in detail. It is found that the introduction of chirality parameter may reduce the axial TF exerted on chiral multi-layered cell. Thus, it is more difficult to trap and manipulate stratified chiral cells than to trap general isotropic cells. Also it is shown that the TF of chiral cells can be significantly discriminatory in nature, depending upon both the handedness of the interacting particles and the polarization of the incident light. Thus, an appropriately polarized beam should be considered in trapping chiral cells. For chiral multi-layered cells with small loss in the inner layer, when the inner refractive indices are less than the outmost refractive index, the TF of multi-layered chiral cell becomes stronger with the outmost radius decreasing. Conversely, for the inner refractive indices are greater than the outer refractive index, TF becomes weaker as the outmost radius decreases. Besides, compared with the traditional circular Gaussian beam, the strong convergence of elliptical Gaussian beam can be easier to achieve three-dimensional capture of stratified chiral cells, which may provide a recipe to understand the light interaction with more complex chiral cells with the aid of the analytical approach and could be a promising avenue for the design of optical trapping systems.

Keywords:

Authors and contacts

1.
School of Electronic Engineering, Xi’an University of Posts & Telecommunications, Xi’an 710121, China
2.
The 39 th Research Institute of China Electronics Technology Corporation, Xi’an 710065, China
3.
School of Physics and Optoelectronic Engineering, Xidian University, Xi’an 710071, China

Corresponding author: Bai Jing, jbaiyoudian@163.com

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 62001377).

FullText HTML : translate this paragraph

References

[1]	Ashkin A 1970 Phys. Rev. Lett. 24 156 Google Scholar
[2]	Ashkin A 1980 Science 210 1081 Google Scholar
[3]	蒋云峰, 陆璇辉, 赵承良 2010 59 3959 Google Scholar Jiang Y F, Lu X H, Zhao C L 2010 Acta Phys. Sin. 59 3959 Google Scholar
[4]	吴鹏, 韩一平, 刘德芳 2005 54 2676 Google Scholar Wu P, Han Y P, Liu D F 2005 Acta Phys. Sin. 54 2676 Google Scholar
[5]	Ren K F, Gréha G, Gouesbet G 1994 Opt. Commun. 108 343 Google Scholar
[6]	Lock J A 2004 Endocrinology 43 2532
[7]	Gouesbet G, Lock J A 1994 J. Opt. Soc. Am. A: 11 2503
[8]	Ren K F, Gouesbet G, Gréha G 1998 Appl. Opt. 37 4218 Google Scholar
[9]	韩一平, 杜云刚, 张华永 2006 55 4557 Google Scholar Han Y P, Du Y G, Zhang H Y 2006 Acta Phys. Sin. 55 4557 Google Scholar
[10]	韩国霞, 韩一平 2009 58 6167 Google Scholar Han G X, Han Y P 2009 Acta Phys. Sin. 58 6167 Google Scholar
[11]	Onofri F, Gréha G, Gouesbet G 1995 Appl. Opt. 34 7113 Google Scholar
[12]	Li H Y, Wu Z S, Li Z J 2009 Chin. Phys. Lett. 26 104203 Google Scholar
[13]	Ladutenko K, Pal U, Rivera A, Rodríguez O 2007 Comput. Phys. Commun. 214 225
[14]	Pei S, Pan Q, Cui F, Xu S S, Cao Z L 2018 Optik 180 379
[15]	Bohren G F, Huffman D R 1983 Absorption and Scattering of Light by Small Particles (New York: Wiley)
[16]	Kerker M 1969 The Scattering of Light and Other Electromagnetic Radiation (New York: Academic)
[17]	Wu Z S, Wang Y P 1991 Radio Sci. 26 1393 Google Scholar
[18]	李海英, 吴振森 2008 57 833 Google Scholar Li H Y, Wu Z S 2008 Acta Phys. Sin. 57 833 Google Scholar
[19]	Chen Z Y, Han Y P, Cui Z W, Shi X W 2015 Opt. Commun. 340 5 Google Scholar
[20]	Yu M P, Han Y P, Cui Z W, Sun H Y 2018 J. Opt. Soc. Am. A 35 1504
[21]	Shore R A 2015 IEEE Antennas Propag. Mag. 57 69
[22]	Wang H B, Liu X Z, Gao S, Cui J, Liu H J, He A J, Zhang G T 2018 Chin. Phys. B 27 034302 Google Scholar
[23]	Schut T C B, Hesselink G, de Grooth B G, Greve J 1991 Cytomety 12 479 Google Scholar
[24]	Rohrbach A, Stelzer E 2001 J. Opt. Soc. Am. A: 18 839
[25]	Ermutlu M E, Sihvola A H 1994 Prog. Electromagnet. Res. 9 87 Google Scholar
[26]	Ren W 1994 Prog. Electromagnet. Res. 9 103 Google Scholar
[27]	Simpson S H, Hanna S 2011 Phys. Rev. A 84 053808 Google Scholar
[28]	Cooray M F R, Ciric I R 1993 J. Opt. Soc. Am. A: 10 1197
[29]	Jaggard D L, Liu J C 1999 IEEE Trans. Antennas Propag. 47 1201 Google Scholar
[30]	Yan B, Liu C H, Zhang H Y, Shi Y 2015 Opt. Commun. 338 261 Google Scholar
[31]	Wang W J, Sun Y F, Zhang H Y 2017 Opt. Commun. 385 54 Google Scholar
[32]	Gao X, Zhang H 2017 Optik 129 43 Google Scholar
[33]	Zheng M, Zhang H Y, Sun Y F, Wang Z G 2015 J. Quant. Spectrosc. Radiat. Transfer 151 192 Google Scholar
[34]	Li L W, Dan Y, Leong M, et al. 1999 Prog. Electromagnet. Res. 23 1203
[35]	Shang Q, Wu Z, Qu T, Li Z, Bai L 2016 J. Quant. Spectrosc. Radiat. Transfer 173 72 Google Scholar
[36]	Ren K F, Grehan G, Gouesbet G 1994 J. Opt. 25 165 Google Scholar
[37]	Ren K F, Gérard G 1993 Part. Part. Syst. Char. 10 146 Google Scholar
[38]	Naqwi A A, Liu X Z, Durst F 1992 Part. Part. Syst. Char. 9 44 Google Scholar
[39]	Naqwi A A, Liu X Z, Franz D 1990 Part. Part. Syst. Char. 7 45 Google Scholar
[40]	Rockwell D, Magness C, Towfighi J, Akin O, Corcoran T 1993 Exp. Fluids 14 181 Google Scholar
[41]	Adrian R J 1984 Appl. Opt. 23 1690 Google Scholar
[42]	Gréhan D G, Gouesbet G, Naqwi D A, Durst F 1993 Part. Part. Syst. Char. 10 332 Google Scholar
[43]	Doicu D I A, Ebert D I F, Schabel D I S 1996 Part. Part. Syst. Char. 13 79 Google Scholar
[44]	郭红莲, 曹勤红, 任东涛, 刘国琴, 段建发, 李兆霖, 张道中, 韩学海 2003 科学通报 48 6 Guo H L, Cao Q H, Ren D T, Liu G Q, Duan J F, Li Z L, Zhang D Z, Han X M 2003 Chin. Sci. Bull. 48 6
[45]	Wang W, Shen J Q 2018 J. Quant. Spectrosc. Radiat. Transfer 212 139 Google Scholar
[46]	Shen J Q, Liu X, Wang W, Yu H T 2018 J. Opt. Soc. Am. A. 35 8 Google Scholar
[47]	李应乐, 李瑾, 王明军, 董群峰 2014 中国科学: 物理学力学天文学 5 7 Li Y L, Li Q, Wang M J, Dong Q F 2014 Sci. Chin. -Phys. Mech. Astron. 5 7
[48]	李应乐, 李瑾, 王明军, 董群峰 2013 激光与光电子学进展 50 6 Li Y L, Li Q, Wang M J, Dong Q F 2013 Laser Optoelectron. Prog. 50 6
[49]	Ma N Z, Li R X 2010 International Symposium on Antennas Propagation & Em Theory Guangzhou, Novenber 29–December 2, 2010 p646
[50]	Li R X, Ren K F, Han X 2013 J. Quant. Spectrosc. Radiat. Transfer 126 69 Google Scholar
[51]	Ren K F, Gréhan G, Gouesbet G 1994 J. Opt. Soc. Am. A 11 2072
[52]	Gouesbet G, Grehan G, Maheu B 1988 Appl. Opt. 27 4874 Google Scholar
[53]	Barton J P, Alexander D R, Schaub S A 1989 J. Appl. Phys. 66 4594 Google Scholar
[54]	Rohrbach A, Stelzer E H K 2000 J. Opt. Soc. Am. A 18 839
[55]	Harada Y, Asakura T 1996 Opt. Commun. 124 529 Google Scholar
[56]	Schut T C, Hesselink G, Grooth B G 1991 Cytometry 12 479 Google Scholar
[57]	Nemoto, Togo H 1998 Appl. Opt. 37 6386 Google Scholar
[58]	Nahmias Y K, Gao B Z, Odde D J 2004 Appl. Opt. 43 3999 Google Scholar
[59]	Drezek R, Dunn A, Richards-Kortum R 1999 Appl. Opt. 38 3651 Google Scholar

Cited By

图 1 非均匀手征分层球对椭圆高斯波束散射图

Figure 1. Geometry for scattering of a non-uniform multi-layered chiral sphere induced by laser sheet.

DownLoad: Full-Size Img PowerPoint

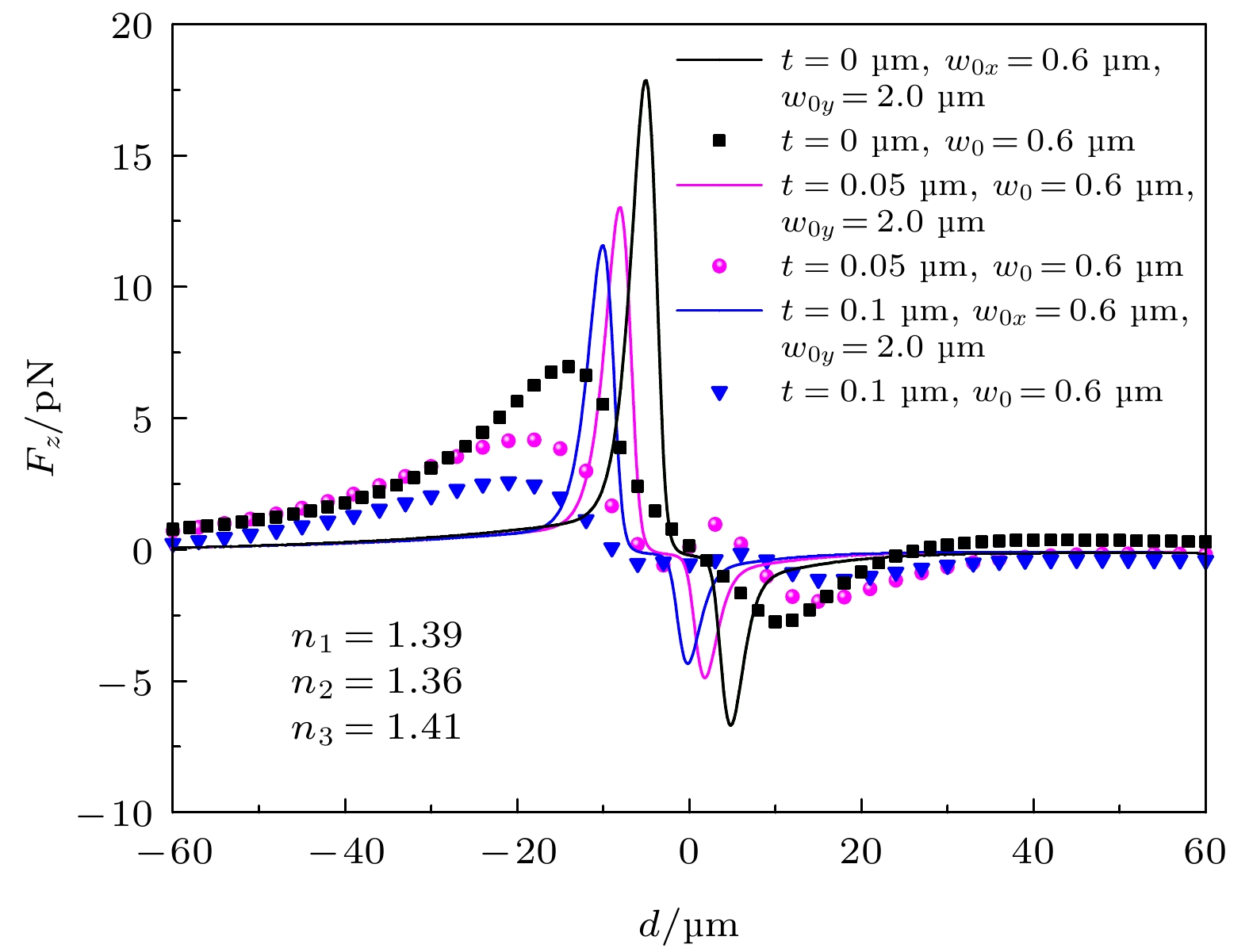
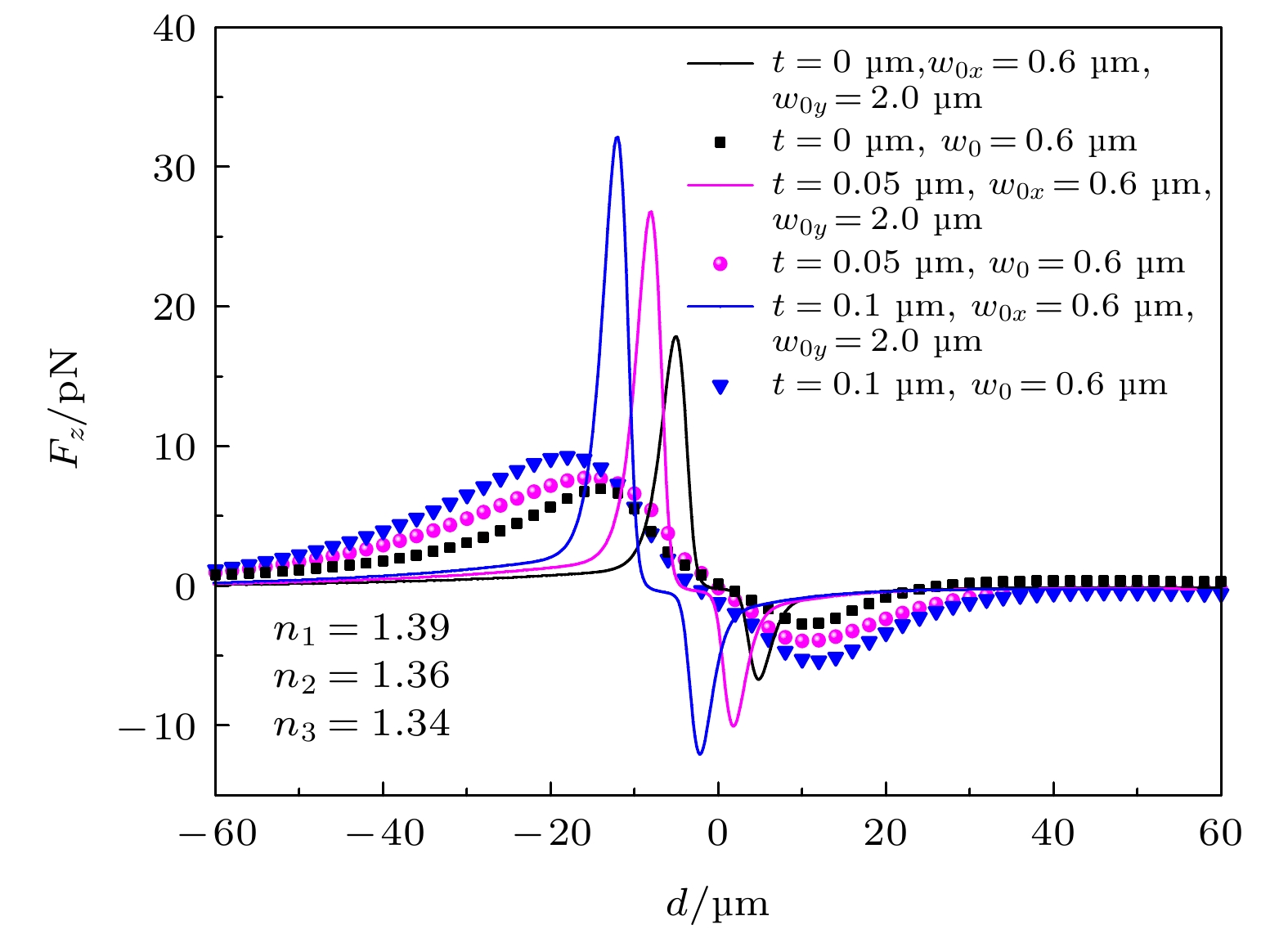
图 2 手征多层球退化为各向同性多层球的辐射俘获力与实验及文献结果进行对比　(a) 单层球对比轴向俘获力${F_z}$; (b) 双层球对比轴向俘获力${F_z}$; (c)五层球对比横向俘获力截面${C_{{\text{pr}}, x}}$

Figure 2. Comparisons of trapping force (TF) from the theory when multi-layered chiral sphere is degenerated into stratified isotropic sphere with the results from existing references and experiments: (a) Comparisons of axial TF${F_z}$on a single-layered sphere; (b) comparisons of axial TF${F_z}$on a double-layered sphere; (c) comparisons of transverse TF cross section${C_{{\text{pr}}, x}}$on a five-layered sphere.

DownLoad: Full-Size Img PowerPoint

图 3 不同手征参数对轴向俘获力${F_z}$随粒子离轴位置$d$变化的影响

Figure 3. Effects of chirality parameter on axial TF with the varying position$d$of the chiral cell off axis.

DownLoad: Full-Size Img PowerPoint

图 4 不同极化状态对轴向俘获力${F_z}$随粒子离轴位置$d$变化的影响

Figure 4. Effects of polarization states on axial TF with the varying position$d$of the chiral cell off axis.

DownLoad: Full-Size Img PowerPoint

图 5 不同束腰半径对轴向俘获力${F_z}$随粒子离轴位置$d$变化的影响

Figure 5. Effects of beam waist widths on axial TF with the varying position$d$of the chiral cell off axis.

DownLoad: Full-Size Img PowerPoint

图 6 内层损耗变化对轴向俘获力${F_z}$随粒子离轴位置$d$变化的影响

Figure 6. Effects of inner material loss on axial TF with the varying position$d$of the chiral cell off axis.

DownLoad: Full-Size Img PowerPoint

图 7 最外层厚度变化对轴向俘获力${F_z}$随粒子离轴位置$d$变化的影响(内层及次外层折射率小于最外层时)

Figure 7. Effects of outmost particle size on axial TF with the varying position$d$of the chiral cell off axis (the inner refractive index is less than the outmost refractive index case).

DownLoad: Full-Size Img PowerPoint

图 8 最外层厚度变化对轴向俘获力${F_z}$随粒子离轴位置$d$变化的影响(内层及次外层折射率大于最外层时)

Figure 8. Effects of the outmost particle size on axial TF with the varying position$d$of the chiral cell off axis (the inner refractive index is greater than the outmost refractive index case).

DownLoad: Full-Size Img PowerPoint

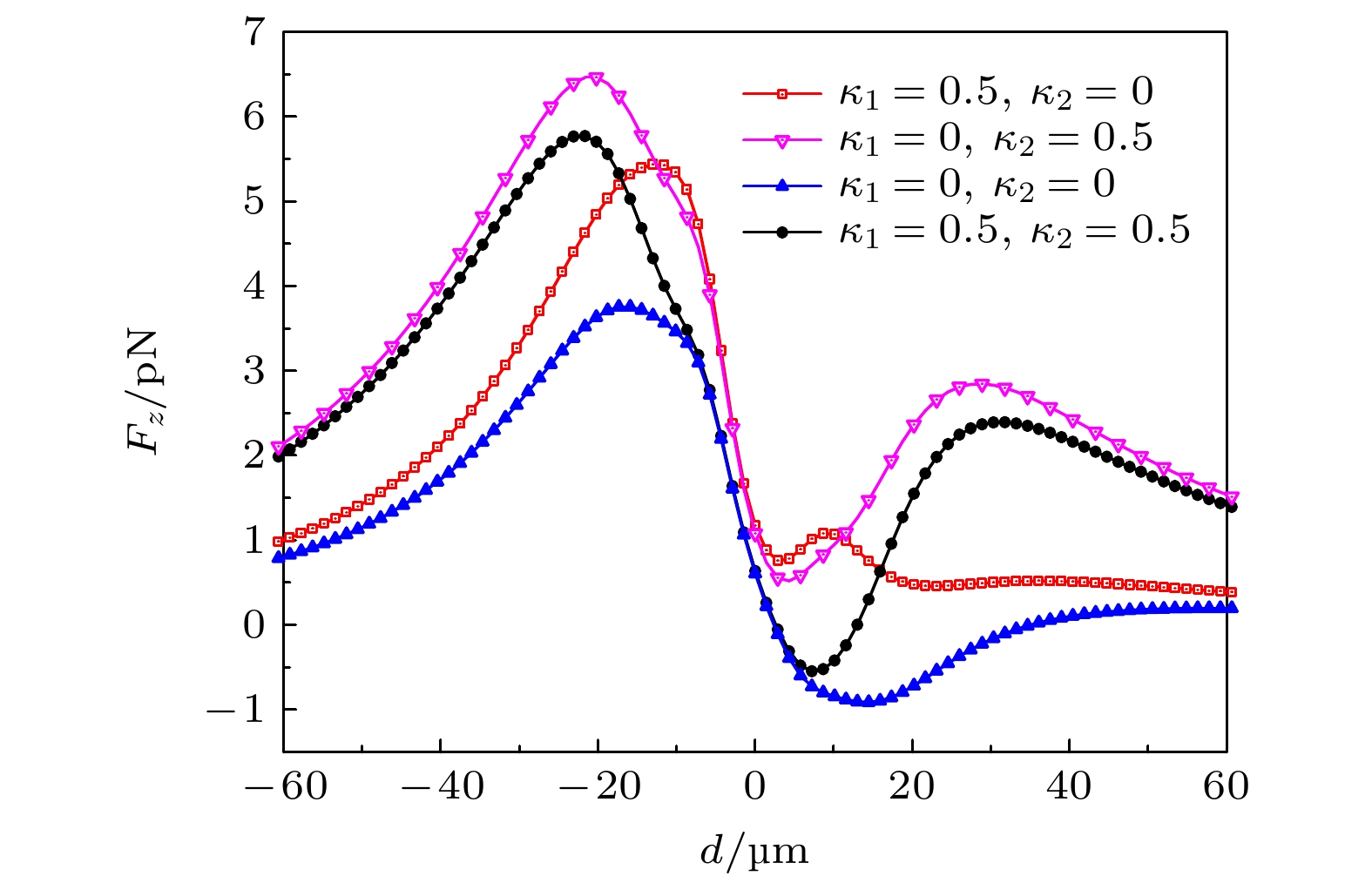
图 9 不同束腰半径对横向俘获力随粒子离轴位置$d$变化的影响 (a) ${F_x}$随粒子离轴位置$d$变化; (b) ${F_y}$随粒子离轴位置$d$变化

Figure 9. Effects of beam waist width on transverse TF with the varying position$d$of the chiral cell off axis: (a) ${F_x}$changes with the varying position$d$off axis; (b) ${F_y}$changes with the varying position$d$off axis.

DownLoad: Full-Size Img PowerPoint

map

[1]	Ashkin A 1970 Phys. Rev. Lett. 24 156 Google Scholar
[2]	Ashkin A 1980 Science 210 1081 Google Scholar
[3]	蒋云峰, 陆璇辉, 赵承良 2010 59 3959 Google Scholar Jiang Y F, Lu X H, Zhao C L 2010 Acta Phys. Sin. 59 3959 Google Scholar
[4]	吴鹏, 韩一平, 刘德芳 2005 54 2676 Google Scholar Wu P, Han Y P, Liu D F 2005 Acta Phys. Sin. 54 2676 Google Scholar
[5]	Ren K F, Gréha G, Gouesbet G 1994 Opt. Commun. 108 343 Google Scholar
[6]	Lock J A 2004 Endocrinology 43 2532
[7]	Gouesbet G, Lock J A 1994 J. Opt. Soc. Am. A: 11 2503
[8]	Ren K F, Gouesbet G, Gréha G 1998 Appl. Opt. 37 4218 Google Scholar
[9]	韩一平, 杜云刚, 张华永 2006 55 4557 Google Scholar Han Y P, Du Y G, Zhang H Y 2006 Acta Phys. Sin. 55 4557 Google Scholar
[10]	韩国霞, 韩一平 2009 58 6167 Google Scholar Han G X, Han Y P 2009 Acta Phys. Sin. 58 6167 Google Scholar
[11]	Onofri F, Gréha G, Gouesbet G 1995 Appl. Opt. 34 7113 Google Scholar
[12]	Li H Y, Wu Z S, Li Z J 2009 Chin. Phys. Lett. 26 104203 Google Scholar
[13]	Ladutenko K, Pal U, Rivera A, Rodríguez O 2007 Comput. Phys. Commun. 214 225
[14]	Pei S, Pan Q, Cui F, Xu S S, Cao Z L 2018 Optik 180 379
[15]	Bohren G F, Huffman D R 1983 Absorption and Scattering of Light by Small Particles (New York: Wiley)
[16]	Kerker M 1969 The Scattering of Light and Other Electromagnetic Radiation (New York: Academic)
[17]	Wu Z S, Wang Y P 1991 Radio Sci. 26 1393 Google Scholar
[18]	李海英, 吴振森 2008 57 833 Google Scholar Li H Y, Wu Z S 2008 Acta Phys. Sin. 57 833 Google Scholar
[19]	Chen Z Y, Han Y P, Cui Z W, Shi X W 2015 Opt. Commun. 340 5 Google Scholar
[20]	Yu M P, Han Y P, Cui Z W, Sun H Y 2018 J. Opt. Soc. Am. A 35 1504
[21]	Shore R A 2015 IEEE Antennas Propag. Mag. 57 69
[22]	Wang H B, Liu X Z, Gao S, Cui J, Liu H J, He A J, Zhang G T 2018 Chin. Phys. B 27 034302 Google Scholar
[23]	Schut T C B, Hesselink G, de Grooth B G, Greve J 1991 Cytomety 12 479 Google Scholar
[24]	Rohrbach A, Stelzer E 2001 J. Opt. Soc. Am. A: 18 839
[25]	Ermutlu M E, Sihvola A H 1994 Prog. Electromagnet. Res. 9 87 Google Scholar
[26]	Ren W 1994 Prog. Electromagnet. Res. 9 103 Google Scholar
[27]	Simpson S H, Hanna S 2011 Phys. Rev. A 84 053808 Google Scholar
[28]	Cooray M F R, Ciric I R 1993 J. Opt. Soc. Am. A: 10 1197
[29]	Jaggard D L, Liu J C 1999 IEEE Trans. Antennas Propag. 47 1201 Google Scholar
[30]	Yan B, Liu C H, Zhang H Y, Shi Y 2015 Opt. Commun. 338 261 Google Scholar
[31]	Wang W J, Sun Y F, Zhang H Y 2017 Opt. Commun. 385 54 Google Scholar
[32]	Gao X, Zhang H 2017 Optik 129 43 Google Scholar
[33]	Zheng M, Zhang H Y, Sun Y F, Wang Z G 2015 J. Quant. Spectrosc. Radiat. Transfer 151 192 Google Scholar
[34]	Li L W, Dan Y, Leong M, et al. 1999 Prog. Electromagnet. Res. 23 1203
[35]	Shang Q, Wu Z, Qu T, Li Z, Bai L 2016 J. Quant. Spectrosc. Radiat. Transfer 173 72 Google Scholar
[36]	Ren K F, Grehan G, Gouesbet G 1994 J. Opt. 25 165 Google Scholar
[37]	Ren K F, Gérard G 1993 Part. Part. Syst. Char. 10 146 Google Scholar
[38]	Naqwi A A, Liu X Z, Durst F 1992 Part. Part. Syst. Char. 9 44 Google Scholar
[39]	Naqwi A A, Liu X Z, Franz D 1990 Part. Part. Syst. Char. 7 45 Google Scholar
[40]	Rockwell D, Magness C, Towfighi J, Akin O, Corcoran T 1993 Exp. Fluids 14 181 Google Scholar
[41]	Adrian R J 1984 Appl. Opt. 23 1690 Google Scholar
[42]	Gréhan D G, Gouesbet G, Naqwi D A, Durst F 1993 Part. Part. Syst. Char. 10 332 Google Scholar
[43]	Doicu D I A, Ebert D I F, Schabel D I S 1996 Part. Part. Syst. Char. 13 79 Google Scholar
[44]	郭红莲, 曹勤红, 任东涛, 刘国琴, 段建发, 李兆霖, 张道中, 韩学海 2003 科学通报 48 6 Guo H L, Cao Q H, Ren D T, Liu G Q, Duan J F, Li Z L, Zhang D Z, Han X M 2003 Chin. Sci. Bull. 48 6
[45]	Wang W, Shen J Q 2018 J. Quant. Spectrosc. Radiat. Transfer 212 139 Google Scholar
[46]	Shen J Q, Liu X, Wang W, Yu H T 2018 J. Opt. Soc. Am. A. 35 8 Google Scholar
[47]	李应乐, 李瑾, 王明军, 董群峰 2014 中国科学: 物理学力学天文学 5 7 Li Y L, Li Q, Wang M J, Dong Q F 2014 Sci. Chin. -Phys. Mech. Astron. 5 7
[48]	李应乐, 李瑾, 王明军, 董群峰 2013 激光与光电子学进展 50 6 Li Y L, Li Q, Wang M J, Dong Q F 2013 Laser Optoelectron. Prog. 50 6
[49]	Ma N Z, Li R X 2010 International Symposium on Antennas Propagation & Em Theory Guangzhou, Novenber 29–December 2, 2010 p646
[50]	Li R X, Ren K F, Han X 2013 J. Quant. Spectrosc. Radiat. Transfer 126 69 Google Scholar
[51]	Ren K F, Gréhan G, Gouesbet G 1994 J. Opt. Soc. Am. A 11 2072
[52]	Gouesbet G, Grehan G, Maheu B 1988 Appl. Opt. 27 4874 Google Scholar
[53]	Barton J P, Alexander D R, Schaub S A 1989 J. Appl. Phys. 66 4594 Google Scholar
[54]	Rohrbach A, Stelzer E H K 2000 J. Opt. Soc. Am. A 18 839
[55]	Harada Y, Asakura T 1996 Opt. Commun. 124 529 Google Scholar
[56]	Schut T C, Hesselink G, Grooth B G 1991 Cytometry 12 479 Google Scholar
[57]	Nemoto, Togo H 1998 Appl. Opt. 37 6386 Google Scholar
[58]	Nahmias Y K, Gao B Z, Odde D J 2004 Appl. Opt. 43 3999 Google Scholar
[59]	Drezek R, Dunn A, Richards-Kortum R 1999 Appl. Opt. 38 3651 Google Scholar

[1]	Bai Jing, Ma Wen-Hao, Ge Cheng-Xian, Wu Zhen-Sen, Xu Tong. Radiation force characteristics of non-uniform chiral stratified particles in standing wave field. Acta Physica Sinica, 2024, 73(18): 184201. doi: 10.7498/aps.73.20240842
[2]	Ning Lu-Hui, Zhang Xue, Yang Ming-Cheng, Zheng Ning, Liu Peng, Peng Yi. Effect of passive particle shape on effective force in active bath. Acta Physica Sinica, 2024, 73(15): 158202. doi: 10.7498/aps.73.20240650
[3]	Wang Yan, Peng Miao, Cheng Wei, Peng Zheng, Cheng Hao, Zang Sheng-Yin, Liu Hao, Ren Xiao-Dong, Shuai Yu-Bei, Huang Cheng-Zhi, Wu Jia-Gui, Yang Jun-Bo. Controllable multi-trap optical tweezers based on low loss optical phase change and metalens. Acta Physica Sinica, 2023, 72(2): 027801. doi: 10.7498/aps.72.20221794
[4]	Wang Yue, Liang Yan-Sheng, Yan Shao-Hui, Cao Zhi-Liang, Cai Ya-Nan, Zhang Yan, Yao Bao-Li, Lei Ming. Axial multi-particle trapping and real-time direct observation. Acta Physica Sinica, 2018, 67(13): 138701. doi: 10.7498/aps.67.20180460
[5]	Qian Hui, Chen Hu, Yan Jie. Frontier of soft matter experimental technique: single molecular manipulation. Acta Physica Sinica, 2016, 65(18): 188706. doi: 10.7498/aps.65.188706
[6]	Zhang Zhi-Gang, Liu Feng-Rui, Zhang Qing-Chuan, Cheng Teng, Wu Xiao-Ping. Trapping of multiple particles by space speckle field and infrared microscopy. Acta Physica Sinica, 2014, 63(2): 028701. doi: 10.7498/aps.63.028701
[7]	Huang Xue-Feng, Li Sheng-Ji, Zhou Dong-Hui, Zhao Guan-Jun, Wang Guan-Qing, Xu Jiang-Rong. Trap, ignition, and diffusion combustion characteristics of active carbon micro-particles at a meso-scale studied by optical tweezers. Acta Physica Sinica, 2014, 63(17): 178802. doi: 10.7498/aps.63.178802
[8]	Ren Hong-Liang. Design and error analysis for optical tweezers based on finite conjugate microscope. Acta Physica Sinica, 2013, 62(10): 100701. doi: 10.7498/aps.62.100701
[9]	Zhang Zhi-Gang, Liu Feng-Rui, Zhang Qing-Chuan, Cheng Teng, Gao Jie, Wu Xiao-Ping. Infrared microscopic observation of trapped absorbing particles. Acta Physica Sinica, 2013, 62(20): 208702. doi: 10.7498/aps.62.208702
[10]	Zhou Dan-Dan, Ren Yu-Xuan, Liu Wei-Wei, Gong Lei, Li Yin-Mei. Calibration of optical tweezers using time of flight method. Acta Physica Sinica, 2012, 61(22): 228702. doi: 10.7498/aps.61.228702
[11]	Ren Hong-Liang, Ding Pan-Feng, Li Xiao-Yan. Influences of axial position manipulation and misalignments of optical elements on radial trap position manipulation. Acta Physica Sinica, 2012, 61(21): 210701. doi: 10.7498/aps.61.210701
[12]	Hu Geng-Jun, Li Jing, Long Qian, Tao Tao, Zhang Gong-Xuan, Wu Xiao-Ping. FDTD numerical simulation of the trapping force of microspherein single optical tweezers. Acta Physica Sinica, 2011, 60(3): 030301. doi: 10.7498/aps.60.030301
[13]	Xu Jin, Wang Wen-Xiang, Yue Ling-Na, Gong Yu-Bin, Wei Yan-Yu. Propagation characteristics of an elliptical chirowaveguide. Acta Physica Sinica, 2009, 58(9): 6291-6295. doi: 10.7498/aps.58.6291
[14]	Han Guo-Xia, Han Yi-Ping. Radiation force of a sphere with an eccentric inclusion illuminated by a laser beam. Acta Physica Sinica, 2009, 58(9): 6167-6173. doi: 10.7498/aps.58.6167
[15]	Yang Hao, Feng Guo-Ying, Zhu Qi-Hua, Zhang Da-Yong, Zhou Shou-Huan. Study on trapping force of focused optical field on the microsphere with the FDTD method. Acta Physica Sinica, 2008, 57(9): 5506-5512. doi: 10.7498/aps.57.5506
[16]	Zeng Xia-Hui, Wu Feng-Tie, Liu Lan. The description of bottle beam based on the interferential theory. Acta Physica Sinica, 2007, 56(2): 791-797. doi: 10.7498/aps.56.791
[17]	Xu Chun-Hua, Liu Chun-Xiang, Guo Hong-Lian, Li Zhao-Lin, Jiang Yu-Qiang, Zhang Dao-Zhong, Yuan Ming. Photosensitive breaking of fluorescent labeled microtubules and its mechanism. Acta Physica Sinica, 2006, 55(1): 206-210. doi: 10.7498/aps.55.206
[18]	Zhang Yan-Li, Zhao Yi-Qiong, Zhan Qi-Wen, Li Yong-Ping. Study of 3D optical chain with highly focused vector beam. Acta Physica Sinica, 2006, 55(3): 1253-1258. doi: 10.7498/aps.55.1253
[19]	Han Yi-Ping, Du Yun-Gang, Zhang Hua-Yong. Radiation trapping forces acting on a two-layered spherical particle in a Gaussian beam. Acta Physica Sinica, 2006, 55(9): 4557-4562. doi: 10.7498/aps.55.4557
[20]	Jiang Yu-Qiang, Guo Hong-Lian, Liu Chun-Xiang, Li Zhao-Lin, Cheng Bing-Ying, Zhang Dao-Zhong, Jia Suo-Tang. Trapping stiffness measurement with brownian motion analysis method at low sampling frequency. Acta Physica Sinica, 2004, 53(6): 1721-1726. doi: 10.7498/aps.53.1721

Catalog

Vol. 71, Issue 10 - 2022-05-20

Metrics

Abstract views: 3925
PDF Downloads: 51
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板