Collective dynamics of higher-order coupled phase oscillators

Cai Zong-Kai; Xu Can; Zheng Zhi-Gang

doi:10.7498/aps.70.20211206

Abstract

The Kuramoto model consisting of large ensembles of coupled phase oscillators serves as an illustrative paradigm for studying the synchronization transitions and collective behaviors in various self-sustained systems. In recent years, the research of the high-order coupled phase oscillators has attracted extensive interest for the high-order coupled structure playing an essential role in modeling the dynamics of code and data storage. By studying the effects of high-order coupling, we extend the Kuramoto model of high-order structure by considering the correlations between frequency and coupling, which reflects the intrinsic properties of heterogeneity of interactions between oscillators. Several novel dynamic phenomena occur in the model, including clustering, extensive multistability, explosive synchronization and oscillatory state. The universal form of the critical coupling strength characterizing the transition from disorder to order is obtained via an analysis of the stability of the incoherent state. Furthermore, we present the self-consistent approach and find the multi-cluster with their expressions of order parameters. The stability analysis of multi-cluster is performed in the subspace getting stability condition together with the stable solutions of order parameters. The discussion of all the results summarizes the mechanism of the transition from hysteresis to oscillatory states. In addition, we emphasize that the combination of the Kuramoto order parameter capturing the asymmetry of the system and the Daido order parameter representing the clustering can give a complete description of the macroscopic dynamics of the system. The research of this paper can improve the understanding of the effects of the heterogeneity among populations and the explosive synchronization occurring in higher-order coupled phase oscillators.

Keywords:

Authors and contacts

1.
College of Information Science and Engineering, Huaqiao University, Xiamen 361021, China
2.
Institute of Systems Science, Huaqiao University, Xiamen 361021, China

Corresponding author: Xu Can, xucan@hqu.edu.cn ; Zheng Zhi-Gang, zgzheng@hqu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 11875135), the Young Scientists Fund of the National Natural Science Foundation of China (Grant No. 11905068), the Scientific and Technological Foundation of Quanzhou, China (Grant No. 2018C085R), and the Scientific Research Funds of Huaqiao University, China (Grant No. ZQN-810)

FullText HTML : translate this paragraph

References

[1]	Wiesenfeld K, Colet P, Strogatz S H 1998 Phys. Rev. E 57 1563
[2]	Rohden M, Sorge A, Timme M, Witthaut D 2012 Phys. Rev. Lett. 109 064101 Google Scholar
[3]	Pikovsky A, Rosenblum M, Kurths J 2001 Synchronization: A Universal Concept in Nonlinear Sciences (Cambridge: Cambridge University Press) pp18–28
[4]	Hoppensteadt F C, Izhikevich E M 1999 Phys. Rev. Lett. 82 2983 Google Scholar
[5]	Strogatz S H 2003 Sync: The Emerging Science of Spontaneous Order (New York: Hypernion) pp59–60
[6]	Arenas A, Díaz-Guilera A, Kurths J, Moreno Y, Zhou C 2008 Phys. Rep. 469 93 Google Scholar
[7]	郑志刚 2019 复杂系统的涌现动力学: 从同步到集体输运 (北京: 科学出版社) 第95−176页 Zheng Z G 2019 Emergence Dynamics in Complex Systems: From Synchronization to Collective Transport (Beijing: Science Press) pp95−176 (in Chinese)
[8]	Kuramoto Y 1975 Int. Symp. on Mathematical Problems in Theoretical Physics (Lecture Notes in Physics Vol. 30) ed Araki H (New York: Springer) pp4−20
[9]	Strogatz S H 2000 Physica D 143 1 Google Scholar
[10]	Acebrón J A, Bonilla L L, Pérez Vicente C J, Ritort F, Spigler R 2005 Rev. Mod. Phys. 77 137 Google Scholar
[11]	Pikovsky A, Rosenblum M 2015 Chaos 25 097616 Google Scholar
[12]	Daido H 1992 Prog. Theor. Phys. 88 1213 Google Scholar
[13]	Skardal P S, Ott E, Restrepo J G 2011 Phys. Rev. E 84 036208 Google Scholar
[14]	Komarov M, Pikovsky A 2013 Phys. Rev. Lett. 111 204101 Google Scholar
[15]	Xu C, Xiang H, Gao J, Zheng Z 2016 Sci. Rep. 6 31133 Google Scholar
[16]	Wang H, Han W, Yang J 2017 Phys. Rev. E 96 022202 Google Scholar
[17]	Gong C C, Pikovsky A 2019 Phys. Rev. E 100 062210 Google Scholar
[18]	Czolczynski K, Perlikowski P, Stefanski A, Kapitaniak T 2013 Commun. Nonlinear Sci. Numer. Simul. 18 386 Google Scholar
[19]	Goldobin E, Koelle D, Kleiner R, Mints R G 2011 Phys. Rev. Lett. 107 227001 Google Scholar
[20]	Goldobin E, Kleiner R, Koelle D, Mints R G 2103 Phys. Rev. Lett. 11 057004
[21]	Kiss I Z, Zhai Y, Hudson J L 2005 Phys. Rev. Lett. 94 248301 Google Scholar
[22]	Kiss I Z, Zhai Y, Hudson J L 2006 Prog. Theor. Phys. Suppl. 161 99 Google Scholar
[23]	Ashwin P, Rodrigues A 2016 Physica D 325 14 Google Scholar
[24]	León I, Pazó D 2019 Phys. Rev. E 100 012211 Google Scholar
[25]	Giusti C, Ghrist R, Bassett D S 2016 J. Comput. Neurosci. 41 1 Google Scholar
[26]	Komarov M, Pikovsky A 2015 Phys. Rev. E 92 020901 Google Scholar
[27]	Bick C, Ashwin P, Rodrigues A 2016 Chaos 26 094814 Google Scholar
[28]	Millán A P, Torres J J, Bianconi G 2020 Phys. Rev. Lett. 124 218301 Google Scholar
[29]	Skardal P S, Arenas A 2020 Commun. Phys. 3 218 Google Scholar
[30]	Skardal P S, Arenas A 2019 Phys. Rev. Lett. 122 248301 Google Scholar
[31]	Xu C, Wang X, Skardal P S 2020 Phys. Rev. Res. 2 023281 Google Scholar
[32]	Xu C, Skardal P S 2021 Phys. Rev. Res. 3 013013 Google Scholar
[33]	Vlasov V, Rosenblum M, Pikovsky A 2016 J. Phys. A: Math.Theor. 49 31LT02 Google Scholar
[34]	Chen B, Engelbrecht J R, Mirollo R 2017 J. Phys. A: Math. Theor. 50 355101 Google Scholar
[35]	王学彬, 徐灿, 郑志刚 2020 69 170501 Google Scholar Wang X B, Xu C, Zheng Z G 2020 Acta Phys. Sin. 69 170501 Google Scholar
[36]	Iatsenko D, Petkoski S, McClintock P V E, Stefanovska A 2013 Phys. Rev. Lett. 110 064101 Google Scholar
[37]	Hong H, Strogatz S H 2011 Phys. Rev. Lett. 106 054102 Google Scholar
[38]	Wang H, Li X 2011 Phys. Rev. E 83 066214 Google Scholar
[39]	Zhang X, Hu X, Kurths J, Liu Z 2013 Phys. Rev. E 88 010802 Google Scholar
[40]	Yuan D, Zhang M, Zhong J 2014 Phys. Rev. E 89 012910 Google Scholar
[41]	Bi H, Hu X, Boccaletti S, Wang X, Zou Y, Liu Z, Guan S 2016 Phys. Rev. Lett. 117 204101 Google Scholar
[42]	朱廷祥, 吴晔, 肖井华 2012 62 040502 Google Scholar Zhu T X, Wu Y, Xiao J H 2012 Acta Phys. Sin. 62 040502 Google Scholar
[43]	Xu C, Gao J, Xiang H, Jia W, Guan S, Zheng Z 2016 Phys. Rev. E 94 062204 Google Scholar
[44]	Xu C, Boccaletti S, Guan S, Zheng Z 2018 Phys. Rev. E 98 050202 Google Scholar
[45]	Xu C, Boccaletti S, Zheng Z, Guan S 2019 New J. Phys. 21 113018 Google Scholar
[46]	Xiao Y, Jia W, Xu C, Lü H, Zheng Z 2017 Europhys. Lett. 118 60005 Google Scholar
[47]	郑志刚, 翟云 2020 中国科学: 物理学力学天文学 50 010505 Google Scholar Zheng Z G, Zhai Y 2020 Sci. Sin. Phys., Mech. Astron. 50 010505 Google Scholar
[48]	Gómez-Gardeñes J, Gómez S, Arenas A, Moreno Y 2011 Phys. Rev. Lett. 106 128701 Google Scholar
[49]	Zou Y, Pereira T, Small M, Liu Z, Kurths J 2014 Phys. Rev. Lett. 112 114102 Google Scholar
[50]	Xu C, Gao J, Sun Y, Huang X, Zheng Z 2015 Sci. Rep. 5 12039 Google Scholar
[51]	Vlasov V, Zou Y, Pereira T 2015 Phys. Rev. E 92 012904 Google Scholar
[52]	管曙光 2020 中国科学: 物理学力学天文学 50 010504 Google Scholar Guan S G 2020 Sci. Sin. Phys., Mech. Astron. 50 010504 Google Scholar
[53]	Xu C, Gao J, Boccaletti S, Zheng Z and Guan S 2019 Phys. Rev. E 100 012212 Google Scholar
[54]	Mirollo R, Strogatz S H 2007 J. Nonlinear Sci. 17 309 Google Scholar
[55]	Omel’chenko O E, Wolfrum M 2013 Physica D 263 74 Google Scholar
[56]	Strogatz S H, Mirollo R E 1991 J. Stat. Phys. 63 613 Google Scholar
[57]	Xu C, Zheng Z 2019 Nonlinear Dyn. 98 2365 Google Scholar

Cited By

图 1 序参量$ R_2 $和$ R_1 $随耦合强度$ K $的相变图. $ g(\omega) $为双峰洛伦兹分布, 且$ \varDelta = 0.10 $, $ \omega_0 $分别取(a)$ 0.08 $, (b)$ 0.12 $, (c)$ 0.30 $, (d)$ 0.40 $. 其中正三角形$ \vartriangle $表示耦合强度$ K $增大的方向, 倒三角形$ \triangledown $表示耦合强度$ K $ 减小的方向. (a2)−(d2)中$ R1 $的相变曲线自上往下$ \alpha $分别取$ 1.00 $(粉, 方形), $ 0.90 $(青, 圆形), $ 0.80 $(蓝, 菱形), $ 0.70 $(绿, 左三角), $ 0.60 $(红, 右三角)

Figure 1. Phase transition diagram of order parameters $ R_ 2 $ and $ R_1 $ with the coupling strength $ K $. $ g(\omega) $ is bimodal Lorentz distribution with $ \varDelta = 0.10 $, and $ \omega_ 0 = $$ 0.08 $(a), $ 0.12 $(b), $ 0.30 $(c), $ 0.40 $(d), respectively. The regular triangle $ \vartriangle $ indicates the direction of the increase of coupling strength $ K $ and the inverted triangle $ \triangledown $ indicates the direction of the decrease of coupling strength $ K $. In (a2)−(d2), phase transition of $ R_1 $ with $ \alpha = $$ 1.00 $(pink, square), $ 0.90 $(cyan, circle), $ 0.80 $(blue, diamond), $ 0.70 $(green, left triangle) and $ 0.60 $(red, right triangle) from top to bottom, respectively.

DownLoad: Full-Size Img PowerPoint

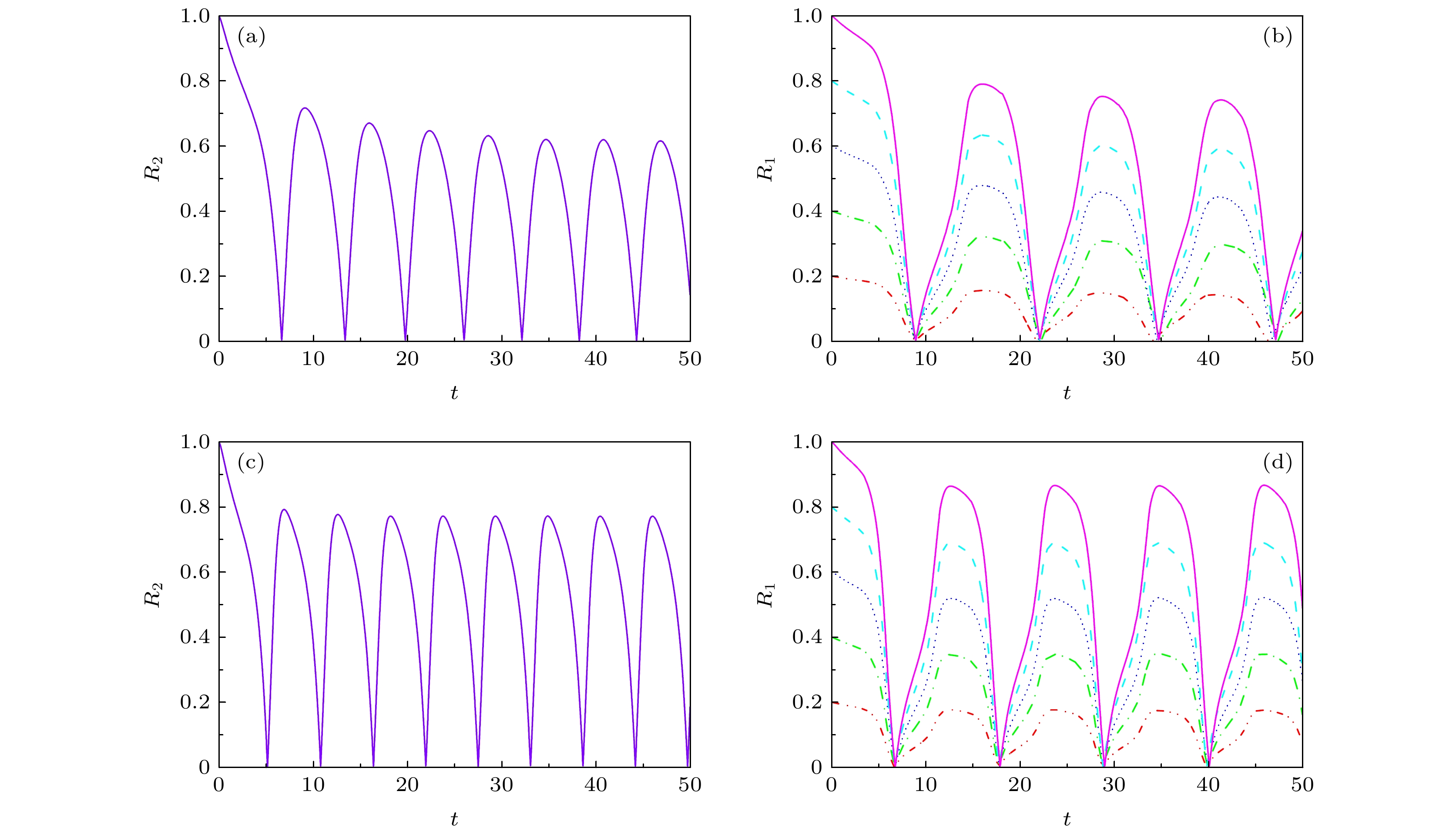
图 2 耦合强度$ K = 1.6 $时序参量$ R_2 $和$ R_1 $随时间$ t $的演化. $ g(\omega) $为双峰洛伦兹分布, 且$\varDelta = 0.1$, $ \omega_0 $分别取(a), (b)$ 0.30 $, (c), (d)$ 0.40 $. (b), (d)中$ R_1(t) $曲线自上往下$ \alpha $分别取$ 1.0 $(粉, 实线), $ 0.9 $(青, 划线), $ 0.8 $(蓝, 点线), $ 0.7 $(绿, 点划线), $ 0.6 $(红, 双点划线)

Figure 2. Evolution of the order parameters $ R_ 2 $ and $ R_1 $ with time $ t $ at coupling strength $ K = 1.60 $. $ g(\omega) $ is bimodal Lorentz distribution with $ \varDelta = 0.10 $, and $ \omega_0 = $$ 0.30 $ ((a), (b)), $ 0.40 $((c), (d)), respectively. The evolution of $ R_ 1(t) $ with $ \alpha = $$ 1.00 $(pink, solid line), $ 0.90 $(cyan, dash line), $ 0.80 $(blue, dot line), $ 0.70 $(green, dash dot line), $ 0.60 $(red, dash dots line) from top to bottom, respectively.

DownLoad: Full-Size Img PowerPoint

图 3 $ g(\omega) $为均匀分布, $ \gamma = 1.0 $ (a), (b)序参量$ R_2 $和$ R_1 $随耦合强度$ K $的相变图. 其中相变曲线自上往下$ \alpha $分别取$ 1.00 $(粉, 方形), $ 0.90 $(青, 圆形), $ 0.80 $(蓝, 菱形), $ 0.70 $(绿, 左三角), $ 0.60 $(红, 右三角). (c), (d)耦合强度$ K = 1.90 $时序参量$ R_2 $和$ R_1 $随时间$ t $的演化. 图中$ R_1 $曲线自上往下$ \alpha $分别取$ 1.0 $(粉, 实线), $ 0.9 $(青, 划线), $ 0.8 $(蓝, 点线), $ 0.7 $(绿, 点划线), $ 0.6 $(红, 双点划线)

Figure 3. $ g(\omega) $ is uniform distribution with $ \gamma = 1.0 $ (a), (b) Phase transition diagram of order parameters $ R_ 2 $ and $ R_1 $ with the coupling strength $ K $. Phase transition of $ R_1 $ with $ \alpha = $$ 1.00 $(pink, square), $ 0.90 $(cyan, circle), $ 0.80 $(blue, diamond), $ 0.70 $(green, left triangle) and $ 0.60 $(red, right triangle) from top to bottom, respectively. (c), (d) Evolution of the order parameters $ R_ 2 $ and $ R_1 $ with time $ t $ at coupling strength $ K = 1.90 $. The curve of $ R_ 1 $ with $ \alpha = $$ 1.00 $(pink, solid line), $ 0.90 $(cyan, dash line), $ 0.80 $(blue, dot line), $ 0.70 $(green, dash dot line), $ 0.60 $(red, dash dots line) from top to bottom, respectively.

DownLoad: Full-Size Img PowerPoint

map

[1]	Wiesenfeld K, Colet P, Strogatz S H 1998 Phys. Rev. E 57 1563
[2]	Rohden M, Sorge A, Timme M, Witthaut D 2012 Phys. Rev. Lett. 109 064101 Google Scholar
[3]	Pikovsky A, Rosenblum M, Kurths J 2001 Synchronization: A Universal Concept in Nonlinear Sciences (Cambridge: Cambridge University Press) pp18–28
[4]	Hoppensteadt F C, Izhikevich E M 1999 Phys. Rev. Lett. 82 2983 Google Scholar
[5]	Strogatz S H 2003 Sync: The Emerging Science of Spontaneous Order (New York: Hypernion) pp59–60
[6]	Arenas A, Díaz-Guilera A, Kurths J, Moreno Y, Zhou C 2008 Phys. Rep. 469 93 Google Scholar
[7]	郑志刚 2019 复杂系统的涌现动力学: 从同步到集体输运 (北京: 科学出版社) 第95−176页 Zheng Z G 2019 Emergence Dynamics in Complex Systems: From Synchronization to Collective Transport (Beijing: Science Press) pp95−176 (in Chinese)
[8]	Kuramoto Y 1975 Int. Symp. on Mathematical Problems in Theoretical Physics (Lecture Notes in Physics Vol. 30) ed Araki H (New York: Springer) pp4−20
[9]	Strogatz S H 2000 Physica D 143 1 Google Scholar
[10]	Acebrón J A, Bonilla L L, Pérez Vicente C J, Ritort F, Spigler R 2005 Rev. Mod. Phys. 77 137 Google Scholar
[11]	Pikovsky A, Rosenblum M 2015 Chaos 25 097616 Google Scholar
[12]	Daido H 1992 Prog. Theor. Phys. 88 1213 Google Scholar
[13]	Skardal P S, Ott E, Restrepo J G 2011 Phys. Rev. E 84 036208 Google Scholar
[14]	Komarov M, Pikovsky A 2013 Phys. Rev. Lett. 111 204101 Google Scholar
[15]	Xu C, Xiang H, Gao J, Zheng Z 2016 Sci. Rep. 6 31133 Google Scholar
[16]	Wang H, Han W, Yang J 2017 Phys. Rev. E 96 022202 Google Scholar
[17]	Gong C C, Pikovsky A 2019 Phys. Rev. E 100 062210 Google Scholar
[18]	Czolczynski K, Perlikowski P, Stefanski A, Kapitaniak T 2013 Commun. Nonlinear Sci. Numer. Simul. 18 386 Google Scholar
[19]	Goldobin E, Koelle D, Kleiner R, Mints R G 2011 Phys. Rev. Lett. 107 227001 Google Scholar
[20]	Goldobin E, Kleiner R, Koelle D, Mints R G 2103 Phys. Rev. Lett. 11 057004
[21]	Kiss I Z, Zhai Y, Hudson J L 2005 Phys. Rev. Lett. 94 248301 Google Scholar
[22]	Kiss I Z, Zhai Y, Hudson J L 2006 Prog. Theor. Phys. Suppl. 161 99 Google Scholar
[23]	Ashwin P, Rodrigues A 2016 Physica D 325 14 Google Scholar
[24]	León I, Pazó D 2019 Phys. Rev. E 100 012211 Google Scholar
[25]	Giusti C, Ghrist R, Bassett D S 2016 J. Comput. Neurosci. 41 1 Google Scholar
[26]	Komarov M, Pikovsky A 2015 Phys. Rev. E 92 020901 Google Scholar
[27]	Bick C, Ashwin P, Rodrigues A 2016 Chaos 26 094814 Google Scholar
[28]	Millán A P, Torres J J, Bianconi G 2020 Phys. Rev. Lett. 124 218301 Google Scholar
[29]	Skardal P S, Arenas A 2020 Commun. Phys. 3 218 Google Scholar
[30]	Skardal P S, Arenas A 2019 Phys. Rev. Lett. 122 248301 Google Scholar
[31]	Xu C, Wang X, Skardal P S 2020 Phys. Rev. Res. 2 023281 Google Scholar
[32]	Xu C, Skardal P S 2021 Phys. Rev. Res. 3 013013 Google Scholar
[33]	Vlasov V, Rosenblum M, Pikovsky A 2016 J. Phys. A: Math.Theor. 49 31LT02 Google Scholar
[34]	Chen B, Engelbrecht J R, Mirollo R 2017 J. Phys. A: Math. Theor. 50 355101 Google Scholar
[35]	王学彬, 徐灿, 郑志刚 2020 69 170501 Google Scholar Wang X B, Xu C, Zheng Z G 2020 Acta Phys. Sin. 69 170501 Google Scholar
[36]	Iatsenko D, Petkoski S, McClintock P V E, Stefanovska A 2013 Phys. Rev. Lett. 110 064101 Google Scholar
[37]	Hong H, Strogatz S H 2011 Phys. Rev. Lett. 106 054102 Google Scholar
[38]	Wang H, Li X 2011 Phys. Rev. E 83 066214 Google Scholar
[39]	Zhang X, Hu X, Kurths J, Liu Z 2013 Phys. Rev. E 88 010802 Google Scholar
[40]	Yuan D, Zhang M, Zhong J 2014 Phys. Rev. E 89 012910 Google Scholar
[41]	Bi H, Hu X, Boccaletti S, Wang X, Zou Y, Liu Z, Guan S 2016 Phys. Rev. Lett. 117 204101 Google Scholar
[42]	朱廷祥, 吴晔, 肖井华 2012 62 040502 Google Scholar Zhu T X, Wu Y, Xiao J H 2012 Acta Phys. Sin. 62 040502 Google Scholar
[43]	Xu C, Gao J, Xiang H, Jia W, Guan S, Zheng Z 2016 Phys. Rev. E 94 062204 Google Scholar
[44]	Xu C, Boccaletti S, Guan S, Zheng Z 2018 Phys. Rev. E 98 050202 Google Scholar
[45]	Xu C, Boccaletti S, Zheng Z, Guan S 2019 New J. Phys. 21 113018 Google Scholar
[46]	Xiao Y, Jia W, Xu C, Lü H, Zheng Z 2017 Europhys. Lett. 118 60005 Google Scholar
[47]	郑志刚, 翟云 2020 中国科学: 物理学力学天文学 50 010505 Google Scholar Zheng Z G, Zhai Y 2020 Sci. Sin. Phys., Mech. Astron. 50 010505 Google Scholar
[48]	Gómez-Gardeñes J, Gómez S, Arenas A, Moreno Y 2011 Phys. Rev. Lett. 106 128701 Google Scholar
[49]	Zou Y, Pereira T, Small M, Liu Z, Kurths J 2014 Phys. Rev. Lett. 112 114102 Google Scholar
[50]	Xu C, Gao J, Sun Y, Huang X, Zheng Z 2015 Sci. Rep. 5 12039 Google Scholar
[51]	Vlasov V, Zou Y, Pereira T 2015 Phys. Rev. E 92 012904 Google Scholar
[52]	管曙光 2020 中国科学: 物理学力学天文学 50 010504 Google Scholar Guan S G 2020 Sci. Sin. Phys., Mech. Astron. 50 010504 Google Scholar
[53]	Xu C, Gao J, Boccaletti S, Zheng Z and Guan S 2019 Phys. Rev. E 100 012212 Google Scholar
[54]	Mirollo R, Strogatz S H 2007 J. Nonlinear Sci. 17 309 Google Scholar
[55]	Omel’chenko O E, Wolfrum M 2013 Physica D 263 74 Google Scholar
[56]	Strogatz S H, Mirollo R E 1991 J. Stat. Phys. 63 613 Google Scholar
[57]	Xu C, Zheng Z 2019 Nonlinear Dyn. 98 2365 Google Scholar

[1]	Zhang Xiu-Fang, Ma Jun, Xu Ying, Ren Guo-Dong. Synchronization between FitzHugh-Nagumo neurons coupled with phototube. Acta Physica Sinica, 2021, 70(9): 090502. doi: 10.7498/aps.70.20201953
[2]	Wang Xue-Bin, Xu Can, Zheng Zhi-Gang. Synchronization in coupled oscillators with multiplex interactions. Acta Physica Sinica, 2020, 69(17): 170501. doi: 10.7498/aps.69.20200394
[3]	Zheng Zhi-Gang, Zhai Yun, Wang Xue-Bin, Chen Hong-Bin, Xu Can. Synchronization of coupled phase oscillators: Order parameter theory. Acta Physica Sinica, 2020, 69(8): 080502. doi: 10.7498/aps.69.20191968
[4]	Huang Xia, Xu Can, Sun Yu-Ting, Gao Jian, Zheng Zhi-Gang. Multiple synchronous states in a ring of coupled phase oscillators. Acta Physica Sinica, 2015, 64(17): 170504. doi: 10.7498/aps.64.170504
[5]	Xing Xue, Wang Xiao-Fei, Zhang Qing-Li, Sun Gui-Hua, Liu Wen-Peng, Sun Dun-Lu, Yin Shao-Tang. Phase transition and structure of LuTaO4. Acta Physica Sinica, 2014, 63(24): 248107. doi: 10.7498/aps.63.248107
[6]	Sun Zong-Xin, Yu Yang, Zhou Feng, Liu Song-Zuo, Qiao Gang. Underwater acoustic synchronization telemetry research based on binary offset carrier modulated signal with zero correlation window. Acta Physica Sinica, 2014, 63(10): 104301. doi: 10.7498/aps.63.104301
[7]	Chen Xing-Ji, Tian Tao-Tao, Zhou Zhen-Wei, Hu Yi-Bo, Tang Guo-Ning. Synchronization of two spiral waves interacting through a passive medium. Acta Physica Sinica, 2012, 61(21): 210509. doi: 10.7498/aps.61.210509
[8]	Hu Wen, Li Jun-Ping, Zhang Gong, Liu Wen-Bo, Zhao Guang-Hao. The chaotic self-FM system and its FM code coupled synchronization. Acta Physica Sinica, 2012, 61(1): 010504. doi: 10.7498/aps.61.010504
[9]	Lü Ling, Li Gang, Zhang Meng, Li Yu-Shan, Wei Lin-Ling, Yu Miao. Parameter identification and synchronization of spatiotemporal chaos in globally coupled network. Acta Physica Sinica, 2011, 60(9): 090505. doi: 10.7498/aps.60.090505
[10]	Wu Yong-Feng, Zhang Shi-Ping, Sun Jin-Wei, Peter Rolfe. Abrupt change of synchronization of ring coupled Duffing oscillator. Acta Physica Sinica, 2011, 60(2): 020511. doi: 10.7498/aps.60.020511
[11]	Pei Li-Jun, Qiu Ben-Hua. Generalization of mode decomposition approach to studies of the synchronization of non-identical coupled dynamical systems. Acta Physica Sinica, 2010, 59(1): 164-170. doi: 10.7498/aps.59.164
[12]	Lü Ling, Li Gang, Shang Jin-Yu, Shen Na, Zhang Xin, Liu Shuang, Zhu Jia-Bo. The synchronization of spatiotemporal chaos of nearest-neighbor coupled network. Acta Physica Sinica, 2010, 59(9): 5966-5971. doi: 10.7498/aps.59.5966
[13]	Bian Qiu-Xiang, Yao Hong-Xing. Synchronization of weighted complex networks with multi-links and nonlinear coupling. Acta Physica Sinica, 2010, 59(5): 3027-3034. doi: 10.7498/aps.59.3027
[14]	Jing Xiao-Dan, Lü Ling. The synchronization of spatiotemporal chaos of all-to-all network using nonlinear coupling. Acta Physica Sinica, 2009, 58(11): 7539-7543. doi: 10.7498/aps.58.7539
[15]	Wang Xing-Yuan, Wu Xiang-Jun. Adaptive control and synchronization of a coupled dynamos system. Acta Physica Sinica, 2006, 55(10): 5077-5082. doi: 10.7498/aps.55.5077
[16]	Qian Yu, Song Xuan-Yu, Shi Wei, Chen Guang-Zhi, Xue Yu. Turbulence synchronization and suppression by coupling in excitable media. Acta Physica Sinica, 2006, 55(9): 4420-4427. doi: 10.7498/aps.55.4420
[17]	Liu Hong, Wang Hui. Phase transition in biaxial nematic liquid crystal. Acta Physica Sinica, 2005, 54(3): 1306-1312. doi: 10.7498/aps.54.1306
[18]	Wu Ying, Xu Jian-Xue, He Dai-Hai, Jin Wu-Yin. Study on nonlinear characteristics of two synchronizing uncoupled Hindmarsh-Rose neurons. Acta Physica Sinica, 2005, 54(7): 3457-3464. doi: 10.7498/aps.54.3457
[19]	Yu Hong-Jie, Liu Yan-Zhu. Synchronization of symmetrically nonlinear-coupled chaotic systems. Acta Physica Sinica, 2005, 54(7): 3029-3033. doi: 10.7498/aps.54.3029
[20]	Zhang Xu, Shen Ke. Unilaterally coupled synchronization of spatiotemporal chaos. Acta Physica Sinica, 2002, 51(12): 2702-2706. doi: 10.7498/aps.51.2702

Catalog

Vol. 70, Issue 22 - 2021-11-20

Metrics

Abstract views: 6336
PDF Downloads: 218
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板