Fast autaptic feedback induced-paradoxical changes of mixed-mode bursting and bifurcation mechanism

Jiang Yi-Lan; Lu Bo; Zhang Wan-Qin; Gu Hua-Guang

doi:10.7498/aps.70.20210208

Abstract

Bursting is a complex multiple-time-scale nonlinear phenomenon in a nervous system and exhibits diverse patterns, which is modulated by the excitatory or inhibitory effect to achieve the physiological functions. According to the bifurcations of the fast subsystem, bursting is classified as different patterns including the mixed-mode bursting. Recently, many studies have found the paradoxical phenomena contrary to the common concept that the inhibitory effect induces the electrical activity to decrease or the excitatory effect induces the discharge activity to increase, which enriches the connotation of the nonlinear dynamics. To identify more examples of paradoxical phenomena of different bursting patterns and the underlying nonlinear mechanism, in the present study the paradoxical phenomena for the complex mixed-mode oscillations of the bursting pattern induced by the self-feedback mediated by both the inhibitory autapse and excitatory autapse and the bifurcation mechanism are acquired in the modified Morris-Lecar model. By using the fast/slow variable dissection method, the complex dynamics of the bursting is acquired, which is that the depolarization block behavior appears after the burst and before the quiescent state. The burst begins from a saddle-node bifurcation in an invariant cycle (SNIC) and terminates at a fold limit cycle (FLC) bifurcation. Furthermore, the FLC bifurcation is identified to play a key role in generating the paradoxical phenomenon. The inhibitory autapse induces the FLC bifurcation to shift leftward. However, the SNIC point remains unchanged. The change of FLC bifurcation point leads the parameter range of the burst to widen, the number of spikes per burst to become larger, and the average firing frequency to turn higher. Unlike the inhibitory autapse, the excitatory autapse induces the FLC bifurcation to shift rightward, and SNIC to be unchanged, thus reducing the bursting activity. Such results are different from those of the paradoxical phenomenon induced by the inhibitory autapse instead of excitatory autapse for the other bursting pattern and by the slow auatpse, which present a novel example and regulation mechanism of the paradoxical phenomena of the bursting patterns and show the diversity of the paradoxical phenomena, thus helping understand the potential functions of the bursting and self-feedback modulations of the brain neurons.

Keywords:

Authors and contacts

1.
School of Mathematical Science, Henan Institute of Science and Technology, Xinxiang 453003, China
2.
School of Aerospace Engineering and Applied Mechanics, Tongji University, Shanghai 200092, China

Corresponding author: Lu Bo, cheersnow@163.com

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12072236, 11872276), the Postdoctoral Research Project of Henan Province, China (Grant No. 19030095), and the Key R&D and Promotion Special Project of Henan Province(Science and Technology Targeting), China (Grant No. 212102210543)

FullText HTML : translate this paragraph

References

[1]	Yang Y, Cui Y H, Sang K N, Dong Y Y, NI Z Y, Ma S S, Hu H L 2018 Nature 554 317 Google Scholar
[2]	Wang X J 2010 Phys. Rev. 90 1195 Google Scholar
[3]	Mondal A, Upadhyay R K, Ma J, Yadav B K, Sharma S K, Mondal A 2019 Cogn. Neurodyn. 13 393 Google Scholar
[4]	Glass L 2001 Nature 410 277 Google Scholar
[5]	Izhikevich E M 2000 Int. J. Bifurcation Chaos 10 1171 Google Scholar
[6]	Duan L X, Liang W J, Ji W C, Xi H G 2020 Int. J. Bifurcation Chaos 30 2050192 Google Scholar
[7]	Desroches M, Guckenheimer J, Krauskopf B, Kuehn C, Osinga H M, Wechselberger M 2012 SIAM Rev. 54 211 Google Scholar
[8]	Lu B, Liu S, Jiang X, Jing W, Wang X 2017 Discrete Cont. Dyn. -S 10 487 Google Scholar
[9]	Zhan F B, Liu S Q, Zhang X H, Wang J, Lu B 2018 Nonlinear Dyn. 94 807 Google Scholar
[10]	Lisman J E 1997 Trends Neurosci. 20 38 Google Scholar
[11]	Braun H A, Wissing H, Schäfer K, Hirsch M C 1994 Nature 367 270 Google Scholar
[12]	Gu H G, Pan B B 2015 Front. Comput. Neurosci. 9 108 Google Scholar
[13]	Gu H G, Pan B B, Chen G R, Duan L X 2014 Nonlinear Dyn. 78 391 Google Scholar
[14]	Yin L P, Zheng R, Ke W, He Q, Zhang Y, Li J, Wang B, Mi Z, Long Y S, Rasch M J, Li T, Luan G, Shu Y 2018 Nat. Commun. 9 4890 Google Scholar
[15]	Costa K P, Ulrich E, Michael S, Petra R, Dan R, Peter F, Nikolaos K, Lana K I, Joseph K 2021 Cereb. Cortex 31 2013
[16]	Satterlie R A 1985 Science 229 402 Google Scholar
[17]	Beiderbeck B, Myoga M H, Müller N, Callan A R, Friauf E, Grothe B, Pecka M 2018 Nat. Commun. 9 1771 Google Scholar
[18]	Li Y Y, Gu H G, Ding X L 2019 Nonlinear Dyn. 97 2091 Google Scholar
[19]	华洪涛, 陆博, 古华光 2020 69 090502 Google Scholar Hua H T, Lu B, Gu H G 2020 Acta Phys. Sin. 69 090502 Google Scholar
[20]	杨永霞, 李玉叶, 古华光 2020 69 040501 Google Scholar Yang Y X, Li Y Y, Gu H G 2020 Acta Phys. Sin. 69 040501 Google Scholar
[21]	Wu F Q, Gu H G 2020 Int. J. Bifurcation Chaos 30 2030009 Google Scholar
[22]	Zhao Z G, Li L, Gu H G 2018 Front. Cell. Neurosci. 12 62 Google Scholar
[23]	Dodla R, Rinzel J 2006 Phys. Rev. E 73 010903 Google Scholar
[24]	Uzuntarla M, Torres J J, Calim A, Barreto E 2019 Neural Networks 110 131 Google Scholar
[25]	Elson R C, Selverston A I, Abarbanel H D I, Rabinovich M I 2002 J. Neurophysiol. 88 1166 Google Scholar
[26]	Jia B, Wu Y, He D, Guo B H, Xue L 2018 Nonlinear Dyn. 93 1599 Google Scholar
[27]	Yao C G, He Z W, Nakano T, Qian Y, Shuai J W 2019 Nonlinear Dyn. 97 1425 Google Scholar
[28]	Jia Y B, Gu H G, Li Y Y, Ding X L 2021 Commun. Nonlinear Sci. Numer. Simul. 95 105643 Google Scholar
[29]	Van Der Loos H, Glaser E M 1972 Brain Res. 48 355 Google Scholar
[30]	Bacci A, Huguenard J R, Prince D A 2003 J. Neurosci. 23 859 Google Scholar
[31]	Bacci A, Huguenard J R 2006 Neuron 49 119 Google Scholar
[32]	Saada R, Miller N, Hurwitz I, Susswein A J 2009 Curr. Biol. 19 479 Google Scholar
[33]	Szegedi V, Paizs M, Baka J, Barzó P, Molnár G, Tamas G, Lamsa K 2020 Elife 9 e51691 Google Scholar
[34]	Wang H T, Ma J, Chen Y L, Chen Y 2014 Commun. Nonlinear Sci. Numer. Simul. 19 3242 Google Scholar
[35]	Guo D Q, Chen M M, Perc M, Wu S D, Xia C, Zhang Y S, Xu P, Xia Y, Yao D Z 2016 Europhys. Lett. 114 30001 Google Scholar
[36]	Ge M Y, Xu Y, Zhang Z K, Peng Y X, Kang W J, Yang L J, Jia Y 2018 Eur. Phys. J. Spec. Top. 227 799 Google Scholar
[37]	Song X L, Wang H T, Chen Y 2019 Nonlinear Dyn. 96 2341 Google Scholar
[38]	Wu Y N, Gong Y B, Wang Q 2015 Chaos 25 043113 Google Scholar
[39]	丁学利, 贾冰, 李玉叶 2019 68 180502 Google Scholar Ding X L, Jia B, Li Y Y 2019 Acta Phys. Sin. 68 180502 Google Scholar
[40]	曲良辉, 都琳, 曹子露, 胡海威, 邓子辰 2020 69 230501 Google Scholar Qu L H, Du L, Cao Z L, Hu H W, Deng Z C 2020 Acta Phys. Sin. 69 230501 Google Scholar
[41]	Ma J, Song X L, Tang J, Wang C N 2015 Neurocomputing 167 378 Google Scholar
[42]	Yang X L, Yu Y H, Sun Z K 2017 Chaos 27 083117 Google Scholar
[43]	Zhang X H, Liu S Q 2018 Chin. Phys. B 27 040501 Google Scholar
[44]	Tikidji-Hamburyan R A, Martinez J J, White J A, Canavier C C 2015 J. Neurosci. 35 15682 Google Scholar
[45]	Zhao Z G, Li L, Gu H G, Gao Y 2020 Nonlinear Dyn. 99 1129 Google Scholar
[46]	Deleuze C, Bhumbra G S, Pazienti A, Lourenco J, Mailhes C, Aguirre A, Beato M, Bacci A 2019 PLoS Biol. 17 e3000419 Google Scholar
[47]	Connelly W M 2014 PLoS ONE 9 e89995 Google Scholar
[48]	Zhao Z G, Li L, Gu H G 2020 Commun. Nonlinear Sci. Numer. Simul. 85 105250 Google Scholar
[49]	丁学利, 李玉叶 2016 65 210502 Google Scholar Ding X L, Li Y Y 2016 Acta Phys. Sin. 65 210502 Google Scholar
[50]	曹奔, 关利南, 古华光 2018 67 240502 Google Scholar Cao B, Guan L N, Gu H G 2018 Acta Phys. Sin. 67 240502 Google Scholar
[51]	Li Y Y, Gu H G, Jia B, Ding X L 2021 Sci. China Ser. E: Technol. Sci. 64 1459
[52]	Zhao Z G, Jia B, Gu H G 2016 Nonlinear Dyn. 86 1549 Google Scholar
[53]	Ermentrout B 2002 Simulating, Analyzing, and Animating Dynamical Systems: A Guide to XPPAUT for Researchers and Students (Philadelphia: SIAM) pp161−194
[54]	Xu Y, Liu M H, Zhu Z G, Ma J 2020 Chin. Phys. B 29 098704 Google Scholar
[55]	Zhang X J, Gu H G, Guan L N 2019 Sci. China Ser. E:Technol. Sci. 62 1502 Google Scholar
[56]	Wang Z L, Shi X R 2020 Cogn. Neurodyn. 14 115 Google Scholar

Cited By

图 1 MML模型未加自突触的混合簇放电的膜电位　(a) 周期3, $ {V_u}{{ = }}0.02 $; (b) 周期4, $ {V_u}{{ = }}0.05 $; (c) 周期6, $ {V_u}{{ = }}0.1 $, 数字1—6表示一个簇内的第1—6个峰; (d) 周期8, $ {V_u}{{ = }}0.12 $

Figure 1. Membrane potential of mixed oscillations in the MML model without autapse: (a) Period-3 bursting when $ {V_u}{{ = }}0.02 $; (b) Period-4 bursting when $ {V_u}{{ = }}0.05 $; (c) Period-6 bursting when $ {V_u}{{ = }}0.1 $, The numbers 1–6 represent the 1^st–6^th spikes within a burst; (d) Period-8 bursting when $ {V_u}{{ = }}0.12 $.

DownLoad: Full-Size Img PowerPoint

图 2 周期6簇放电的快慢变量分离　(a) 快子系统的平衡点及分岔. 上支黑色实线表示稳定的焦点, 黑色虚线表示不稳定的焦点, 中支黑色虚线表示鞍点, 下支黑色实线表示稳定的结点, H点为sub-Hopf分岔点, SNIC为不变环上的鞍结分岔点. $ {V_{\max }} $和$ {V_{\min }} $表示稳定极限环的极大和极小值. (b) 快子系统的极限环分岔(蓝色实线表示稳定的极限环, 蓝色虚线表示不稳定的极限环)与图(a)的叠加. (c)周期6簇放电的相轨线(红)与图(b)的叠加. (d) 图(c)的局部放大, 数字1—6表示一个簇内的第1—6个峰

Figure 2. Fast/slow variable dissection of the period-6 bursting: (a) Bifurcation diagram of the equilibria of the fast subsystem. Upper black solid line, upper black dotted line, middle black dotted line, and lower black solid line is composed of the stable focus, unstable focus, saddle, and stable node, respectively. H and SNIC represents the subcritical Hopf bifurcation and saddle-node bifurcation on an invariant circle. 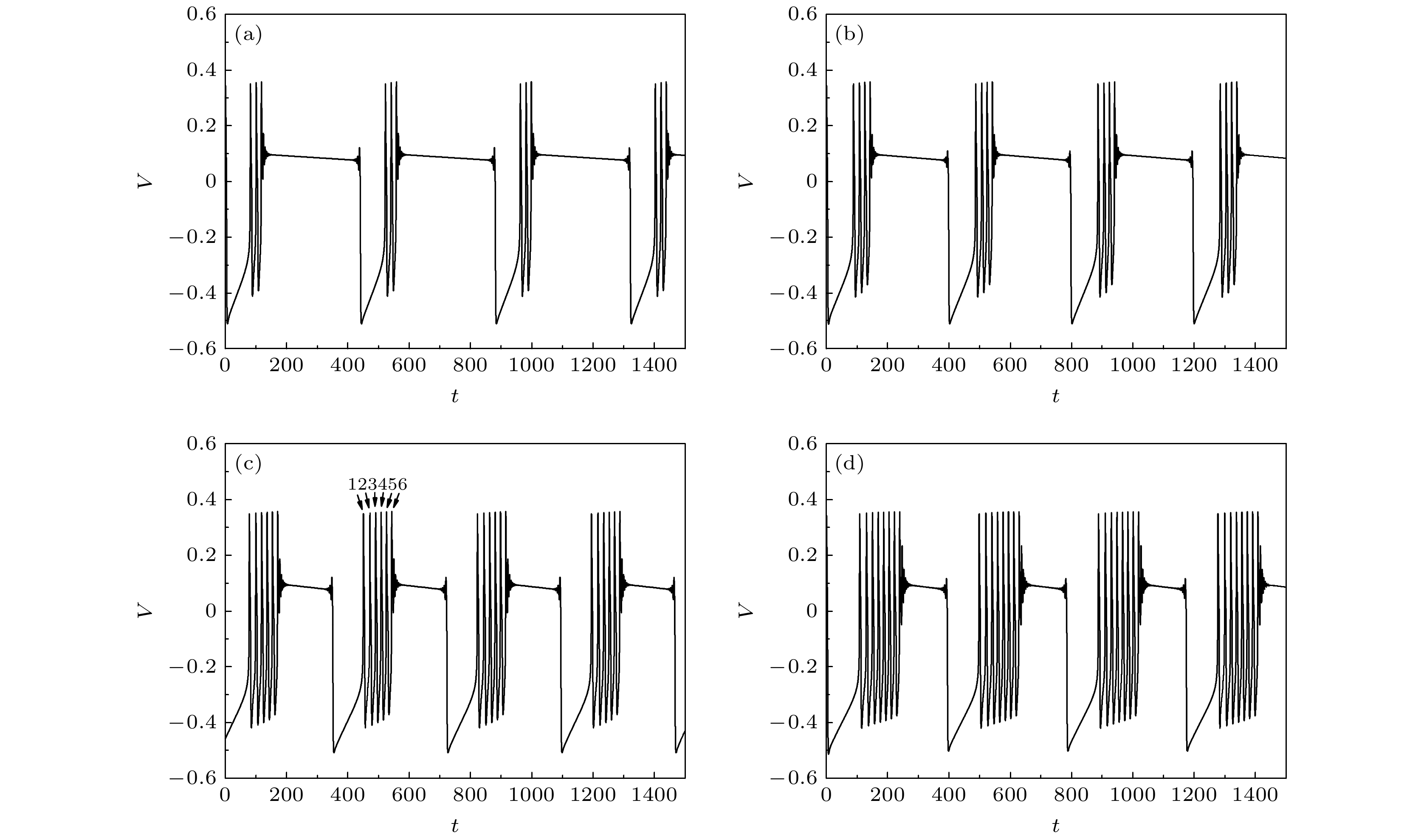$ {V_{\max }} $ and $ {V_{\min }} $ represent the maximal and minimal value of the stable limit cycle, respectively. (b) Bifurcation diagram of the limit cycles (solid and dashed bule lines represent the stable and unstable limit cycle) of the fast subsystem and Fig. (a) plotted in one figure. (c) The trajectory of the period-6 bursting (red) superimposes Fig. (b). (d) Partial enlargement of Fig. (c). The numbers 1–6 represents the 1^st–6^th spikes within a burst.

DownLoad: Full-Size Img PowerPoint

图 3 自突触电流(红)影响下的膜电位(黑)　(a1)—(a3) 抑制性自突触 (a1) 周期8簇放电, $ g = 0.01 $; (a2) 周期10簇放电, $ g = 0.015 $; (a3) 周期19簇, $ g = 0.02 $. (b1)—(b3) 兴奋性自突触 (b1) 周期3簇放电, $ g = 0.02 $; (b2) 周期2簇放电, $ g = 0.03 $; (b3) 周期1簇放电, $ g = 0.04 $

Figure 3. Membrane potential (black) under the influence of autaptic current (red). (a1)–(a3) Inhibitory autapse: (a1) Period-8 bursting when $ g = 0.01 $; (a2) period-10 bursting when $ g = 0.015 $; (a3) period-19 bursting when $ g = 0.02 $. (b1)–(b3) Excitatory autupse: (b1) Period-3 bursting when $ g = 0.02 $; (b2) period-2 bursting when $ g = 0.03 $; (b3) Period-1 bursting when $ g = 0.04 $.

DownLoad: Full-Size Img PowerPoint

图 4 不同自突触电导下的簇放电的峰峰间期ISI随序号的变化　(a) 抑制性自突触, $ g = 0 $(蓝), $ g = 0.01 $(绿), $ g = 0.015 $(红)和$ g = 0.02 $(黑); (b) 兴奋性自突触, $ g = 0 $(蓝), $ g = 0.02 $(粉)和$ g = 0.03 $(青)

Figure 4. Change of ISI respect to the sequential number at different levels of autaptic conductance: (a) Inhibitory autaptic current, blue, green, red, and black lines represent $ g = 0 $, $ g = 0.01 $, $ g = 0.015 $, and $ g = 0.02 $, respectively; (b) excitatory autaptic current, blue, pink, and cyan-blue lines represents $ g = 0 $, $ g = 0.02 $, and $ g = 0.03 $, respectively.

DownLoad: Full-Size Img PowerPoint

图 5 平均放电频率(上, 绿)与自突触电流的平均值(下, 蓝)随自突触电导g的变化　(a) 抑制性自突触; (b) 兴奋性自突触

Figure 5. Change of the mean firing frequency (green, upper) and the mean value of autaptic current (blue, lower) with respect to g: (a) Inhibitory autapse; (b) excitatory autapse.

DownLoad: Full-Size Img PowerPoint

图 6 不同自突触电导强度下快子系统的分岔　(a1)—(a4) 抑制性自突触: (a1)平衡点分岔; (a2) 图(a1)的局部放大; (a3) 极限环分岔与图(a1)的叠加; (a4) 图(a3)的局部放大. 电导强度的取值为$ g = 0 $(蓝), $ g = 0.01 $(绿), $ g = 0.015 $(红)和$ g = 0.02 $(黑). (b1)—(b4)兴奋性自突触: (b1) 平衡点分岔; (b2) 图(b1)的局部放大; (b3) 极限环分岔与图(b1)的叠加; (b4) 图(b3)的局部放大. 电导强度的取值为$ g = 0 $(蓝), $ g = 0.02 $(粉), $ g = 0.03 $(青)和$ g = 0.04 $(棕)

Figure 6. Bifurcations of the fast subsystem at different values of the autapse conductance. (a1)–(a4) Inhibitory autapse: (a1) Bifurcations of the equilibria; (a2) partial enlargement of Fig. (a1); (a3) bifurcations of the limit cycles superimposes Fig. (a1); (a4) partial enlargement of Fig. (a3). Blue, green, red, and black lines represent $ g = 0 $, $ g = 0.01 $, $ g = 0.015 $, and $ g = 0.02 $, respectively. (b1)–(b4) Excitatory autapse: (b1) Bifurcations of the equilibria; (b2) partial enlargement of Fig. (b1); (b3) bifurcation of the limit cycles superimposes Fig. (b1); (b4) partial enlargement of Fig. (b3). Blue, pink, cyan-blue, and brown curves represents $ g = 0 $, $ g = 0.02 $, $ g = 0.03 $ and $ g = 0.04 $, respectively.

DownLoad: Full-Size Img PowerPoint

图 7 不同抑制性自突触电导强度下的簇放电的快慢变量分离　(a1) $ g = 0 $; (a2) 图(a1)的放大; (b1) $ g = 0.01 $, 数字1—6代表簇内第1—6个放电峰; (b2) 图(b1)的放大; (c1) $ g = 0.015 $; (c2) 图(c1)的放大; (d1) $ g = 0.02 $; (d2) 图(d1)的放大

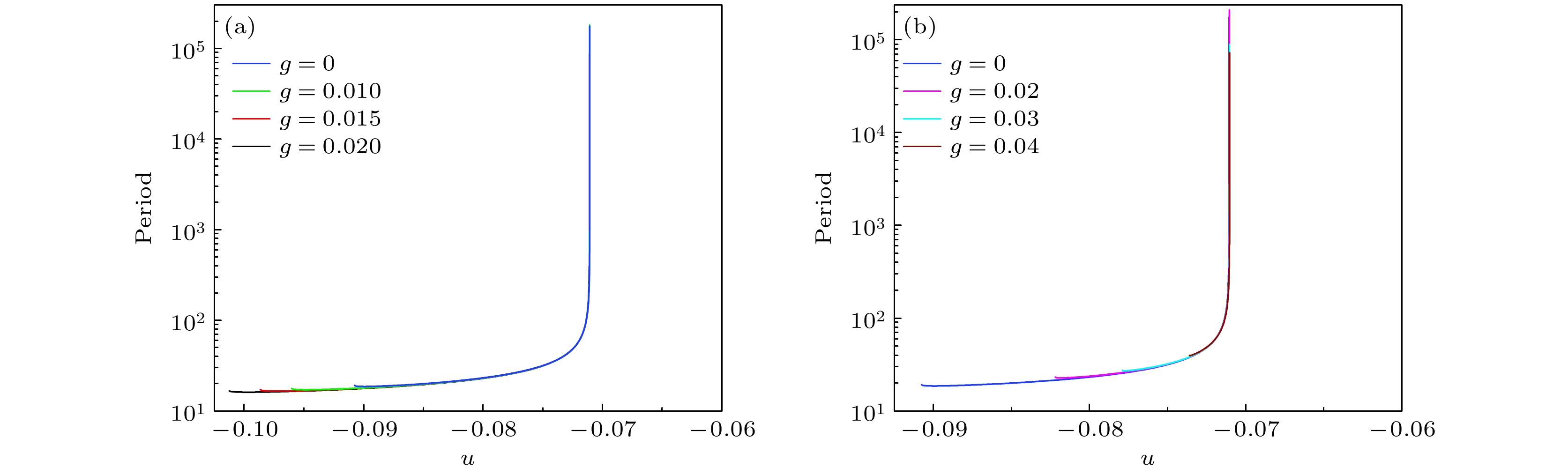
Figure 7. Fast/slow variable dissection of bursting at different values of the inhibitory autapse conductance: (a1) $ g = 0 $, and the numbers 1–6 represent the 1^st–6^th spikes within a burst; (a2) enlargement of Fig. (a1); (b1) $ g = 0.01 $; (b2) enlargement of Fig. (b1); (c1) $ g = 0.015 $; (c2) enlargement of Fig. (c1); (d1) $ g = 0.02 $; (d2) enlargement of Fig. (d1).

DownLoad: Full-Size Img PowerPoint

图 8 不同兴奋性自突触电导强度下簇放电的快慢变量分离　(a) $ g = 0 $; (b) $ g = 0.02 $; (c) $ g = 0.03 $; (d) $ g = 0.04 $

Figure 8. Fast/slow variable dissection of the bursting at different values of the excitatory autapse conductance: (a) $ g = 0 $; (b) $ g = 0.02 $; (c) $ g = 0.03 $; (d) $ g = 0.04 $.

DownLoad: Full-Size Img PowerPoint

图 9 快子系统的极限环的周期随分岔参数u的变化　(a) 抑制性自突触, 蓝, 绿, 红和黑色曲线分别对应$ g = 0 $, $ g = 0.01 $, $ g = 0.015 $和$ g = 0.02 $; (b) 兴奋性自突触, 蓝、粉、青和棕色曲线分别对应g = 0, g = 0.02, g = 0.03和g = 0.04

Figure 9. Period of the limit cycle of the fast system changes with respect to the bifurcation parameter u: (a) Inhibitory autupse. The blue, green, red, and black curves correspond to $ g = 0 $, $ g = 0.01 $, $ g = 0.015 $, and $ g = 0.02 $, respectively. (b) Excitatory autupse. The blue, pink, cyan-blue, and brown curves correspond to g = 0, g = 0.02, g = 0.03, and g = 0.04, respectively.

DownLoad: Full-Size Img PowerPoint

图 10 快子系统在$ (u, {\text{g}}) $平面的双参数分岔　(a1) 抑制性自突触; (a2) 图(a1)的局部放大; (b1) 兴奋性自突触; (b2) 图(b1)的局部放大

Figure 10. Double-parameter bifurcation in $ (u, {\text{g}}) $ plane of the fast subsystem: (a1) Inhibitory autapse; (a2) enlargement of Fig (a1); (b1) excitatory autapse; (b2) enlargement of Fig (b1).

DownLoad: Full-Size Img PowerPoint

map

[1]	Yang Y, Cui Y H, Sang K N, Dong Y Y, NI Z Y, Ma S S, Hu H L 2018 Nature 554 317 Google Scholar
[2]	Wang X J 2010 Phys. Rev. 90 1195 Google Scholar
[3]	Mondal A, Upadhyay R K, Ma J, Yadav B K, Sharma S K, Mondal A 2019 Cogn. Neurodyn. 13 393 Google Scholar
[4]	Glass L 2001 Nature 410 277 Google Scholar
[5]	Izhikevich E M 2000 Int. J. Bifurcation Chaos 10 1171 Google Scholar
[6]	Duan L X, Liang W J, Ji W C, Xi H G 2020 Int. J. Bifurcation Chaos 30 2050192 Google Scholar
[7]	Desroches M, Guckenheimer J, Krauskopf B, Kuehn C, Osinga H M, Wechselberger M 2012 SIAM Rev. 54 211 Google Scholar
[8]	Lu B, Liu S, Jiang X, Jing W, Wang X 2017 Discrete Cont. Dyn. -S 10 487 Google Scholar
[9]	Zhan F B, Liu S Q, Zhang X H, Wang J, Lu B 2018 Nonlinear Dyn. 94 807 Google Scholar
[10]	Lisman J E 1997 Trends Neurosci. 20 38 Google Scholar
[11]	Braun H A, Wissing H, Schäfer K, Hirsch M C 1994 Nature 367 270 Google Scholar
[12]	Gu H G, Pan B B 2015 Front. Comput. Neurosci. 9 108 Google Scholar
[13]	Gu H G, Pan B B, Chen G R, Duan L X 2014 Nonlinear Dyn. 78 391 Google Scholar
[14]	Yin L P, Zheng R, Ke W, He Q, Zhang Y, Li J, Wang B, Mi Z, Long Y S, Rasch M J, Li T, Luan G, Shu Y 2018 Nat. Commun. 9 4890 Google Scholar
[15]	Costa K P, Ulrich E, Michael S, Petra R, Dan R, Peter F, Nikolaos K, Lana K I, Joseph K 2021 Cereb. Cortex 31 2013
[16]	Satterlie R A 1985 Science 229 402 Google Scholar
[17]	Beiderbeck B, Myoga M H, Müller N, Callan A R, Friauf E, Grothe B, Pecka M 2018 Nat. Commun. 9 1771 Google Scholar
[18]	Li Y Y, Gu H G, Ding X L 2019 Nonlinear Dyn. 97 2091 Google Scholar
[19]	华洪涛, 陆博, 古华光 2020 69 090502 Google Scholar Hua H T, Lu B, Gu H G 2020 Acta Phys. Sin. 69 090502 Google Scholar
[20]	杨永霞, 李玉叶, 古华光 2020 69 040501 Google Scholar Yang Y X, Li Y Y, Gu H G 2020 Acta Phys. Sin. 69 040501 Google Scholar
[21]	Wu F Q, Gu H G 2020 Int. J. Bifurcation Chaos 30 2030009 Google Scholar
[22]	Zhao Z G, Li L, Gu H G 2018 Front. Cell. Neurosci. 12 62 Google Scholar
[23]	Dodla R, Rinzel J 2006 Phys. Rev. E 73 010903 Google Scholar
[24]	Uzuntarla M, Torres J J, Calim A, Barreto E 2019 Neural Networks 110 131 Google Scholar
[25]	Elson R C, Selverston A I, Abarbanel H D I, Rabinovich M I 2002 J. Neurophysiol. 88 1166 Google Scholar
[26]	Jia B, Wu Y, He D, Guo B H, Xue L 2018 Nonlinear Dyn. 93 1599 Google Scholar
[27]	Yao C G, He Z W, Nakano T, Qian Y, Shuai J W 2019 Nonlinear Dyn. 97 1425 Google Scholar
[28]	Jia Y B, Gu H G, Li Y Y, Ding X L 2021 Commun. Nonlinear Sci. Numer. Simul. 95 105643 Google Scholar
[29]	Van Der Loos H, Glaser E M 1972 Brain Res. 48 355 Google Scholar
[30]	Bacci A, Huguenard J R, Prince D A 2003 J. Neurosci. 23 859 Google Scholar
[31]	Bacci A, Huguenard J R 2006 Neuron 49 119 Google Scholar
[32]	Saada R, Miller N, Hurwitz I, Susswein A J 2009 Curr. Biol. 19 479 Google Scholar
[33]	Szegedi V, Paizs M, Baka J, Barzó P, Molnár G, Tamas G, Lamsa K 2020 Elife 9 e51691 Google Scholar
[34]	Wang H T, Ma J, Chen Y L, Chen Y 2014 Commun. Nonlinear Sci. Numer. Simul. 19 3242 Google Scholar
[35]	Guo D Q, Chen M M, Perc M, Wu S D, Xia C, Zhang Y S, Xu P, Xia Y, Yao D Z 2016 Europhys. Lett. 114 30001 Google Scholar
[36]	Ge M Y, Xu Y, Zhang Z K, Peng Y X, Kang W J, Yang L J, Jia Y 2018 Eur. Phys. J. Spec. Top. 227 799 Google Scholar
[37]	Song X L, Wang H T, Chen Y 2019 Nonlinear Dyn. 96 2341 Google Scholar
[38]	Wu Y N, Gong Y B, Wang Q 2015 Chaos 25 043113 Google Scholar
[39]	丁学利, 贾冰, 李玉叶 2019 68 180502 Google Scholar Ding X L, Jia B, Li Y Y 2019 Acta Phys. Sin. 68 180502 Google Scholar
[40]	曲良辉, 都琳, 曹子露, 胡海威, 邓子辰 2020 69 230501 Google Scholar Qu L H, Du L, Cao Z L, Hu H W, Deng Z C 2020 Acta Phys. Sin. 69 230501 Google Scholar
[41]	Ma J, Song X L, Tang J, Wang C N 2015 Neurocomputing 167 378 Google Scholar
[42]	Yang X L, Yu Y H, Sun Z K 2017 Chaos 27 083117 Google Scholar
[43]	Zhang X H, Liu S Q 2018 Chin. Phys. B 27 040501 Google Scholar
[44]	Tikidji-Hamburyan R A, Martinez J J, White J A, Canavier C C 2015 J. Neurosci. 35 15682 Google Scholar
[45]	Zhao Z G, Li L, Gu H G, Gao Y 2020 Nonlinear Dyn. 99 1129 Google Scholar
[46]	Deleuze C, Bhumbra G S, Pazienti A, Lourenco J, Mailhes C, Aguirre A, Beato M, Bacci A 2019 PLoS Biol. 17 e3000419 Google Scholar
[47]	Connelly W M 2014 PLoS ONE 9 e89995 Google Scholar
[48]	Zhao Z G, Li L, Gu H G 2020 Commun. Nonlinear Sci. Numer. Simul. 85 105250 Google Scholar
[49]	丁学利, 李玉叶 2016 65 210502 Google Scholar Ding X L, Li Y Y 2016 Acta Phys. Sin. 65 210502 Google Scholar
[50]	曹奔, 关利南, 古华光 2018 67 240502 Google Scholar Cao B, Guan L N, Gu H G 2018 Acta Phys. Sin. 67 240502 Google Scholar
[51]	Li Y Y, Gu H G, Jia B, Ding X L 2021 Sci. China Ser. E: Technol. Sci. 64 1459
[52]	Zhao Z G, Jia B, Gu H G 2016 Nonlinear Dyn. 86 1549 Google Scholar
[53]	Ermentrout B 2002 Simulating, Analyzing, and Animating Dynamical Systems: A Guide to XPPAUT for Researchers and Students (Philadelphia: SIAM) pp161−194
[54]	Xu Y, Liu M H, Zhu Z G, Ma J 2020 Chin. Phys. B 29 098704 Google Scholar
[55]	Zhang X J, Gu H G, Guan L N 2019 Sci. China Ser. E:Technol. Sci. 62 1502 Google Scholar
[56]	Wang Z L, Shi X R 2020 Cogn. Neurodyn. 14 115 Google Scholar

[1]	Liang Yan-Mei, Lu Bo, Gu Hua-Guang. Analysis to dynamics of complex electrical activities in Wilson model of brain neocortical neuron using fast-slow variable dissection with two slow variables. Acta Physica Sinica, 2022, 71(23): 230502. doi: 10.7498/aps.71.20221416
[2]	Zhao Ya-Qi, Liu Mou-Tian, Zhao Yong, Duan Li-Xia. Dynamics of mixed bursting in coupled pre-Bötzinger complex. Acta Physica Sinica, 2021, 70(12): 120501. doi: 10.7498/aps.70.20210093
[3]	Yang Hong-Li, Liu Nan, Yang Lian-Gui. Influence of Mdm2-mediated positive feedback loop on the oscillation behavior of p53 gene network. Acta Physica Sinica, 2021, 70(13): 138701. doi: 10.7498/aps.70.20210015
[4]	Zheng Lai-Yun, Zhao Bing-Xin, Yang Jian-Qing. Bifurcation and nonlinear evolution of convection in binary fluid mixtures with weak Soret effect. Acta Physica Sinica, 2020, 69(7): 074701. doi: 10.7498/aps.69.20191836
[5]	Yang Yong-Xia, Li Yu-Ye, Gu Hua-Guang. Synchronization transition from bursting to spiking and bifurcation mechanism of the pre-Bötzinger complex. Acta Physica Sinica, 2020, 69(4): 040501. doi: 10.7498/aps.69.20191509
[6]	Cao Ben, Guan Li-Nan, Gu Hua-Guang. Bifurcation mechanism of not increase but decrease of spike number within a neural burst induced by excitatory effect. Acta Physica Sinica, 2018, 67(24): 240502. doi: 10.7498/aps.67.20181675
[7]	Xie Yong, Cheng Jian-Hui. A direct algorithm with square wave perturbation for calculating phase response curve. Acta Physica Sinica, 2017, 66(9): 090501. doi: 10.7498/aps.66.090501
[8]	Li Jia-Jia, Wu Ying, Du Meng-Meng, Liu Wei-Ming. Dynamic behavior in firing rhythm transitions of neurons under electromagnetic radiation. Acta Physica Sinica, 2015, 64(3): 030503. doi: 10.7498/aps.64.030503
[9]	Yang Yi-Wei, Liu Jia-Lin, Li Bin. Bifurcation of proportion-integration-based voltage-mode Buck converter. Acta Physica Sinica, 2014, 63(4): 040502. doi: 10.7498/aps.63.040502
[10]	Meng Zong, Fu Li-Yuan, Song Ming-Hou. Bifurcation of a kind of nonlinear-relative rotational system with combined harmonic excitation. Acta Physica Sinica, 2013, 62(5): 054501. doi: 10.7498/aps.62.054501
[11]	Zhang Fang-Ying, Yang Ru, Long Xiao-Li, Xie Chen-Yue, Chen Hong. Mechanism of instability behaviors and stabilization on V2 controlled buck converter. Acta Physica Sinica, 2013, 62(21): 218404. doi: 10.7498/aps.62.218404
[12]	Lu Yan-Ling, Jiang Guo-Ping, Song Yu-Rong. Stability and bifurcation of epidemic spreading on adaptive network. Acta Physica Sinica, 2013, 62(13): 130202. doi: 10.7498/aps.62.130202
[13]	Wang Fu-Xia, Xie Yong. Synchronization of "Hopf/homoclinic" bursting with "SubHopf/homoclinic" bursting. Acta Physica Sinica, 2013, 62(2): 020509. doi: 10.7498/aps.62.020509
[14]	Ji Ying, Bi Qin-Sheng. Bifurcation analysis of slow-fast behavior in modified Chua's circuit. Acta Physica Sinica, 2012, 61(1): 010202. doi: 10.7498/aps.61.010202
[15]	Yang Zhuo-Qin, Guan Ting-Ting, Gan Chun-Biao, Zhang Jiao-Ying. Study on bursting of pancreatic cells in codimension-2 bifurcation regions. Acta Physica Sinica, 2011, 60(11): 110202. doi: 10.7498/aps.60.110202
[16]	Zhang Bo, Xie Fan, Yang Ru. Study on border collision and bifurcation of two-dimensional piecewise smooth systems in current mode controlled Buck converter. Acta Physica Sinica, 2010, 59(12): 8393-8406. doi: 10.7498/aps.59.8393
[17]	Chen Zhang-Yao, Bi Qin-Sheng. Bifurcations and chaos of coupled Jerk systems. Acta Physica Sinica, 2010, 59(11): 7669-7678. doi: 10.7498/aps.59.7669
[18]	Zhang Xiao-Fang, Chen Zhang-Yao, Bi Qin-Sheng. Complicated oscillations in coupled electrical circuits. Acta Physica Sinica, 2009, 58(5): 2963-2970. doi: 10.7498/aps.58.2963
[19]	Ma Xi-Kui, Yang Mei, Zou Jian-Long, Wang Ling-Tao. Study of complex behavior in a time-delayed van der Pol’s electromagnetic system (Ⅰ)——The phenomena of bifurcations and chaos. Acta Physica Sinica, 2006, 55(11): 5648-5656. doi: 10.7498/aps.55.5648
[20]	Luo Xiao-Shu, Wang Bing-Hong, Guanrong Chen, Quan Hong-Jun, Fang Jin-Qing, Zou Yan-Li, Jiang Pin-Qun. Research on bifurcation behaviour and chaos control in DC-DC buck converter. Acta Physica Sinica, 2003, 52(1): 12-17. doi: 10.7498/aps.52.12

Catalog

Vol. 70, Issue 17 - 2021-09-05

Metrics

Abstract views: 4802
PDF Downloads: 104
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板