基于不同流动时间占比的紧凑式室温磁制冷系统实验研究

李瑞; 沈俊; 张志鹏; 李振兴; 莫兆军; 高新强; 海鹏; 付琪

doi:10.7498/aps.73.20231066

摘要

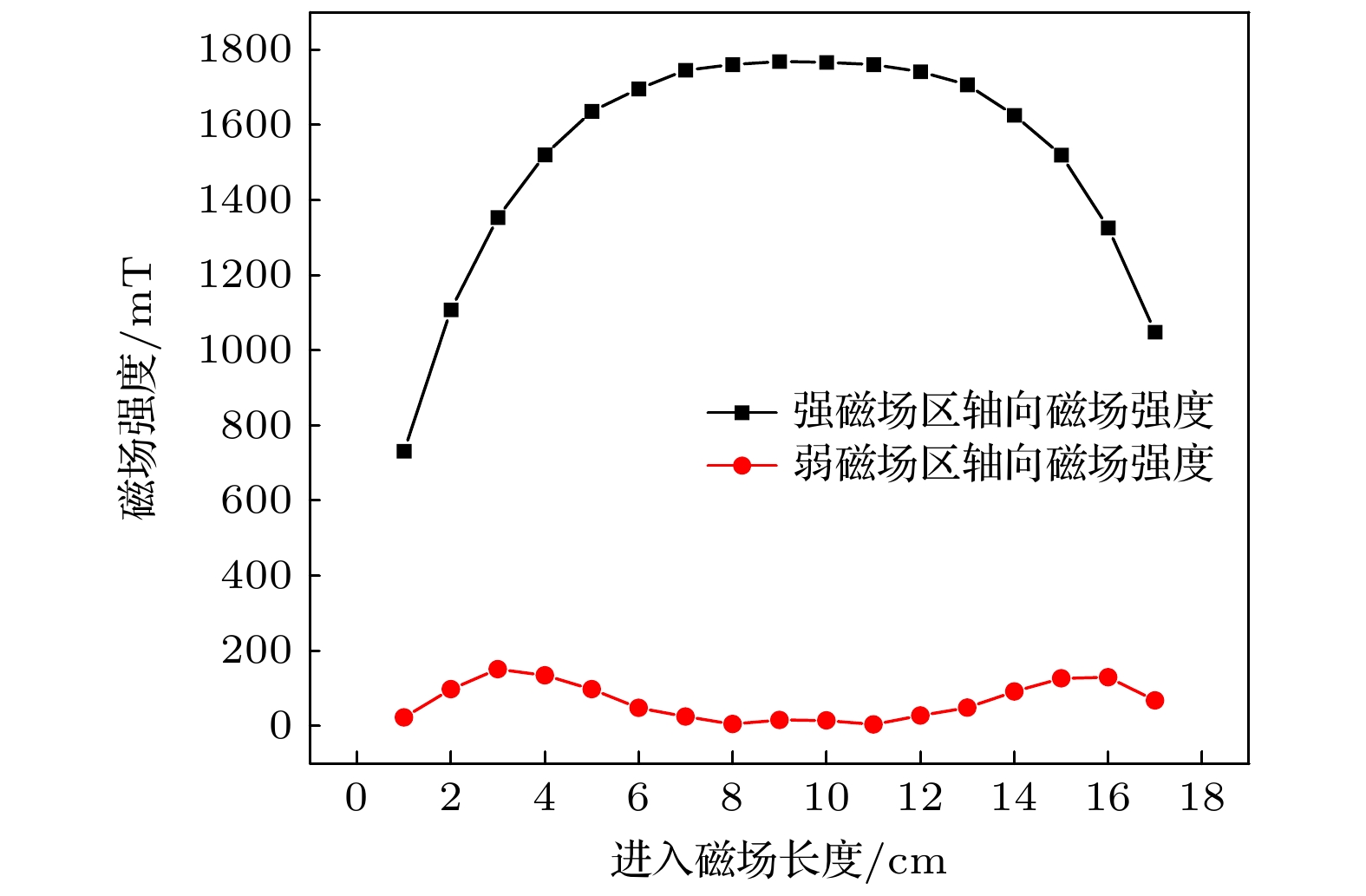
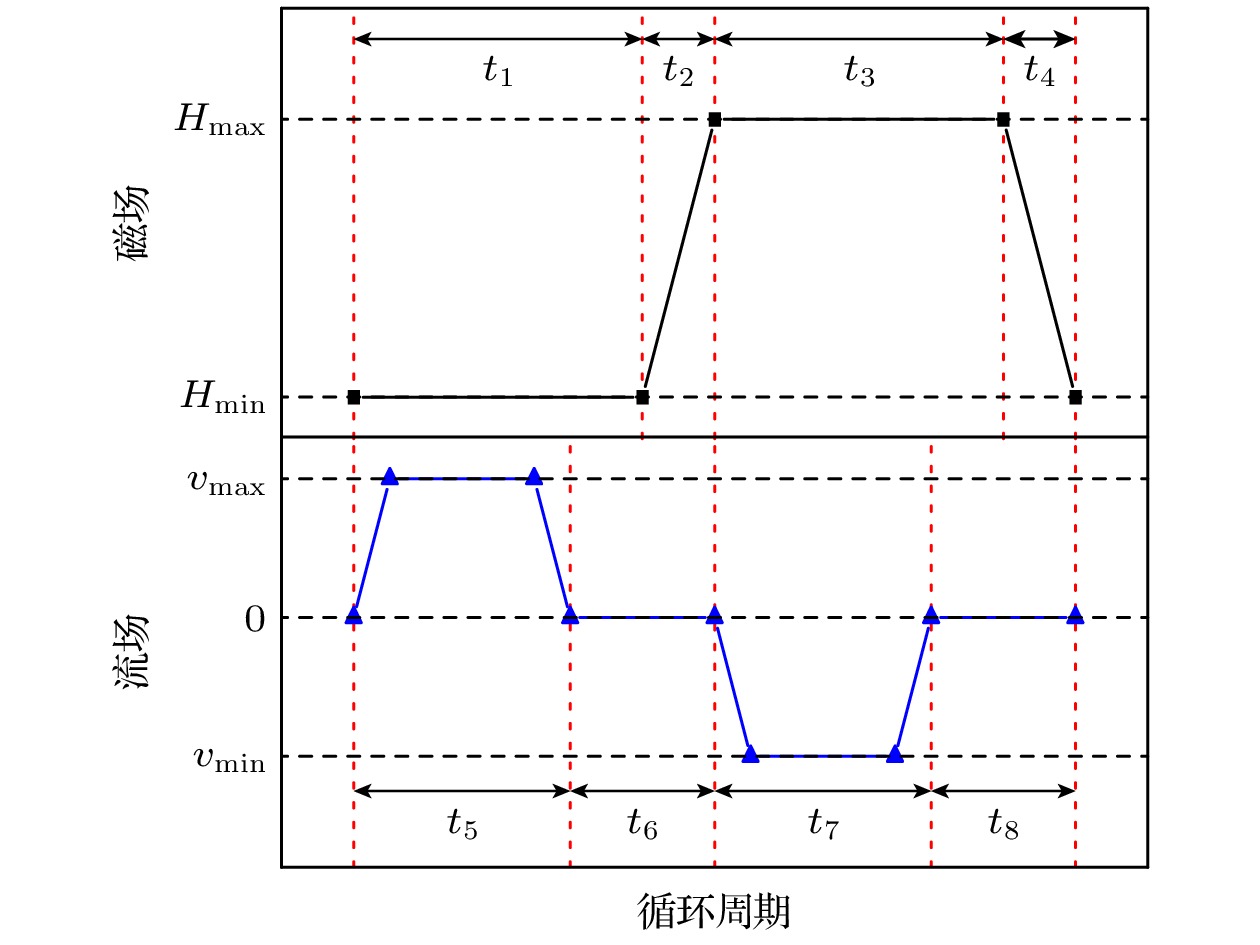
磁制冷因高效、环保、结构简单等特点, 正有望成为替代传统蒸气制冷的新型室温制冷技术之一. 当前针对单一影响因子对磁制冷样机的影响作用规律研究较为丰富, 但对磁场-流场时序中流动时间占比工况的研究较少, 且影响规律尚不明晰. 本文以前期研制的紧凑型室温磁制冷系统为基础, 在固定的磁场时序情况下开展不同流动时间占比的实验研究, 探索制冷温跨、制冷量、压降及性能系数与流动时间占比之间的关联. 以磁场时序1∶4∶1∶4与频率0.45 Hz的工况为例, 开展了固定磁场时序的不同流动时间占比(100%, 80%, 60%)的实验研究. 结果表明: 1)小利用系数和高流动时间的组合可获得较大温跨, 大利用系数和高流动时间占比的组合可获得较大冷量, 其中当利用系数为0.42、流动时间占比为100%时, 获得最大无负荷制冷温跨26.2 K; 2)对比研究了利用系数与流动时间占比对回热器压降及性能系数的影响, 流动时间占比的增大和利用系数的减小均会造成流体速度的减小, 会使压降进一步减小, 性能系数进一步增大. 本研究阐明了制冷温跨、制冷量、压降及性能系数与流动时间占比之间的影响规律, 为进一步提升室温磁制冷机的性能奠定基础.

关键词:

Abstract

Magnetic refrigeration has become a promising new technology to replace conventional vapor-compression refrigeration technology, for it has excellent application characteristics such as the high efficiency, environmental friendliness and structural simplicity. Many studies have been carried out to analyze the various subsystems, but the interaction laws between the systems are not yet clear, and the optimization of each subsystem is still an area of research worth exploring. This work is based on a compact room temperature magnetic refrigeration system developed before, and carries out experimental research on the different flow time ratio to explore the correlation among refrigeration temperature span, cooling capacity, pressure drop, coefficient of performance (COP) and blow fraction under a fixed magnetic field timing. Especially, the effects of different flow time ratios (100%, 80%, 60%) on the system performance are studied under magnetic field timing of 1∶4∶1∶4 and a frequency of 0.45 Hz. The experimental results reveal that a low utilization factor combined with a high flow time ratio can achieve a greater temperature spread, whereas a high utilization factor combined with a high flow time ratio can accomplish a bigger cooling capacity. When the utilization factor is 0.42 and the flow time ratio is 100%, the maximum unloaded cooling temperature span is 26.2 K. Meanwhile, the effects of the utilization factor and flow time ratio on the pressure drop and COP of the regenerator are studied in detail. It is discovered that raising the flow time ratio and reducing the utilization factor both result in a fall in fluid velocity, which leads the pressure to further decrease and the COP to rise. In a word, this research investigates the relationship among cooling temperature span, cooling capacity, pressure drop, COP, and flow time ratio in a fixed magnetic field timing, thus providing the groundwork for future improving the performances of room temperature magnetic refrigeration systems.

Keywords:

作者及机构信息

1.
中国科学院赣江创新研究院, 赣州　341119

2.
北京理工大学, 北京　100081

通信作者: 沈俊, jshen@mail.ipc.ac.cn ; 李振兴, zxli@bit.edu.cn ;

基金项目: 国家重点研发计划(批准号: 2022YFB3505100)、国家自然科学基金(批准号: 51925605, 52206034)和中国科学院科研仪器设备研制项目(批准号: YJKYYQ20200076)资助的课题.

Authors and contacts

1.
Ganjiang Innovation Academy, Chinese Academy of Sciences, Ganzhou 341119, China

2.
Beijing Institute of Technology, Beijing 100081, China

Corresponding author: Shen Jun, jshen@mail.ipc.ac.cn ; Li Zhen-Xing, zxli@bit.edu.cn ;

Funds: Project supported by the the National Key R&D Program of China (Grant No. 2022YFB3505100), the National Natural Science Foundation of China (Grant Nos. 51925605, 52206034), and the Scientific Instrument Developing Project of the Chinese Academy of Sciences (Grant No. YJKYYQ20200076).

文章全文 : translate this paragraph

参考文献

[1]	Franco G, Victorino, Blázquez G, Javier S, Ipus B, Jhon J, Law, Jia Y, Moreno R, Luis M, Conde A, Alejandro 2018 Prog. Mater. Sci. 93 112 Google Scholar
[2]	李连生 2011 制冷学报 32 53 Google Scholar Li L S 2011 J. Refrig. 32 53 Google Scholar
[3]	张朝晖, 陈敬良, 高钰, 刘晓红 2015 制冷与空调 15 1 Google Scholar Zhang Z H, Chen J L, Gao Y, Liu X H 2015 Refrig. Air-Conditioning 15 1 Google Scholar
[4]	DiPirro M, Tuttle J, Jackson M, Canavan E, Warner B, Shirron P 2006 AIP Conf. Proc. 823 969 Google Scholar
[5]	Warburg E 1881 Ann. Phys. 249 141 Google Scholar
[6]	Brown G V 1976 J. Appl. Phys. 47 3673 Google Scholar
[7]	Zimm C, Boeder A, Chell J, Sternberg A, Fujita A, Fujieda S, Fukamichi K 2006 Int. J. Refrig. 29 1302 Google Scholar
[8]	Jacobs S, Auringer J, Boeder A, Chell J, Komorowski L, Leonard J, Russek S, Zimm C 2014 Int. J. Refrig. 37 84 Google Scholar
[9]	Nakashima A T D, Dutra S L, Trevizoli P V, Barbosa J R 2018 Int. J. Refrig. 93 159 Google Scholar
[10]	Nakashima A T D, Dutra S L, Trevizoli P V, Barbosa J R 2018 Int. J. Refrig. 93 236 Google Scholar
[11]	李振兴, 李珂, 沈俊, 戴巍, 贾际深, 郭小惠, 高新强, 公孙琼 2017 低温工程 215 13 Li Z X, Li K, Shen J, Dai W, Jia J C, Guo X H, Gao X Q, Gong S M 2017 Cryogenics 215 13
[12]	Li Z X, Li K, Guo X H, Gao X Q, Dai W, Gong S M, Shen J 2021 Appl. Therm. Eng. 187 116477 Google Scholar
[13]	于世霖, 赵金良, 李振兴, 海鹏, 李珂, 莫兆军, 高新强, 戴巍, 沈俊 2022 工程 43 3204 Yu S L, Zhao J L, Li Z X, Hai P, Li K, Mo Z J, Gao X Q, Dai W, Shen J 2022 J. Eng. Thermophys. 43 3204
[14]	Hai P, Shen J, Li Z X, Li K, Huang H M, Zheng W S, Dai W, Gao X Q, Mo Z J 2023 Appl. Therm. Eng. 219 119561 Google Scholar
[15]	Tušek J, Kitanovski A, Zupan S, Prebil I, Poredoš A 2013 Appl. Therm. Eng. 53 57 Google Scholar
[16]	Trevizoli P V, Nakashima A T, Peixer G F, Barbosa J R 2017 Appl. Energy 187 847 Google Scholar
[17]	Bjørk R, Bahl C R H, Smith A, Christensen D V, Pryds N 2010 J. Magn. Magn. Mater. 322 3324 Google Scholar
[18]	Teyber R, Trevizoli P V, Christiaanse T V, Govindappa P, Niknia I, Rowe A 2017 J. Magn. Magn. Mater. 442 87 Google Scholar
[19]	Lei T, Engelbrecht K, Nielsen K K, Veje C T 2017 Appl. Therm. Eng. 111 1232 Google Scholar
[20]	You Y H, Guo Y, Xiao S F, Yu S, Ji H, Luo X B 2016 J. Magn. Magn. Mater. 405 231 Google Scholar

施引文献

图 1 室温磁制冷系统原理图

Fig. 1. Schematic diagram of room temperature magnetic refrigeration system.

下载: 全尺寸图片幻灯片

图 2 双层同心Halbach永磁体组横截面示意图^[13]

Fig. 2. Schematic diagram of the cross-section of the double-layer concentric Halbach magnet group^[13].

下载: 全尺寸图片幻灯片

图 3 双层同心Halbach磁体组轴线中心处磁场强度示意图

Fig. 3. Schematic diagram of the magnetic field intensity at the center of the axis of the double-layer concentric Halbach magnet group.

下载: 全尺寸图片幻灯片

图 4 磁场及流场波形图

Fig. 4. Time diagram of magnetic field and fluid flow rate.

下载: 全尺寸图片幻灯片

图 5 (a)运行频率0.45 Hz、流动时间占比F_B = 100%, 利用系数U = 0.42的工况下整机系统的降温(蓝色)和升温曲线(红色); (b)—(d)分别在F_B = 100%, 80%, 60%流动时间占比下, 不同利用系数下制冷温跨随制冷量的变化曲线

Fig. 5. (a) Cooling (blue) and heating (red) curves of the entire system under the operating condition with 0.45 Hz, F_B = 100%, U = 0.42; (b)–(d) the variation curve of cooling temperature difference with refrigeration capacity under F_B = 100%, 80%, 60%.

下载: 全尺寸图片幻灯片

图 6 三种流动时间占比F_B, 无负荷工况下制冷温跨随利用系数的变化曲线

Fig. 6. For the three flow time ratios, refrigeration temperature span vs. utilization factor change curve under no-load conditions.

下载: 全尺寸图片幻灯片

图 7 不同热载荷下, 最大制冷温跨所对应的利用系数

Fig. 7. Utilization factor corresponding to the maximum refrigeration temperature span at different heat loads.

下载: 全尺寸图片幻灯片

图 8 (a)不同流动时间占比下回热器压降与利用系数的关系曲线; (b)不同流动时间占比下COP与利用系数的关系曲线, 插图显示不同流动时间占比下输入电功率与利用系数的关系曲线

Fig. 8. (a) Variation curves of pressure drop with utilization factor under different flow time ratios; (b) variation curves of COP with utilization factor under different flow time ratios, the inset figure shows variation curves of input electrical power with utilization factor under different flow time ratios.

下载: 全尺寸图片幻灯片

map

[1]	Franco G, Victorino, Blázquez G, Javier S, Ipus B, Jhon J, Law, Jia Y, Moreno R, Luis M, Conde A, Alejandro 2018 Prog. Mater. Sci. 93 112 Google Scholar
[2]	李连生 2011 制冷学报 32 53 Google Scholar Li L S 2011 J. Refrig. 32 53 Google Scholar
[3]	张朝晖, 陈敬良, 高钰, 刘晓红 2015 制冷与空调 15 1 Google Scholar Zhang Z H, Chen J L, Gao Y, Liu X H 2015 Refrig. Air-Conditioning 15 1 Google Scholar
[4]	DiPirro M, Tuttle J, Jackson M, Canavan E, Warner B, Shirron P 2006 AIP Conf. Proc. 823 969 Google Scholar
[5]	Warburg E 1881 Ann. Phys. 249 141 Google Scholar
[6]	Brown G V 1976 J. Appl. Phys. 47 3673 Google Scholar
[7]	Zimm C, Boeder A, Chell J, Sternberg A, Fujita A, Fujieda S, Fukamichi K 2006 Int. J. Refrig. 29 1302 Google Scholar
[8]	Jacobs S, Auringer J, Boeder A, Chell J, Komorowski L, Leonard J, Russek S, Zimm C 2014 Int. J. Refrig. 37 84 Google Scholar
[9]	Nakashima A T D, Dutra S L, Trevizoli P V, Barbosa J R 2018 Int. J. Refrig. 93 159 Google Scholar
[10]	Nakashima A T D, Dutra S L, Trevizoli P V, Barbosa J R 2018 Int. J. Refrig. 93 236 Google Scholar
[11]	李振兴, 李珂, 沈俊, 戴巍, 贾际深, 郭小惠, 高新强, 公孙琼 2017 低温工程 215 13 Li Z X, Li K, Shen J, Dai W, Jia J C, Guo X H, Gao X Q, Gong S M 2017 Cryogenics 215 13
[12]	Li Z X, Li K, Guo X H, Gao X Q, Dai W, Gong S M, Shen J 2021 Appl. Therm. Eng. 187 116477 Google Scholar
[13]	于世霖, 赵金良, 李振兴, 海鹏, 李珂, 莫兆军, 高新强, 戴巍, 沈俊 2022 工程 43 3204 Yu S L, Zhao J L, Li Z X, Hai P, Li K, Mo Z J, Gao X Q, Dai W, Shen J 2022 J. Eng. Thermophys. 43 3204
[14]	Hai P, Shen J, Li Z X, Li K, Huang H M, Zheng W S, Dai W, Gao X Q, Mo Z J 2023 Appl. Therm. Eng. 219 119561 Google Scholar
[15]	Tušek J, Kitanovski A, Zupan S, Prebil I, Poredoš A 2013 Appl. Therm. Eng. 53 57 Google Scholar
[16]	Trevizoli P V, Nakashima A T, Peixer G F, Barbosa J R 2017 Appl. Energy 187 847 Google Scholar
[17]	Bjørk R, Bahl C R H, Smith A, Christensen D V, Pryds N 2010 J. Magn. Magn. Mater. 322 3324 Google Scholar
[18]	Teyber R, Trevizoli P V, Christiaanse T V, Govindappa P, Niknia I, Rowe A 2017 J. Magn. Magn. Mater. 442 87 Google Scholar
[19]	Lei T, Engelbrecht K, Nielsen K K, Veje C T 2017 Appl. Therm. Eng. 111 1232 Google Scholar
[20]	You Y H, Guo Y, Xiao S F, Yu S, Ji H, Luo X B 2016 J. Magn. Magn. Mater. 405 231 Google Scholar

[1]	王宇枭, 成泽帅, 江可扬, 魏琳扬, 历秀明. 基于辐射制冷与电致变色的可调节多层膜性能研究. , 2024, 73(21): 214401. doi: 10.7498/aps.73.20240863
[2]	郑茂文, 郭浩文, 卫铃佼, 潘子杰, 邹佳润, 李瑞鑫, 赵密广, 陈厚磊, 梁惊涛. 稀释制冷技术. , 2024, 73(23): 230701. doi: 10.7498/aps.73.20241211
[3]	俎红叶, 程维军, 王亚男, 王晓涛, 李珂, 戴巍. 冷凝泵型稀释制冷机实验研究. , 2023, 72(8): 080701. doi: 10.7498/aps.72.20222257
[4]	刘旭明, 潘长钊, 张宇, 廖奕, 郭伟杰, 俞大鹏. 4 K大冷量GM型脉冲管制冷机. , 2023, 72(19): 190701. doi: 10.7498/aps.72.20230910
[5]	阳润恒, 安顺, 尚文, 邓涛. 仿生辐射制冷的研究进展. , 2022, 71(2): 024401. doi: 10.7498/aps.71.20211854
[6]	徐帅, 杨贇贇, 刘行, 何济洲. 基于一维弹道导体的三端纳米线制冷机的性能优化. , 2022, 71(2): 020501. doi: 10.7498/aps.71.20211077
[7]	付柏山, 廖奕, 周俊. 稀释制冷机及其中的热交换问题. , 2021, 70(23): 230202. doi: 10.7498/aps.70.20211760
[8]	陈浩, 王存海, 程子明, 魏琳扬, 王富强, 张欣欣. 基于辐射制冷-温室效应的热电系统性能分析. , 2021, 70(21): 214401. doi: 10.7498/aps.70.20210356
[9]	何济洲, 徐帅. 基于一维弹道导体的三端纳米线制冷机的性能优化. , 2021, (): . doi: 10.7498/aps.70.20211077
[10]	王昌, 李珂, 沈俊, 戴巍, 王亚男, 罗二仓, 沈保根, 周远. 用于亚开温区的极低温绝热去磁制冷机. , 2021, 70(9): 090702. doi: 10.7498/aps.70.20202237
[11]	李唯, 符婧, 杨贇贇, 何济洲. 光子驱动量子点制冷机. , 2019, 68(22): 220501. doi: 10.7498/aps.68.20191091
[12]	张荣, 卢灿灿, 李倩文, 刘伟, 白龙. 线性不可逆热力学框架下一个无限尺寸热源而有限尺寸冷源的制冷机的性能分析. , 2018, 67(4): 040502. doi: 10.7498/aps.67.20172010
[13]	李振兴, 李珂, 沈俊, 戴巍, 高新强, 郭小惠, 公茂琼. 室温磁制冷技术的研究进展. , 2017, 66(11): 110701. doi: 10.7498/aps.66.110701
[14]	高新强, 沈俊, 和晓楠, 唐成春, 戴巍, 李珂, 公茂琼, 吴剑峰. 耦合高压斯特林制冷效应的复合磁制冷循环的数值模拟. , 2015, 64(21): 210201. doi: 10.7498/aps.64.210201
[15]	何弦, 何济洲, 肖宇玲. 四能级量子制冷循环. , 2012, 61(15): 150302. doi: 10.7498/aps.61.150302
[16]	罗小光, 何济洲. 摇摆型棘齿热电子隧穿制冷. , 2011, 60(9): 090506. doi: 10.7498/aps.60.090506
[17]	贺兵香, 何济洲, 缪贵玲. 纳米线异质结构对电子制冷机性能的影响. , 2011, 60(4): 040509. doi: 10.7498/aps.60.040509
[18]	孙海生, 贾佑华, 纪宪明, 印建平. Tm3+掺杂光纤激光制冷的理论分析. , 2006, 55(6): 3112-3118. doi: 10.7498/aps.55.3112
[19]	何济洲, 王磊, 李俊彬. 量子简并性对气体斯特林制冷循环性能的影响. , 2005, 54(1): 24-29. doi: 10.7498/aps.54.24
[20]	秦伟平, 秦冠仕, 张继森, 吴长锋, 王继伟, 杜国同. 单分子-光子制冷泵的热力学行为. , 2001, 50(8): 1467-1474. doi: 10.7498/aps.50.1467

计量

文章访问数: 2354
PDF下载量: 55
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

基于不同流动时间占比的紧凑式室温磁制冷系统实验研究