-
基于密度泛函理论的第一性原理平面波赝势法, 结合广义梯度近似(GGA + U), 系统研究了Ge-S/F共掺杂对Li2MSiO4 (M = Mn, Fe)晶体结构稳定性和电化学性能的影响. 计算结果表明Ge-S/F共掺杂Li2MSiO4 (M = Mn, Fe) 体系在脱锂过程中均会发生Li和M的位置交换, 与Li2MSiO4(M = Mn, Fe) 相比, 掺杂体系具有更好的韧性, 且锂离子在掺杂体系中更容易迁移. 同时发生了位置交换的掺杂体系结构在脱锂过程中大多更为稳定, 尤其是Li2Mn0.5Ge0.5SiO3.5S0.5在整个脱锂过程中体积变化均很小, 说明其具有良好的结构循环稳定性. 此外, Ge-S/F共掺杂均降低了Li2MSiO4 (M = Mn, Fe) 的理论平均脱嵌电压. 结合态密度图和磁矩结果分析表明, Ge-S/F共掺杂可以提高Li2MnSiO4的导电性和延缓Li2MnSiO4体系中Jahn-Teller效应的出现, 有利于提高Li2MnSiO4的结构循环稳定性. 同时, 共掺杂不仅提高了Li2FeSiO4的导电性, 也有利于Li2FeSiO4体系脱出更多的Li+, 特别是Ge-F共掺杂体系有望实现完全脱锂.
-
关键词:
- 第一性原理计算 /
- Li2MSiO4 (M = Mn, Fe) /
- 位置交换 /
- 电化学性能
The effects of Ge-S/F co-doping on the structural stability and electrochemical properties of Li2MSiO4 (M = Mn, Fe) crystal are systematically studied by the first-principle calculations based on density functional theory combined with the generalized gradient approximation (GGA) + U method. The calculation results show that the Ge-S/F co-doping Li2MSiO4 (M = Mn, Fe) system undergoes the site exchange between Li and M in the delithiation process. Compared with Li2MSiO4(M = Mn, Fe), the doped system has good toughness, and lithium ions migrate easily in the doped system. And the doped system with site exchange is more stable in the process of delithium, especially the volume change of Li2Mn0.5Ge0.5SiO3.5S0.5 is very small, indicating that it has good structural cyclic stability. Moreover, the theoretical average deintercalation voltages of Li2MSiO4 (M = Mn, Fe) are reduced by Ge-S/F co-doping. The combination of the density of states with magnetic moment shows that the Ge-S/F co-doping can improve the conductivity of Li2MnSiO4 and delay the appearance of the Jahn-Teller effect in the Li2MnSiO4 system, which is beneficial to the improvement of the structural cycling stability of Li2MnSiO4. Meanwhile, the Ge-S/F co-doping can not only improve the conductivity of Li2FeSiO4, but also facilitate the removal of more Li+ from Li2FeSiO4 system, especially the complete delithium of Ge-F co-doping system is expected to be achieved.-
Keywords:
- first-principles calculation /
- Li2MSiO4 (M = Mn, Fe) /
- site exchange /
- electrochemical properties
[1] Dominko R, Bele M, Kokalj A, Gaberscek, M, Jamnik J 2007 J. Power Sources 174 457
Google Scholar
[2] Sasaki H, Nemoto A, Moriya M, Masahiko M, Mana H, Shingo K, Yuji A, Akira N, Shinichi H 2015 Ceram. Int. 41 S680
Google Scholar
[3] Li Y X, Gong Z L, Yang Y 2007 J. Power Sources 174 528
Google Scholar
[4] Dominko R 2008 J. Power Sources 184 462
Google Scholar
[5] Muraliganth T, Stroukoff K R, Manthiram A 2010 Chem. Mater. 22 5754
Google Scholar
[6] Liu S S, Song L J, Yu B J, Wang C Y, Li M W 2016 Electrochim. Acta 188 145
Google Scholar
[7] Nyten A, Abouimrane A, Armand M, Gustafsson T, Thomas J O 2005 Electrochem. Commun. 7 156
Google Scholar
[8] Arroyo-de Dompablo M E, Armand M, Tarascon J M, Amador U 2006 Electrochem. Commun. 8 1292
Google Scholar
[9] Wang C, Xu Y L, Zhang B F, Ma X N 2019 Solid State Ionics 338 39
Google Scholar
[10] Ma D W, Feng Y Y, Zhang B, Feng J, Pan J H 2021 Scr. Mater. 193 122
Google Scholar
[11] Wang K, Teng G F, Yang J L, Tan R, Duan Y D, Zheng J X, Pan F 2015 J. Mater. Chem. A 3 24437
Google Scholar
[12] Li T, Jiang X T, Gao K, Wang C Y, Li S D 2016 J. Chin. Chem. Soc. 63 800
Google Scholar
[13] Zhu L, Li L, Cheng T M, Xu D S 2015 J. Mater. Chem. A 10 5449
[14] Singh S, Raj A K, Sen R, Johari P, Mitra S 2017 ACS Appl. Mater. Interfaces 9 26885
Google Scholar
[15] Nytén A, Kamali S, Haggstrom L, Torbjorn G, John O T 2006 J. Mater. Chem. 16 2266
Google Scholar
[16] Yan X T, Hou Y H, Huang Y L, Zheng S H, Shi Z Q, Tao X M 2019 J. Electrochem. Soc. 166 A3874
Google Scholar
[17] Yan X T, Hou Y H, Zheng S H, Huang Y L, Shi Z Q, Tao X M 2020 Phys. Chem. Chem. Phys. 22 14712
Google Scholar
[18] Zheng S H, Hou Y H, Guo X L, Huang Y L, Li W, Tao X M 2021 Electrochim. Acta 367 137553
Google Scholar
[19] Kresse G, Furthmüller J 1996 Comput. Mater. Sci. 6 15
Google Scholar
[20] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[21] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[22] Blöchl P E 1994 Phys. Rev. B 50 17953
Google Scholar
[23] Monkhorst H J 1976 Phys. Rev. B 13 5188
Google Scholar
[24] Anisimov V I, Zaanen J, Andersen O K 1991 Phys. Rev. B 44 943
Google Scholar
[25] Feng Y M, Ji R, Ding Z P, Zhang D L, Liang C P, Chen L B, Ivey, Douglas G, Wei W F 2018 Inorg. Chem. 57 3223
Google Scholar
[26] Zeng Y, Chiu H C, Ouyang B, Song J, Zaghib K, Demopoulos G P 2019 J. Mater. Chem. A 7 25399
Google Scholar
[27] Lian D X, Zhao Y H, Hou H, Wang S, Wen Z Q, Zhang Q, Guo Q W 2019 Comput. Mater. Sci. 168 260
Google Scholar
[28] Mouhat F, Coudert F X 2014 Phys. Rev. B 90 224104
Google Scholar
[29] Li L, Zhu L, Xu L H, Cheng T M, Wang W, Li X, Sui Q T 2014 J. Mater. Chem. A 2 4251
Google Scholar
[30] Hill R 1952 Proc. Phys. Soc. 65 349
Google Scholar
[31] Watt J P 1979 J. Appl. Phys. 50 6290
Google Scholar
[32] Pugh S F 1954 Philos. Mag. 45 823
Google Scholar
[33] Frantsevich I N, Voronov F F, Bokuta S A 1983 Naukova Dumka Kiev 43 60
[34] 郑寿红, 李 伟, 姜茗浩, 闫小童, 侯育花, 陶小马 2021 功能材料 52 06084
Google Scholar
Zheng S H, Li W, Jiang M H, Yan X T, Hou Y H, Tao X M 2021 Funct. Mater. 52 06084
Google Scholar
[35] Dominko R, Arcon I, Kodre A, Hanzel D, Gaberšček M 2009 J. Power Sources 189 51
Google Scholar
[36] Zhong G H, Li Y L, Yan P, Liu, Z, Xie M H, Lin H Q 2010 J. Phys. Chem. C 114 3693
Google Scholar
[37] Marianetti C A, Kotliar G, Ceder G 2004 Phy. Rev. Lett. 92 196405
Google Scholar
[38] Brese B N E, O’Keeffe M 1991 Acta Crystallogr. B47 192
-
图 1 (a) 位置交换的LiMn0.5Ge0.5SiO3.5S0.5的晶胞结构; (b) 位置交换的LiMn0.5Ge0.5SiO3.5F0.5; (c) 位置交换的LiFe0.5Ge0.5SiO3.5S0.5; (d) 位置交换的LiFe0.5Ge0.5SiO3.5F0.5
Fig. 1. Crystal cell structure of (a) site exchange LiMn0.5Ge0.5SiO3.5S0.5, (b) site exchange LiMn0.5Ge0.5SiO3.5F0.5, (c) site exchange LiFe0.5Ge0.5SiO3.5S0.5, (d) site exchange LiFe0.5Ge0.5SiO3.5F0.5.
图 2 (a) 初始LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1, 2) 的晶胞体积; (b) 发生位置交换LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1, 2) 的晶胞体积
Fig. 2. (a) The unit cell volume of initial LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1, 2); (b) the unit cell volume of site exchange LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1, 2).
图 3 初始和位置交换LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1, 2) 的晶格常数
Fig. 3. Lattice parameters of initial and site exchange LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1, 2).
图 4 (a) 初始和位置交换Li2Mn0.5Ge0.5SiO3.5R0.5的平均脱嵌电压(R = S, F); (b) 初始和位置交换Li2Fe0.5Ge0.5SiO3.5R0.5的平均脱嵌电压 (R = S, F)
Fig. 4. (a) Average deintercalation voltage of initial and site exchange Li2Mn0.5Ge0.5SiO3.5R0.5 (R = S, F); (b) average deintercalation voltage of initial and site exchange Li2Fe0.5Ge0.5SiO3.5R0.5 (R = S, F).
图 5 (a) 初始LixMn0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2)的TDOS和PDOS; (b) 初始 LixMn0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2) 中 Mn和Ge的PDOS; (c) 位置交换LixMn0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2)的TDOS和PDOS; (d) Mn和Ge在位置交换 LixMn0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2) 中的PDOS
Fig. 5. (a) TDOS and PDOS of initial LixMn0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2); (b) PDOS of Mn and Ge in initial LixMn0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2); (c) TDOS and PDOS of site exchange LixMn0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2); (d) PDOS of Mn and Ge in site exchange LixMn0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2).
图 6 (a) 初始LixMn0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2)的TDOS和PDOS; (b) 初始 LixMn0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2) 中Mn和Ge的 PDOS; (c) 位置交换LixMn0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2)的TDOS和PDOS; (d) 位置交换LixMn0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2) 中的Mn和Ge的PDOS
Fig. 6. (a) TDOS and PDOS of initial LixMn0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2); (b) PDOS of Mn and Ge in initial LixMn0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2); (c) TDOS and PDOS of site exchange LixMn0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2); (d) PDOS of Mn and Ge in site exchange LixMn0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2).
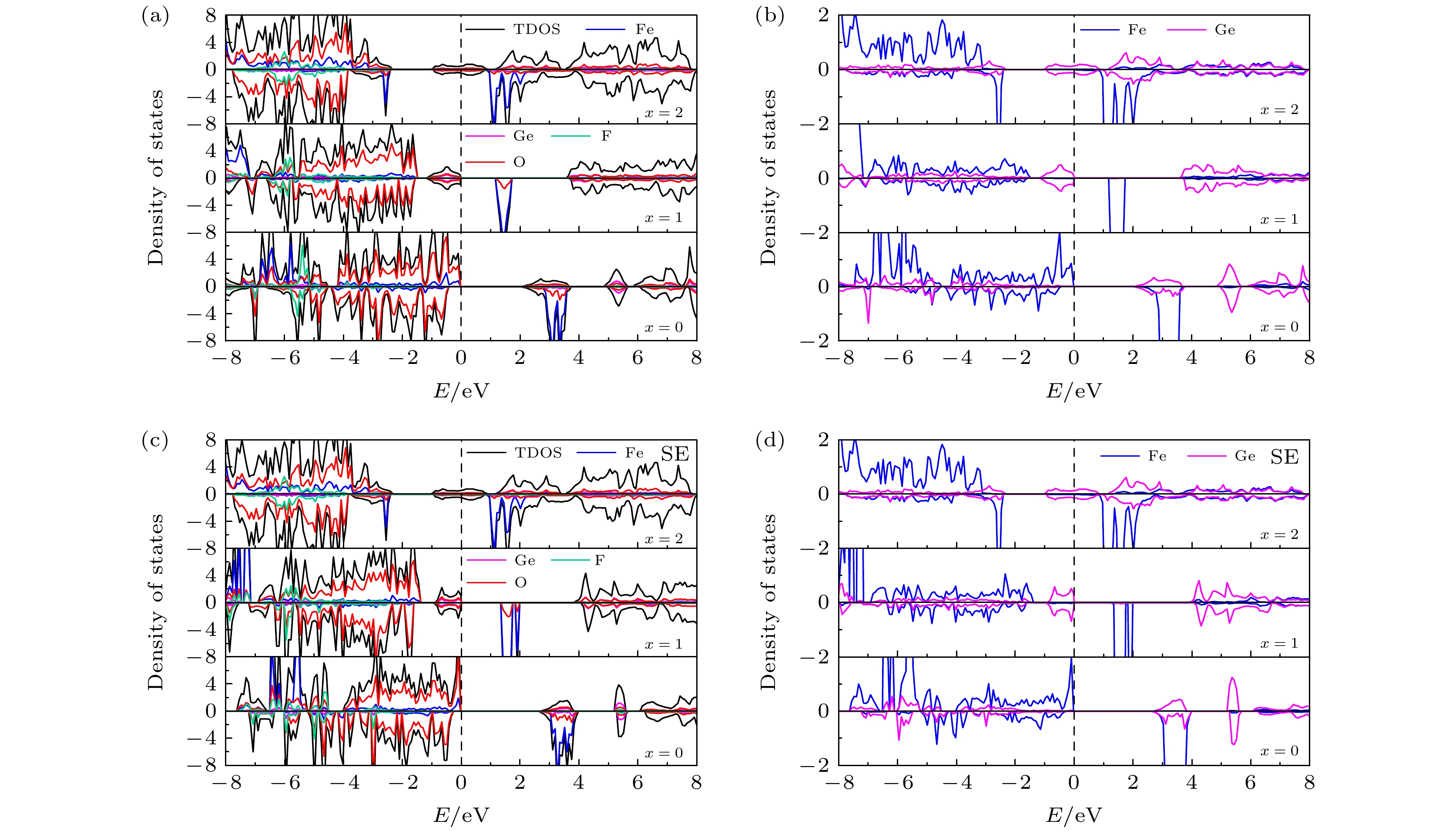
图 7 (a) 初始LixFe0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2)的TDOS和PDOS; (b) 初始 LixFe0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2) 中Fe和Ge的 PDOS; (c) 位置交换LixFe0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2)的TDOS和PDOS; (d) 位置交换LixFe0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2)中Fe和Ge的 PDOS
Fig. 7. (a) TDOS and PDOS of initial LixFe0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2); (b) PDOS of Fe and Ge in initial LixFe0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2); (c) TDOS and PDOS of site exchange LixFe0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2); (d) PDOS of Fe and Ge in site exchange LixFe0.5Ge0.5SiO3.5S0.5 (x = 0, 1, 2).
图 8 (a) 初始LixFe0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2)的TDOS和PDOS; (b) 初始 LixFe0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2) 中Fe和Ge的PDOS; (c) 位置交换LixFe0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2)的TDOS和PDOS; (d) 位置交换LixFe0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2)中Fe和Ge的PDOS
Fig. 8. (a) TDOS and PDOS of initial LixFe0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2); (b) PDOS of Fe and Ge in initial LixFe0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2); (c) TDOS and PDOS of site exchange LixFe0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2); (d) PDOS of Fe and Ge in site exchange LixFe0.5Ge0.5SiO3.5F0.5 (x = 0, 1, 2) .
表 1 Li2M0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F)的弹性常数矩阵的特征值和形成能(ΔEf)
Table 1. The eigenvalues of the elastic constant matrix and formation energy (ΔEf) of Li2M0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F).
Mn-S Mn-F Fe-S Fe-F 特征值 12.00 23.56 16.17 13.52 19.08 28.85 20.12 22.87 29.87 37.69 20.76 36.42 37.22 53.05 39.82 53.41 62.41 73.17 53.52 68.55 207.87 209.12 222.90 225.26 ΔEf /eV –17.39 –17.88 –17.02 –17.30 
下载: 导出CSV
表 2 计算的Li2M0.5Ge0.5SiO3.5R0.5体积模量B、剪切模量G、模量比B/G、泊松比ν、杨氏模量E和德拜温度θD (M = Mn, Fe; R = S, F )
Table 2. Calculated bulk modulus B, shear modulus G, modulus ratio B/G, Poisson’s ratio ν, Young’s modulus E and Debye temperature θD of Li2M0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F).
B/GPa G/GPa B/G ν E/ GPa θD/K Li2Mn0.5Ge0.5SiO3.5S0.5 56.06 21.52 2.60 0.33 57.24 387 Li2Mn0.5Ge0.5SiO3.5F0.5 67.03 30.37 2.21 0.30 79.15 462 Li2Fe0.5Ge0.5SiO3.5S0.5 63.91 21.15 3.02 0.35 57.15 383 Li2Fe0.5Ge0.5SiO3.5F0.5 68.85 25.65 2.68 0.33 68.45 426
下载: 导出CSV
表 3 位置交换的LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1)的平均键长 (单位: Å)
Table 3. The average bond length (in Å) of LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1) with the site exchange case
M—O M—R Ge—O Ge—R Si1—O Si1—R Si2—O Si2—R Mn—S (x = 2) 2.220 2.473 2.216 — 1.638 2.156 1.667 — Mn—S (x = 1∶SE) 2.092 — 1.810 2.196 1.633 2.178 1.657 — Mn—S (x = 0∶SE) 1.826 — 1.794 2.200 1.644 2.131 1.639 — Mn—F (x = 2) 2.108 — 2.163 2.892 1.656 — 1.644 1.725 Mn—F (x = 1∶SE) 2.010 — 1.863 — 1.656 — 1.624 1.658 Mn—F (x = 0∶SE) 1.938 — 1.817 — 1.628 — 1.708 1.666 Fe—S (x = 2) 2.146 2.450 2.214 — 1.666 — 1.642 2.137 Fe—S (x = 1∶SE) 1.982 2.350 1.804 — 1.654 — 1.703 2.057 Fe—S (x = 0∶SE) 1.852 — 1.784 2.239 1.639 — 1.626 2.179 Fe—F (x = 2) 2.058 — 2.180 2.396 1.658 — 1.647 2.646 Fe—F (x = 1∶SE) 1.900 — 1.975 2.793 1.651 — 1.626 1.661 Fe—F (x = 0∶SE) 1.863 — 1.755 1.983 1.644 — 1.612 1.817
下载: 导出CSV
表 4 位置交换的LixM0.5Ge0.5SiO3.5R0.5中(M = Mn, Fe; R = S, F; x = 0, 1 )、Ge 和 Si 的键合价和 BVS
Table 4. Bond-valence sums (BVS) of M (M = Mn, Fe), Ge and Si in LixM0.5Ge0.5SiO3.5R0.5 (M = Mn, Fe; R = S, F; x = 0, 1) with site exchange case.
M Ge Si1 Si2 Mn—S (x = 2) 1.42 1.23 3.81 3.56 Mn—S (x = 1∶SE) 1.77 3.60 3.81 3.68 Mn—S (x = 0∶SE) 3.35 3.70 3.83 3.85 Mn—F (x = 2) 1.70 1.01 3.67 3.52 Mn—F (x = 1∶SE) 2.28 2.20 3.70 3.82 Mn—F (x = 0∶SE) 2.50 3.35 3.96 3.21 Fe—S (x = 2) 1.44 1.18 3.57 3.83 Fe—S (x = 1∶SE) 2.14 3.45 3.72 3.67 Fe—S (x = 0∶SE) 3.12 3.66 3.85 3.85 Fe—F (x = 2) 1.68 1.08 3.65 2.88 Fe—F (x = 1∶SE) 2.74 1.67 3.72 3.78 Fe—F (x = 0∶SE) 3.02 3.37 3.82 3.65
下载: 导出CSV
表 5 初始和位点交换情况下 M (M = Mn, Fe) 离子的磁矩 (μB) 和氧化态
Table 5. Magnetic moment (in μB) and oxidation state of M (M = Mn, Fe) ions in the case of initial and site exchange.
结构 磁矩M(初始)/
M(位置交换)氧化态M(初始)/
M(位置交换)Mn—S (x = 2) 4.64/4.64 +2(3d5)/+2(3d5) Mn—S (x = 1) 4.61/4.65 +2(3d5)/+2(3d5) Mn—S (x = 0) 3.78/3.41 +(3 + δ)(3d(4–δ))/
+(3 + φ)(3d(4–φ))Mn—F (x = 2) 4.65/4.65 +2(3d5)/+2(3d5) Mn—F (x = 1) 4.64/4.17 +2(3d5)/
+(2 + α)(3d(5–α))Mn—F (x = 0) 3.87/3.98 +3(3d4)/+3(3d4) Fe—S (x = 2) 3.73/3.73 +2(3d6)/+2(3d6) Fe—S (x = 1) 3.71/3.68 +2(3d6)/+2(3d6) Fe—S (x = 0) 4.02/4.14 +(3 + τ)(3d(5–τ))/
+(3 + ψ)(3d(5–ψ))Fe—F (x = 2) 3.73/3.73 +2(3d6)/+2(3d6) Fe—F (x = 1) 4.23/4.23 +3(3d5)/+3(3d5) Fe—F (x = 0) 4.25/4.26 +3(3d5)/+3(3d5)
下载: 导出CSV
-
[1] Dominko R, Bele M, Kokalj A, Gaberscek, M, Jamnik J 2007 J. Power Sources 174 457
Google Scholar
[2] Sasaki H, Nemoto A, Moriya M, Masahiko M, Mana H, Shingo K, Yuji A, Akira N, Shinichi H 2015 Ceram. Int. 41 S680
Google Scholar
[3] Li Y X, Gong Z L, Yang Y 2007 J. Power Sources 174 528
Google Scholar
[4] Dominko R 2008 J. Power Sources 184 462
Google Scholar
[5] Muraliganth T, Stroukoff K R, Manthiram A 2010 Chem. Mater. 22 5754
Google Scholar
[6] Liu S S, Song L J, Yu B J, Wang C Y, Li M W 2016 Electrochim. Acta 188 145
Google Scholar
[7] Nyten A, Abouimrane A, Armand M, Gustafsson T, Thomas J O 2005 Electrochem. Commun. 7 156
Google Scholar
[8] Arroyo-de Dompablo M E, Armand M, Tarascon J M, Amador U 2006 Electrochem. Commun. 8 1292
Google Scholar
[9] Wang C, Xu Y L, Zhang B F, Ma X N 2019 Solid State Ionics 338 39
Google Scholar
[10] Ma D W, Feng Y Y, Zhang B, Feng J, Pan J H 2021 Scr. Mater. 193 122
Google Scholar
[11] Wang K, Teng G F, Yang J L, Tan R, Duan Y D, Zheng J X, Pan F 2015 J. Mater. Chem. A 3 24437
Google Scholar
[12] Li T, Jiang X T, Gao K, Wang C Y, Li S D 2016 J. Chin. Chem. Soc. 63 800
Google Scholar
[13] Zhu L, Li L, Cheng T M, Xu D S 2015 J. Mater. Chem. A 10 5449
[14] Singh S, Raj A K, Sen R, Johari P, Mitra S 2017 ACS Appl. Mater. Interfaces 9 26885
Google Scholar
[15] Nytén A, Kamali S, Haggstrom L, Torbjorn G, John O T 2006 J. Mater. Chem. 16 2266
Google Scholar
[16] Yan X T, Hou Y H, Huang Y L, Zheng S H, Shi Z Q, Tao X M 2019 J. Electrochem. Soc. 166 A3874
Google Scholar
[17] Yan X T, Hou Y H, Zheng S H, Huang Y L, Shi Z Q, Tao X M 2020 Phys. Chem. Chem. Phys. 22 14712
Google Scholar
[18] Zheng S H, Hou Y H, Guo X L, Huang Y L, Li W, Tao X M 2021 Electrochim. Acta 367 137553
Google Scholar
[19] Kresse G, Furthmüller J 1996 Comput. Mater. Sci. 6 15
Google Scholar
[20] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[21] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[22] Blöchl P E 1994 Phys. Rev. B 50 17953
Google Scholar
[23] Monkhorst H J 1976 Phys. Rev. B 13 5188
Google Scholar
[24] Anisimov V I, Zaanen J, Andersen O K 1991 Phys. Rev. B 44 943
Google Scholar
[25] Feng Y M, Ji R, Ding Z P, Zhang D L, Liang C P, Chen L B, Ivey, Douglas G, Wei W F 2018 Inorg. Chem. 57 3223
Google Scholar
[26] Zeng Y, Chiu H C, Ouyang B, Song J, Zaghib K, Demopoulos G P 2019 J. Mater. Chem. A 7 25399
Google Scholar
[27] Lian D X, Zhao Y H, Hou H, Wang S, Wen Z Q, Zhang Q, Guo Q W 2019 Comput. Mater. Sci. 168 260
Google Scholar
[28] Mouhat F, Coudert F X 2014 Phys. Rev. B 90 224104
Google Scholar
[29] Li L, Zhu L, Xu L H, Cheng T M, Wang W, Li X, Sui Q T 2014 J. Mater. Chem. A 2 4251
Google Scholar
[30] Hill R 1952 Proc. Phys. Soc. 65 349
Google Scholar
[31] Watt J P 1979 J. Appl. Phys. 50 6290
Google Scholar
[32] Pugh S F 1954 Philos. Mag. 45 823
Google Scholar
[33] Frantsevich I N, Voronov F F, Bokuta S A 1983 Naukova Dumka Kiev 43 60
[34] 郑寿红, 李 伟, 姜茗浩, 闫小童, 侯育花, 陶小马 2021 功能材料 52 06084
Google Scholar
Zheng S H, Li W, Jiang M H, Yan X T, Hou Y H, Tao X M 2021 Funct. Mater. 52 06084
Google Scholar
[35] Dominko R, Arcon I, Kodre A, Hanzel D, Gaberšček M 2009 J. Power Sources 189 51
Google Scholar
[36] Zhong G H, Li Y L, Yan P, Liu, Z, Xie M H, Lin H Q 2010 J. Phys. Chem. C 114 3693
Google Scholar
[37] Marianetti C A, Kotliar G, Ceder G 2004 Phy. Rev. Lett. 92 196405
Google Scholar
[38] Brese B N E, O’Keeffe M 1991 Acta Crystallogr. B47 192
下载:
计量
- 文章访问数: 4103
- PDF下载量: 56
- 被引次数: 0
