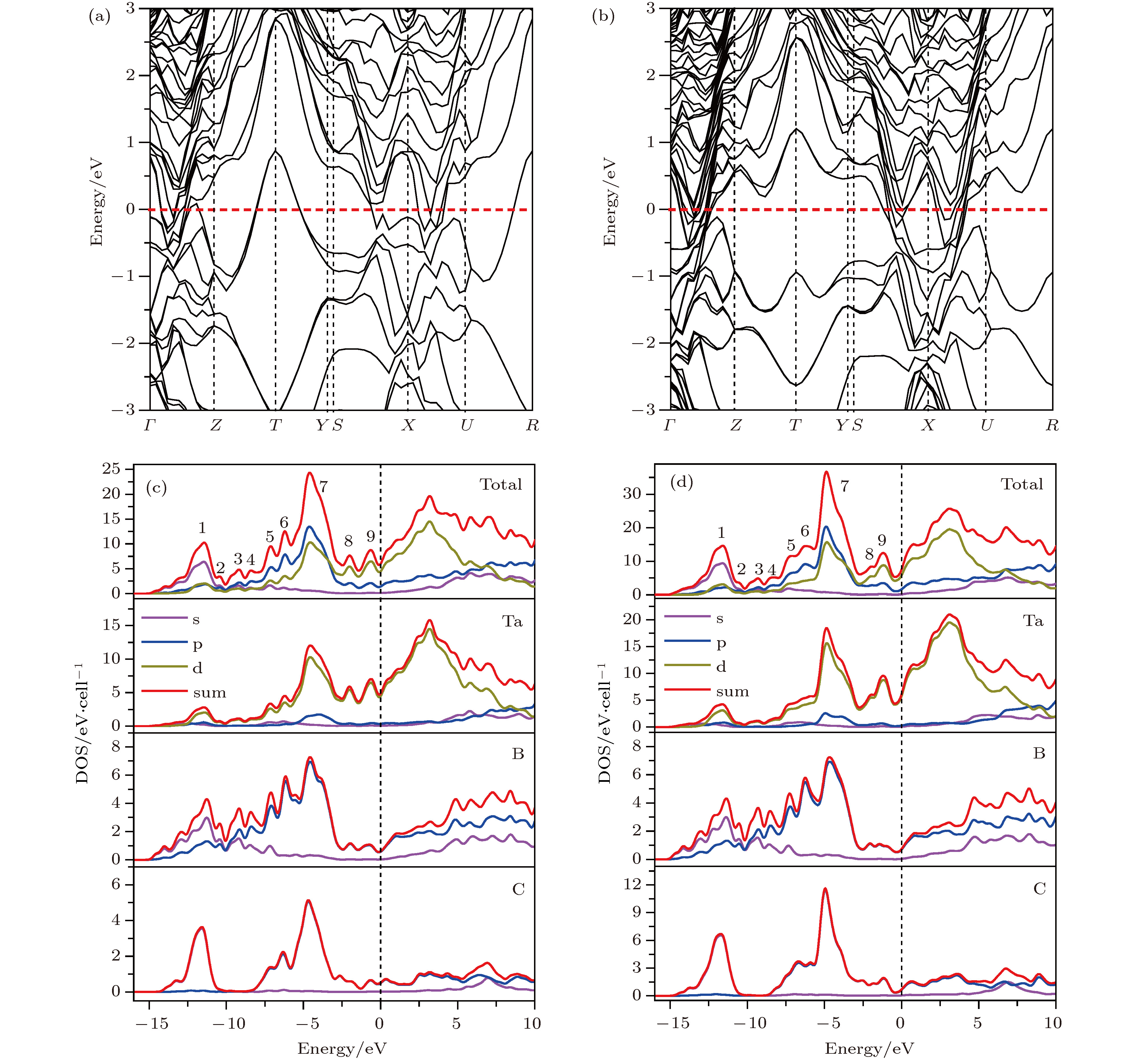
-
在过渡金属轻元素化合物中, 寻找新的硬质或者超硬材料是当前的一个研究热点. 目前寻找范围多集中在过渡金属硼化物、碳化物和氮化物等二元体系, 三元相的研究则相对较少. 本文以已知Nb3B3C和Nb4B3C2结构为模板, 采用元素替代法构建了29种TM3B3C (TM为过渡金属元素)结构和29种TM4B3C2结构, 采用基于密度泛函理论的第一性原理计算方法, 成功找到了热力学、动力学以及力学都稳定的Ta3B3C和Ta4B3C2两种新相. 结构搜索计算确认了这两相为全局能量最优结构. 能带结构和态密度的计算显示这两相均为导体, 导电性主要源于Ta原子的d电子. 这两种新相的硬度大约为26 GPa, 说明Ta3B3C和Ta4B3C2属于高硬度材料, 但不是超硬材料.To search new hard or superhard materials in transition-metal light-element compounds is a current research focus. Most of the past researches focused on binary phases such as transition metal borides, carbides and nitrides, while the researches on ternary phases were relatively rare. The single crystals Nb3B3C and Nb4B3C2 were synthesized recently by using Al-Cu alloys as auxiliary metals and their structures were determined by Hillebrechtand Gebhardt. In the present paper, 29 TM3B3C and 29 TM4B3C2 configurations are constructed by TM atoms (TM = Sc to Zn, Y to Cd, Hf to Hg) replacing Nb atoms in the known Nb3B3C and Nb4B3C2 configuration. By calculating the formation energy from first-principles density functional theories, only 13 TM3B3C and 11 TM4B3C2 phases are stable compared with the three elemental substances of TM, boron and carbon. However compared with the most competing phases, only Ta3B3C, Nb3B3C and Nb4B3C2 phases are stable thermodynamically. The metastable Ta4B3C2 phase at 0 K becomes stable when temperature is higher than 250 K. Thus two new phases of Ta3B3C and Ta4B3C2 are uncovered to be stable thermodynamically. Global structure searches conducted by the popular USPEX and CALYPSO softwares prove the Ta3B3C and Ta4B3C2 phases to be the most favorable energetically. By calculating the phonon dispersion curves of the Ta3B3C and Ta4B3C2 phase, no imaginary phonon frequencies are observed in the whole Brillouin zone, which demonstrates the dynamical stability of the Ta3B3C and Ta4B3C2 phase. The calculated elastic constant of the Ta3B3C and Ta4B3C2 phases satisfy the criteria of mechanical stability, showing that the Ta3B3C and Ta4B3C2 phase are stable mechanically. The calculations of band structure and density of state show that the Ta3B3C and Ta4B3C2 phases are both conducting, which mainly arises from the d electrons of Ta atoms. The calculated bulk modulus and shear modulus of the Ta3B3C and Ta4B3C2 phases show their brittle nature. The hardness of Ta3B3C and Ta4B3C2 phase are nearly the same and calculated to be about 26 GPa by Chen’s and Tian’s models, which illuminates that the two phases are hard but not superhard.
-
Keywords:
- hard materials /
- first-principles calculations /
- stability /
- hardness
[1] Tian Y J, Xu B, Zhao Z S 2012 Int. J. Refract. Met. Hard Mat. 33 93
Google Scholar
[2] 包括, 马帅领, 徐春红, 崔田 2017 66 036104
Google Scholar
Bao K, Ma S L, Xu C H, Cui T 2017 Acta Phys. Sin. 66 036104
Google Scholar
[3] Zhou X F, Sun J, Fan Y X, Chen J, Wang H T, Guo X J, He J L, Tian Y J 2007 Phys. Rev. B 76 100101
Google Scholar
[4] Dong H F, Oganov A R, Wang Q G, Wang S N, Wang Z H, Zhang J, Esfahani M M D, Zhou X F, Wu F G, Zhu Q 2016 Sci. Rep. 6 31288
Google Scholar
[5] Tian Y J, Xu B, Yu D L, Ma Y M, Wang Y B, Jiang Y B, Hu W T, Tang C C, Gao Y F, Luo K, Zhao Z S, Wang L M, Wen B, He J L, Liu Z Y 2013 Nature 493 385
Google Scholar
[6] Huang Q, Yu D L, Xu B, Hu W T, Ma Y M, Wang Y B, Zhao Z S, Wen B, He J L, Liu Z Y, Tian Y J 2014 Nature 510 250
Google Scholar
[7] 徐波, 田永君 2017 66 036201
Google Scholar
Xu B, Tian Y J 2017 Acta Phys. Sin. 66 036201
Google Scholar
[8] Kaner R B, Gilman J J, Tolbert S H 2005 Science 308 1268
Google Scholar
[9] Cumberland R W, Weinberger M B, Gilman J J, Clark S M, Tolbert S H, Kaner R B 2005 J. Am. Chem. Soc. 127 7264
Google Scholar
[10] Chung H Y, Weinberger M B, Levine J B, Kavner A, Yang J M, Tolbert S H, Kaner R B 2007 Science 316 436
Google Scholar
[11] Li Q, Zhou D, Zheng W T, Ma Y M, Chen C F 2013 Phys. Rev. Lett. 110 136403
Google Scholar
[12] Zhao C M, Duan Y F, Gao J, Liu W J, Dong H M, Dong H F, Zhang D K, Oganov A R 2018 Phys. Chem. Chem. Phys. 20 24665
Google Scholar
[13] Gregoryanz E, Sanloup C, Somayazulu M, Badro J, Fiquet G, Mao H K, Hemley R J 2004 Nat. Mater. 3 294
Google Scholar
[14] Young A F, Sanloup C, Gregoryanz E, Scandolo S, Hemley R J, Mao H K 2006 Phys. Rev. Lett. 96 155501
Google Scholar
[15] Ivanovskii A L 2012 Prog. Mater. Sci. 57 184
Google Scholar
[16] 陶强, 马帅领, 崔田, 朱品文 2017 66 036103
Google Scholar
Tao Q, Ma S L, Cui T, Zhu P W 2017 Acta Phys. Sin. 66 036103
Google Scholar
[17] Hillebrecht H, Gebhardt K 2001 Angewandte Chemie (Int. Ed. in English)
40 1445 Google Scholar
[18] Oganov A R, Glass C W 2006 J. Chem. Phys. 124 244704
Google Scholar
[19] Wang Y C, Lü J A, Zhu L, Ma Y M 2010 Phys. Rev. B 82 094116
Google Scholar
[20] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[21] Le Page Y, Saxe P 2002 Phys. Rev. B 65 104104
Google Scholar
[22] Togo A, Tanaka I 2015 Scr. Mater. 108 1
Google Scholar
[23] Jain A, Ong S P, Hautier G, Chen W, Richards W D, Dacek S, Cholia S, Gunter D, Skinner D, Ceder G, Persson K A 2013 APL Mater. 1 011002
Google Scholar
[24] Togo A, Chaput L, Tanaka I, Hug G 2010 Phys. Rev. B 81 174301
Google Scholar
[25] Mouhat F, Coudert F X 2014 Phys. Rev. B 90 224104
Google Scholar
[26] Wu Z J, Zhao E J, Xiang H P, Hao X F, Liu X J, Meng J 2007 Phys. Rev. B 76 054115
Google Scholar
[27] Pugh S F 1954 Philos. Mag. 45 823
Google Scholar
[28] Chen X Q, Niu H Y, Li D Z, Li Y Y 2011 Intermetallics 19 1275
Google Scholar
-
图 1 (a), (b) Nb3B3C和(c), (d) Nb4B3C2的晶体结构(棕球, Nb原子; 蓝球, B原子; 粉球, C原子; Nb6B三棱柱和Nb6C八面体分别用绿色和褐色表示)
Fig. 1. Crystal structures of the (a), (b) Nb3B3C and (c), (d) Nb4B3C2. The light brown, blue and pink spheres represent Nb, B and C atoms, respectively. The Nb6B trigonal prisms and Nb6C octahedrons are painted green and dark brown.
图 2 不同温度下Ta3B3C, Ta4B3C2相分别和其相应最稳定竞争组合相的自由能之差
Fig. 2. Energy differences of Ta3B3C and Ta4B3C2 phases with respect to their most competing phases as a function of temperature.
图 3 (a) Ta3B3C和(b) Ta4B3C2结构的声子色散曲线
Fig. 3. Phonon dispersion curves of (a) Ta3B3C and (b) Ta4B3C2 structures.
图 4 (a), (c) Ta3B3C和(b), (d) Ta4B3C2结构的能带结构和态密度图
Fig. 4. Band structures and density of states of (a), (c) Ta3B3C and (b), (d) Ta4B3C2 structures.
表 1 Nb3B3C, Nb4B3C2, Ta3B3C和Ta4B3C2晶体的结构参数
Table 1. Structural parameters of the Nb3B3C, Nb4B3C2, Ta3B3C and Ta4B3C2 configurations.
模型 晶系和空间群 晶格参数(Å, degree) 原子坐标 Nb3B3C Orthorhombic Cmcm a = 3.284, 3.265a, b = 28.877, 28.710a, c = 3.144, 3.129a, α = β = γ = 90 Nb1 (4c) (0, 0.2128, 0.25), Nb2 (4c) (0, 0.3620, 0.25), Nb3 (4c) (0, 0.4532, 0.25), B1 (4c) (0, 0.1120, 0.25), B2 (4c) (0, 0.0155, 0.25), B3 (4c) (0, 0.0790, 0.25), C (4c) (0, 0.2878, 0.25) Nb4B3C2 Orthorhombic Cmcm a = 3.257, 3.229a, b = 37.874, 37.544a, c = 3.153, 3.133a, α = β = γ = 90 Nb1 (4c) (0, 0.1621, 0.75), Nb2 (4c) (0, 0.2805, 0.75), Nb3 (4c) (0, 0.3946, 0.75), Nb4 (4c) (0, 0.4642, 0.25), B1 (4c) (0, 0.0854, 0.75), B2 (4c) (0, 0.0118, 0.25), B3 (4c) (0, 0.0602, 0.25), C1 (4c) (0, 0.2202, 0.75), C2 (4c) (0, 0.3383, 0.75) Ta3B3C Orthorhombic Cmcm a = 3.267, b = 28.688, c = 3.133, α = β = γ = 90 Ta1 (4c) (0, 0.2121, 0.25), Ta2 (4c) (0, 0.3619, 0.25), Ta3 (4c) (0, 0.4531, 0.25), B1 (4c) (0, 0.1130, 0.25), B2 (4c) (0, 0.0155, 0.25), B3 (4c) (0, 0.0791, 0.25), C (4c) (0, 0.2874, 0.25) Ta4B3C2 Orthorhombic Cmcm a = 3.243, b = 37.609, c = 3.141, α = β = γ = 90 Ta1 (4c) (0, 0.1615, 0.75), Nb2 (4c) (0, 0.2806, 0.75), Nb3 (4c) (0, 0.3945, 0.75), Nb4 (4c) (0, 0.4641, 0.25), B1 (4c) (0, 0.0861, 0.75), B2 (4c) (0, 0.0118, 0.25), B3 (4c) (0, 0.0602, 0.25), C1 (4c) (0, 0.2202, 0.75), C2 (4c) (0, 0.3380, 0.75) 注: a文献[17]中的实验值. 
下载: 导出CSV
表 2 不同成分TM3B3C和TM4B3C2的形成焓(eV/atom)
Table 2. Calculated formation enthalpies of different TM3B3C and TM4B3C2 phases (eV/atom).
TM TM3B3C TM4B3C2 $\Delta {H_{{\rm{elements}}}}$ $\Delta {H_{{\rm{comp}}}}$ 最稳定竞争组合 $\Delta {H_{{\rm{elements}}}}$ $\Delta {H_{{\rm{comp}}}}$ 最稳定竞争组合 Sc –0.637 0.071 6ScB2 + Sc4C3 + Sc2C = 4Sc3B3C –0.520 0.144 10ScB2 + 4Sc4C3 + Sc2BC2 = 7Sc4B3C2 Ti –0.896 0.019 9TiB2 + TiC + Ti8C5 = 6Ti3B3C –0.863 0.018 9TiB2 + 7TiC + Ti8C5 = 6Ti4B3C2 V –0.687 0.101 3VB + C = V3B3C –0.628 0.092 18VB + 7C + V6C5 = 6V4B3C2 Cr –0.294 0.159 3CrB + C = Cr3B3C –0.194 0.178 9CrB + 4C + Cr3C2 = 3Cr4B3C2 Mn –0.100 0.195 3MnB + C = Mn3B3C 0.024 Fe 0.002 0.139 Co 0.094 0.255 Ni 0.296 0.456 Cu 0.738 0.959 Zn 0.713 0.929 Y –0.385 0.089 9YB2 + 5Y2C + Y2B3C2 = 7Y3B3C –0.283 0.160 6YB2 + 8Y2C + 3Y2B3C2 = 7Y4B3C2 Zr –0.851 0.019 3ZrB2 + 2ZrC + Zr = 2Zr3B3C –0.838 0.020 3ZrB2 + 4ZrC + Zr = 2Zr4B3C2 Nb –0.698 –0.023 3NbB + C = Nb3B3C –0.661 –0.002 C + 6Nb3B3C + Nb6C5 = 6Nb4B3C2 Mo –0.257 0.175 3MoB + C = Mo3B3C –0.155 0.202 3MoB + C + MoC = Mo4B3C2 Tc –0.005 0.326 12TcB2 + 11C + 3Tc7B3 = 11Tc3B3C 0.138 Ru 0.211 –0.369 Rh 0.230 –0.406 Pd 0.552 0.744 Ag 1.027 1.295 Cd 0.846 1.112 Hf –0.920 0.016 3HfB2 + 2HfC + Hf = 2Hf3B3C –0.922 0.018 3HfB2 + 4HfC + Hf = 2Hf4B3C2 Ta –0.704 0.003 3Ta3B4 + C + 3TaC = 4Ta3B3C –0.691 –0.010 3Ta3B4 + C + 7TaC = 4Ta4B3C2 W –0.094 0.227 3WB + C = W3B3C –0.007 0.273 3WB + C + WC = W4B3C2 Re 0.281 0.425 Os 0.590 0.755 Ir 0.604 0.758 Pt 0.708 0.855 Au 1.096 1.310 Hg 1.186 1.333
下载: 导出CSV
表 3 Ta3B3C, Ta4B3C2结构的弹性常数、体弹模量、剪切模量和维氏硬度 (GPa)
Table 3. Calculated elastic constants Cij, bulk modulus B, shear modulus G, Vickers hardness HV of Ta3B3C and Ta4B3C2 configurations (GPa).
结构 弹性常数 力学性能a 硬度 C11 C22 C33 C44 C55 C66 C12 C13 C23 B G B/G HChen HTian Ta3B3C 569.6 514.4 563.5 194.1 180.0 261.8 187.1 147.3 173.9 295.9 200.8 1.47 25.3 25.3 Ta4B3C2 581.1 535.3 602.1 197.3 185.1 275.8 200.3 146.0 170.2 305.7 209.0 1.46 26.2 26.2 Nb3B3C 544.3 479.8 522.8 181.5 171.9 245.3 170.9 132.9 162.2 275.3 189.7 1.45 24.8 24.7 Nb4B3C2 551.5 499.2 548.5 184.0 175.1 257.1 183.2 132.7 157.8 282.9 195.8 1.44 25.5 25.4 TaB2 302 200 1.51 24.4 24.5 NbB2 287 195 1.47 24.8 24.8 TaC 324 215 1.51 25.6 25.9 NbC 239 161 1.48 21.6 21.4 SiC 213 187 1.14 33.6 32.2 Al2O3 232 147 1.58 18.7 18.7 TiN 259 180 1.44 24.3 24.0 注: a二元相力学性能数据来自Materials Project网站.
下载: 导出CSV
-
[1] Tian Y J, Xu B, Zhao Z S 2012 Int. J. Refract. Met. Hard Mat. 33 93
Google Scholar
[2] 包括, 马帅领, 徐春红, 崔田 2017 66 036104
Google Scholar
Bao K, Ma S L, Xu C H, Cui T 2017 Acta Phys. Sin. 66 036104
Google Scholar
[3] Zhou X F, Sun J, Fan Y X, Chen J, Wang H T, Guo X J, He J L, Tian Y J 2007 Phys. Rev. B 76 100101
Google Scholar
[4] Dong H F, Oganov A R, Wang Q G, Wang S N, Wang Z H, Zhang J, Esfahani M M D, Zhou X F, Wu F G, Zhu Q 2016 Sci. Rep. 6 31288
Google Scholar
[5] Tian Y J, Xu B, Yu D L, Ma Y M, Wang Y B, Jiang Y B, Hu W T, Tang C C, Gao Y F, Luo K, Zhao Z S, Wang L M, Wen B, He J L, Liu Z Y 2013 Nature 493 385
Google Scholar
[6] Huang Q, Yu D L, Xu B, Hu W T, Ma Y M, Wang Y B, Zhao Z S, Wen B, He J L, Liu Z Y, Tian Y J 2014 Nature 510 250
Google Scholar
[7] 徐波, 田永君 2017 66 036201
Google Scholar
Xu B, Tian Y J 2017 Acta Phys. Sin. 66 036201
Google Scholar
[8] Kaner R B, Gilman J J, Tolbert S H 2005 Science 308 1268
Google Scholar
[9] Cumberland R W, Weinberger M B, Gilman J J, Clark S M, Tolbert S H, Kaner R B 2005 J. Am. Chem. Soc. 127 7264
Google Scholar
[10] Chung H Y, Weinberger M B, Levine J B, Kavner A, Yang J M, Tolbert S H, Kaner R B 2007 Science 316 436
Google Scholar
[11] Li Q, Zhou D, Zheng W T, Ma Y M, Chen C F 2013 Phys. Rev. Lett. 110 136403
Google Scholar
[12] Zhao C M, Duan Y F, Gao J, Liu W J, Dong H M, Dong H F, Zhang D K, Oganov A R 2018 Phys. Chem. Chem. Phys. 20 24665
Google Scholar
[13] Gregoryanz E, Sanloup C, Somayazulu M, Badro J, Fiquet G, Mao H K, Hemley R J 2004 Nat. Mater. 3 294
Google Scholar
[14] Young A F, Sanloup C, Gregoryanz E, Scandolo S, Hemley R J, Mao H K 2006 Phys. Rev. Lett. 96 155501
Google Scholar
[15] Ivanovskii A L 2012 Prog. Mater. Sci. 57 184
Google Scholar
[16] 陶强, 马帅领, 崔田, 朱品文 2017 66 036103
Google Scholar
Tao Q, Ma S L, Cui T, Zhu P W 2017 Acta Phys. Sin. 66 036103
Google Scholar
[17] Hillebrecht H, Gebhardt K 2001 Angewandte Chemie (Int. Ed. in English)
40 1445 Google Scholar
[18] Oganov A R, Glass C W 2006 J. Chem. Phys. 124 244704
Google Scholar
[19] Wang Y C, Lü J A, Zhu L, Ma Y M 2010 Phys. Rev. B 82 094116
Google Scholar
[20] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[21] Le Page Y, Saxe P 2002 Phys. Rev. B 65 104104
Google Scholar
[22] Togo A, Tanaka I 2015 Scr. Mater. 108 1
Google Scholar
[23] Jain A, Ong S P, Hautier G, Chen W, Richards W D, Dacek S, Cholia S, Gunter D, Skinner D, Ceder G, Persson K A 2013 APL Mater. 1 011002
Google Scholar
[24] Togo A, Chaput L, Tanaka I, Hug G 2010 Phys. Rev. B 81 174301
Google Scholar
[25] Mouhat F, Coudert F X 2014 Phys. Rev. B 90 224104
Google Scholar
[26] Wu Z J, Zhao E J, Xiang H P, Hao X F, Liu X J, Meng J 2007 Phys. Rev. B 76 054115
Google Scholar
[27] Pugh S F 1954 Philos. Mag. 45 823
Google Scholar
[28] Chen X Q, Niu H Y, Li D Z, Li Y Y 2011 Intermetallics 19 1275
Google Scholar
下载:
计量
- 文章访问数: 10036
- PDF下载量: 113
- 被引次数: 0
