-
首先建立(2+1)维(二维空间和一维时间)Zakharov方程的自相似变换, 并将该系统转换为(1+1)维非线性薛定谔(nonlinear Schrödinger, NLS)方程; 然后基于该相似变换和已知的(1+1)维NLS方程有理形式解, 通过选择合适参数得到了(2+1)维Zakharov方程在x-y平面上丰富的线怪波簇激发, 发现产生线怪波簇最大辐值时的传播距离
$ z $ 值完全不同, 而且形状和幅度可以得到有效调控; 最后借助图示展现了二维怪波的传播特征. 此外, 发现在x-y平面上, 当参数$ \gamma = 1 $ 时, 呈现线怪波; 而当参数$ \gamma \ne 1 $ 时, 线怪波转变为离散的局域怪波. 随参数$ \gamma $ 的增大, 可以在x-y平面限定区域获得时空局域的怪波, 这与Peregrine在(1+1)维NLS方程中发现的“Kuznetsov-Ma孤子”(Kuznetsov-Ma soliton, KMS)或“Akhmediev呼吸子”(Akhmediev breather, AB)极限情形的“Peregrine 孤子”(Peregrine soliton, PS)类似. 本文提出的(2+1)维Zakharov方程怪波方法可以作为获得高维怪波激发的有效途径, 并推广应用于其他(2+1)维非线性系统.-
关键词:
- 线怪波 /
- 自相似变换 /
- Zakharov方程 /
- (2+1)维
The search for the excitation of two-dimensional rogue wave in a (2+1)-dimensional nonlinear evolution model is a research hotspot. In this paper, the self-similar transformation of the (2+1)-dimensional Zakharov equation is established, and this equation is transformed into the (1+1)-dimensional nonlinear Schrödinger equation. Based on the similarity transformation and the rational formal solution of the (1+1)-dimensional nonlinear Schrödinger equation, the rogue wave excitation of the (2+1)-dimensional Zakharov equation is obtained by selecting appropriate parameters. We can see that the shape and amplitude of the rogue waves can be effectively controlled. Finally, the propagation characteristics of line rogue waves are diagrammed visually. We also find that the line-type characteristics of two-dimensional rogue wave are present in the x-y plane when the parameter$ \gamma = 1 $ . The line rogue wave is converted into discrete localized rogue wave in the x-y plane when the parameter$ \gamma \ne 1 $ . The spatial localized rogue waves with short-life can be obtained in the required x-y plane region. This is similar to the Peregrine soliton (PS) first discovered by Peregrine in the (1+1)-dimensional NLS equation, which is the limit case of the “Kuznetsov-Ma soliton” (KMS) or “Akhmediev breather” (AB). The proposed approach to constructing the line rogue waves of the (2+1) dimensional Zakharov equation can serve as a potential physical mechanism to excite two-dimensional rogue waves, and can be extended to other (2+1)-dimensional nonlinear systems.-
Keywords:
- line rogue wave /
- self-similar transformation /
- Zakharov equation /
- (2+1)-dimensional
[1] Divinsky B V, Levin B V, Lopatukhin L I, Pelinovsky E N, Slyunyaev A V 2004 Dokl. Earth Sci. 395 438
[2] Onorato M, Osborne A R, Serio M, Bertone S 2001 Phys. Rev. Lett. 86 5831
Google Scholar
[3] Ginzburg N S, Rozental R M, Sergeev A S, Fedotov A E, Zotova I V, Tarakanov V P 2017 Phys. Rev. Lett. 119 034801
Google Scholar
[4] Akhmediev N, Dudley J M, Solli D R, Turitsyn S K 2013 J. Opt. 15 060201
Google Scholar
[5] Bludov Y V, Konotop V V, Akhmediev N 2009 Phys. Rev. A 80 033610
Google Scholar
[6] Moslem W M 2011 Phys. Plasm. 18 032301
Google Scholar
[7] Stenfl L, Marklund M 2010 J. Plasm. Phys. 76 293
Google Scholar
[8] Onorato M, Residori S, Bortolozzo U, Montina A, Arecchi F 2013 Phys. Rep. 528 47
Google Scholar
[9] Müller P, Garrett C, Osborne A 2005 Oceanography 18 66
Google Scholar
[10] Hohmann R, Kuhl U, Stockmann H J, Kaplan L, Heller E J 2010 Phys. Rev. Lett. 104 093901
Google Scholar
[11] Ganshin A N, Efimov V B, Kolmakov G V, Mezhov Deglin P V, McClintock E 2008 Phys. Rev. Lett. 101 065303
Google Scholar
[12] Yan Z Y 2010 Commun. Theor. Phys. 54 947
Google Scholar
[13] Shats M, Punzmann H, Xia H 2010 Phys. Rev. Lett. 104 104503
Google Scholar
[14] Xia H, Maimbourg T, Punzmann H, Shats M 2012 Phys. Rev. Lett. 109 114502
Google Scholar
[15] Pu J C, Li J, Chen Y 2021 Chin. Phys. B 30 060202
Google Scholar
[16] Zhou H J, Chen Y 2021 Nonlinear. Dynam. 106 3437
Google Scholar
[17] Solli R, Ropers C, Koonath P, Jalali B 2007 Nature 450 1054
Google Scholar
[18] Chabchoub A, Hoffmann N P, Akhmediev N 2011 Phys. Rev. Lett. 106 204502
Google Scholar
[19] Peregrine D H 1983 J. Aust. Math. Soc. Ser. B:Appl. Math. 25 16
Google Scholar
[20] Akhmediev N, Ankiewicz A, Soto Crespo J M 2009 Phys. Rev. E 80 026601
Google Scholar
[21] Kedziora D J, Ankiewicz A, Akhmediev N 2012 Phys. Rev. E 86 056602
Google Scholar
[22] 张解放, 金美贞, 胡文成 2020 69 244205
Google Scholar
Zhang J F, Jin M Z, Hu W C 2020 Acta Phys. Sin. 69 244205
Google Scholar
[23] 张解放, 金美贞 2020 69 214203
Google Scholar
Zhang J F, Jin M Z 2020 Acta Phys. Sin. 69 214203
Google Scholar
[24] Zakharov V E (edited by Bullough R, Caudrey P) 1980 The Inverse Scattering Method (Vol. 17) (Berlin: Springer) pp243–285 DOI: 10.1007/978-3-642-81448-8_7
[25] Radha R, Lakshmanan M 1994 Inverse Problems 10 L29
Google Scholar
[26] Strachan I A B 1992 Inverse Problems 8 L21
Google Scholar
[27] Radha R, Lakshmanan M 1997 J. Phys. A:Math. Gen. 30 3229
Google Scholar
[28] 沈守枫, 张隽 2008 应用数学和力学 29 1254
Google Scholar
Shen S F, Zhang J 2008 Appl. Math. Mech. 29 1254
Google Scholar
[29] Wang J, Chen L W, Liu C F 2014 Appl. Math. Comput. 249 76
[30] 程丽, 张翼 2016 长江大学学报(自科版) 13 35
Chen L, Zhang Y 2016 J. Yangtze Univ. (Nat. Sci. Ed.) 13 35 (in Chinese)
[31] Wang X B, Tian S F, Zhang T T 2018 Proceedings of Roceedings of the American Society 146 3353
Google Scholar
[32] Chen M D, Li B 2017 Modern Phys. Lett. B 31 1750298
[33] Fokas A S 1994 Inverse Problems 10 19
Google Scholar
[34] Chen J C, Chen Y 2014 J. Nonlinear Math. Phys. 21 454
[35] Radha R, Lakshmanan M 1997 Chaos, Solitons and Fractals 8 17
Google Scholar
[36] Villarroel J, Prada J, Estévez P G 2009 Stud. Appl. Math. 122 395
Google Scholar
[37] Estévez P G 1999 J. Math. Phys. 40 1406
Google Scholar
[38] Rao J G, Wang L H, Zhang Y, He J S 2015 Commun. Theor. Phys. 64 605
Google Scholar
[39] Chen T T, Hu P Y, He J S 2019 Commun. Theor. Phys. 71 496
Google Scholar
[40] Rao J G, Mihalacheb D, Cheng Y, He J S 2019 Phys. Lett. A 383 1138
Google Scholar
-
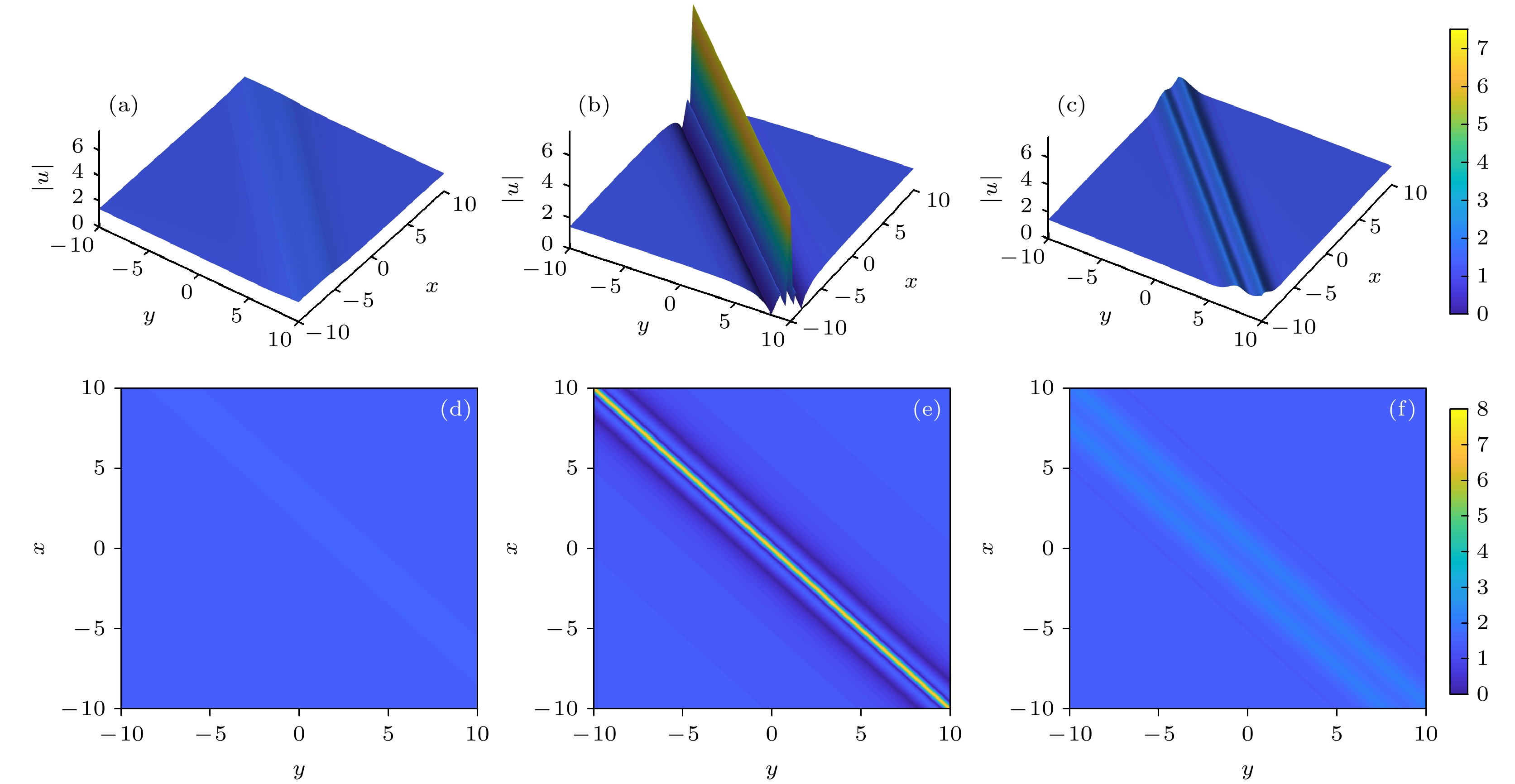
图 1 传播距离
$ z = - 3, 0, 1 $ 时一阶线怪波$ \left| u \right| $ (a), (b), (c) 三维图; (d), (e), (f) 对应x-y面上的投影图; (a), (d) A = 1.45; (b), (e) A = 4.24; (c), (f) A = 1.71Fig. 1. One-order line rogue wave
$ \left| u \right| $ respectively at the propagation distance$ z = - 3, 0, 1 $ : (a), (b), (c) Three-dimensional plots; (d), (e), (f) the corresponding contour plots in the x-y plane; (a), (d) A = 1.45; (b), (e) A = 4.24; (c), (f) A = 1.71.
图 2 传播距离
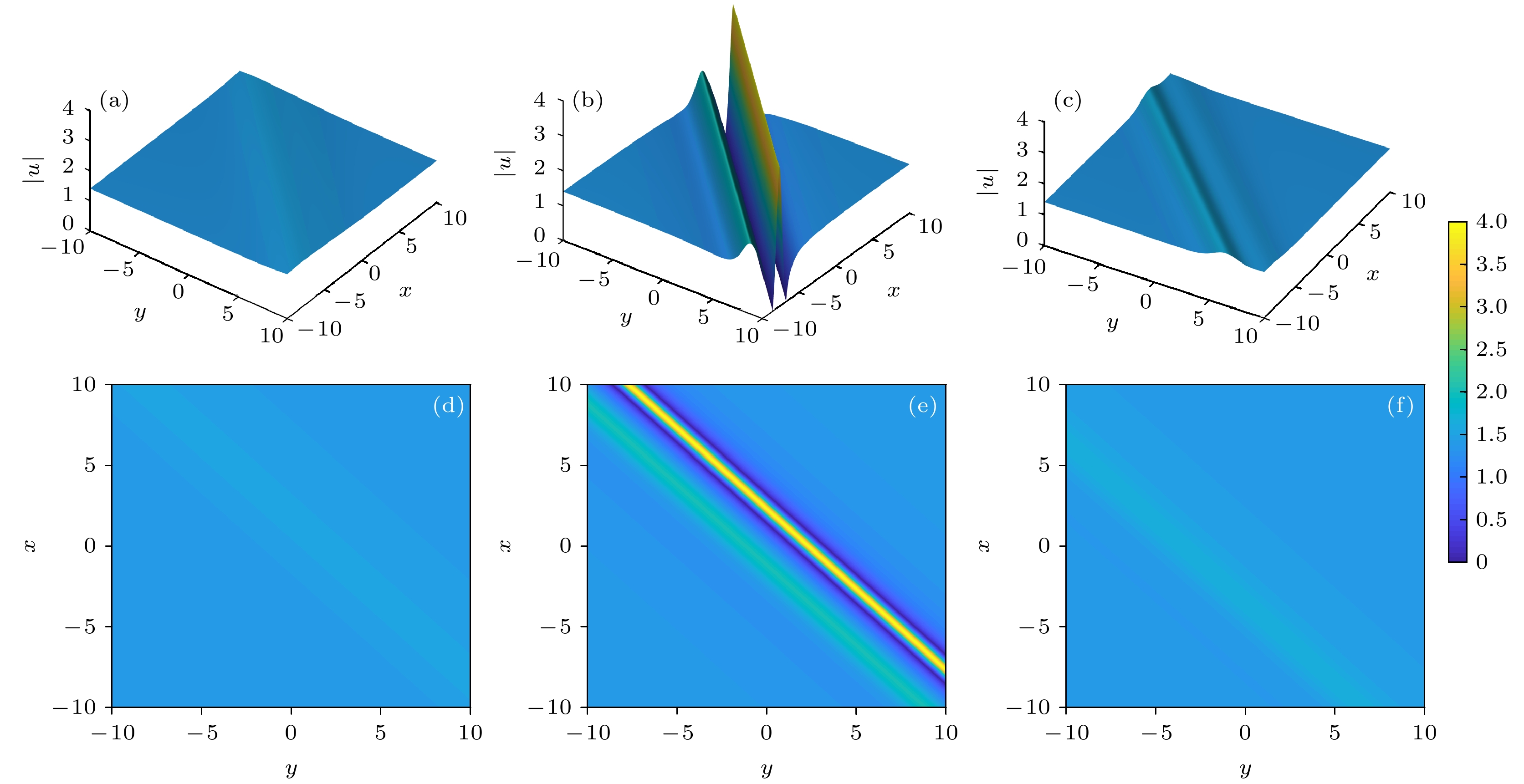
$ z = - 3, 0, 1 $ 时二阶线怪波$ \left| u \right| $ (a), (b), (c)三维图; (d), (e), (f) 对应x-y面上的投影图; (a), (d) A = 1.58; (b), (e) A = 7.32; (c), (f) A=1.93Fig. 2. Two-order line rogue wave
$ \left| u \right| $ respectively at the propagation distance$ z = - 3, 0, 1 $ : (a), (b), (c) Three-dimensional plots; (d), (e), (f) the corresponding contour plots in the x-y plane; (a), (d) A = 1.58; (b), (e) A = 7.32; (c), (f) A = 1.93.
图 3 传播距离
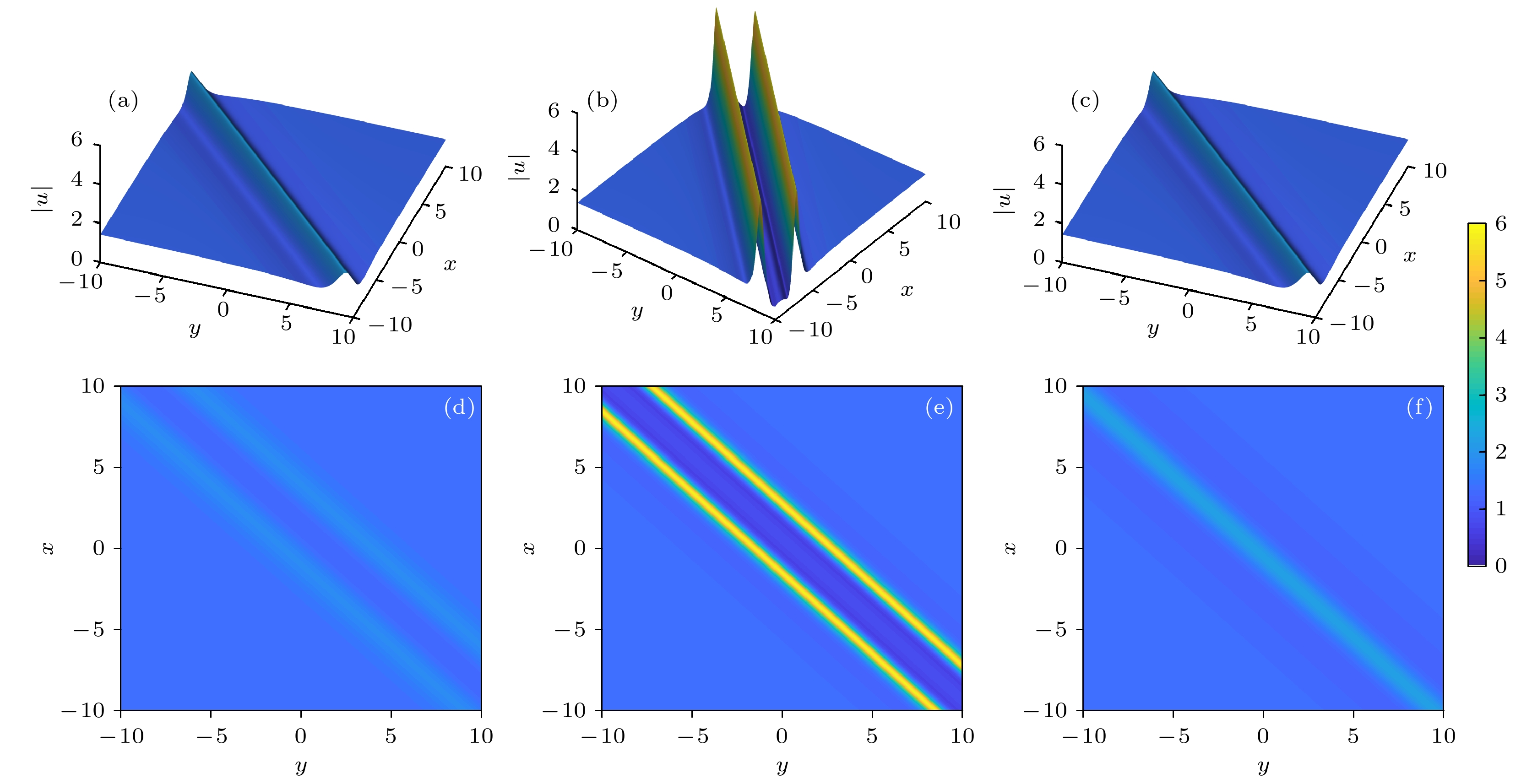
$ z = - 3, 0, {\text{ 2}} $ 时二阶线怪波$ \left| u \right| $ (a), (b), (c) 三维图; (d), (e), (f) 对应x-y面上的投影图; (a), (d) A = 1.52; (b), (e) A = 3.99; (c), (f) A = 1.69; 参数$ {w_0} = \gamma = {\beta _0} = 1, {\varsigma _0} = {\zeta _0} = {\varphi _0} = \delta = 0, \mu = 100 $ Fig. 3. Two-order line rogue wave
$ \left| u \right| $ respectively at the propagation distance$ z = - 3, 0, {\text{ 2}} $ : (a), (b), (c) Three-dimensional plots; (d), (e), (f) the corresponding contour plots in the x-y plane; (a), (d) A = 1.52; (b), (e) A = 3.99; (c), (f) A = 1.69; the parameters are$ {w_0} = \gamma = {\beta _0} = 1, {\varsigma _0} = {\zeta _0} = {\varphi _0} = \delta = {\text{0, }} $ $ \mu = 100 $ .
图 4 传播距离
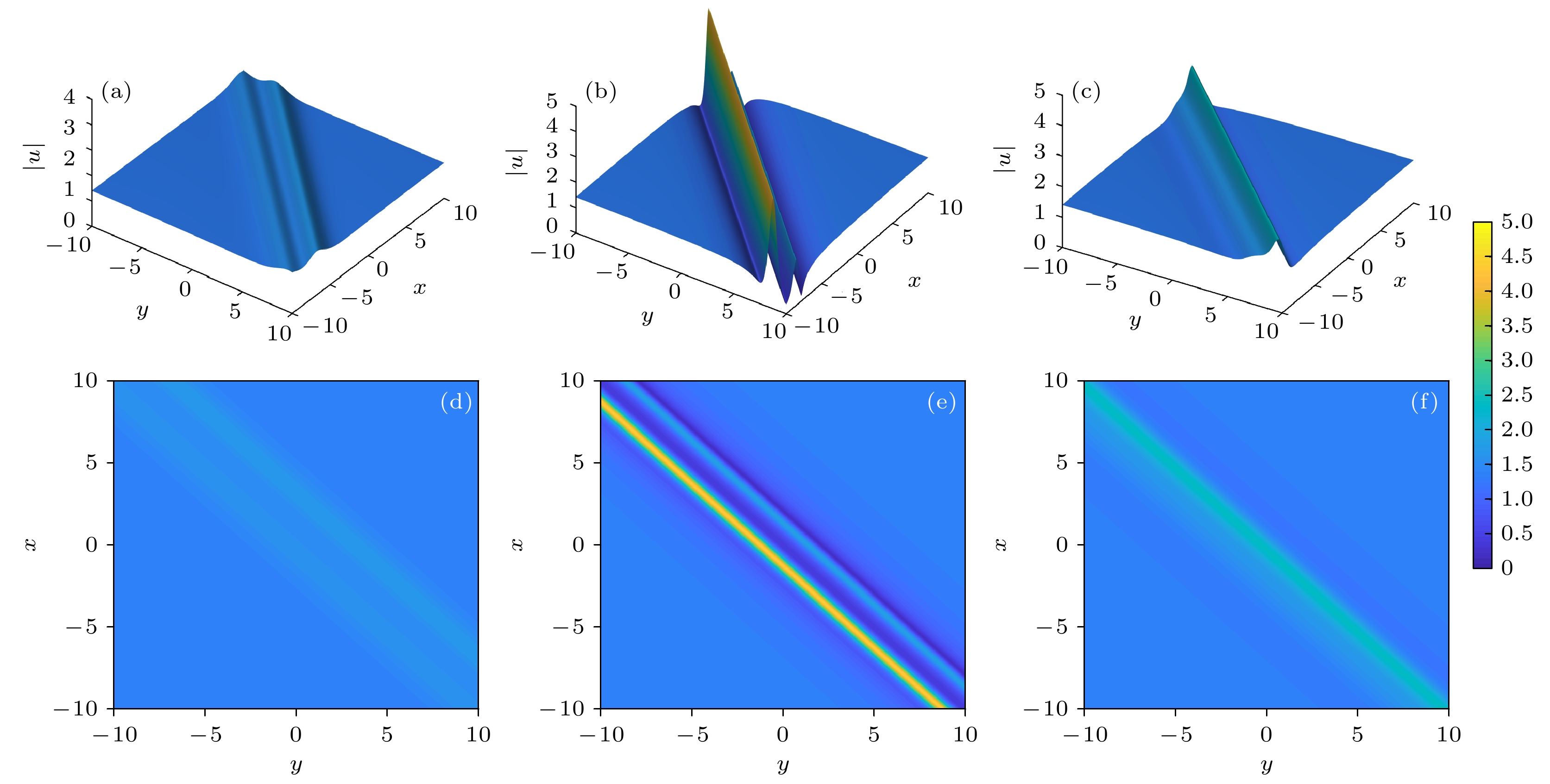
$ z = - {\text{1}}{\text{.5, }} - 0.{\text{635}}, {\text{ 0}}{\text{.5}} $ 时二阶线怪波簇$ \left| u \right| $ (a), (b), (c) 三维图; (d), (e), (f) 对应x-y面上的投影图; (a), (d) A = 1.80; (b), (e) A = 5.81; (c), (f) A = 2.23; 参数$ {w_0} = \gamma = {\beta _0} = 1, {\varsigma _0} = {\zeta _0} = {\varphi _0} = \mu = 0, \delta = 100 $ Fig. 4. Two-order line rogue wave
$ \left| u \right| $ respectively at the propagation distance$ z = - {\text{1}}{\text{.5, }} - 0.{\text{635}}, {\text{ 0}}{\text{.5}} $ : (a), (b), (c) Three-dimensional plots; (d), (e), (f) the corresponding contour plots in the x-y plane; (a), (d) A = 1.80; (b), (e) A = 5.81; (c), (f) A=2.23; the parameters are$ {w_0} = \gamma = {\beta _0} = 1, {\varsigma _0} = {\zeta _0} = {\varphi _0} = \mu = 0, \delta = 100 $ .
图 5 传播距离
$ z = 0{, }0.{\text{5, 1}}{\text{.0}} $ 时二阶线怪波簇$ \left| u \right| $ (a), (b), (c)三维图; (d), (e), (f) 对应x-y面上的投影图; (a), (d) A = 2.76; (b), (e) A = 4.36; (c), (f) A = 2.78; 参数$ {w_0} = \gamma = {\beta _0} = 1, {\varsigma _0} = {\zeta _0} = {\varphi _0} = 0, \mu = 40, \delta = 20 $ Fig. 5. Two-order line rogue wave
$ \left| u \right| $ respectively at the propagation distance$ z = 0{, }0.{\text{5, 1}}{\text{.0}} $ : (a), (b), (c) Three-dimensional plots; (d), (e), (f) the corresponding contour plots in the x-y plane; (a), (d) A = 2.76; (b), (e) A = 4.36; (c), (f) A = 2.78; the parameters are$ {w_0} = \gamma = {\beta _0} = 1, {\varsigma _0} = {\zeta _0} = {\varphi _0} = 0, \mu = 40, \delta = 20 $ .
图 6 (a), (b), (c)传播距离
$ z = - {\text{1, }}0.{\text{29}}, 1{\text{.5}} $ 时二线怪波簇$ \left| u \right| $ 在x-y平面上的三维图; (d), (e), (f)对应的投影图; (a), (d) A = 2.76; (b), (e) A = 4.53; (c), (f) A = 1.72; 参数$ {w_0} = \gamma = {\beta _0} = 1, {\varsigma _0} = {\zeta _0} = {\varphi _0} = 0, \mu = 20, \delta = - 20 $ Fig. 6. Two-order line rogue wave
$ \left| u \right| $ respectively at the propagation distance$ z = - {\text{1, }}0.{\text{29}}, 1{\text{.5}} $ : (a), (b), (c) Three-dimensional plots; (d), (e), (f) the corresponding contour plots in the x-y plane; (a), (d) A=2.76; (b), (e) A = 4.53; (c), (f) A = 1.72; the parameters are$ {w}_{0}=\gamma ={\beta }_{0}=1, {\varsigma }_{0}={\zeta }_{0}={\phi }_{0}=\text{0, } $ $ \mu = 20, \delta = - 20 $ .
图 7 传播距离
$ z = - {\text{1}}{\text{.5, }} - 0.{\text{295, 1}} $ 时二阶线怪波簇$ \left| u \right| $ (a), (b), (c) 三维图; (d), (e), (f)对应x-y面上的投影图; (a), (d) A = 1.72; (b), (e) A = 4.39; (c), (f) A = 2.39; 参数$ {w_0} = \gamma = {\beta _0} = 1, {\varsigma _0} = {\zeta _0} = {\varphi _0} = {\text{0, }} $ $ \mu = - 20, \delta = 20 $ Fig. 7. Two-order line rogue wave
$ \left| u \right| $ respectively at the propagation distance$ z = - {\text{1}}{\text{.5, }} - 0.{\text{295, 1}} $ : (a), (b), (c) Three-dimensional plots; (d), (e), (f) the corresponding contour plots in the x-y plane; (a), (d) A = 1.72; (b), (e) A = 4.39; (c), (f) A = 2.39; the parameters are$ {w}_{0}=\gamma ={\beta }_{0}=1, \text{ }{\varsigma }_{0}={\zeta }_{0}={\phi }_{0}=\text{0, } $ $ \mu = - 20, \delta = 20 $ .
图 8 在
$ z = 0 $ 时, 一阶线怪波$ \left| u \right| $ 在x-y平面上不同参数$ \gamma $ 下的形态 (a)$ \gamma = 1 $ ; (b)$ \gamma = {\text{3}} $ ; (c)$ \gamma = {\text{6}} $ ; (d)$ \gamma = {\text{10}} $ ; (e)$ \gamma = {\text{15}} $ ; (f)$ \gamma = {\text{2}}0 $ Fig. 8. One-order rogue wave
$ \left| u \right| $ in the x-y plane under the different$ \gamma $ at the propagation distance$ z = 0 $ : (a)$ \gamma = 1 $ ; (b)$ \gamma = {\text{3}} $ ; (c)$ \gamma = {\text{6}} $ ; (d)$ \gamma = {\text{10}} $ ; (e)$ \gamma = {\text{15}} $ ; (f)$ \gamma = {\text{2}}0 $ . -
[1] Divinsky B V, Levin B V, Lopatukhin L I, Pelinovsky E N, Slyunyaev A V 2004 Dokl. Earth Sci. 395 438
[2] Onorato M, Osborne A R, Serio M, Bertone S 2001 Phys. Rev. Lett. 86 5831
Google Scholar
[3] Ginzburg N S, Rozental R M, Sergeev A S, Fedotov A E, Zotova I V, Tarakanov V P 2017 Phys. Rev. Lett. 119 034801
Google Scholar
[4] Akhmediev N, Dudley J M, Solli D R, Turitsyn S K 2013 J. Opt. 15 060201
Google Scholar
[5] Bludov Y V, Konotop V V, Akhmediev N 2009 Phys. Rev. A 80 033610
Google Scholar
[6] Moslem W M 2011 Phys. Plasm. 18 032301
Google Scholar
[7] Stenfl L, Marklund M 2010 J. Plasm. Phys. 76 293
Google Scholar
[8] Onorato M, Residori S, Bortolozzo U, Montina A, Arecchi F 2013 Phys. Rep. 528 47
Google Scholar
[9] Müller P, Garrett C, Osborne A 2005 Oceanography 18 66
Google Scholar
[10] Hohmann R, Kuhl U, Stockmann H J, Kaplan L, Heller E J 2010 Phys. Rev. Lett. 104 093901
Google Scholar
[11] Ganshin A N, Efimov V B, Kolmakov G V, Mezhov Deglin P V, McClintock E 2008 Phys. Rev. Lett. 101 065303
Google Scholar
[12] Yan Z Y 2010 Commun. Theor. Phys. 54 947
Google Scholar
[13] Shats M, Punzmann H, Xia H 2010 Phys. Rev. Lett. 104 104503
Google Scholar
[14] Xia H, Maimbourg T, Punzmann H, Shats M 2012 Phys. Rev. Lett. 109 114502
Google Scholar
[15] Pu J C, Li J, Chen Y 2021 Chin. Phys. B 30 060202
Google Scholar
[16] Zhou H J, Chen Y 2021 Nonlinear. Dynam. 106 3437
Google Scholar
[17] Solli R, Ropers C, Koonath P, Jalali B 2007 Nature 450 1054
Google Scholar
[18] Chabchoub A, Hoffmann N P, Akhmediev N 2011 Phys. Rev. Lett. 106 204502
Google Scholar
[19] Peregrine D H 1983 J. Aust. Math. Soc. Ser. B:Appl. Math. 25 16
Google Scholar
[20] Akhmediev N, Ankiewicz A, Soto Crespo J M 2009 Phys. Rev. E 80 026601
Google Scholar
[21] Kedziora D J, Ankiewicz A, Akhmediev N 2012 Phys. Rev. E 86 056602
Google Scholar
[22] 张解放, 金美贞, 胡文成 2020 69 244205
Google Scholar
Zhang J F, Jin M Z, Hu W C 2020 Acta Phys. Sin. 69 244205
Google Scholar
[23] 张解放, 金美贞 2020 69 214203
Google Scholar
Zhang J F, Jin M Z 2020 Acta Phys. Sin. 69 214203
Google Scholar
[24] Zakharov V E (edited by Bullough R, Caudrey P) 1980 The Inverse Scattering Method (Vol. 17) (Berlin: Springer) pp243–285 DOI: 10.1007/978-3-642-81448-8_7
[25] Radha R, Lakshmanan M 1994 Inverse Problems 10 L29
Google Scholar
[26] Strachan I A B 1992 Inverse Problems 8 L21
Google Scholar
[27] Radha R, Lakshmanan M 1997 J. Phys. A:Math. Gen. 30 3229
Google Scholar
[28] 沈守枫, 张隽 2008 应用数学和力学 29 1254
Google Scholar
Shen S F, Zhang J 2008 Appl. Math. Mech. 29 1254
Google Scholar
[29] Wang J, Chen L W, Liu C F 2014 Appl. Math. Comput. 249 76
[30] 程丽, 张翼 2016 长江大学学报(自科版) 13 35
Chen L, Zhang Y 2016 J. Yangtze Univ. (Nat. Sci. Ed.) 13 35 (in Chinese)
[31] Wang X B, Tian S F, Zhang T T 2018 Proceedings of Roceedings of the American Society 146 3353
Google Scholar
[32] Chen M D, Li B 2017 Modern Phys. Lett. B 31 1750298
[33] Fokas A S 1994 Inverse Problems 10 19
Google Scholar
[34] Chen J C, Chen Y 2014 J. Nonlinear Math. Phys. 21 454
[35] Radha R, Lakshmanan M 1997 Chaos, Solitons and Fractals 8 17
Google Scholar
[36] Villarroel J, Prada J, Estévez P G 2009 Stud. Appl. Math. 122 395
Google Scholar
[37] Estévez P G 1999 J. Math. Phys. 40 1406
Google Scholar
[38] Rao J G, Wang L H, Zhang Y, He J S 2015 Commun. Theor. Phys. 64 605
Google Scholar
[39] Chen T T, Hu P Y, He J S 2019 Commun. Theor. Phys. 71 496
Google Scholar
[40] Rao J G, Mihalacheb D, Cheng Y, He J S 2019 Phys. Lett. A 383 1138
Google Scholar
下载:
计量
- 文章访问数: 3731
- PDF下载量: 83
- 被引次数: 0
