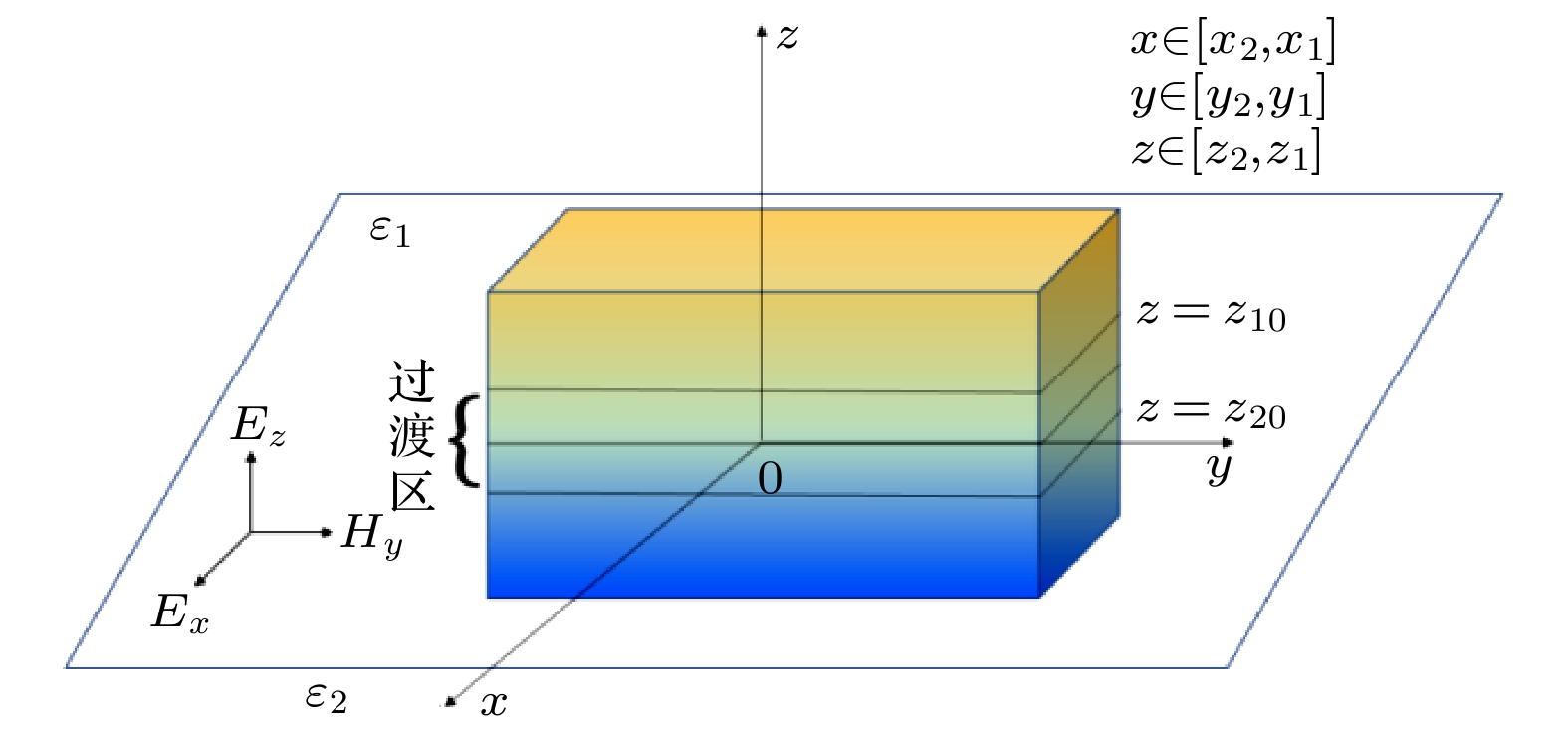
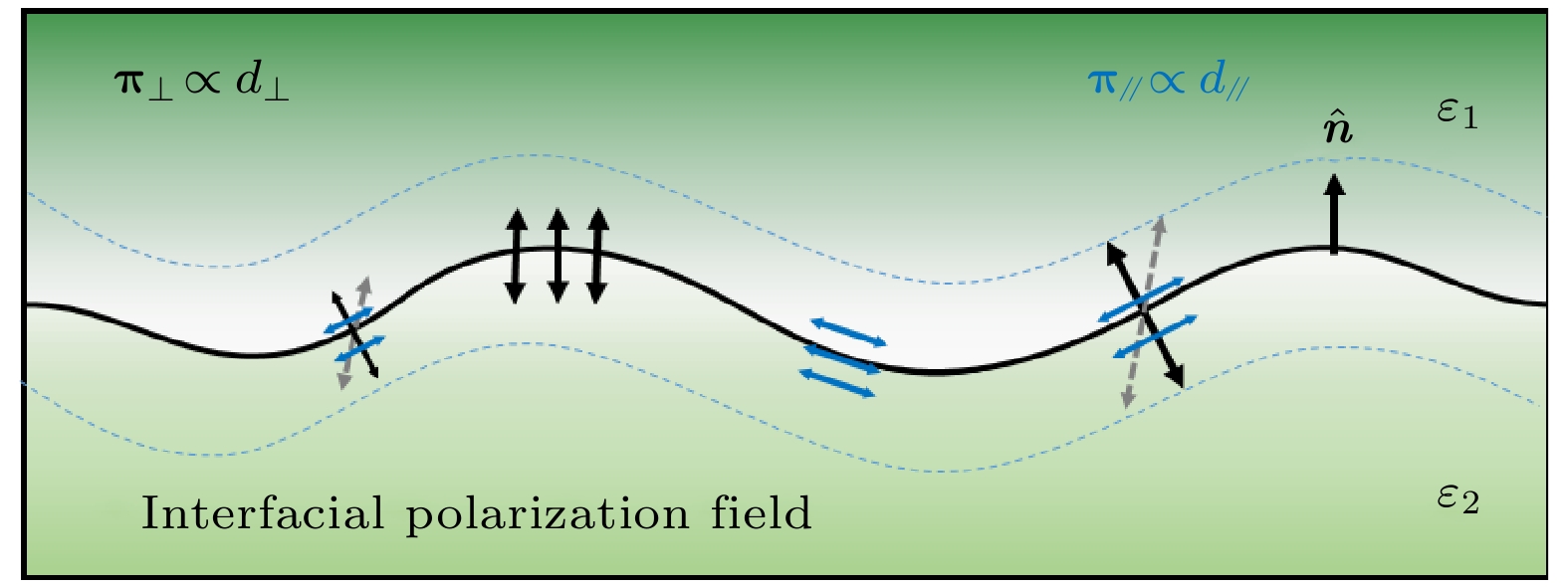
-
电磁边界条件是研究界面光学、电磁学性质的物理基础. 本文考虑界面上物质响应的非突变特性在两种材料界面上构建过渡层, 利用积分形式的麦克斯韦方程组, 推导出纳米尺度下的电磁边界条件, 得到的两个界面响应函数
${d}_{\perp }, {d}_{//}$ 分别对应于界面等效极化电荷的中心位置和界面极化电流密度沿垂直界面方向的梯度中心位置, 根据界面响应函数的定义式分析了界面响应函数受界面两侧材料的介电常数、电场过渡线型、频率等因素的影响. 发现铜-银界面上的界面响应函数可以高达数纳米. 当界面响应函数可以忽略不计时, 纳米电磁边界条件退化为突变结给出的经典的边界条件. 在此基础上, 引入界面电偶极矩, 界面上的等效极化电荷面密度、界面极化电流线密度和磁荷流密度参量给出3种不同形式的纳米电磁边界条件. 该模型对研究纳米尺度电磁学、界面光学问题提供了清晰的物理图像和必要的理论基础.The electromagnetic boundary conditions have great important applications in many physical branchs. Here, the nanoscale electromagnetic boundary conditions are derived by using the integral Maxwell’s equations through constructing the dielectric transition layer across the interface between the two materials. The two interface response functions are obtained to reflect the electromagnetic field response characteristics of the interface. Based on the Maxwell’s equations, the physical meanings of the interface response functions are given as the position of the equivalent interfacial polarization charge and the gradient position of interfacial polarization current density, respectively. The influence of the dielectric constant of the medium, the transition line shape of the electric field and the frequency on the interface response functions are analyzed. When the material scale is large, the interface response function can be ignored, and the nanoscale electromagnetic boundary conditions degenerate to the classical boundary conditions given by the abrupt junction. On this basis, the interface electric dipole moment, the equivalent interfacial polarization charge area density, the equivalent interfacial polarization current density and the equivalent interfacial magnetic current density are introduced, leading to three forms of nanoscale electromagnetic boundary conditions. The results provide a clear physical picture and necessary theoretical basis for nanoscale electromagnetism and interface optics.[1] Born M, Wolf E 1999 Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light (7th Expanded Eidition) (Cambridge: Cambridge University Press)
[2] 郭硕鸿 2008 电动力学 (第三版) (北京: 高等教育出版社) 第24−28页
Guo S H 2008 Electrodynamics (3rd Ed.) (Beijing: Higher Education Press) pp24−28 (in Chinese)
[3] Chikkaraddy R, de Nijs B, Benz F, Barrow S J, Scherman O A, Rosta E, Demetriadou A, Fox P, Hess O, Baumberg J J 2016 Nature 535 127
Google Scholar
[4] Christensen T, Yan W, Jauho A-P, Soljacic M, Mortensen N A 2017 Phys. Rev. Lett. 118 157402
Google Scholar
[5] Ciraci C, Hill R T, Mock J J, Urzhumov Y, Fernandez-Dominguez A I, Maier S A, Pendry J B, Chilkoti A, Smith D R 2012 Science 337 1072
Google Scholar
[6] Cottancin E, Celep G, Lerme J, Pellarin M, Huntzinger J R, Vialle J L, Broyer M 2006 Theor. Chem. Acc. 116 514
Google Scholar
[7] Feibelman P J 1982 Prog. Surf. Sci. 12 287
Google Scholar
[8] Feibelman P J 1994 Phys. Re. Lett. 72 788
Google Scholar
[9] Yang Y, Zhu D, Yan W, Agarwal A, Zheng M, Joannopoulos J D, Lalanne P, Christensen T, Berggren K K, Soljacic M 2019 Nature 576 248
Google Scholar
[10] Liebsch A (Edited by Halevi P) 1995 Photonic Probes of Surfaces (Amsterdam: Elsevier)
[11] Scholl J, Koh A, Dionne J 2012 Nature 483 421
Google Scholar
[12] Apell P 1981 Physica Scripta 24 795
Google Scholar
[13] Johnson P B, Christy R W 1972 Phys. Rev. B 6 4370
Google Scholar
-
图 1 计算电场切向分量的跃变使用的积分区域
Fig. 1. Integral area for calculating the tangential component discontinuity of the electric field.
图 2 计算电位移矢量法向分量跃变使用的积分区域
Fig. 2. Integral box for obtaining the normal component discontinuity of the electric displacement vector.
图 3 界面电偶极矩
${\boldsymbol{\pi }}_{\perp }, {\boldsymbol{\pi }}_{// }$ 的物理图示Fig. 3. Equivalent interfacial electric dipole moment
${\boldsymbol{\pi }}_{\perp }, {\boldsymbol{\pi }}_{// }$ .
图 4 不同函数形式下银-真空界面上
$ {d}_{\perp } $ (实线)以及金-真空界面以指数形式过渡的$ {d}_{\perp } $ (虚线)实部(a), 虚部(b) 随频率的变化Fig. 4. Real part (a) and image part (b) variations of the interfacial response function
$ {d}_{\perp } $ with the frequency for different function forms. Solid lines are for the Ag-vacuum interface and the dashed line represents$ {d}_{\perp } $ at the interface of Au-vacuum.
图 5 不同金属-真空界面
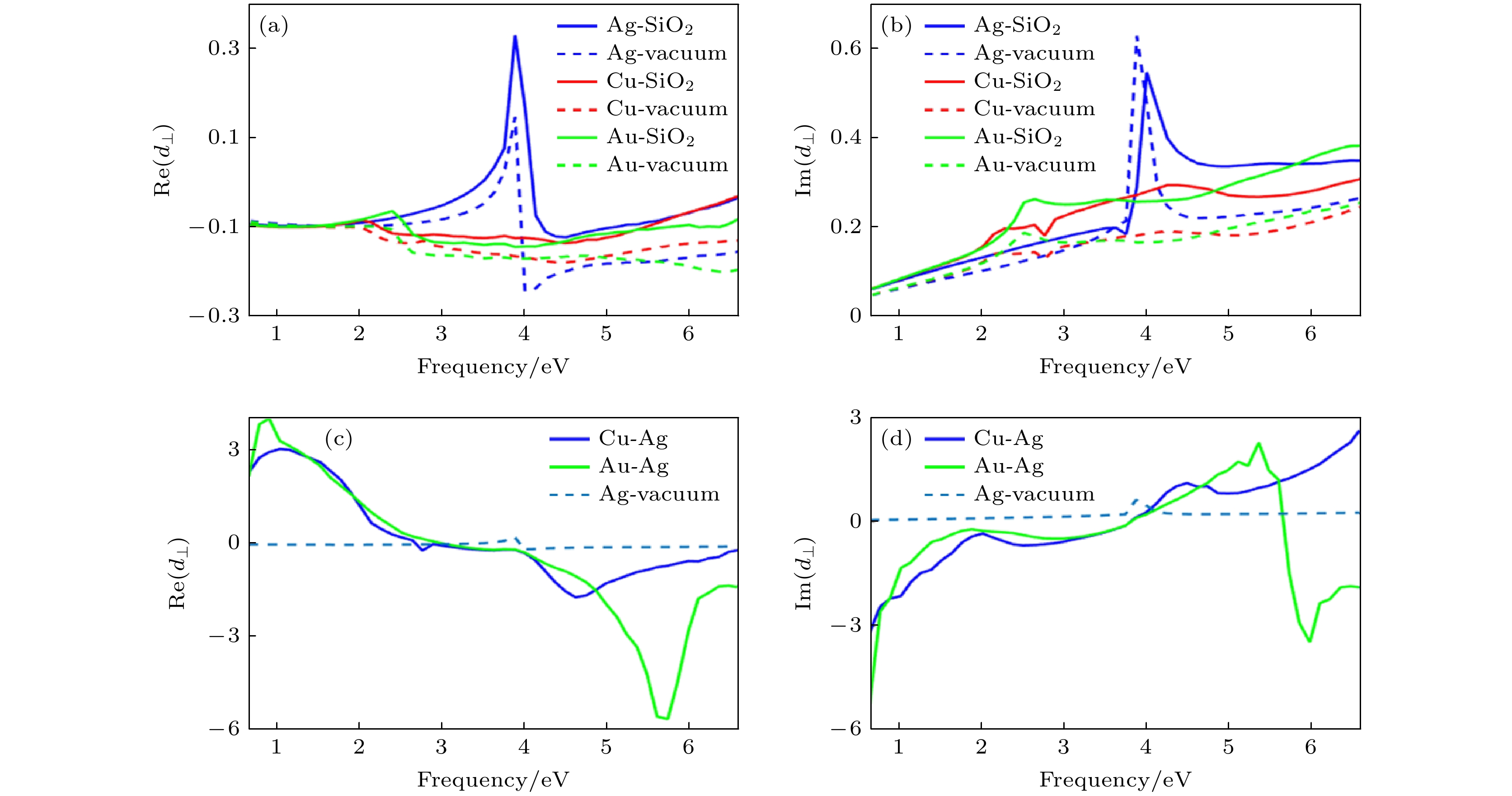
$ {d}_{\perp } $ (实线)以及金属-二氧化硅界面的$ {d}_{\perp } $ (虚线)实部 (a) 与虚部 (b) 随频率的变化; (c) (d) 金属-金属界面$ {d}_{\perp } $ (实线)以及金属-介质界面的$ {d}_{\perp } $ (虚线)的实部 (c) 与虚部 (d) 随频率的变化Fig. 5. Real part (a) and image part (b) variations of the interfacial response function
$ {d}_{\perp } $ with the frequency of metal-vacuum interface in contrast with metal-$ {\rm{S}}{\rm{i}}{{\rm{O}}}_{2} $ interface. Real part (c) and image part (d) variations of the interfacial response function$ {d}_{\perp } $ with the frequency of metal-metal interface in contrast with metal-dielectric interface. -
[1] Born M, Wolf E 1999 Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light (7th Expanded Eidition) (Cambridge: Cambridge University Press)
[2] 郭硕鸿 2008 电动力学 (第三版) (北京: 高等教育出版社) 第24−28页
Guo S H 2008 Electrodynamics (3rd Ed.) (Beijing: Higher Education Press) pp24−28 (in Chinese)
[3] Chikkaraddy R, de Nijs B, Benz F, Barrow S J, Scherman O A, Rosta E, Demetriadou A, Fox P, Hess O, Baumberg J J 2016 Nature 535 127
Google Scholar
[4] Christensen T, Yan W, Jauho A-P, Soljacic M, Mortensen N A 2017 Phys. Rev. Lett. 118 157402
Google Scholar
[5] Ciraci C, Hill R T, Mock J J, Urzhumov Y, Fernandez-Dominguez A I, Maier S A, Pendry J B, Chilkoti A, Smith D R 2012 Science 337 1072
Google Scholar
[6] Cottancin E, Celep G, Lerme J, Pellarin M, Huntzinger J R, Vialle J L, Broyer M 2006 Theor. Chem. Acc. 116 514
Google Scholar
[7] Feibelman P J 1982 Prog. Surf. Sci. 12 287
Google Scholar
[8] Feibelman P J 1994 Phys. Re. Lett. 72 788
Google Scholar
[9] Yang Y, Zhu D, Yan W, Agarwal A, Zheng M, Joannopoulos J D, Lalanne P, Christensen T, Berggren K K, Soljacic M 2019 Nature 576 248
Google Scholar
[10] Liebsch A (Edited by Halevi P) 1995 Photonic Probes of Surfaces (Amsterdam: Elsevier)
[11] Scholl J, Koh A, Dionne J 2012 Nature 483 421
Google Scholar
[12] Apell P 1981 Physica Scripta 24 795
Google Scholar
[13] Johnson P B, Christy R W 1972 Phys. Rev. B 6 4370
Google Scholar
下载:
计量
- 文章访问数: 5459
- PDF下载量: 130
- 被引次数: 0
