一类任意<i>m</i>×<i>n</i>阶矩形网络的电特性

谭志中; 谭震

doi:10.7498/aps.69.20191303

摘要

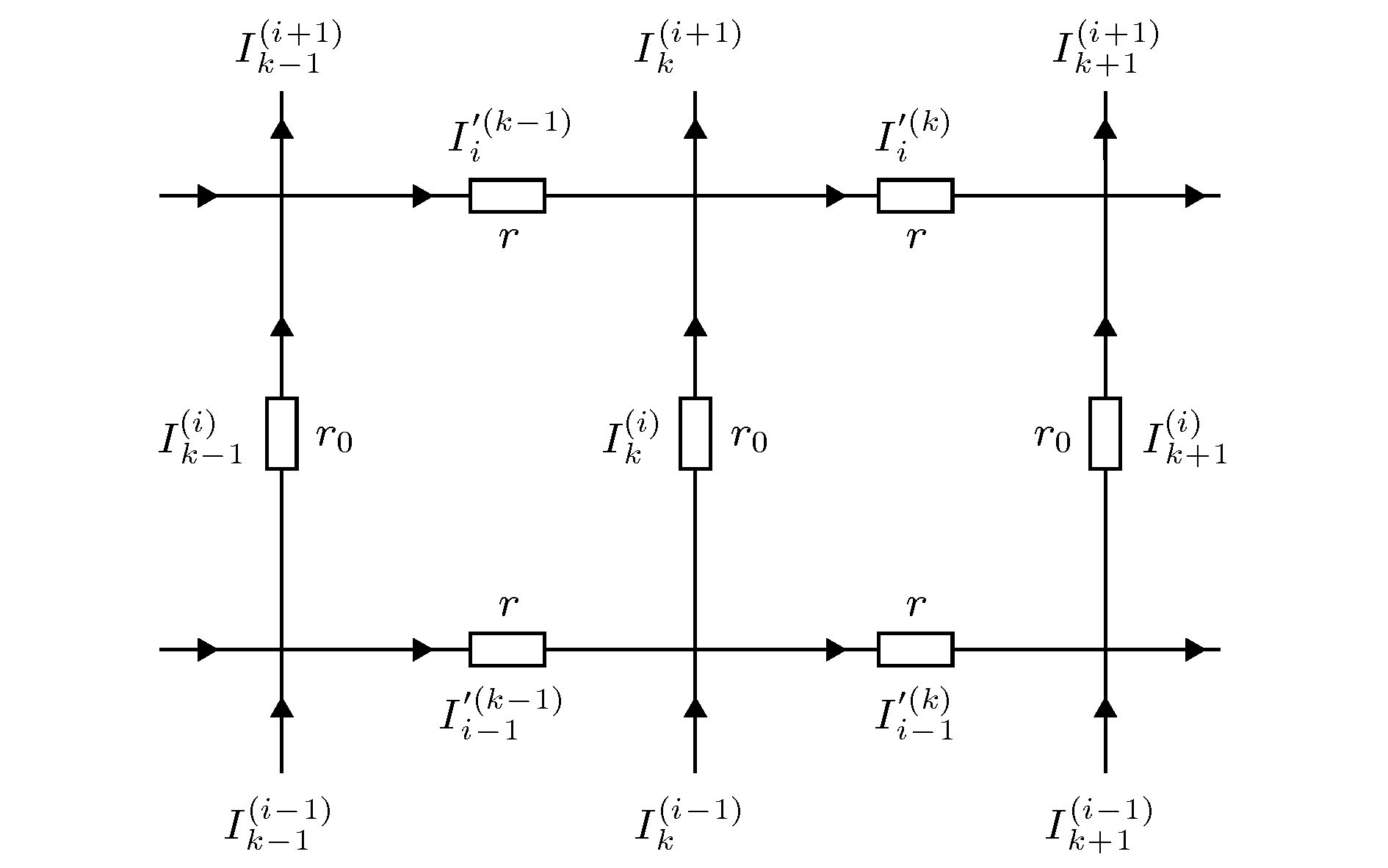
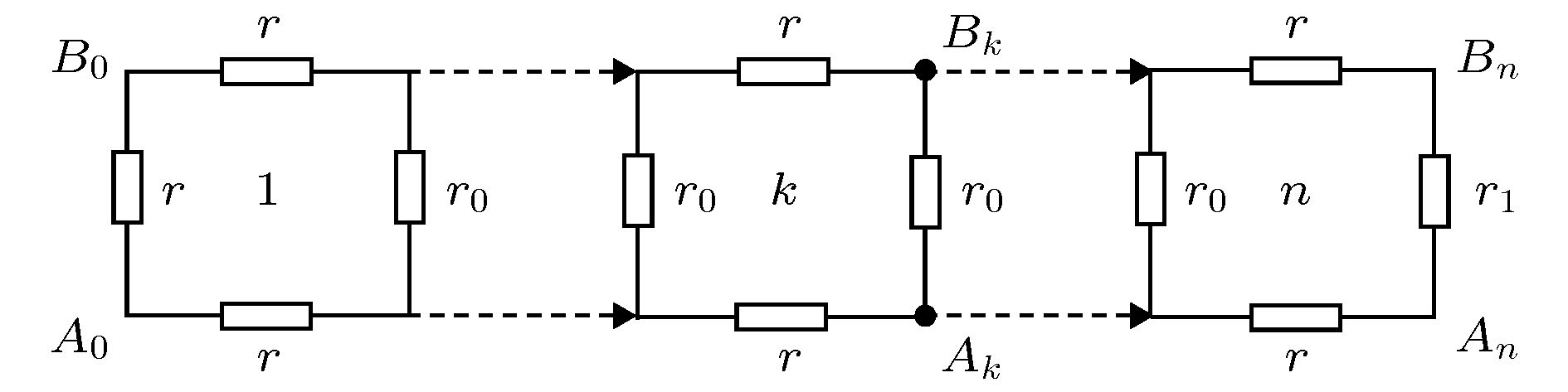
任意矩形电路网络中的电位分布问题一直是科学研究的难题. 本研究发展了研究电阻网络的递推-变换(RT)理论使之能够用于计算任意m × n阶电路网络模型. 研究了一类含有任意边界的m × n阶矩形网络的电位分布及等效电阻, 这是一个之前一直没有解决的深刻问题, 因为之前的研究依赖于规则的边界条件或一个含有零电阻的边界条件. 其他方法如格林函数技术和拉普拉斯矩阵方法计算电位函数比较困难, 研究含有任意边界的电阻网络也是不可能的. 电位函数问题是自然科学和工程技术领域研究的一个重要内容, 如拉普拉斯方程的求解问题就是其中之一. 本文给出了含有一条任意边界的m × n矩形电阻网络的节点电位函数解析式, 并且得到了任意两节点间的等效电阻公式, 同时导出了一些特殊情形下的结果. 在对不同结果的比较研究时, 得到了一个新的数学分式恒等式.

关键词:

Abstract

The development of natural science raises many complex new problems and requires people to find the basic method to resolve them. It was found that many problems could be resolved by building the resistor network model. In 1845, the German scientist Kirchhoff set up the node current law and the circuit voltage law.Since then the basic theory of electric circuit has been established. At present, three general theories for studying large-scale resistor networks have been developed, for example, In 2000 Cserti [Am. J. Phys. 2000, 68 , 896] set up the Green function technique to evaluate the resistance of infinite lattices. In 2004 Wu [J. Phys. A: Math. Gen. 2014, 37 , 6653] formulated a Laplacian matrix method and calculated the resistance of arbitrary finite and infinite lattices by using the eigenvalues and eigenvectors. In 2011 Tan [Resistance Network Model (Xi’an: Xidian University Press) 2011, pp16–216] proposed the recursion-transform (RT) method which depends on the one matrix along one directions and avoids the trouble of the Laplacian method that depends on two matrices along two directions. Among them, only two theories can calculate both finite and infinite networks. One is Wu's Laplacian matrix method and the other is Tan's RT method. However, there is only one way to compute a resistor network with arbitrary boundary, that is, the Tan's RT method.Potential distribution problem in arbitrary rectangular circuit network has always been a problem of scientific research. In this paper, we develop the RT-I theory of resistor networks to calculate the arbitrary m × n circuit network model. We study the potential distribution and the equivalent resistance of a class of m × n rectangular network with an arbitrary boundary, a profound problem that has not been resolved so far, because previous research depends on the boundary conditions of rules or a zero-resistance boundary condition. Other methods, such as Green function technique and Laplacian method to calculate potential function are difficult and also impossible to study the resistor network with arbitrary boundary. Potential function problem is an important research subject in natural science and engineering technology, for example, the solution of Laplace's equation is one of research work. In this paper, we present an analytical expression of the node potential function of m × n rectangular resistor network with an arbitrary boundary, and also obtainan equivalent resistance formula between any two nodes, and the results in some special cases as well. In the comparative study of different results, a new mathematical identity and several interesting inferences are discovered.

Keywords:

作者及机构信息

谭志中¹,
谭震²

1.
南通大学物理系, 南通　226019

2.
南通大学信息科学技术学院, 南通　226019

通信作者: 谭志中, tanzzh@163.com

基金项目: 江苏省自然科学基金(批准号: BK20161278)资助的课题

Authors and contacts

Tan Zhi-Zhong¹,
Tan Zhen²

1.
Department of Physics, Nantong University, Nantong 226019, China

2.
School of Information Science and Technology, Nantong University, Nantong 226019, China

Corresponding author: Tan Zhi-Zhong, tanzzh@163.com

Funds: Project supported by the Natural Science Foundation of Jiangsu Province, China (Grant No. BK20161278)

文章全文 : translate this paragraph

参考文献

[1]	Kirkpatrick S 1973 Rev. Mod. Phys. 45 574 Google Scholar
[2]	Melnikov A V, Shuba M, Lambin P 2018 Phys. Rev. E 97 043307 Google Scholar
[3]	Cserti J 2000 Am. J. Phys. 68 896 Google Scholar
[4]	Cserti J, David G, Piroth A 2002 Am. J. Phys. 70 153 Google Scholar
[5]	Cserti J, Szechenyi G, David G 2011 J. Phys. A: Math. Theor. 44 215201 Google Scholar
[6]	梁昆淼, 刘法, 缪国庆 1998 数学物理方法 (北京: 高等教育出版社) 第459−467页 Liang K M, Liu F, Miao G Q 1998 Mathematical Physics Methods (Beijing: Higher Education Press) pp459−467 (in Chinese)
[7]	Asad J H, Diab A A, Hijjawi R S, Khalifeh J M 2013 Eur. Phys. J. Plus 128 1 Google Scholar
[8]	Asad J H 2013 J. Stat. Phys. 150 1177 Google Scholar
[9]	Owaidat M Q, Hijjawi R S, Khalifeh J M 2014 Eur. Phys. J. Plus 129 29 Google Scholar
[10]	Owaidat M Q, Asad J H, Tan Z Z 2016 Int. J. Mod. Phys. B 30 1650166 Google Scholar
[11]	Owaidat M Q, Al-Badawi A A, Asad J H, Al-Twiessi M 2018 Chin. Phys. Lett. 35 020502 Google Scholar
[12]	Owaidat M Q, Asad J H 2016 Eur. Phys. J. Plus 131 309 Google Scholar
[13]	Wu F Y 2004 J. Phys. A: Math. Gen. 37 6653 Google Scholar
[14]	Tzeng W J, Wu F Y 2006 J. Phys. A: Math. Gen. 39 8579 Google Scholar
[15]	Izmailian N S, Huang M C 2010 Phys. Rev. E 82 011125 Google Scholar
[16]	Essam J W, Wu F Y 2009 J. Phys. A: Math. Theor. 42 025205 Google Scholar
[17]	Chair N 2012 Ann. Phys. 327 3116 Google Scholar
[18]	Chair N 2014 Ann. Phys. 341 56 Google Scholar
[19]	Chair N 2014 J. Stat. Phys. 154 1177 Google Scholar
[20]	Izmailian N S, Kenna R, Wu F Y 2014 J. Phys. A: Math. Theor. 47 035003 Google Scholar
[21]	Izmailian N S, Kenna R 2014 J. Stat. Mech. E 09 1742
[22]	Izmailian N S, Kenna R 2015 Chin. J. Phys. 53 040703
[23]	Essam J W, Izmailyan N S, Kenna R, Tan Z Z 2015 Roy. Soc. Open Sci. 2 140420 Google Scholar
[24]	谭志中 2011 电阻网络模型 (西安: 西安电子科技大学出版社) 第16—216页 Tan Z Z 2011 Resistance Network Model (Xi’an: Xidian University Press) pp16–216 (in Chinese)
[25]	Tan Z Z, Zhou L, Yang J H 2013 J. Phys. A: Math. Theor. 46 195202 Google Scholar
[26]	Tan Z Z, Essam J W, Wu F Y 2014 Phys. Rev. E 90 012130 Google Scholar
[27]	Essam J W, Tan Z Z, Wu F Y 2014 Phys. Rev. E 90 032130 Google Scholar
[28]	Tan Z Z 2015 Chin. Phys. B 24 020503 Google Scholar
[29]	Tan Z Z 2015 Phys. Rev. E 91 052122
[30]	Tan Z Z 2015 Sci. Rep. 5 11266 Google Scholar
[31]	Tan Z Z 2015 Int. J. Circ. Theor. Appl. 43 1687 Google Scholar
[32]	Tan Z Z, Zhang Q H 2015 Int. J. Circ. Theor. Appl. 43 944 Google Scholar
[33]	Tan Z Z 2016 Chin. Phys. B 25 050504 Google Scholar
[34]	谭志中, 张庆华 2017 66 070501 Google Scholar Tan Z Z, Zhang Q H 2017 Acta Phys. Sin. 66 070501 Google Scholar
[35]	Tan Z Z 2017 Chin. Phys. B 26 090503 Google Scholar
[36]	Tan Z, Tan Z Z, Chen J X 2018 Sci. Rep. 8 5798 Google Scholar
[37]	Tan Z Z, Asad J H, Owaidat M Q 2017 Int. J. Circ. Theor. Appl. 45 1942 Google Scholar
[38]	Zhou L, Tan Z Z, Zhang Q H 2017 Front. Inf. Technol. Electron. Eng. 18 1186 Google Scholar
[39]	Tan Z, Tan Z Z, Asad J H, Owaidat M Q 2019 Phys. Scripta 94 055203 Google Scholar
[40]	Tan Z Z, Zhu H, Asad J H, Xu C, Tang H 2017 Front. Inf. Technol. Electron. Eng. 18 2070 Google Scholar
[41]	Tan Z, Tan Z Z, Zhou L 2018 Commun. Theor. Phys. 69 610 Google Scholar
[42]	Tan Z, Tan Z Z 2018 Sci. Rep. 8 9937 Google Scholar
[43]	Tan Z Z 2017 Commun. Theor. Phys. 67 280 Google Scholar

施引文献

图 1 含有一个任意边界的矩形电阻网络, 右边界上的任意电阻为r₁, 其他在水平和竖直方向的电阻元素分别为r和r₀

Fig. 1. An arbitrary rectangular m × n resistor network with an arbitrary boundary. Bonds in the horizontal and vertical directions are resistors r and r₀ except for r₁ on the right boundary.

下载: 全尺寸图片幻灯片

图 2 含有电流参数和方向的部分电阻网络

Fig. 2. Segment of resistor network with current directions and parameters.

下载: 全尺寸图片幻灯片

图 3 一类任意m × n阶Fan电阻网络模型

Fig. 3. An arbitrary m × n Fan resistor network.

下载: 全尺寸图片幻灯片

图 4 一类含有任意右边界的1 × n阶电阻网络

Fig. 4. 1 × n resistor network with an arbitrary right boundary.

下载: 全尺寸图片幻灯片

map

[1]	Kirkpatrick S 1973 Rev. Mod. Phys. 45 574 Google Scholar
[2]	Melnikov A V, Shuba M, Lambin P 2018 Phys. Rev. E 97 043307 Google Scholar
[3]	Cserti J 2000 Am. J. Phys. 68 896 Google Scholar
[4]	Cserti J, David G, Piroth A 2002 Am. J. Phys. 70 153 Google Scholar
[5]	Cserti J, Szechenyi G, David G 2011 J. Phys. A: Math. Theor. 44 215201 Google Scholar
[6]	梁昆淼, 刘法, 缪国庆 1998 数学物理方法 (北京: 高等教育出版社) 第459−467页 Liang K M, Liu F, Miao G Q 1998 Mathematical Physics Methods (Beijing: Higher Education Press) pp459−467 (in Chinese)
[7]	Asad J H, Diab A A, Hijjawi R S, Khalifeh J M 2013 Eur. Phys. J. Plus 128 1 Google Scholar
[8]	Asad J H 2013 J. Stat. Phys. 150 1177 Google Scholar
[9]	Owaidat M Q, Hijjawi R S, Khalifeh J M 2014 Eur. Phys. J. Plus 129 29 Google Scholar
[10]	Owaidat M Q, Asad J H, Tan Z Z 2016 Int. J. Mod. Phys. B 30 1650166 Google Scholar
[11]	Owaidat M Q, Al-Badawi A A, Asad J H, Al-Twiessi M 2018 Chin. Phys. Lett. 35 020502 Google Scholar
[12]	Owaidat M Q, Asad J H 2016 Eur. Phys. J. Plus 131 309 Google Scholar
[13]	Wu F Y 2004 J. Phys. A: Math. Gen. 37 6653 Google Scholar
[14]	Tzeng W J, Wu F Y 2006 J. Phys. A: Math. Gen. 39 8579 Google Scholar
[15]	Izmailian N S, Huang M C 2010 Phys. Rev. E 82 011125 Google Scholar
[16]	Essam J W, Wu F Y 2009 J. Phys. A: Math. Theor. 42 025205 Google Scholar
[17]	Chair N 2012 Ann. Phys. 327 3116 Google Scholar
[18]	Chair N 2014 Ann. Phys. 341 56 Google Scholar
[19]	Chair N 2014 J. Stat. Phys. 154 1177 Google Scholar
[20]	Izmailian N S, Kenna R, Wu F Y 2014 J. Phys. A: Math. Theor. 47 035003 Google Scholar
[21]	Izmailian N S, Kenna R 2014 J. Stat. Mech. E 09 1742
[22]	Izmailian N S, Kenna R 2015 Chin. J. Phys. 53 040703
[23]	Essam J W, Izmailyan N S, Kenna R, Tan Z Z 2015 Roy. Soc. Open Sci. 2 140420 Google Scholar
[24]	谭志中 2011 电阻网络模型 (西安: 西安电子科技大学出版社) 第16—216页 Tan Z Z 2011 Resistance Network Model (Xi’an: Xidian University Press) pp16–216 (in Chinese)
[25]	Tan Z Z, Zhou L, Yang J H 2013 J. Phys. A: Math. Theor. 46 195202 Google Scholar
[26]	Tan Z Z, Essam J W, Wu F Y 2014 Phys. Rev. E 90 012130 Google Scholar
[27]	Essam J W, Tan Z Z, Wu F Y 2014 Phys. Rev. E 90 032130 Google Scholar
[28]	Tan Z Z 2015 Chin. Phys. B 24 020503 Google Scholar
[29]	Tan Z Z 2015 Phys. Rev. E 91 052122
[30]	Tan Z Z 2015 Sci. Rep. 5 11266 Google Scholar
[31]	Tan Z Z 2015 Int. J. Circ. Theor. Appl. 43 1687 Google Scholar
[32]	Tan Z Z, Zhang Q H 2015 Int. J. Circ. Theor. Appl. 43 944 Google Scholar
[33]	Tan Z Z 2016 Chin. Phys. B 25 050504 Google Scholar
[34]	谭志中, 张庆华 2017 66 070501 Google Scholar Tan Z Z, Zhang Q H 2017 Acta Phys. Sin. 66 070501 Google Scholar
[35]	Tan Z Z 2017 Chin. Phys. B 26 090503 Google Scholar
[36]	Tan Z, Tan Z Z, Chen J X 2018 Sci. Rep. 8 5798 Google Scholar
[37]	Tan Z Z, Asad J H, Owaidat M Q 2017 Int. J. Circ. Theor. Appl. 45 1942 Google Scholar
[38]	Zhou L, Tan Z Z, Zhang Q H 2017 Front. Inf. Technol. Electron. Eng. 18 1186 Google Scholar
[39]	Tan Z, Tan Z Z, Asad J H, Owaidat M Q 2019 Phys. Scripta 94 055203 Google Scholar
[40]	Tan Z Z, Zhu H, Asad J H, Xu C, Tang H 2017 Front. Inf. Technol. Electron. Eng. 18 2070 Google Scholar
[41]	Tan Z, Tan Z Z, Zhou L 2018 Commun. Theor. Phys. 69 610 Google Scholar
[42]	Tan Z, Tan Z Z 2018 Sci. Rep. 8 9937 Google Scholar
[43]	Tan Z Z 2017 Commun. Theor. Phys. 67 280 Google Scholar

[1]	崔俊英, 徐舒琪, 那旭, 潘黎明, 吕琳媛. 基于复杂网络理论的供应链研究. , 2024, 73(19): 198901. doi: 10.7498/aps.73.20240702
[2]	赖煜成, 陈苏琪, 牟兰雅, 王兆娜. 基于麦克斯韦方程组的纳米尺度电磁边界条件. , 2021, 70(23): 230301. doi: 10.7498/aps.70.20211025
[3]	林晨森, 陈硕, 肖兰兰. 适用复杂几何壁面的耗散粒子动力学边界条件. , 2019, 68(14): 140204. doi: 10.7498/aps.68.20190533
[4]	武喜萍, 杨红雨, 韩松臣. 基于复杂网络理论的多元混合空管技术保障系统网络特征分析. , 2016, 65(14): 140203. doi: 10.7498/aps.65.140203
[5]	欧阳博, 金心宇, 夏永祥, 蒋路茸, 吴端坡. 疾病传播与级联失效相互作用的研究:度不相关网络中疾病扩散条件的分析. , 2014, 63(21): 218902. doi: 10.7498/aps.63.218902
[6]	吴腾飞, 周昌乐, 王小华, 黄孝喜, 谌志群, 王荣波. 基于平均场理论的微博传播网络模型. , 2014, 63(24): 240501. doi: 10.7498/aps.63.240501
[7]	王亚奇, 王静, 杨海滨. 基于复杂网络理论的微博用户关系网络演化模型研究. , 2014, 63(20): 208902. doi: 10.7498/aps.63.208902
[8]	李雨珊, 吕翎, 刘烨, 刘硕, 闫兵兵, 常欢, 周佳楠. 复杂网络时空混沌同步的Backstepping设计. , 2013, 62(2): 020513. doi: 10.7498/aps.62.020513
[9]	刘汉涛, 常建忠. 直接模拟中不同边界条件的实施及对沉降规律的影响. , 2013, 62(8): 084401. doi: 10.7498/aps.62.084401
[10]	吕翎, 李钢, 徐文, 吕娜, 范鑫. 复Ginzburg-Landau方程时空混沌的网络同步与参量辨识. , 2012, 61(6): 060507. doi: 10.7498/aps.61.060507
[11]	吕翎, 商锦玉, 朱佳博, 沈娜, 柳爽, 张新. 激光Maxwell-Bloch 方程时空混沌网络的同步研究. , 2012, 61(14): 140504. doi: 10.7498/aps.61.140504
[12]	梁义, 王兴元. 基于低阶矩阵最大特征值的复杂网络牵制混沌同步. , 2012, 61(3): 038901. doi: 10.7498/aps.61.038901
[13]	郑啸, 陈建平, 邵佳丽, 别立东. 基于复杂网络理论的北京公交网络拓扑性质分析. , 2012, 61(19): 190510. doi: 10.7498/aps.61.190510
[14]	刘刚, 李永树. 基于引力场理论的复杂网络路由选择策略研究. , 2012, 61(24): 248901. doi: 10.7498/aps.61.248901
[15]	任刚, 陆丽丽, 王炜. 基于元胞自动机和复杂网络理论的双向行人流建模. , 2012, 61(14): 144501. doi: 10.7498/aps.61.144501
[16]	周漩, 张凤鸣, 李克武, 惠晓滨, 吴虎胜. 利用重要度评价矩阵确定复杂网络关键节点. , 2012, 61(5): 050201. doi: 10.7498/aps.61.050201
[17]	崔爱香, 傅彦, 尚明生, 陈端兵, 周涛. 复杂网络局部结构的涌现:共同邻居驱动网络演化. , 2011, 60(3): 038901. doi: 10.7498/aps.60.038901
[18]	张旭, 周玉泽, 闭强, 杨兴华, 俎云霄. 有边界条件的忆阻元件模型及其性质. , 2010, 59(9): 6673-6680. doi: 10.7498/aps.59.6673
[19]	许丹, 李翔, 汪小帆. 复杂网络病毒传播的局域控制研究. , 2007, 56(3): 1313-1317. doi: 10.7498/aps.56.1313
[20]	李季, 汪秉宏, 蒋品群, 周涛, 王文旭. 节点数加速增长的复杂网络生长模型. , 2006, 55(8): 4051-4057. doi: 10.7498/aps.55.4051

计量

文章访问数: 9028
PDF下载量: 163
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

一类任意m×n阶矩形网络的电特性