-
提出并设计一种基于Pancharatnam-Berry (P-B)相位超表面的二维光学微分器, 并实现对光学图像的二维光学边缘检测. 在环形光栅相位的作用下, 该P-B相位超表面可将光束的左右旋分量在径向进行分离, 在滤除中间重叠部分的线偏振光后, 保留下来的光学信息即为二维光学微分结果. 同时, 通过调节该二维光学微分器的光轴分布函数可对边缘信息分辨率进行灵活调控. 研究结果表明, 上述P-B相位超表面可用于光学图像的二维边缘信息提取, 相比于一维光栅式超表面, 该方法得到的边缘信息更加完整、清晰. 可以预期, 这种二维光学微分器在超快光学计算与光学图像处理等方面具有重要的潜在应用价值.
-
关键词:
- 超表面 /
- Pancharatnam-Berry相位 /
- 光子自旋霍尔效应
With the rapid development of metasurface and metamaterials, the image edge detection based on the optical spatial differential calculation becomes an interesting topic in recent years. There have been a certain number of studies in this region, but most of them are applicable only to one-dimensional optical spatial differential calculation. In this work, a two-dimensional optical differentiator using Pancharatnam-Berry (P-B) phase metasurface is proposed and implemented in optical image two-dimensional edge detection. Based on the principle of the spin-dependent splitting from P-B phase devices, this metasurface is capable of separating the left-handed circularly polarized light from the right-handed circularly polarized light at a certain spatial distance. After filtering out the overlapped linear polarization, the left optical information is the result of the two-dimensional optical spatial differential. Meanwhile, the resolution of the image edge information is adjustable by changing the optic axis distribution of this two-dimensional optical differentiator. These results indicate that our P-B phase metasurface can be applied to the extraction of the optical image two-dimensional edge information, and the extracted edge information is more complete than the previous one-dimensional grating metasurface. For these advantages, this two-dimensional optical differentiator shows great potential applications in ultrafast optical calculation and image processing.-
Keywords:
- metasurface /
- Pancharatnam-Berry phase /
- photonic spin Hall effect
[1] Hubel D H, Wiesel T N 1962 J. Physiol 160 106
Google Scholar
[2] Farmahini-Farahani M, Cheng J, Mosallaei H 2013 J. Opt. Soc. Am. B 30 2365
Google Scholar
[3] Zhu T F, Lou Y J, Zhou Y H, Zhang J H, Huang J Y, Li Y, Luo H L, Wen S C, Zhu S Y, Gong Q H, Qiu M, Ruan Z C 2019 Phys. Rev. Appl. 11 034043
Google Scholar
[4] Silva A, Monticone F, Castaldi G, Galdi V, Alù A, Engheta N 2014 Science 343 160
Google Scholar
[5] Ruan Z C 2015 Opt. Lett. 40 601
Google Scholar
[6] Solli D R, Jalali B 2015 Nat. Photonics 9 704
Google Scholar
[7] Liu F F, Wang T, Qiang L, Ye T, Zhang Z Y, Qiu M, Su Y K 2008 Opt. Express 16 15800
[8] Slavík R, Park Y, Ayotte N, Doucet S, Ahn T, LaRochelle S, Azaña J 2008 Opt. Express 16 18202
Google Scholar
[9] Yang T, Dong J, Lu L, Zhou L, Zheng A, Zhang X, Chen J 2014 Sci. Rep. 4 5581
[10] Ruan Z C, Wu H, Qiu M, Fan S H 2014 Opt. Lett. 39 3587
Google Scholar
[11] Saba A, Tavakol M R, Karimi-Khoozani P, Khavasi A 2018 IEEE Photonics Technol. Lett. 30 853
Google Scholar
[12] Pors A, Nielsen M G, Bozhevolnyi S I 2015 Nano Lett. 15 791
Google Scholar
[13] Doskolovich L L, Bykov D A, Bezus E A, Soifer V A 2014 Opt. Lett. 39 1278
Google Scholar
[14] Zhu T F, Zhou Y H, Lou Y J, Ye H, Qiu M, Ruan Z C, Fan S H 2017 Nat. Commun. 8 15391
Google Scholar
[15] Zhou J X, Qian H L, Chen C F, Zhao J X, Li G R, Wu Q Y, Luo H L, Wen S C, Liu Z W 2019 P. Natl. Acad. Sci. USA 116 11137
Google Scholar
[16] Bykov D A, Doskolovich L L, Bezus E A, Soifer V A 2014 Opt. Express 22 25084
Google Scholar
[17] 程杨, 姚佰承, 吴宇, 王泽高, 龚元, 饶云江 2013 62 237805
Google Scholar
Cheng Y, Yao B C, Wu Y, Wang Z G, Gong Y, Rao Y J 2013 Acta Phys. Sin. 62 237805
Google Scholar
[18] 李鑫, 吴立祥, 杨元杰 2019 68 187103
Google Scholar
Li X, Wu L X, Yang Y J 2019 Acta Phys. Sin. 68 187103
Google Scholar
[19] 郭文龙, 王光明, 李海鹏, 侯海生 2016 65 074101
Google Scholar
Guo W L, Wang G M, Li H P, Hou H S 2016 Acta Phys. Sin. 65 074101
Google Scholar
[20] 余观夏, 付晶晶, 杜文文, 吕一航, 骆敏 2019 中国物理B 28 024101
Yu G X, Fu J J, Du W W, Lü Y H, Luo M 2019 Chinese Phys. B 28 024101
[21] Marrucci L, Manzo C, Paparo, D 2006 Phys. Rev. Lett. 96 163905
Google Scholar
[22] Biener G, Niv A, Kleiner V, Hasman E 2002 Opt. Lett. 27 1875
Google Scholar
[23] Luo W, Xiao S, He Q, Sun S, Zhou L 2015 Adv. Opt. Mater. 3 1102
Google Scholar
[24] Shitrit N, Bretner I, Gorodetski Y, Kleiner V, Hasman E 2011 Nano Lett. 11 2038
Google Scholar
[25] Yin X B, Ye Z L, 1 Rho J, Wang Y, Zhang X 2013 Science 339 1405
Google Scholar
[26] Luo X G, Pu M B, Li X, Ma X L 2017 Light Sci. Appl. 6 e16276
Google Scholar
[27] Declin R C, Khorasaninejad M, Chen W T, Oh J, Capasso F 2016 P. Natl. Acad. Sci. USA 113 10473
Google Scholar
[28] Declin R C, Ambrosio A, Rubin N A, Mueller J P B, Capasso F 2017 Science 358 896
Google Scholar
[29] Bomzon Z, Biener G, Kleiner V, Hasman E 2002 Opt. Lett. 27 1141
Google Scholar
-
图 1 (a)单元结构示意图; (b)与(c) x与y方向线偏振入射光相位响应与介质柱长(l)、宽(w)之间的关系; (d) x和y方向上的相位差随l和w变化关系; (e)介质柱的旋转角与附加相位关系图.
Fig. 1. (a) Schematic for basic unit structure; (b) and (c) phase response of different length (l) and width (w) of the dielectric column under x- and y- LP incident beams; (d) phase difference between the x- and y-polarized light for different length (l) and width (w) of the dielectric column; (e) relationship between the rotation angle of the dielectric column and the additional phase.
图 2 (a)光学二维边缘检测原理图; (b) LHCP与RHCP通过PB相位超表面后获得的相位梯度变化; (c) P-B相位超表面示意图; (d)和(e) RHCP与LHCP平面波通过超表面后波前变化图
Fig. 2. (a) Schematic diagram of the 2D optical edge detection; (b) phase gradient of the LHCP and RHCP component after the P-B phase matesurface; (c) diagram of the metasurface; (d) and (e) wavefront changes of RHCP and LHCP plane waves through the metasurface.
图 3 (a) 深圳大学校徽掩模板; (b)−(d)周期T = 4 mm, 2 mm, 1 mm时, 一维边缘检测效果; (e)−(g)周期T = 4 mm, 2 mm, 1 mm时二维边缘检测效果
Fig. 3. (a) The mask used in the simulation; (b)−(d) the result of 1D edge extraction when the period T = 4 mm, 2 mm, 1 mm; (e)−(g) the result of 2D edge extraction when the period T = 4 mm, 2 mm, 1 mm.
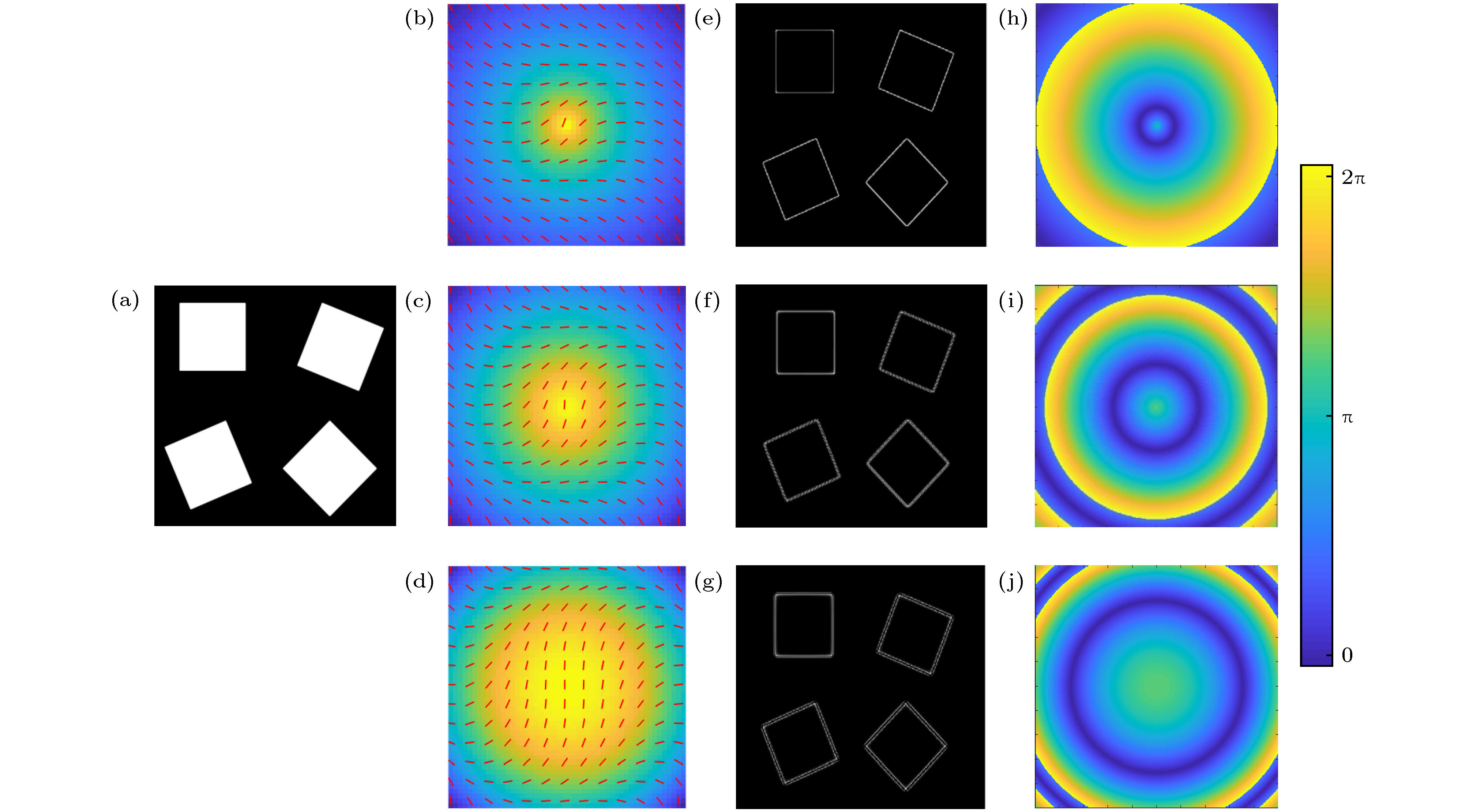
图 4 (a)形状不同的正方形掩膜板; (b)−(d)超表面的快轴分布以及LHCP通过超表面后的相位分布; (e)−(g)不同光轴分布的超表面实现边缘提取效果; (h)−(j)经过传输距离为0.1 m后LHCP和RHCP的相位差分布
Fig. 4. (a) Mask patterns of different squre; (b)−(d) metasurface fast-axis distributions and phase distributions of LHCP after metasurface; (e)−(g) results of the edge extraction with different Metasurface fast-axis distributions; (h)−(j) phase difference distributions of LHCP and RHCP at 0.1 m transmission distance.
图 5 幂指数不同的光轴分布函数分别在x与y方向上的空间传递函数
Fig. 5. Spatial spectral transfer function of the optical axis distribution functions with different power exponent in the x- and y- direction respectively.
-
[1] Hubel D H, Wiesel T N 1962 J. Physiol 160 106
Google Scholar
[2] Farmahini-Farahani M, Cheng J, Mosallaei H 2013 J. Opt. Soc. Am. B 30 2365
Google Scholar
[3] Zhu T F, Lou Y J, Zhou Y H, Zhang J H, Huang J Y, Li Y, Luo H L, Wen S C, Zhu S Y, Gong Q H, Qiu M, Ruan Z C 2019 Phys. Rev. Appl. 11 034043
Google Scholar
[4] Silva A, Monticone F, Castaldi G, Galdi V, Alù A, Engheta N 2014 Science 343 160
Google Scholar
[5] Ruan Z C 2015 Opt. Lett. 40 601
Google Scholar
[6] Solli D R, Jalali B 2015 Nat. Photonics 9 704
Google Scholar
[7] Liu F F, Wang T, Qiang L, Ye T, Zhang Z Y, Qiu M, Su Y K 2008 Opt. Express 16 15800
[8] Slavík R, Park Y, Ayotte N, Doucet S, Ahn T, LaRochelle S, Azaña J 2008 Opt. Express 16 18202
Google Scholar
[9] Yang T, Dong J, Lu L, Zhou L, Zheng A, Zhang X, Chen J 2014 Sci. Rep. 4 5581
[10] Ruan Z C, Wu H, Qiu M, Fan S H 2014 Opt. Lett. 39 3587
Google Scholar
[11] Saba A, Tavakol M R, Karimi-Khoozani P, Khavasi A 2018 IEEE Photonics Technol. Lett. 30 853
Google Scholar
[12] Pors A, Nielsen M G, Bozhevolnyi S I 2015 Nano Lett. 15 791
Google Scholar
[13] Doskolovich L L, Bykov D A, Bezus E A, Soifer V A 2014 Opt. Lett. 39 1278
Google Scholar
[14] Zhu T F, Zhou Y H, Lou Y J, Ye H, Qiu M, Ruan Z C, Fan S H 2017 Nat. Commun. 8 15391
Google Scholar
[15] Zhou J X, Qian H L, Chen C F, Zhao J X, Li G R, Wu Q Y, Luo H L, Wen S C, Liu Z W 2019 P. Natl. Acad. Sci. USA 116 11137
Google Scholar
[16] Bykov D A, Doskolovich L L, Bezus E A, Soifer V A 2014 Opt. Express 22 25084
Google Scholar
[17] 程杨, 姚佰承, 吴宇, 王泽高, 龚元, 饶云江 2013 62 237805
Google Scholar
Cheng Y, Yao B C, Wu Y, Wang Z G, Gong Y, Rao Y J 2013 Acta Phys. Sin. 62 237805
Google Scholar
[18] 李鑫, 吴立祥, 杨元杰 2019 68 187103
Google Scholar
Li X, Wu L X, Yang Y J 2019 Acta Phys. Sin. 68 187103
Google Scholar
[19] 郭文龙, 王光明, 李海鹏, 侯海生 2016 65 074101
Google Scholar
Guo W L, Wang G M, Li H P, Hou H S 2016 Acta Phys. Sin. 65 074101
Google Scholar
[20] 余观夏, 付晶晶, 杜文文, 吕一航, 骆敏 2019 中国物理B 28 024101
Yu G X, Fu J J, Du W W, Lü Y H, Luo M 2019 Chinese Phys. B 28 024101
[21] Marrucci L, Manzo C, Paparo, D 2006 Phys. Rev. Lett. 96 163905
Google Scholar
[22] Biener G, Niv A, Kleiner V, Hasman E 2002 Opt. Lett. 27 1875
Google Scholar
[23] Luo W, Xiao S, He Q, Sun S, Zhou L 2015 Adv. Opt. Mater. 3 1102
Google Scholar
[24] Shitrit N, Bretner I, Gorodetski Y, Kleiner V, Hasman E 2011 Nano Lett. 11 2038
Google Scholar
[25] Yin X B, Ye Z L, 1 Rho J, Wang Y, Zhang X 2013 Science 339 1405
Google Scholar
[26] Luo X G, Pu M B, Li X, Ma X L 2017 Light Sci. Appl. 6 e16276
Google Scholar
[27] Declin R C, Khorasaninejad M, Chen W T, Oh J, Capasso F 2016 P. Natl. Acad. Sci. USA 113 10473
Google Scholar
[28] Declin R C, Ambrosio A, Rubin N A, Mueller J P B, Capasso F 2017 Science 358 896
Google Scholar
[29] Bomzon Z, Biener G, Kleiner V, Hasman E 2002 Opt. Lett. 27 1141
Google Scholar
下载:
计量
- 文章访问数: 15452
- PDF下载量: 505
- 被引次数: 0
