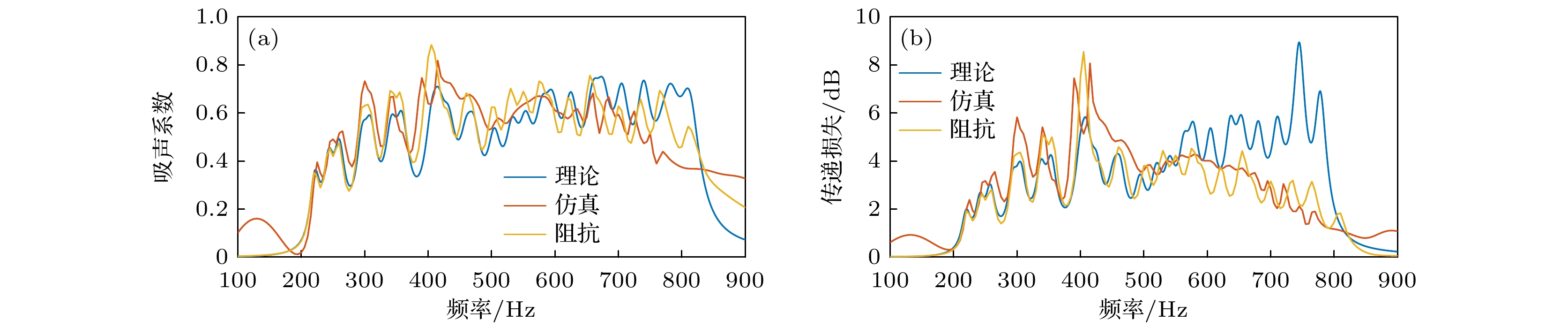
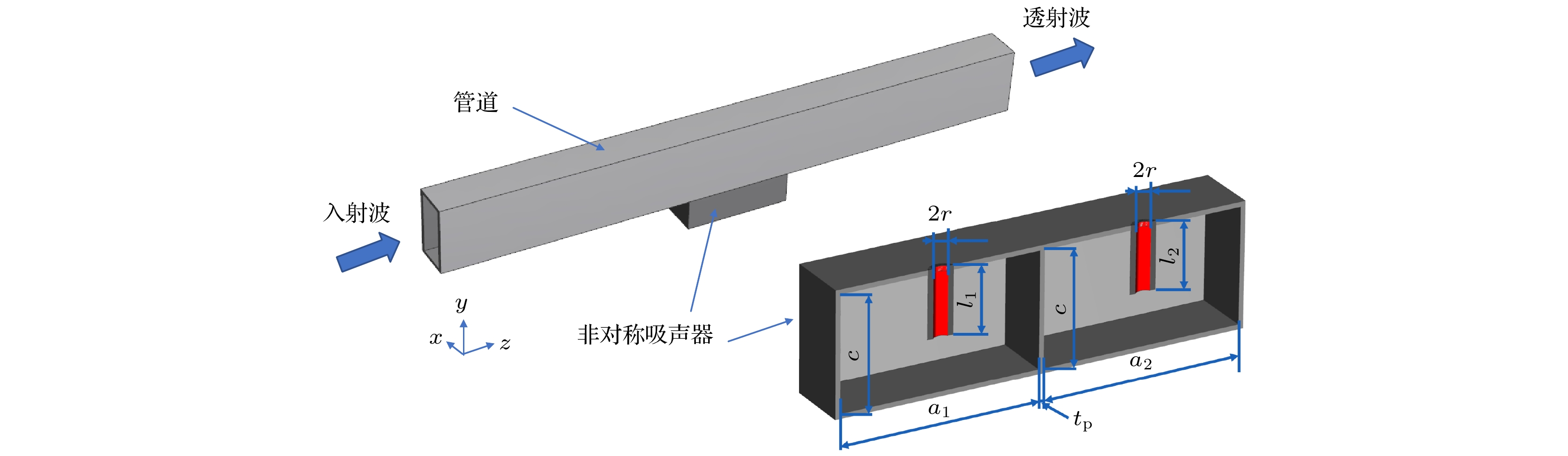
-
为了解决发动机低频噪声问题, 基于双端口非对称吸声器原理, 设计了一种尺寸渐变的吸声超表面, 用于发动机声衬降噪设计. 首先, 建立了非对称共振吸声器的理论分析模型和仿真分析模型, 揭示了降噪机理, 并分析了其降噪效果的影响因素. 然后基于非对称共振吸声器设计了一种声学超表面声衬, 用全模型理论计算、等效阻抗理论计算和COMSOL有限元仿真三种方法深入分析了声衬的降噪效果, 并用全模型理论计算和等效阻抗理论计算方法考虑了流速对降噪效果的影响, 然后对此结构进行了参数优化. 研究结果表明, 所设计的基于非对称吸声器的声学超表面声衬在厚度仅为2.5 cm (仅为252 Hz对应波长的1/54)的情况下, 可实现252—692 Hz的频带范围内3 dB以上的降噪效果, 为发动机降噪设计提供了一种新的设计思路.In order to solve the problem of low frequency noise of engine, based on the principle of dual port asymmetric sound absorber, a kind of gradually changing size sound absorbing metasurface is designed to reduce the noise of engine acoustic liner. Firstly, the theoretical analysis model and simulation analysis model of the asymmetric resonance sound absorber are established, the noise reduction mechanism is revealed, and the influencing factors of the noise reduction effect are analyzed. Then an acoustic metasurface acoustic liner is designed based on the asymmetric resonance sound absorber. The noise reduction effect of the acoustic liner is analyzed in depth by using three methods: full model theoretical calculation, equivalent impedance theoretical calculation and COMSOL finite element simulation. Then, the parameters of this structure are optimized, and the influence of flow velocity on the noise reduction effect is considered by using the full model theoretical calculation and equivalent impedance theoretical calculation. The research results show that the acoustic metasurface acoustic liner designed based on asymmetric sound absorber can achieve noise reduction effect of more than 3 dB in a frequency band range from 252 to 692 Hz when the thickness is only 2.5 cm (only 1/54 of the corresponding wavelength of 252 Hz), which provides a new idea for designing engine noise reduction.
-
Keywords:
- asymmetric /
- metasurface /
- engine /
- acoustic liner /
- flow velocity
[1] Kaltenbach M, Maschke C, Heb F, Niemann H, Führ M 2016 Int. J. Environ. Prot. 6 15
[2] Čavka I, Čokorilo O, Vasov L 2016 Energy Build. 115 63
Google Scholar
[3] 乔渭阳, 许开富, 武兆伟, 黄文超, 秦浩明 2008 航空学报 29 534
Google Scholar
Qiao W Y, Xu K F, Wu Z W, Huang W C, Qin H M 2008 Acta Aeronaut. Astronaut. Sin. 29 534
Google Scholar
[4] 陈俊, 周驯黄, 徐珺, 刘常春, 许尧 2019 推进技术 40 1498
Chen J, Zhou X H, Xu J, Liu C C, Xu Y 2019 Promotion Technol. 40 1498
[5] 陈超, 闫照华, 李晓东 2018 航空动力学报 33 3041
Chen C, Yan Z H, Li X D 2018 J. Aerospace Power 33 3041
[6] 霍施宇, 杨嘉丰, 邓云华, 燕群 2022 航空学报 43 519
Huo S Y, Yang J F, Deng Y H, Yan Q 2022 Acta Aeronaut. Astronaut. Sin. 43 519
[7] Zhao D, Ji C, Li J W, Ang L 2018 Appl. Acoust. 141 281
Google Scholar
[8] Jha N, Das D, Tripathi A, Hota R 2019 Appl. Acoust. 150 179
Google Scholar
[9] Dannemann M, Kucher M, Kunze E, Modler N, Knobloch K, Enghardt L, Sarradj E, Höschler K 2018 Appl. Sci. 8 1923
Google Scholar
[10] Knobloch K, Enghardt L, Bake F 2018 24th AIAA/CEAS Aeroacoustics Conference Atlanta, Georgia, June 25–29, 2018 p4102
[11] Simon F 2018 J. Sound and Vibr. 421 1
Google Scholar
[12] Sandu C, Humbert T, Auregan Y, Deaconu M, Totu A, Radu A, Serbescu H, Tipa T 2022 IOP Conference Series: Materials Science and Engineering 1226 012049
Google Scholar
[13] Huang S B, Fang X S, Wang X, Assouar B, Cheng Q, Li Y 2019 J. Acoust. Soc. Am. 145 254
Google Scholar
[14] 黄思博, 周志凌, 李东庭, 刘拓, 王旭, 祝捷, 李勇 2020 科学通报 65 373
Google Scholar
Huang S B, Zhou Z L, Li D T, Liu T, Wang X, Zhu J, Li Y 2020 Sc. Bull. 65 373
Google Scholar
[15] Huang S B, Zhou E M, Huang Z L, Lei P F, Zhou Z L, Li Y 2021 Appl. Phys. Lett. 118 063504
Google Scholar
[16] Ding H, Wang N Y, Qiu S, Huang S B, Zhou Z L, Zhou C C, Jia B, Li Y 2022 Int. J. Mech. Sci. 232 107601
Google Scholar
[17] Cheng B Z, Gao N S, Huang Y K, Hou H 2022 J. Vib. Control 28 410
Google Scholar
[18] Yang X C, Yang F, Shen X M, Wang E S, Zhang X N, Shen C, Peng W Q 2022 Materials 15 5938
Google Scholar
[19] Wang E S, Yang F, Shen X M, Duan H Q, Zhang X N, Yin Q, Peng W Q, Yang X C, Yang L 2022 Materials 15 3417
Google Scholar
[20] Beck B S, Schiller N H, Jones M G 2015 Appl. Acoust. 93 15
Google Scholar
[21] Guo J W, Fang Y, Jiang Z Y, Zhang X 2021 J. Acoust. Soc. Am. 149 70
Google Scholar
[22] Oh T S, Jeon W 2022 Appl. Phys. Lett. 120 044103
Google Scholar
[23] Jiménez N, Romero-García V, Pagneux V, Groby J P 2017 Sci. Rep. 7 1
Google Scholar
[24] Long H Y, Cheng Y, Liu X J 2017 Appl. Phys. Lett. 111 143502
Google Scholar
[25] Long H Y, Liu C, Shao C, Cheng Y, Tao J C, Qiu X J, Liu X J 2020 J. Sound Vib. 479 115371
Google Scholar
[26] Long H Y, Shao C, Cheng Y, Tao J C, Liu X J 2021 Appl. Phys. Lett. 118 263502
Google Scholar
[27] Seo S H, Kim Y H, Kim K J 2018 Appl. Acoust. 138 188
Google Scholar
[28] Guess A 1975 J. Sound Vib. 40 119
Google Scholar
[29] Kooi J, Sarin S 1981 7th Aeroacoustics Conference Palo Alto, California, October 5–7, 1981 p1998
-
图 2 声波正向入射 (a)和反向入射 (b)不同情况下, 吸声器的吸声系数A和反射系数R及在770 Hz处的声压变化
Fig. 2. The sound absorption coefficient A and reflection coefficient R of the sound absorber and the change of sound pressure at 770 Hz under different conditions of normal (a) and reverse (b) incidence of sound waves.
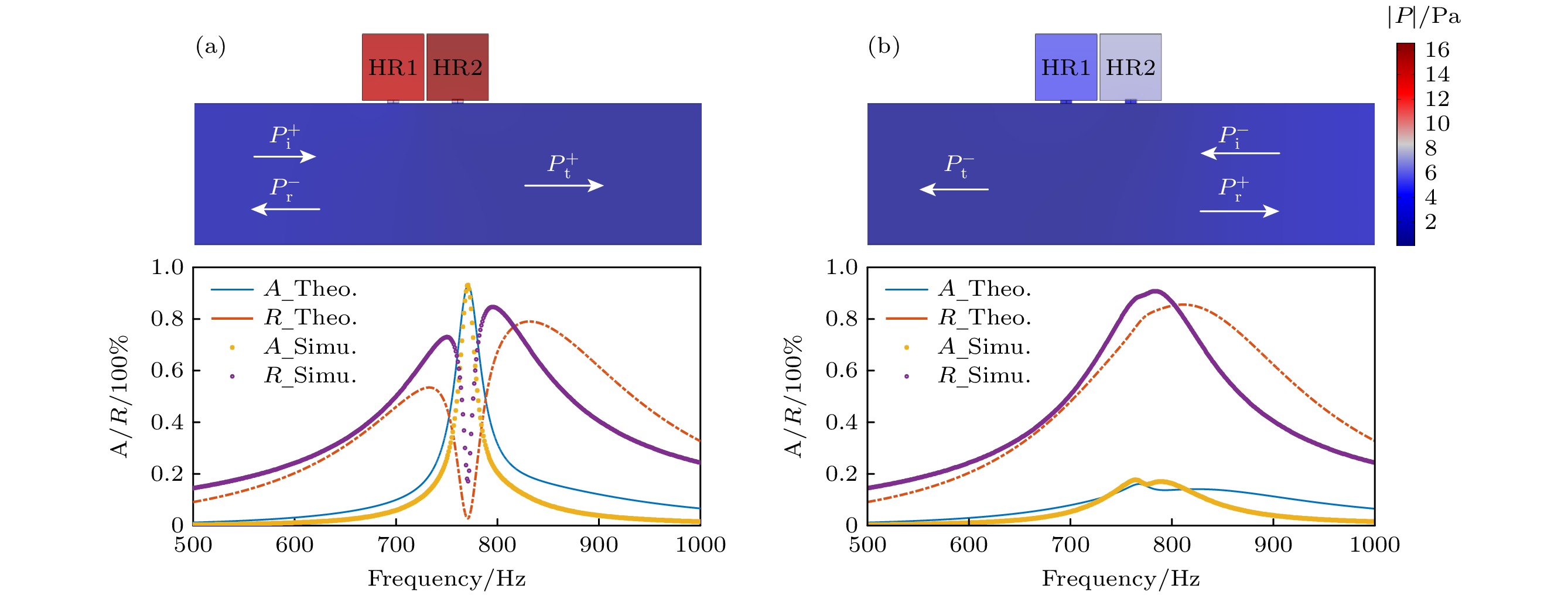
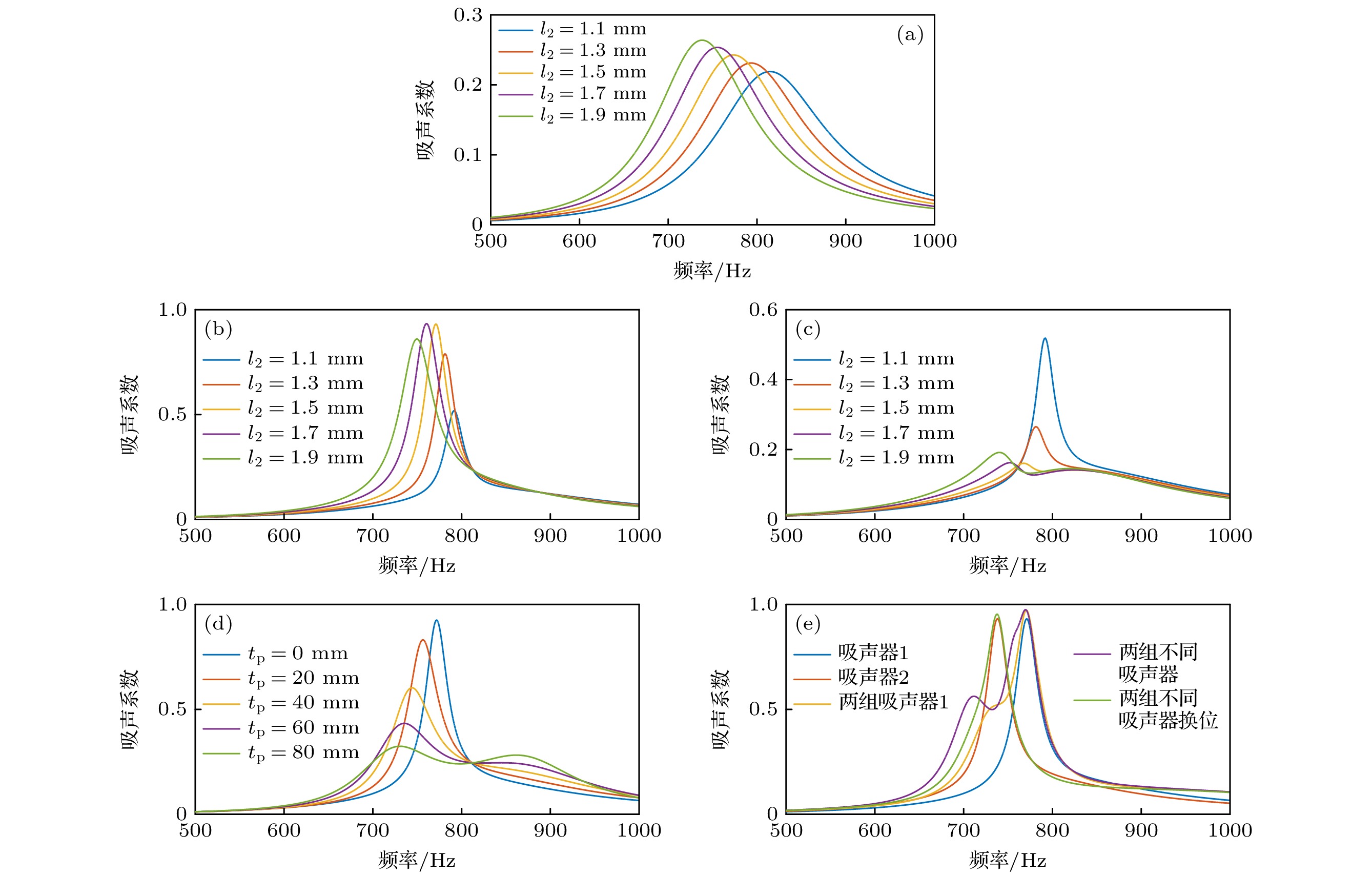
图 3 吸声系数和频率的关系 (a) HR2的内插管长度变化; (b) HR1与内插管长度变化的HR2耦合; (c) 声波反向入射时, HR1与内插管长度变化的HR2耦合; (d) HR1和HR2之间间距变化; (e) 两组吸声器
Fig. 3. The relationship between sound absorption coefficient and frequency: (a) The length change of HR2 endotracheal tube; (b) HR1is coupled with HR2 with varying length of endotracheal tube; (c) HR1 is coupled to HR2 with the change of the length of the endotracheal tube when the acoustic wave is incident in the reverse direction; (d) change in spacing between HR1 and HR2; (e) two sets of sound absorbers.
图 4 (a) 全尺寸建模; (b) 三维等效阻抗建模
Fig. 4. (a) Full scale modeling; (b) three dimensional equivalent impedance modeling.
图 5 用理论、仿真和等效阻抗计算得出的(a)吸声系数和(b)传递损失
Fig. 5. (a) Sound absorption coefficient and (b) transmission loss calculated by substituting theory, simulation and equivalent impedance.
图 6 (a) 二维对称等效阻抗建模; 400 Hz频率处(b)无声衬等效阻抗时和(c) 有声衬等效阻抗时声压变化
Fig. 6. (a) Two dimensional symmetrical equivalent impedance modeling. Sound pressure change at 400 Hz frequency (b) when there is no acoustic liner equivalent impedance and (c) when there is acoustic liner equivalent impedance.
图 7 参数优化后理论计算得到的(a)吸声系数和(b)传递损失
Fig. 7. (a) Sound absorption coefficient and (b) transmission loss calculated theoretically after parameter optimization.
图 8 马赫数为0, 0.1, 0.2时, 声衬的(a)吸声系数和(b)传递损失
Fig. 8. When Mach number is 0, 0.1, 0.2, (a) sound absorption coefficient and (b) transmission loss of sound liner.
表 1 共振器的尺寸
Table 1. Size of resonator.
l/mm a/mm l/mm a/mm l/mm a/mm 1 1.1 22 12 4.4 27 23 10.8 31 2 1.5 22 13 5.5 26 24 11.2 33 3 1.1 24 14 6.0 28 25 13.8 34 4 1.5 24 15 7.1 26 26 14.2 36 5 1.9 24 16 7.6 28 27 13.8 34 6 2.3 24 17 8.5 28 28 14.2 36 7 1.8 28 18 9.0 28 29 17.0 40 8 2.3 28 19 8.5 28 30 19.7 40 9 2.8 28 20 9.0 28 31 17.0 40 10 3.1 28 21 10.8 31 32 19.7 40 11 4.4 25 22 11.2 33 33 23 42 34 23 42 
下载: 导出CSV
表 2 每一个非对称吸声器对应的耦合频率
Table 2. Coupling frequency corresponding to each pair of asymmetric sound absorbers.
非对称
共振
吸声器fcr 非对称
共振
吸声器fcr 非对称
共振
吸声器fcr 1 770 7 490 13 300 2 740 8 450 14 300 3 690 9 400 15 250 4 640 10 400 16 250 5 590 11 350 17 210 6 530 12 350
下载: 导出CSV
表 3 共振器的尺寸
Table 3. Size of resonator.
l/mm a/mm l/mm a/mm l/mm a/mm 1 2.4 22 12 6 27 23 14 31 2 2.5 22 13 7.5 26 24 13.5 33 3 2.7 24 14 7 28 25 14.8 34 4 2.8 24 15 9 26 26 14.5 36 5 3.5 24 16 8.5 28 27 17.5 34 6 3.6 24 17 9.7 28 28 17 36 7 3.45 28 18 10.2 28 29 17.1 40 8 3.65 28 19 11.5 28 30 17.6 40 9 4.45 28 20 12 28 31 20 40 10 4.65 28 21 12 31 32 21 40 11 6.6 25 22 11.5 33 33 23 41 34 23 42
下载: 导出CSV
-
[1] Kaltenbach M, Maschke C, Heb F, Niemann H, Führ M 2016 Int. J. Environ. Prot. 6 15
[2] Čavka I, Čokorilo O, Vasov L 2016 Energy Build. 115 63
Google Scholar
[3] 乔渭阳, 许开富, 武兆伟, 黄文超, 秦浩明 2008 航空学报 29 534
Google Scholar
Qiao W Y, Xu K F, Wu Z W, Huang W C, Qin H M 2008 Acta Aeronaut. Astronaut. Sin. 29 534
Google Scholar
[4] 陈俊, 周驯黄, 徐珺, 刘常春, 许尧 2019 推进技术 40 1498
Chen J, Zhou X H, Xu J, Liu C C, Xu Y 2019 Promotion Technol. 40 1498
[5] 陈超, 闫照华, 李晓东 2018 航空动力学报 33 3041
Chen C, Yan Z H, Li X D 2018 J. Aerospace Power 33 3041
[6] 霍施宇, 杨嘉丰, 邓云华, 燕群 2022 航空学报 43 519
Huo S Y, Yang J F, Deng Y H, Yan Q 2022 Acta Aeronaut. Astronaut. Sin. 43 519
[7] Zhao D, Ji C, Li J W, Ang L 2018 Appl. Acoust. 141 281
Google Scholar
[8] Jha N, Das D, Tripathi A, Hota R 2019 Appl. Acoust. 150 179
Google Scholar
[9] Dannemann M, Kucher M, Kunze E, Modler N, Knobloch K, Enghardt L, Sarradj E, Höschler K 2018 Appl. Sci. 8 1923
Google Scholar
[10] Knobloch K, Enghardt L, Bake F 2018 24th AIAA/CEAS Aeroacoustics Conference Atlanta, Georgia, June 25–29, 2018 p4102
[11] Simon F 2018 J. Sound and Vibr. 421 1
Google Scholar
[12] Sandu C, Humbert T, Auregan Y, Deaconu M, Totu A, Radu A, Serbescu H, Tipa T 2022 IOP Conference Series: Materials Science and Engineering 1226 012049
Google Scholar
[13] Huang S B, Fang X S, Wang X, Assouar B, Cheng Q, Li Y 2019 J. Acoust. Soc. Am. 145 254
Google Scholar
[14] 黄思博, 周志凌, 李东庭, 刘拓, 王旭, 祝捷, 李勇 2020 科学通报 65 373
Google Scholar
Huang S B, Zhou Z L, Li D T, Liu T, Wang X, Zhu J, Li Y 2020 Sc. Bull. 65 373
Google Scholar
[15] Huang S B, Zhou E M, Huang Z L, Lei P F, Zhou Z L, Li Y 2021 Appl. Phys. Lett. 118 063504
Google Scholar
[16] Ding H, Wang N Y, Qiu S, Huang S B, Zhou Z L, Zhou C C, Jia B, Li Y 2022 Int. J. Mech. Sci. 232 107601
Google Scholar
[17] Cheng B Z, Gao N S, Huang Y K, Hou H 2022 J. Vib. Control 28 410
Google Scholar
[18] Yang X C, Yang F, Shen X M, Wang E S, Zhang X N, Shen C, Peng W Q 2022 Materials 15 5938
Google Scholar
[19] Wang E S, Yang F, Shen X M, Duan H Q, Zhang X N, Yin Q, Peng W Q, Yang X C, Yang L 2022 Materials 15 3417
Google Scholar
[20] Beck B S, Schiller N H, Jones M G 2015 Appl. Acoust. 93 15
Google Scholar
[21] Guo J W, Fang Y, Jiang Z Y, Zhang X 2021 J. Acoust. Soc. Am. 149 70
Google Scholar
[22] Oh T S, Jeon W 2022 Appl. Phys. Lett. 120 044103
Google Scholar
[23] Jiménez N, Romero-García V, Pagneux V, Groby J P 2017 Sci. Rep. 7 1
Google Scholar
[24] Long H Y, Cheng Y, Liu X J 2017 Appl. Phys. Lett. 111 143502
Google Scholar
[25] Long H Y, Liu C, Shao C, Cheng Y, Tao J C, Qiu X J, Liu X J 2020 J. Sound Vib. 479 115371
Google Scholar
[26] Long H Y, Shao C, Cheng Y, Tao J C, Liu X J 2021 Appl. Phys. Lett. 118 263502
Google Scholar
[27] Seo S H, Kim Y H, Kim K J 2018 Appl. Acoust. 138 188
Google Scholar
[28] Guess A 1975 J. Sound Vib. 40 119
Google Scholar
[29] Kooi J, Sarin S 1981 7th Aeroacoustics Conference Palo Alto, California, October 5–7, 1981 p1998
下载:
计量
- 文章访问数: 4526
- PDF下载量: 111
- 被引次数: 0
