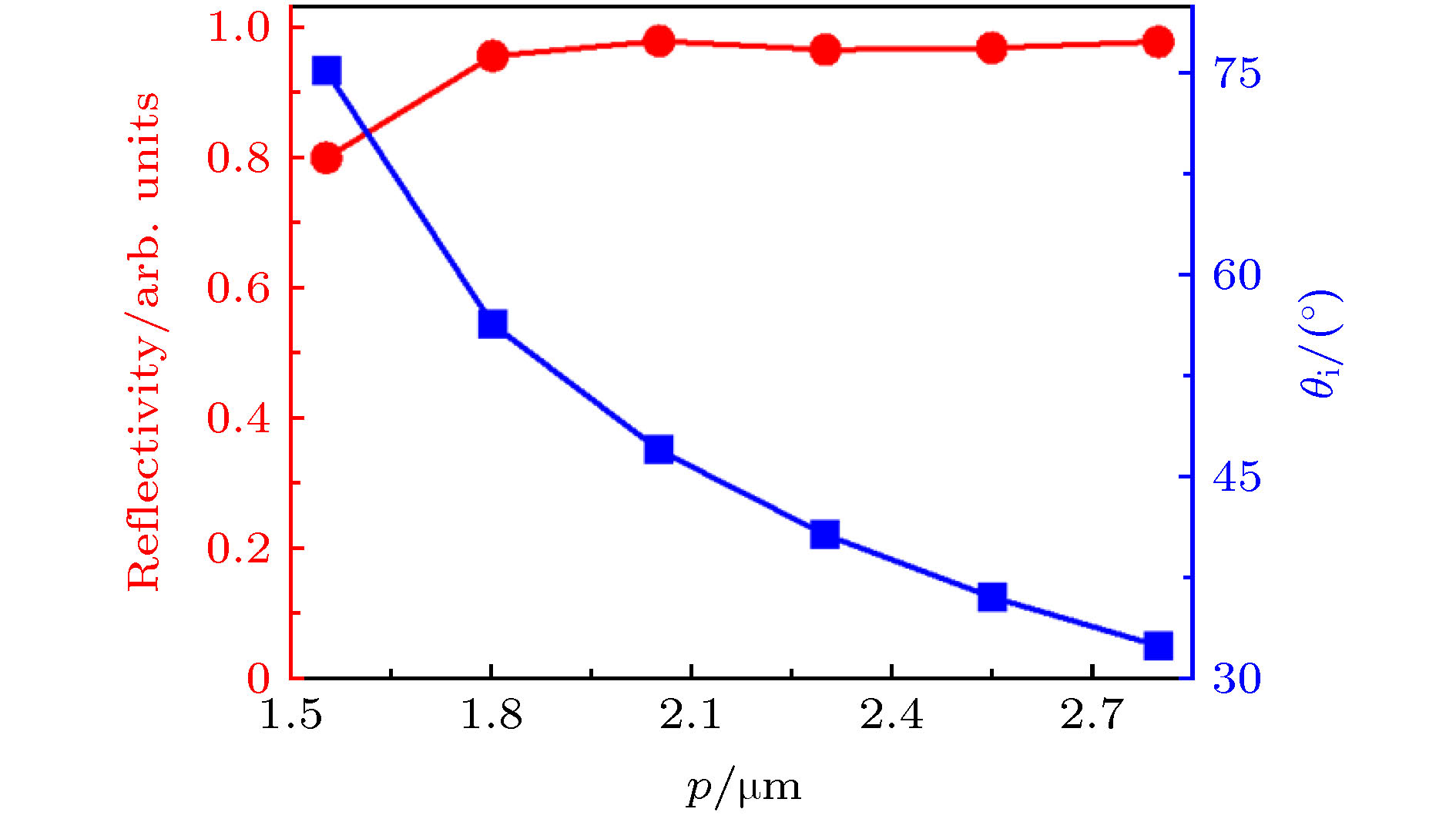
-
How to effectively control the refraction, reflection, propagation and wavefront of dynamic waves or light has become one of hot research points in the field of optics. In the past few years, the concept of phase gradient metasurface has been proposed: it introduces a gradient of the phase discontinuity covering the entire angle 2π along the interface to provide an effective wave vector $\kappa $ and completely control the direction of outing wave. Therefore, the metasurface can possess many novel optical applications, such as holograms, metalenses, photonic spin Hall effect, etc. In this work, we design a simplified reflection-type optical metagrating. The results demonstrate that the metagrating can achive the function of two-channel retroreflection, that is, redirecting the incident wave back toward the source, with a nearly perfect conversion efficiency.The metagrating designed in this paper contains only two sub-cells with π reflection phase difference in period. The working wavelength (λ) of metagrating is fixed at 3 μm. The two sub-cells are filled with an impedance matching material (their material relative refractive indexes are n1 = 1 and n2 = 1.5 respectively and their thickness is d = 1.5 μm.).The period length range is 1.5 μm ≤ p ≤ 3 μm(considering reducing the reflection order). When the incident angle is ${\theta _{\rm{i}}}= \pm \arcsin [\lambda /(2p)]$ , the absolute values of the incident angle and the reflected angle are equal, and then retroreflection occurs. When the wavelength is greater than the period ($\lambda \geqslant p$ ), the angle of retroreflection can be adjusted to any value ($\left| {{\theta _{\rm{i}}}} \right| \geqslant {\rm{3}}{{\rm{0}}^ \circ }$ ) by adjusting the period p. In this work, COMSOL MULTIPHYSICS software is used to simulate the retroreflection reflectivity and field pattern of the designed metagrating. The results verify the two-channel retroreflection property of the metagrating. In addition,as the angle of incidence changes from 30° to 60°, the efficiency of retroreflection at any incident angle can reach to more than 95%. When the incident angle is 75.4°, the metagrating still has an efficiency of 80% retroreflection. Therefore, the metagrating also achieves the function of high-efficiency retroreflection at a large-angle. Comparing with multiple sub-cells’ metasurface, the simplified metagrating with two sub-cells enables a similar function of retroreflection, but has many potential advantages, and can play an important role in high-efficiency sensing, imaging and communication.-
Keywords:
- subwavelength metallic metagrating /
- metasurface /
- retroreflection /
- large-angle
[1] Yu N, Genevet P, Kats M A, Aieta F, Tetienne J P, Capasso F, Gaburro Z 2011 Science 334 333
Google Scholar
[2] Kildishev A V, Boltasseva A, Shalaev V M 2013 Science 339 1232009
Google Scholar
[3] Yu N, Capasso F 2014 Nat. Mater. 13 139
Google Scholar
[4] Zhao Y, Liu X, Alù A 2014 J. Opt. 16 123001
Google Scholar
[5] Xu Y, Fu Y, Chen H 2016 Nat. Rev. Mater. 1 16067
Google Scholar
[6] Shi H Y, Zhang A X, Chen J Z, Wang J F, Xia S, Xu Z 2016 Chin. Phys. B 25 078105
Google Scholar
[7] Zhao J, Yang X, Dai J Y, Cheng Q, Li X, Qi N H, Ke J C, Bai G D, Liu S, Jin S, Alù A, Cui T J 2018 Natl. Sci. Rev. 6 231
[8] Xu Y, Gu C, Hou B, Lai Y, Li J, Chen H 2013 Nat. Commun. 4 2561
Google Scholar
[9] Ni X, Ishii S, Kildishev A V, Shalaev V M 2013 Light Sci. Appl 2 e72
[10] Yin X, Ye Z, Rho J, Wang Y, Zhang X 2013 Science 339 1405
Google Scholar
[11] Xu Y, Fu Y, Chen H 2015 Sci. Rep. 5 12219
Google Scholar
[12] Ra’di Y, Sounas D L, Alù A 2017 Phys. Rev. Lett. 119 067404
Google Scholar
[13] Chalabi H, Ra’di Y, Sounas D L, Alù A 2017 Phys. Rev. B 96 075432
Google Scholar
[14] Fu Y, Cao Y, Xu Y 2019 Appl. Phys. Lett. 114 053502
Google Scholar
[15] Qian E, Fu Y, Xu Y, Chen H 2016 Europhys. Lett. 114 34003
Google Scholar
[16] Ra’di Y, Alù A 2018 ACS Photonics 5 1779
Google Scholar
[17] Fu Y, Shen C, Cao Y, Gao L, Chen H, Chan C T, Cummer S A, Xu Y 2019 Nat. Commun. 10 2326
Google Scholar
[18] Ma Y, Ong C K, Tyc T, Leonhardt U 2009 Nat. Mater. 8 639
Google Scholar
[19] Jia Y, Wang J, Li Y, Pang Y, Yang J, Fan Y, Qu S 2017 AIP Adv. 7 105315
Google Scholar
[20] Yan L, Zhu W, Karim M F, Cai H, Gu A Y, Shen Z, Chong P H J, Kwong D L, Qiu C W, Liu A Q 2018 Adv. Mater. 30 e1802721
Google Scholar
[21] Lipuma D, Meric S, Gillard R 2013 Electron. Lett. 49 2
[22] Zentgraf T, Liu Y, Mikkelsen M H, Valentine J, Zhang X 2011 Nat. Nanotechnol. 6 151
Google Scholar
[23] Nakagawa K, Sanada A 2017 ICMIM 978-1-5090-4354-5
[24] Jiang W X, Bao D, Cui T J 2016 J. Opt. 18 044022
Google Scholar
[25] Fu Y, Li J, Xie Y, Shen C, Xu Y, Chen H, Cummer S A 2018 Phys. Rev. Mater. 2 105202
Google Scholar
[26] Song G, Cheng Q, Cui T J, Jing Y 2018 Phys. Rev. Mater. 2 065201
Google Scholar
[27] Shen C, Díaz-Rubio A, Li J, Cummer S A 2018 Appl. Phys. Lett. 112 183503
Google Scholar
[28] Shen C, Cummer S A 2018 Phys. Rev. Appl. 9 054009
Google Scholar
[29] Cao Y, Fu Y, Zhou Q, Ou X, Gao L, Chen H, Xu Y 2019 Phys. Rev. Appl. 12 024006
Google Scholar
[30] Zhang Z, Chu F, Guo Z, Fan J, Li G, Cheng W 2019 J. Lightwave Technol. 37 2820
Google Scholar
-
图 1 超构光栅的结构示意图 (a)逆向反射超构光栅的示意图, 其中红色和绿色箭头均表示回射, 蓝色箭头表示镜面反射; (b)超构光栅的结构单元示意图; (c)超构光栅入射和反射的等频图
Fig. 1. The structute of the metagrating: (a) The schematic of the retroreflection metagrating, wherein red and green arrows indicate retroreflection and blue arrows indicate specular reflection; (b) the diagram of metagrating with two sub-cells; (c) the iso-frequency contours of the incident wave and reflection wave for the metagrating.
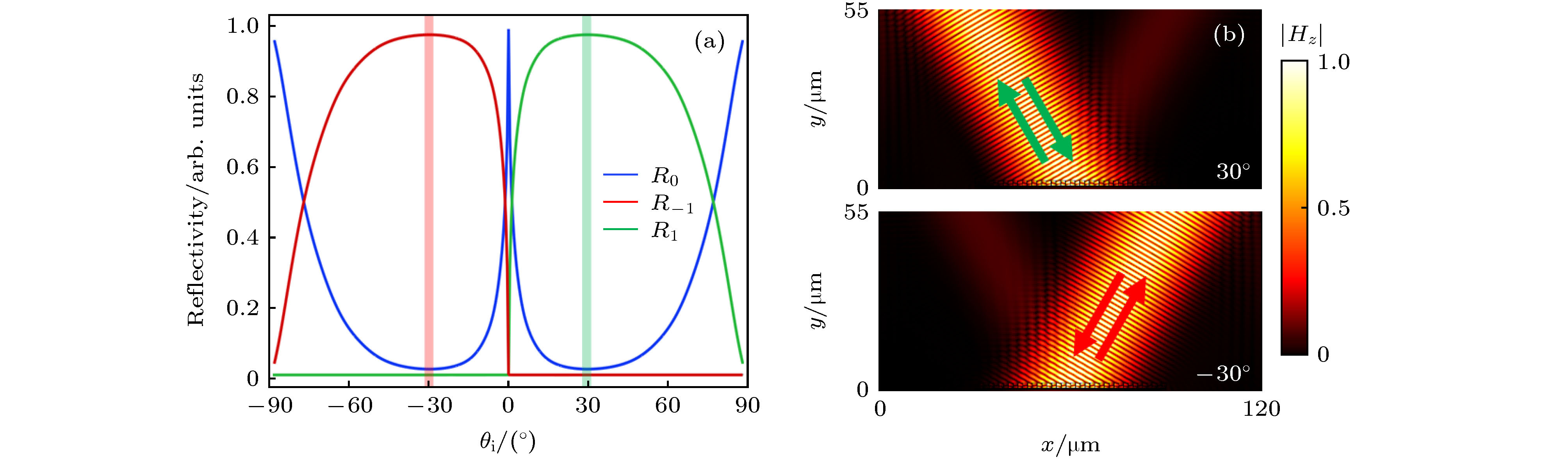
图 2 设计的回射角为
$ \pm {\rm{3}}{{\rm{0}}^ \circ }$ 时, 超构光栅的不同级次的反射效率以及高斯波入射到超构光栅的总磁场图 (a)超构光栅不同级次的反射效率随入射角度变化曲线; (b)高斯波入射到超构光栅, 双通道回射的总磁场图Fig. 2. The reflection efficiency of different orders and the total magnetic field pattern, while the designed retroreflection angle is
$ \pm {\rm{3}}{{\rm{0}}^ \circ }$ : (a) The reflection efficiency of different orders vary with incident angle; (b) the total magnetic field pattern of the two-channel retroreflector.
图 3 设计的回射角为
$ \pm {\rm{6}}{{\rm{0}}^ \circ }$ 时, 超构光栅的不同级次的反射效率以及高斯波入射到超构光栅的总磁场图 (a)超构光栅不同级次的反射效率随入射角度变化曲线; (b)高斯波入射到超构光栅, 双通道回射的总磁场图Fig. 3. The reflection efficiency of different orders and the total magnetic field pattern, while the designed retroreflection angle is
$ \pm 6{{\rm{0}}^ \circ }$ : (a) The reflection efficiency of different orders vary with incident angle; (b) the total magnetic field pattern of the two-channel retroreflector.
图 4 逆向反射的效率和工作角度随周期长度的变化规律. 随
$p$ 改变过程中, 金属槽的占空比和填充介质保持不变Fig. 4. The incident angle of retroreflection and retroreflectivity corresponding to different period lengths
$p$ . With the change of$p$ , the duty cycle and filling medium of the metal slot remain unchanged. -
[1] Yu N, Genevet P, Kats M A, Aieta F, Tetienne J P, Capasso F, Gaburro Z 2011 Science 334 333
Google Scholar
[2] Kildishev A V, Boltasseva A, Shalaev V M 2013 Science 339 1232009
Google Scholar
[3] Yu N, Capasso F 2014 Nat. Mater. 13 139
Google Scholar
[4] Zhao Y, Liu X, Alù A 2014 J. Opt. 16 123001
Google Scholar
[5] Xu Y, Fu Y, Chen H 2016 Nat. Rev. Mater. 1 16067
Google Scholar
[6] Shi H Y, Zhang A X, Chen J Z, Wang J F, Xia S, Xu Z 2016 Chin. Phys. B 25 078105
Google Scholar
[7] Zhao J, Yang X, Dai J Y, Cheng Q, Li X, Qi N H, Ke J C, Bai G D, Liu S, Jin S, Alù A, Cui T J 2018 Natl. Sci. Rev. 6 231
[8] Xu Y, Gu C, Hou B, Lai Y, Li J, Chen H 2013 Nat. Commun. 4 2561
Google Scholar
[9] Ni X, Ishii S, Kildishev A V, Shalaev V M 2013 Light Sci. Appl 2 e72
[10] Yin X, Ye Z, Rho J, Wang Y, Zhang X 2013 Science 339 1405
Google Scholar
[11] Xu Y, Fu Y, Chen H 2015 Sci. Rep. 5 12219
Google Scholar
[12] Ra’di Y, Sounas D L, Alù A 2017 Phys. Rev. Lett. 119 067404
Google Scholar
[13] Chalabi H, Ra’di Y, Sounas D L, Alù A 2017 Phys. Rev. B 96 075432
Google Scholar
[14] Fu Y, Cao Y, Xu Y 2019 Appl. Phys. Lett. 114 053502
Google Scholar
[15] Qian E, Fu Y, Xu Y, Chen H 2016 Europhys. Lett. 114 34003
Google Scholar
[16] Ra’di Y, Alù A 2018 ACS Photonics 5 1779
Google Scholar
[17] Fu Y, Shen C, Cao Y, Gao L, Chen H, Chan C T, Cummer S A, Xu Y 2019 Nat. Commun. 10 2326
Google Scholar
[18] Ma Y, Ong C K, Tyc T, Leonhardt U 2009 Nat. Mater. 8 639
Google Scholar
[19] Jia Y, Wang J, Li Y, Pang Y, Yang J, Fan Y, Qu S 2017 AIP Adv. 7 105315
Google Scholar
[20] Yan L, Zhu W, Karim M F, Cai H, Gu A Y, Shen Z, Chong P H J, Kwong D L, Qiu C W, Liu A Q 2018 Adv. Mater. 30 e1802721
Google Scholar
[21] Lipuma D, Meric S, Gillard R 2013 Electron. Lett. 49 2
[22] Zentgraf T, Liu Y, Mikkelsen M H, Valentine J, Zhang X 2011 Nat. Nanotechnol. 6 151
Google Scholar
[23] Nakagawa K, Sanada A 2017 ICMIM 978-1-5090-4354-5
[24] Jiang W X, Bao D, Cui T J 2016 J. Opt. 18 044022
Google Scholar
[25] Fu Y, Li J, Xie Y, Shen C, Xu Y, Chen H, Cummer S A 2018 Phys. Rev. Mater. 2 105202
Google Scholar
[26] Song G, Cheng Q, Cui T J, Jing Y 2018 Phys. Rev. Mater. 2 065201
Google Scholar
[27] Shen C, Díaz-Rubio A, Li J, Cummer S A 2018 Appl. Phys. Lett. 112 183503
Google Scholar
[28] Shen C, Cummer S A 2018 Phys. Rev. Appl. 9 054009
Google Scholar
[29] Cao Y, Fu Y, Zhou Q, Ou X, Gao L, Chen H, Xu Y 2019 Phys. Rev. Appl. 12 024006
Google Scholar
[30] Zhang Z, Chu F, Guo Z, Fan J, Li G, Cheng W 2019 J. Lightwave Technol. 37 2820
Google Scholar
下载:
计量
- 文章访问数: 12195
- PDF下载量: 218
- 被引次数: 0
