-
How to effectively control the refraction, reflection, propagation and wavefront of electromagnetic wave or light is always one of the advanced researches in the field of optics. In recent years, much effort has been devoted to both theoretical and experimental studies of optical phase gradient metagratings (PGMs) due to the fundamental interest and practical importance of PGMs, such as the generalized Snell’s law (GSL). Typically, the PGMs are constructed as periodic gratings consisting of a supercell spatially repeated along an interface, and each supercell consists of m unit cells, with m being an integer. The key idea of PGMs is to introduce an abrupt phase shift covering the range from 0 to $2\pi $ discretely through m unit cells to ensure the complete control of the outgoing waves. The phase gradient provides a new degree of freedom for the manipulation of light propagation, which has allowed a series of ultrathin devices to realize anomalous scattering, the photon spin Hall effect, and many other phenomena.Intuitively, the number of unit cells m in a supercell does not influence the PGM diffraction characteristics, except that a small value of m will lead to a reduced diffraction efficiency. However, some recent studies have shown that the integer m plays a fundamental role in determining the high-order PGM diffractions when the incident angle is beyond the critical angle predicted by the GSL. In particular, for high-order PGM diffractions, m leads to a new set of diffraction equations expressed as $ \left\{ {\begin{aligned} &{{k_x} = k_x^t - nG,{\text{ for odd L,}}} \\ &{{k_x} = k_x^r - nG,{\text{ for even L}}{\text{. }}} \end{aligned}} \right. $ In addition to the phase gradient, the integer number of unit cells m in a supercell is another degree of freedom that can be employed to control the light propagation. By the parity of m, the higher-order outgoing wave can be reversed between the anomalous transmission channel and the anomalous reflection channel. In this work, according to the concept of abrupt phase and the parity-dependent diffraction law in phase gradient metagrating, we theoretically design and study an optical meta-cage. The meta-cage is a periodic structure with one period that contains m different unit cells. Through numerical simulations and rigorous analytical calculations, we find that the ability of meta-cage to trap light is related to the parity of the number of unit cells m in a supercell. Specifically, when the number of unit cells is odd, the point source placed in the meta-cage can perfectly radiate out of the meta-cage without any reflection. On the contrary, when the number of unit cells is even, the point source can hardly radiate out of the meta-cage, and all the energy is localized within the meta-cage. Moreover, such a phenomenon is robust against the disorder. These results can provide new ideas and theoretical guidance for designing new radar radome and photonic isolation devices. -
Keywords:
- abrupt phase shifts /
- phase gradient metagratings /
- meta-cage /
- parity-dependent radiation
[1] Yu N, Genevet P, Kats M A, Aieta F, Tetienne J P, Capasso F, Gaburro Z 2011 Science 334 333
Google Scholar
[2] Kildishev A V, Boltasseva A, Shalaev V M 2013 Science 339 1232009
Google Scholar
[3] Yu N, Capasso F 2014 Nat. Mater. 13 139
Google Scholar
[4] Zhao Y, Liu X, Alù A 2014 J. Opt. 16 123001
Google Scholar
[5] Xu Y, Fu Y, Chen H 2016 Nat. Rev. Mater. 1 16067
Google Scholar
[6] Shi H Y, Zhang A X, Chen J Z, Wang J F, Xia S, Xu Z 2016 Chin. Phys. B 25 078105
Google Scholar
[7] Zhao J, Yang X, Dai J Y, et al. 2018 Natl. Sci. Rev. 6 231
[8] Pendry J B 2003 Opt. Express 11 755
Google Scholar
[9] Fang N, Lee H, Sun C, Zhang X 2005 Science 308 534
Google Scholar
[10] Smolyaninov I I, Hung Y J, Davis C C 2007 Science 315 1699
Google Scholar
[11] Liu Z, Lee H, Xiong Y, Sun C, Zhang X 2007 Science 315 1686
Google Scholar
[12] Rho J, Zi L Y, Yi X, Xiao B Y, Zhao W L, Hyeunseok C, Guy B, Xiang Z 2010 Nat. Commun. 1 143
Google Scholar
[13] Lu D, Liu Z 2012 Nat. Commun. 3 1205
Google Scholar
[14] Enoch S, Tayeb G, Sabouroux P, Guerin N, Vincent P 2002 Phys. Rev. Lett. 89 213902
Google Scholar
[15] Yuan Y, Lin F S, Li X R, Tao J, Jiang T, Huang F, Jin A K 2008 Phys. Rev. A 77 053821
Google Scholar
[16] Cheng Q, Jiang W X, Cui T J 2011 Appl. Phys. Lett. 99 131913
Google Scholar
[17] Liu X, Starr T, Starr A F, Padilla W J 2010 Phys. Rev. Lett. 104 207403
Google Scholar
[18] Chen H, Jiang F Z, John F O'Hara, Frank C, Abul K A, Antoinette J T 2010 Phys. Rev. Lett. 105 073901
Google Scholar
[19] Shen X, Tie J C, Jun M Z, Hui F M, Wei X J, Hui L 2011 Opt. Express 19 9401
Google Scholar
[20] Ye D, Wang, Wang Z Y, Xu K W, Li H, J, Huangfu J T, Wang Z, Ran L X 2013 Phys. Rev. Lett. 111 187402
Google Scholar
[21] Ma H, Cui T J 2010 Nat. Commun. 1 124
Google Scholar
[22] Zhu B, Feng Y, Zhao J 2010 Appl. Phys. Lett. 97 051906
Google Scholar
[23] Xu Y, Gu C, Hou B, Lai Y, Li J, Chen H 2013 Nat. Commun. 4 2561
Google Scholar
[24] Ni X, Ishii S, Kildishev A V, Shalaev V M 2013 Light Sci. Appl. 2 e72
Google Scholar
[25] Yin X, Ye Z, Rho J, Wang Y, Zhang X 2013 Science 339 1405
Google Scholar
[26] Xu Y, Fu Y, Chen H 2015 Sci. Rep. 5 12219
Google Scholar
[27] Ra’di Y, Sounas D L, Alù A 2017 Phys. Rev. Lett. 119 067404
Google Scholar
[28] Chalabi H, Ra’di Y, Sounas D L, Alù A 2017 Phys. Rev. B 96 075432
Google Scholar
[29] Fu Y, Cao Y, Xu Y 2019 Appl. Phys. Lett. 114 053502
Google Scholar
[30] Qian E T, Fu Y, Xu Y, Chen H 2016 Europhys. Lett. 114 34003
Google Scholar
[31] Du J, Lin Z, Chui S T, Dong G, Zhang W 2013 Phys. Rev. Lett. 110 163902
Google Scholar
[32] Fu Y, Shen C, Cao Y, et al. 2019 Nat. Commun. 10 2326
Google Scholar
-
图 1 单元个数m = 6 (a)和m = 7 (b)的光学超构笼子的结构示意图
Fig. 1. Schematics of the optical meta-cage with the number of unit cells m = 6 (a) and m = 7 (b), respectively.
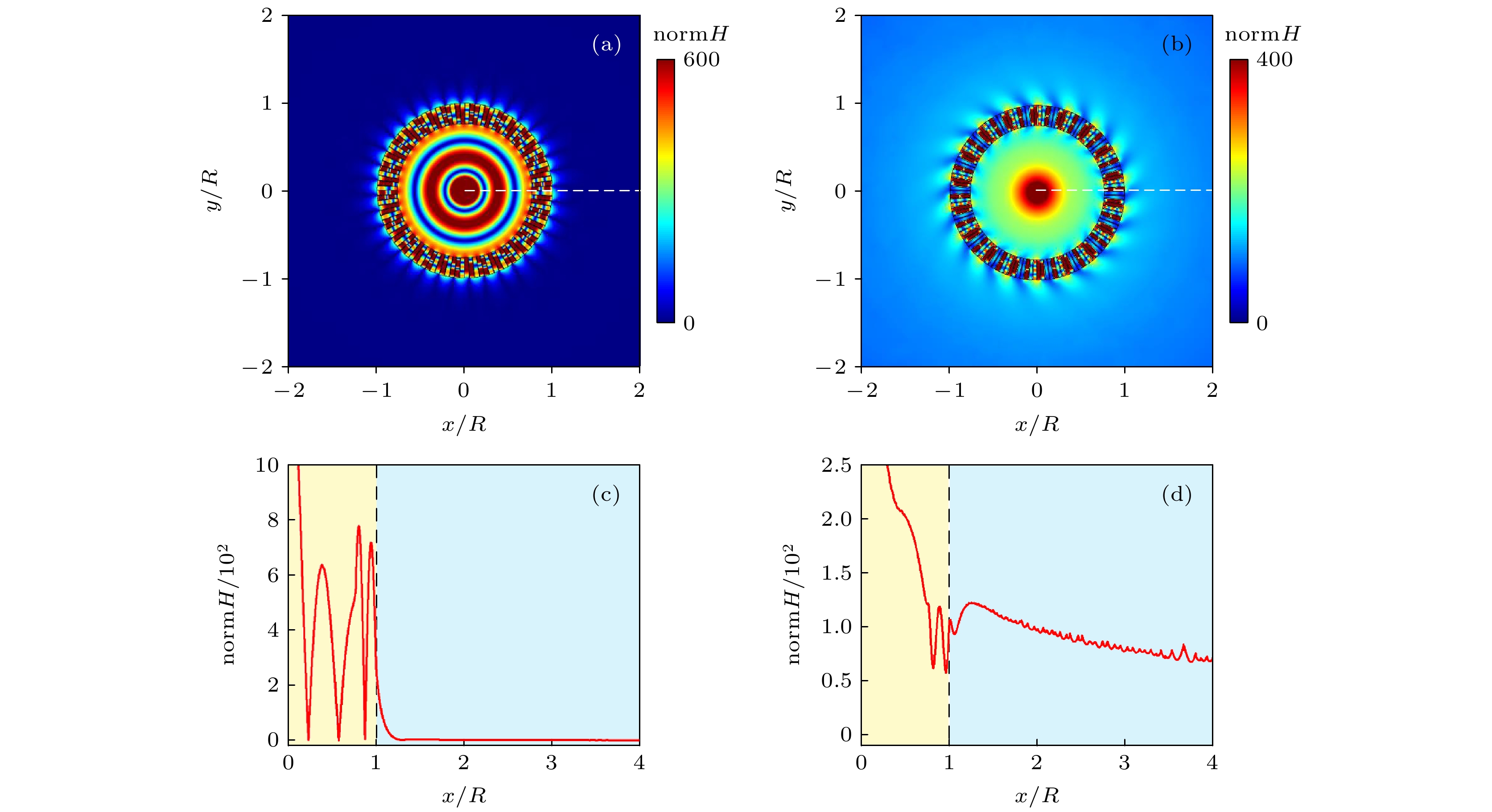
图 2 单元个数为m = 6 (a)和m = 7 (b)时超构笼子的磁场模分布情况; (c), (d)分别对应图(a), (b)中白色虚线处的磁场模具体数值大小
Fig. 2. Magnetic field distribution of the meta-cage for the number of unit cells m = 6 (a) and m = 7 (b), respectively; (c) and (d) correspond to the specific magnitude of the magnetic field along the white dashed line from the center to 4R in Fig. 2(a) and (b), respectively.
图 3 (a) 单元个数m不同时, 数值仿真和解析计算得到的超构笼子的透射效率, 红色圆圈是数值模拟结果, 蓝色五角星是解析计算结果; (b)以m = 6和m = 7为例, 超构笼子的透射效率与入射波长的关系
Fig. 3. (a) The numerical and analytical results for the transmission efficiency of the meta-cage vs. the number of unit cells m. The red circles represent the numerical results and the blue pentagrams represent the analytical results. (b) The transmission efficiency of the meta-cage vs. the incident wavelength for the case of m = 6 and m = 7.
图 4 (a)点源逐渐偏离正中心时超构笼子的透射效率; (b)点源偏离正中心时的磁场模空间分布情况, 上行图对应m = 6, 下行图对应 m = 7
Fig. 4. (a) The transmission efficiency of the meta-cage as the source gradually deviates from the center; (b) the distribution of the magnetic field as the source deviates from the center. The upper row corresponds to the case of m = 6 and the lower row corresponds to the case of m = 7.
-
[1] Yu N, Genevet P, Kats M A, Aieta F, Tetienne J P, Capasso F, Gaburro Z 2011 Science 334 333
Google Scholar
[2] Kildishev A V, Boltasseva A, Shalaev V M 2013 Science 339 1232009
Google Scholar
[3] Yu N, Capasso F 2014 Nat. Mater. 13 139
Google Scholar
[4] Zhao Y, Liu X, Alù A 2014 J. Opt. 16 123001
Google Scholar
[5] Xu Y, Fu Y, Chen H 2016 Nat. Rev. Mater. 1 16067
Google Scholar
[6] Shi H Y, Zhang A X, Chen J Z, Wang J F, Xia S, Xu Z 2016 Chin. Phys. B 25 078105
Google Scholar
[7] Zhao J, Yang X, Dai J Y, et al. 2018 Natl. Sci. Rev. 6 231
[8] Pendry J B 2003 Opt. Express 11 755
Google Scholar
[9] Fang N, Lee H, Sun C, Zhang X 2005 Science 308 534
Google Scholar
[10] Smolyaninov I I, Hung Y J, Davis C C 2007 Science 315 1699
Google Scholar
[11] Liu Z, Lee H, Xiong Y, Sun C, Zhang X 2007 Science 315 1686
Google Scholar
[12] Rho J, Zi L Y, Yi X, Xiao B Y, Zhao W L, Hyeunseok C, Guy B, Xiang Z 2010 Nat. Commun. 1 143
Google Scholar
[13] Lu D, Liu Z 2012 Nat. Commun. 3 1205
Google Scholar
[14] Enoch S, Tayeb G, Sabouroux P, Guerin N, Vincent P 2002 Phys. Rev. Lett. 89 213902
Google Scholar
[15] Yuan Y, Lin F S, Li X R, Tao J, Jiang T, Huang F, Jin A K 2008 Phys. Rev. A 77 053821
Google Scholar
[16] Cheng Q, Jiang W X, Cui T J 2011 Appl. Phys. Lett. 99 131913
Google Scholar
[17] Liu X, Starr T, Starr A F, Padilla W J 2010 Phys. Rev. Lett. 104 207403
Google Scholar
[18] Chen H, Jiang F Z, John F O'Hara, Frank C, Abul K A, Antoinette J T 2010 Phys. Rev. Lett. 105 073901
Google Scholar
[19] Shen X, Tie J C, Jun M Z, Hui F M, Wei X J, Hui L 2011 Opt. Express 19 9401
Google Scholar
[20] Ye D, Wang, Wang Z Y, Xu K W, Li H, J, Huangfu J T, Wang Z, Ran L X 2013 Phys. Rev. Lett. 111 187402
Google Scholar
[21] Ma H, Cui T J 2010 Nat. Commun. 1 124
Google Scholar
[22] Zhu B, Feng Y, Zhao J 2010 Appl. Phys. Lett. 97 051906
Google Scholar
[23] Xu Y, Gu C, Hou B, Lai Y, Li J, Chen H 2013 Nat. Commun. 4 2561
Google Scholar
[24] Ni X, Ishii S, Kildishev A V, Shalaev V M 2013 Light Sci. Appl. 2 e72
Google Scholar
[25] Yin X, Ye Z, Rho J, Wang Y, Zhang X 2013 Science 339 1405
Google Scholar
[26] Xu Y, Fu Y, Chen H 2015 Sci. Rep. 5 12219
Google Scholar
[27] Ra’di Y, Sounas D L, Alù A 2017 Phys. Rev. Lett. 119 067404
Google Scholar
[28] Chalabi H, Ra’di Y, Sounas D L, Alù A 2017 Phys. Rev. B 96 075432
Google Scholar
[29] Fu Y, Cao Y, Xu Y 2019 Appl. Phys. Lett. 114 053502
Google Scholar
[30] Qian E T, Fu Y, Xu Y, Chen H 2016 Europhys. Lett. 114 34003
Google Scholar
[31] Du J, Lin Z, Chui S T, Dong G, Zhang W 2013 Phys. Rev. Lett. 110 163902
Google Scholar
[32] Fu Y, Shen C, Cao Y, et al. 2019 Nat. Commun. 10 2326
Google Scholar
下载:
计量
- 文章访问数: 3290
- PDF下载量: 70
- 被引次数: 0
