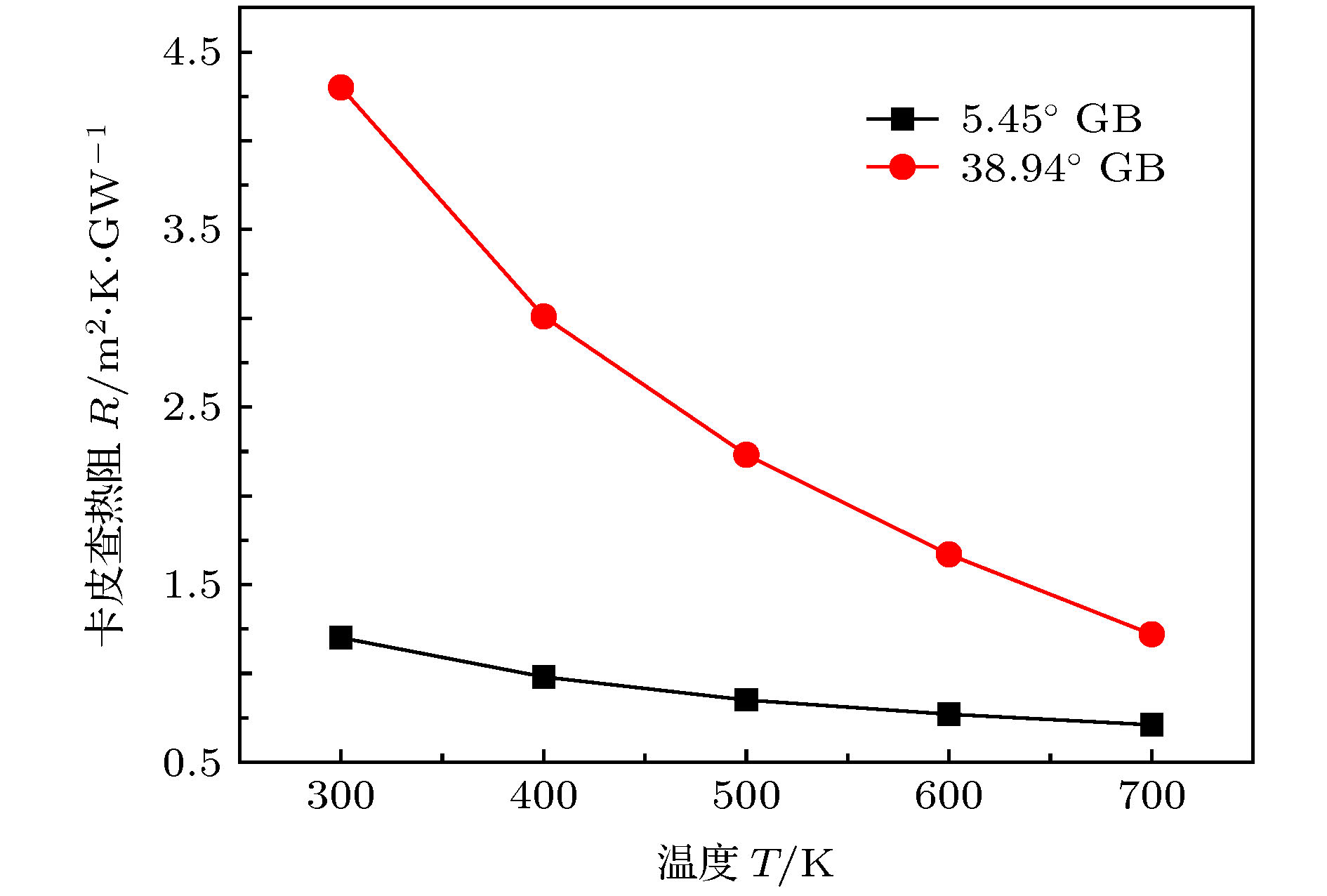
-
用嵌入位错线法和重合位置点阵法构建含有小角度和大角度倾斜角的双晶氧化锌纳米结构. 用非平衡分子动力学方法模拟双晶氧化锌在不同倾斜角度下的晶界能、卡皮查热阻, 并研究了样本长度和温度对卡皮查热阻和热导率的影响. 模拟结果表明, 晶界能在小角度区域随倾斜角线性增加, 而在大角度区域达到稳定, 与卡皮查热阻的变化趋势一致. 热导率随样本长度的增加而增加, 卡皮查热阻表现出相反的趋势. 然而随着温度的增加, 热导率和卡皮查热阻都减小. 通过比较含5.45°和38.94°晶界样本的声子态密度, 发现声子光学支对热传导的影响不大, 主要由声子声学支贡献, 大角度晶界对声子散射作用更强, 声学支波峰向低频率移动.Zinc oxide (ZnO), as a conventional semiconductor material, has excellent characteristics, such as piezoelectricity, photoelectricity, gas sensitivity, etc. With the improvement of nanopreparation technology, different types of nanostructrued ZnO compounds have appeared and their heat conductions have become a main research topic in nanodevices. In order to study the effects of grain boundary on the thermal properties of materials of this kind, bicrystal ZnO containing small-angle and high-angle grain boundaries are constructed by the embedded dislocation line and coincidence site lattice method. The variation of grain boundary energy with tilt angle is studied by the non-equilibrium molecular dynamics simulation. In addition, the dislocation density is calculated by using the Frank-Bilby formula. Our results show that the grain boundary energy and dislocation density increase with the increase of tilt angle in a small-angle region, and they tend to be stable in a high-angle region. The tilt angle of 36.86° is defined as the transition angle. The trend of the Kapitza resistance is the same as that of the grain boundary energy and satisfies the theoretical value from the extended Read-Shockley model. Furthermore, it is found that both the Kapitza resistance and thermal conductivity have a significant size effect. When the sample length is between 23.2 nm and 92.6 nm, the Kapitza resistance decreases sharply with the increase of the length and then tends to be stable. The thermal conductivity of the sample increases with length increasing, but is always less than that of the single crystal. At the same time, temperature is an important factor affecting the heat transport properties. The Kapitza resistance and thermal conductivity decrease with temperature increasing. At different temperatures, the Kapitza resistance of 38.94° grain boundary sample is greater than that of 5.45° grain boundary sample. In order to further explore the influence mechanism of grain boundary angle on heat conduction, the phonon state density of 5.45° and 38.94° grain boundary sample are calculated. The results indicate that the high-angle grain boundary has stronger scattering for acoustic branch phonons and the peak frequency becomes lower, whereas the optical branch ones have almost no effect on the heat conduction.
-
Keywords:
- tilt angle /
- grain boundary energy /
- Kapitza resistance /
- thermal conductivity
[1] Liu J, Jiang Y J 2010 Chin. Phys. B 19 116201
[2] Zhang Z, Kang Z, Liao Q L, Zhang X M, Zhang Y 2017 Chin. Phys. B 26 118102
Google Scholar
[3] 李酽, 李娇, 陈丽丽, 连晓雪, 朱俊武 2018 67 140701
Google Scholar
Li Y, Li J, Chen L L, Lian X X, Zhu J W 2018 Acta. Phys. Sin. 67 140701
Google Scholar
[4] Li J J, Chen Z M, Huang R, Miao Z Y, Cai L, Du Q P 2018 J. Environ. Sci. 73 78
Google Scholar
[5] He Q, Zhou Y J, Wang G F, Zheng B, Qi M, Li X J, Kong L H 2018 Appl. Nanosci. 8 2009
[6] 吴静静, 唐鑫, 龙飞, 唐碧玉 2017 66 137101
Google Scholar
Wu J J, Tang X, Long F, Tang B Y 2017 Acta. Phys. Sin. 66 137101
Google Scholar
[7] Zhang Z Z, Chen J 2018 Chin. Phys. B 27 035101
Google Scholar
[8] El-Brolossy T A, Saber O, Ibrahim S S 2013 Chin. Phys. B 22 074401
Google Scholar
[9] Giri A, Niemela J P, Tynell T, Gaskins J T, Donovan B F, Karppinen M, Hopkins P E 2016 Phys. Rev. B 93 115310
Google Scholar
[10] Liang X, Shen L 2018 Acta. Mater. 148 100
Google Scholar
[11] Swartz E T, Pohl R O 1989 Rev. Mod. Phys. 61 605
Google Scholar
[12] Little W A, Can J 1959 Canadian J. Phys. 37 334
[13] 王琛, 宋海洋, 安敏荣, 2014 66 046201
Google Scholar
Wang C, Song H Y, An M R 2014 Acta. Phys. Sin. 66 046201
Google Scholar
[14] Grimmer H 1976 Acta. Crystallogr. 32 783
Google Scholar
[15] Stukowski A 2010 Modell. Simul. Mater.Sci. 18 015012
Google Scholar
[16] Plimpton S J 1995 J. Comput. Phys. 117 1
Google Scholar
[17] Wunderlich W 1998 Phys. Status. Solidi. A 170 99
Google Scholar
[18] Tai K P, Lawrence A, Harmer M P, Dillon S J 2013 Appl. Phys. Lett. 102 034101
Google Scholar
[19] Liang X 2017 Phys. Rev. B 95 155313
Google Scholar
[20] Chen T Y, Chen D, Sencer B H, Shao L 2014 J. Nucl. Mater. 452 364
Google Scholar
[21] Watanabe T, Ni B, Phillpot S R, Schelling P K, Keblinski P 2007 J. Appl. Phys. 102 063503
[22] Yang X M, Wu S H, Xu J X, Cao B Y, To A C 2018 Physica E 96 46
Google Scholar
-
图 1 含5.45°晶界(左)和38.94°晶界(右)的双晶ZnO结构
Fig. 1. Structures of the 5.45° grain boundary (GB) (left) and the 38.94° GB (right) in bicrystal ZnO.
图 2 NEMD模拟计算热性质的示意图
Fig. 2. Schematic diagram of the NEMD model for calculating the thermal properties.
图 3 晶界能和位错密度与倾斜角的关系
Fig. 3. The GB energy and dislocation density as a function of misorientation angle.
图 5 300 K时卡皮查热阻与样本长度的关系
Fig. 5. Kapitza resistance as a function of sample length at 300 K.
图 7 5.45°和38.95°晶界结构的卡皮查热阻随温度的变化
Fig. 7. Temperature-dependent Kapitza resistance for the 5.45° and 38.94° GBs.
图 8 5.45°和38.95°晶界结构的热导率随温度的变化
Fig. 8. Temperature-dependent thermal conductivity for the 5.45° and 38.94° GBs.
图 9 5.45°和38.94°晶界结构的声子态密度比较
Fig. 9. Phonon density of states for the 5.45° and 38.94° GBs.
表 1 对称倾斜角度与对应的Σ值
Table 1. List of the symmetrical tilt angles and the corresponding Σ values.
Tilt angle θ/(°) Σ 5.45 221 11.42 101 20.02 149 25.98 89 30.51 65 36.86 5 38.94 9 53.13 5 60.0 3 67.38 13 
下载: 导出CSV
-
[1] Liu J, Jiang Y J 2010 Chin. Phys. B 19 116201
[2] Zhang Z, Kang Z, Liao Q L, Zhang X M, Zhang Y 2017 Chin. Phys. B 26 118102
Google Scholar
[3] 李酽, 李娇, 陈丽丽, 连晓雪, 朱俊武 2018 67 140701
Google Scholar
Li Y, Li J, Chen L L, Lian X X, Zhu J W 2018 Acta. Phys. Sin. 67 140701
Google Scholar
[4] Li J J, Chen Z M, Huang R, Miao Z Y, Cai L, Du Q P 2018 J. Environ. Sci. 73 78
Google Scholar
[5] He Q, Zhou Y J, Wang G F, Zheng B, Qi M, Li X J, Kong L H 2018 Appl. Nanosci. 8 2009
[6] 吴静静, 唐鑫, 龙飞, 唐碧玉 2017 66 137101
Google Scholar
Wu J J, Tang X, Long F, Tang B Y 2017 Acta. Phys. Sin. 66 137101
Google Scholar
[7] Zhang Z Z, Chen J 2018 Chin. Phys. B 27 035101
Google Scholar
[8] El-Brolossy T A, Saber O, Ibrahim S S 2013 Chin. Phys. B 22 074401
Google Scholar
[9] Giri A, Niemela J P, Tynell T, Gaskins J T, Donovan B F, Karppinen M, Hopkins P E 2016 Phys. Rev. B 93 115310
Google Scholar
[10] Liang X, Shen L 2018 Acta. Mater. 148 100
Google Scholar
[11] Swartz E T, Pohl R O 1989 Rev. Mod. Phys. 61 605
Google Scholar
[12] Little W A, Can J 1959 Canadian J. Phys. 37 334
[13] 王琛, 宋海洋, 安敏荣, 2014 66 046201
Google Scholar
Wang C, Song H Y, An M R 2014 Acta. Phys. Sin. 66 046201
Google Scholar
[14] Grimmer H 1976 Acta. Crystallogr. 32 783
Google Scholar
[15] Stukowski A 2010 Modell. Simul. Mater.Sci. 18 015012
Google Scholar
[16] Plimpton S J 1995 J. Comput. Phys. 117 1
Google Scholar
[17] Wunderlich W 1998 Phys. Status. Solidi. A 170 99
Google Scholar
[18] Tai K P, Lawrence A, Harmer M P, Dillon S J 2013 Appl. Phys. Lett. 102 034101
Google Scholar
[19] Liang X 2017 Phys. Rev. B 95 155313
Google Scholar
[20] Chen T Y, Chen D, Sencer B H, Shao L 2014 J. Nucl. Mater. 452 364
Google Scholar
[21] Watanabe T, Ni B, Phillpot S R, Schelling P K, Keblinski P 2007 J. Appl. Phys. 102 063503
[22] Yang X M, Wu S H, Xu J X, Cao B Y, To A C 2018 Physica E 96 46
Google Scholar
下载:
计量
- 文章访问数: 6840
- PDF下载量: 90
- 被引次数: 0
