-
准确预测GaN半导体材料的热导率对GaN基功率电子器件的热设计具有重要意义. 本文基于第一性原理计算和经典Debye-Callaway模型,通过分析和完善Debye-Callaway模型中关于声子散射率的子模型, 建立了用于预测温度、同位素、点缺陷、位错、薄膜厚度、应力等因素影响的GaN薄膜热导率的理论模型. 具体来说, 对声子间散射项和同位素散射项基于第一性原理计算数据进行了系数拟合, 讨论了两种典型的处理点缺陷和位错散射的散射率模型, 引入了应用抑制函数描述的各向异性边界散射模型,并对应力的影响进行了建模. 热导率模型预测值和文献中典型实验数据的对比表明, 基于第一性原理计算数据拟合的热导率模型和实验测量值总体符合较好, 300 K温度附近热导率数值及其随温度变化的趋势存在20%左右的偏差. 结合实验数据和热导率模型进一步确认了第一性原理计算会高估同位素散射的影响, 给出了薄膜热导率随薄膜厚度、位错面密度、点缺陷浓度的具体变化关系, 同位素和缺陷散射会减弱薄膜热导率的尺寸效应, 主要体现在100 nm附近及更小的厚度范围.
-
关键词:
- GaN薄膜 /
- 热导率 /
- Debye-Callaway模型 /
- 第一性原理计算
The accurate predicting of thermal conductivity of GaN semiconductors, especially GaN films, is of great importance for the thermal management in electronic devices. In this paper, a theoretical model based on the first-principles calculations and Debye-Callaway model is proposed to predict the thermal conductivity of GaN films, which is a function of temperature, isotope, point defects, dislocations, film thickness, and strain fields. Specifically, the coefficients in our theoretical model that used to capture umklapp scattering and phonon-isotope scattering are fitted with the data from first-principles calculations, and two sub-models for point defects scattering and dislocation scattering are discussed, respectively. The sub-model of boundary scattering with suppression function is introduced to describe the anisotropy of size effect, and the effect of in-plane strain (perpendicular to polar axis) is also discussed. The comparison between theoretical predictions and experimental data shows that the model performs well roughly in a large temperature range from 300 to 500 K, with an around 20% difference at room temperature. Our predicted temperature dependent thermal conductivity deviates slightly from the measurements, which may result from the lack of high-order phonon scattering in our model, e.g., four-phonon scattering. Our results also show that the first-principles calculations for GaN overestimates the influence of isotope scattering. We further study the thermal transport properties of GaN film which are influenced by the thickness, dislocation density, and point defect density through using the new theoretical model. Significant reduction of thermal conductivity is found to occur at a film thickness of 10 μm, which is consistent with the findings from the first-principles calculations. The isotope and defects including point defects and dislocations are found to have a weak influence on the thermal conductivity when the thickness of GaN film is larger than 100 nm, while the influence becomes significant for the film with and below 100 nm in thickness. In addition, dislocations and point defects start to reduce thermal conductivity significantly when the surface density of dislocations increases to 1010 cm–2 and point defect density reaches1018 cm–3.[1] Guggenheim R, Rodes L IEEE International Conference on Microwaves, Antennas, Communications and Electronic Systems (COMCAS) Tel-Aviv, Israel, November 13–15, 2017 p1
[2] Amano H, Baines Y, Beam E, et al. 2018 J. Phys. D: Applied Physics 51 163001
Google Scholar
[3] Hua Y, Li H, Cao B 2019 IEEE Trans. Electron Dev. 66 3296
Google Scholar
[4] Shibata H, Waseda Y, Ohta H, Kiyomi K, Shimoyama K, Fujito K, Nagaoka H, Kagamitani Y, Simura R, Fukuda T 2007 Mater. Trans. 48 2782
Google Scholar
[5] Slack G A, Schowalter L J, Morelli D, Freitas Jr J A 2002 J. Cryst. Growth 246 287
Google Scholar
[6] Jeżowski A, Danilchenko B, Bockowski M, Grzegory I, Krukowski S, Suski T, Paszkiewicz T 2003 Solid State Commun. 128 69
Google Scholar
[7] Jeżowski A, Churiukova O, Mucha J, Suski T, Obukhov I A, Danilchenko B A 2015 Mater. Res. Exp. 2 085902
Google Scholar
[8] Simon R B, Anaya J, Martin. K 2014 Appl. Phys. Lett. 105 202105
Google Scholar
[9] Rounds R, Sarkar B, Sochacki T, Bockowski M, Imanishi M, Mori Y, Kirste R, Collazo R, Sitar Z 2018 J. Appl. Phys. 124 105106
Google Scholar
[10] Li H, Hanus R, Polanco C A, Zeidler A, Koblmüller G, Koh Y K, Lindsay L 2020 Phys. Rev. B 102 014313
Google Scholar
[11] Mion C, Muth J F, Preble E A, Hanser D 2006 Appl. Phys. Lett. 89 092123
Google Scholar
[12] Paskov P P, Slomski M, Leach J H, Muth J F, Paskova T 2017 AIP Adv. 7 095302
Google Scholar
[13] Zheng Q, Li C, Rai A, Leach J H, Broido D A, Cahill D G 2019 Phys. Rev. Mater. 3 014601
Google Scholar
[14] Lindsay L, Hua C, Ruan X L, Lee S 2018 Mater. Today Phys. 7 106
Google Scholar
[15] Bao H, Chen J, Gu X, Cao B 2018 ES Energy Environ. 1 16
Google Scholar
[16] Wang T, Carrete J, Mingo N, Madsen G K H 2019 ACS Appl Mater Interfaces 11 8175
Google Scholar
[17] Katre A, Carrete J, Wang T, Madsen Georg K H, Mingo N 2018 Phys. Rev. Mater. 2 050602
Google Scholar
[18] Lindsay L, Broido D A, Reinecke T L 2012 Phys. Rev. Lett. 109 095901
Google Scholar
[19] Yang J, Qin G, Hu M 2016 Appl. Phys. Lett. 109 242103
Google Scholar
[20] Yuan K, Zhang X, Tang D, Hu M 2018 Phys. Rev. B 98 144303
Google Scholar
[21] Togo A, Chaput L, Tanaka I 2015 Phys. Rev. B 91 094306
Google Scholar
[22] Tang D, Qin G, Hu M, Cao B 2020 J. Appl. Phys. 127 035102
Google Scholar
[23] Callaway J 1959 Phys. Rev. 113 1046
Google Scholar
[24] AlShaikhi A, Barman S, Srivastava G P 2010 Phys. Rev. B 81 195320
Google Scholar
[25] Zou J, Kotchetkov D, Balandin A, Florescu D I, Pollak F H 2002 J. Appl. Phys. 92 2534
Google Scholar
[26] Kotchetkov D, Zou J, Balandin A A, Florescu D I, Pollak F H 2001 Appl. Phys. Lett. 79 4316
Google Scholar
[27] Termentzidis K, Isaiev M, Salnikova A, Belabbas I, Lacroix D, Kioseoglou J 2018 Phys. Chem. Chem. Phys. 20 5159
Google Scholar
[28] Klemens P G 1955 Proc. Phys. Soc. A 68 1113
Google Scholar
[29] Klemens P G 1958 Solid State Physics (Vol. 7) (New York: Academic) pp1−98
[30] Gurunathan R, Hanus R, Dylla M, Katre A, Snyder G J 2020 Phys. Rev. Appl. 13 034011
Google Scholar
[31] Morelli D T, Heremans J P, Slack G A 2002 Phys. Rev. B 66 195304
Google Scholar
[32] Carruthers P 1961 Rev. Mod. Phys. 33 92
Google Scholar
[33] Hua Y, Cao B 2017 Nanosc. Microsc. Therm. Eng. 21 159
Google Scholar
[34] Kresse G, Furthmuller J 1996 Phys. Rev. B 54 11169
Google Scholar
[35] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[36] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
Google Scholar
[37] Monkhorst H J, Pack J D 1976 Phys. Rev. B 13 5188
Google Scholar
[38] Togo A, Tanaka I 2015 Scripta Mater. 108 1
Google Scholar
[39] Li W, Carrete J, A. Katcho N, Mingo N 2014 Comput. Phys. Commun. 185 1747
Google Scholar
[40] Ruf T, Serrano J, Cardona M, Pavone P, Pabst M, Krisch M, D'Astuto M, Suski T, Grzegory I, Leszczynski M 2001 Phys. Rev. Lett. 86 906
Google Scholar
[41] Herring C 1954 Phys. Rev. 95 954
Google Scholar
[42] Ma J, Li W, Luo X 2014 Phys. Rev. B 90 035203
Google Scholar
[43] Shannon R D 1976 Acta Crystallogr A 32 751
Google Scholar
[44] Sun Y, Zhou Y, Han J, Liu W, Nan C, Lin Y, Hu M, Xu B 2019 npj Comput. Mater. 5 97
Google Scholar
[45] Hua Y, Cao B 2016 Int. J. Therm. Sci. 101 126
Google Scholar
[46] Hua Y, Cao B 2017 Appl. Therm. Eng. 111 1401
Google Scholar
[47] Hua Y, Cao B 2017 J. Phys. Chem. C 121 5293
Google Scholar
[48] Li H, Cao B 2018 Nanosc. Microsc. Therm. Eng. 23 10
Google Scholar
[49] Hua Y, Cao B 2016 Int. J. Heat Mass Transfer 92 995
Google Scholar
[50] Fujito K, Kubo S, Nagaoka H, Mochizuki T, Namita H, Nagao S 2009 J. Cryst. Growth 311 3011
Google Scholar
[51] Suihkonen S, Pimputkar S, Sintonen S, Tuomisto F 2017 Adv. Electron. Mater. 3 1600496
Google Scholar
-
图 1 (a) 面向应变下GaN相对热导率随温度和同位素的变化; (b) 相对热导率和应变的拟合关系(二次多项式拟合)
Fig. 1. (a) Variations of thermal conductivity ratios with respect to temperature and isotopes under in-plane strain (quadratic polynomial fitting); (b) Fitting relation between relative thermal conductivity and strain
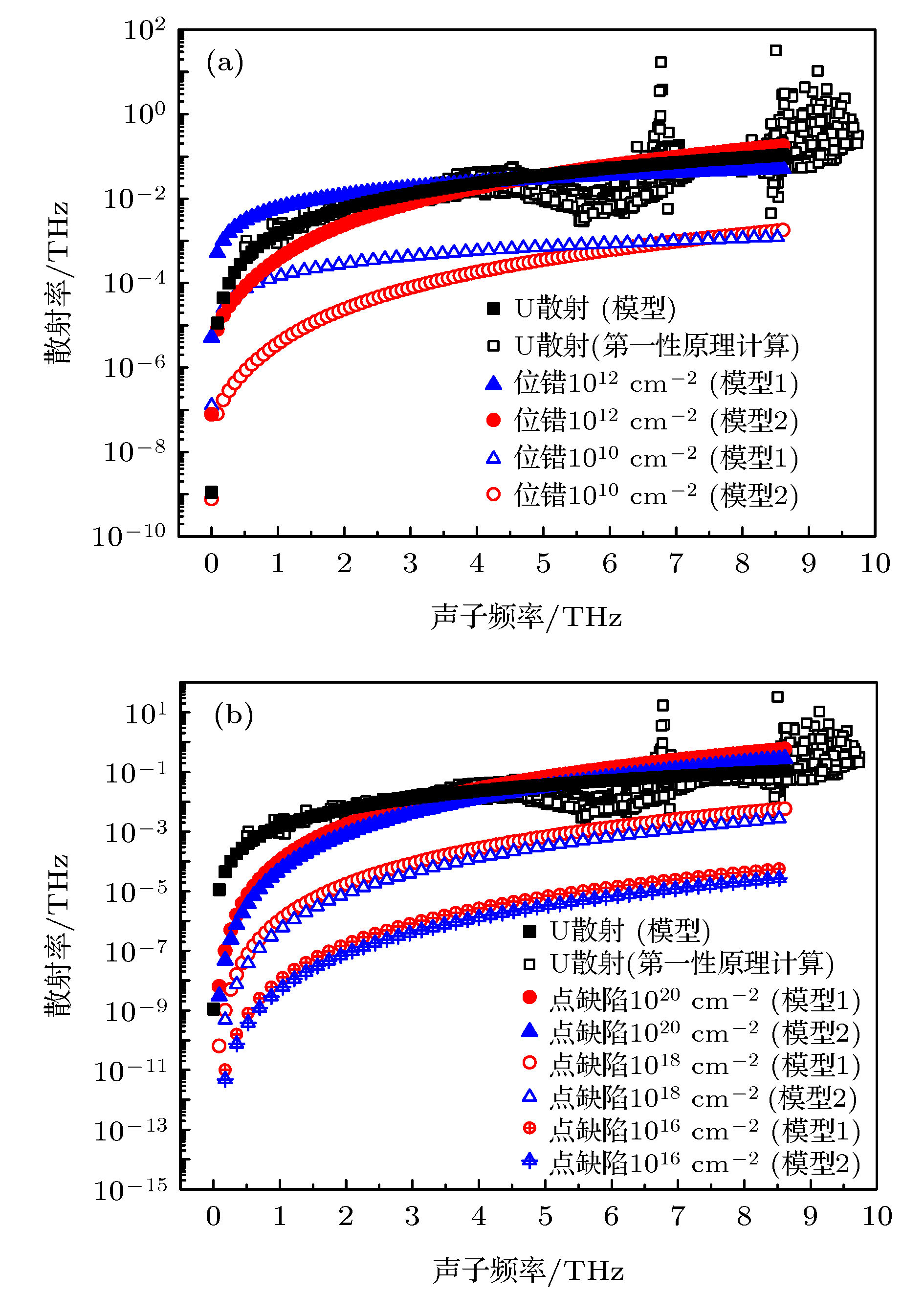
图 2 基于第一性原理计算的GaN热导率数据拟合U散射率和同位素散射率模型中的参数 (a) 纯晶体垂直轴向; (b) 纯晶体沿轴向; (c) 含同位素晶体垂直轴向; (d) 含同位素晶体沿轴向
Fig. 2. Fitting parameters for sub-models of U scattering and isotope scattering based on thermal conductivity data from first-principles calculations: (a) Pure GaN perpendicular to polar axis; (b) pure GaN along polar axis; (c) GaN with isotopes perpendicular to polar axis; (d) GaN with isotopes along polar axis.
图 3 热导率模型值(线)和测量值(点, 均为法向热导率)的比较. (a), (c), (e) 分别对应三组不同的GaN薄膜样品, 实验数据来自文献 [13], 其中(a)中数据为无同位素散射的纯晶体样品. (b), (d), (f)中坐标采用了对数坐标, 图中数据分别和(a), (c), (e)相同. 样品具体表征数据可以参考表1
Fig. 3. Comparisons between cross-plane thermal conductivities from the model (lines) and experiments (dots) in literature[13], in which the GaN films for (a) are pure crystal samples with enriched isotopes. Thermal conductivities of three groups GaN films are shown in (a), (c), and (e), respectively. In (b), (d), and (f), the same data are shown corresponding to (a), (c), and (e) in logarithmic coordinates. The detailed characteristics of samples can refer to Table 1.
图 4 热导率模型值(线)和测量值(点)的比较(线和点颜色对应), 图 (a)—(h)实验数据依次分别来自文献 [4-11]. 样品具体表征数据可以参考表1
Fig. 4. Comparisons between thermal conductivities from the model (lines) and experiments (dots) from literatures [4-11], the lines correspond to the dots with the same color. The detailed characteristics of samples can refer to Table 1.
图 5 U过程声子散射率(300 K)和不同密度下参数的比较 (a)位错散射率;(b)点缺陷散射率(缺陷元素为O元素)
Fig. 5. Comparisons among U scattering rates at 300 K: (a) Dislocation scattering rates at two different densities; (b) point defect scattering rates at two different densities with defect atom O.
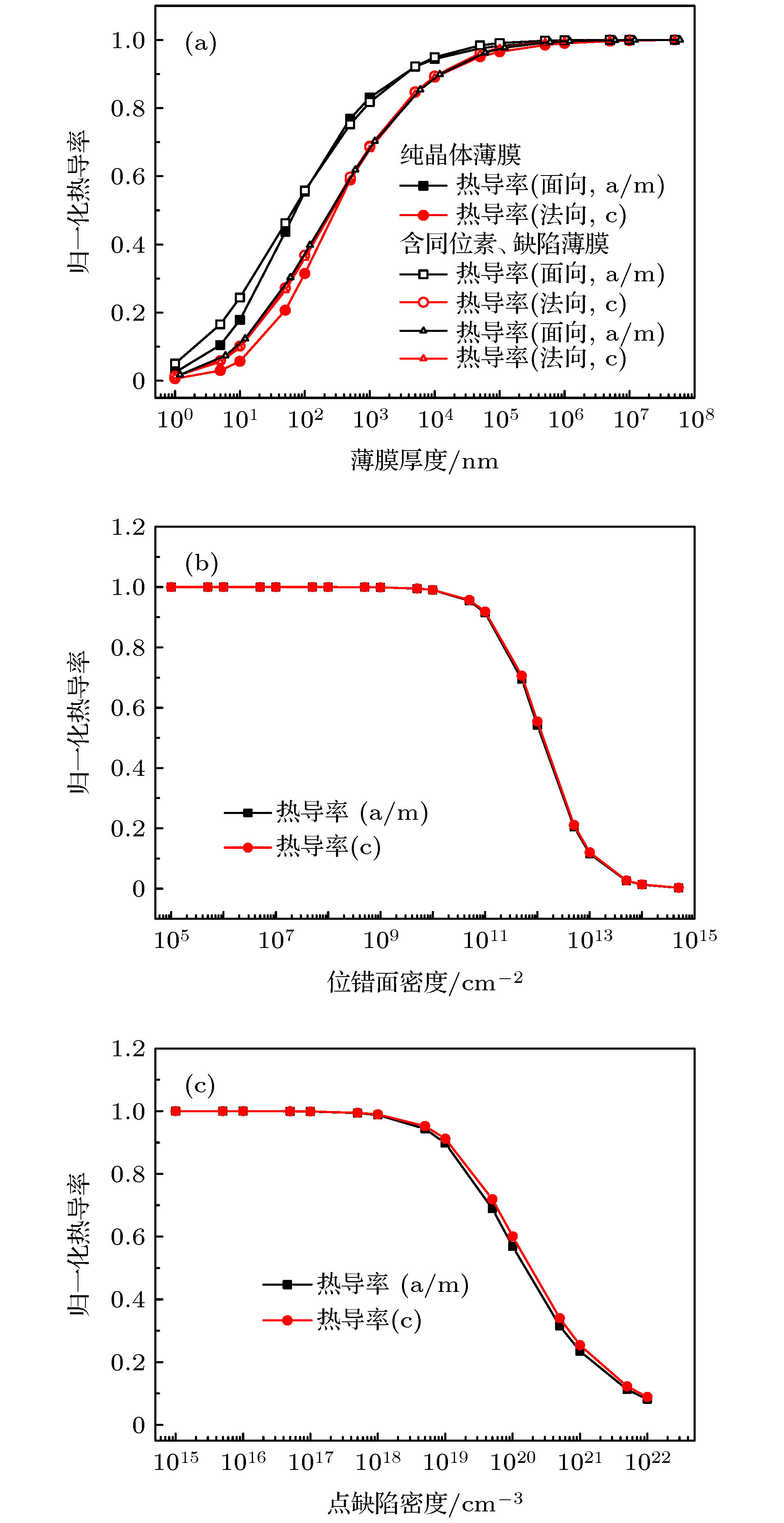
图 6 室温下GaN薄膜归一化热导率随 (a) 薄膜厚度(缺陷设置为位错面密度1012 cm–2, 点缺陷浓度1018 cm–3), (b) 位错面密度, (c) 点缺陷浓度的变化. (a)图中方形和圆形点线表示本文采用的抑制函数边界散射模型((23)式), 三角形点线表示文献中通常使用的边界散射模型((19)式)
Fig. 6. Normalized thermal conductivity of GaN films at room temperature with respect to (a) film thickness (dislocation density 1012 cm–2, point defect density 1018 cm–3), (b) dislocation density, (c) point defect density. Square and circle dots represent normalized thermal conductivity with suppression function model (Eq. ((23)), and triangle dots represent normalized thermal conductivity with common used boundary scattering model (Eq. ((19)).
表 1 文献中的GaN薄膜室温热导率数据及样品表征
Table 1. Thermal conductivity of GaN films at room temperature and characteristic from literature
文献/样品 室温热导率/
(W·m–1·K–1)厚度/μm 点缺陷浓度(缺陷元素)/cm–3 位错面密
度/cm–2同位素 Zheng et al.[13] KMiF 234 7—12 1.5 × 1018 (Al),
5 × 1017 cm–3 (O)< 107 无 KMF 195 6—8 1.5 × 1018 (Al),
5 × 1017 cm–3 (O)< 107 有 AM/KM 197 300—600 0.1 × 1018—20 × 1018 cm–3 (H)
0.1 × 1018—5 × 1018 cm–3 (O)< 107 有 Shibata et al.[4] 1 252 1000 2.1 × 10–17 cm–3 (Si) 5 × 106 有 Slack et al.[5] 1 — 200 2.1 × 1016 cm–3 (O),
0.37 × 1016 cm–3 (Si)— 有 Jezowski et al.[6] 1 218 100 1 × 1020 cm–3 (O),
1 × 1019 cm–3 (C),
1 × 1018 cm–3 (Mg),
7 × 1017 cm–3 (H),
1017 cm–3 (Si),
1 × 1018 cm–3 (Ga原子空位)— 有 2 188 3 157 Jeżowski et al.[7] 1 78 300 4 × 1016 cm–3 (O) — 有 2 162 2.6 × 1018 cm–3 (O) 3 275 1.1 × 1020 cm–3 (O) Simon et al.[8] 1 226 300 2.3 × 1018 cm–3 (O),
2.3 × 1018 cm–3 (Mg)— 有 2 211 9.7 × 1017 cm–3 (O) 3 163 2 × 1019 cm–3 (O) Rounds et al.[9] 1 203 300—600 4 × 1016 cm–3 (O) — 有 2 223 2.2 × 1017 cm–3 (Si) 3 182 8.4 × 1018 cm–3 (H),
8.9 × 1018 cm–3 (O)4 200 1.5 × 1019 cm–3 (H),
1.4 × 1018 cm–3 (O),
9.8 × 1018 cm–3 (Mn)5 163 3.5 × 1018 cm–3 (H),
1.4 × 1018 cm–3 (O),
9.3 × 1017 cm–3 (Mg)Li et al.[10] S1 190 3.19 — 1.8 × 108 有 S2 195 3.52 2.36 × 109 S3 220 400 2 × 107 Mion et al.[11] A 184 200 4 × 1017 (H),
1017 (C),
3 × 1016 (O),
1017 (Si)4.06 × 107 有 B 200 370 1.47 × 107 C 214 1400 8.96 × 106 D 229 2000 5.1 × 104 
下载: 导出CSV
表 2 模型中使用的基本参数
Table 2. Basic parameters used in the model.
参数 数值 参数 数值 a /Å 3.219 $ \bar v $ (垂直轴向)/m·s–1 3621 c /Å 5.245 $ \bar v $ (沿轴向) /m·s–1 3915 V0 /m3 1.176 × 10–29 $ \nu $(泊松比) 0.255
下载: 导出CSV
表 3 GaN弹性常数分量 (单位: GPa).
Table 3. Components of elastic constants of GaN (unit: GPa).
C11 C33 C12 C13 C44 C66 322.7 356.6 110.4 77.7 106.2 90.0
下载: 导出CSV
表 4 声子散射率模型中的拟合参数
Table 4. Fitting parameters in phonon scattering sub-models.
拟合参数 A B C 垂直轴向 (a/m) 1.94 × 10–19 139 2.91 × 10–44 轴向 (c) 1.85 × 10–19 139 2.30 × 10–44
下载: 导出CSV
-
[1] Guggenheim R, Rodes L IEEE International Conference on Microwaves, Antennas, Communications and Electronic Systems (COMCAS) Tel-Aviv, Israel, November 13–15, 2017 p1
[2] Amano H, Baines Y, Beam E, et al. 2018 J. Phys. D: Applied Physics 51 163001
Google Scholar
[3] Hua Y, Li H, Cao B 2019 IEEE Trans. Electron Dev. 66 3296
Google Scholar
[4] Shibata H, Waseda Y, Ohta H, Kiyomi K, Shimoyama K, Fujito K, Nagaoka H, Kagamitani Y, Simura R, Fukuda T 2007 Mater. Trans. 48 2782
Google Scholar
[5] Slack G A, Schowalter L J, Morelli D, Freitas Jr J A 2002 J. Cryst. Growth 246 287
Google Scholar
[6] Jeżowski A, Danilchenko B, Bockowski M, Grzegory I, Krukowski S, Suski T, Paszkiewicz T 2003 Solid State Commun. 128 69
Google Scholar
[7] Jeżowski A, Churiukova O, Mucha J, Suski T, Obukhov I A, Danilchenko B A 2015 Mater. Res. Exp. 2 085902
Google Scholar
[8] Simon R B, Anaya J, Martin. K 2014 Appl. Phys. Lett. 105 202105
Google Scholar
[9] Rounds R, Sarkar B, Sochacki T, Bockowski M, Imanishi M, Mori Y, Kirste R, Collazo R, Sitar Z 2018 J. Appl. Phys. 124 105106
Google Scholar
[10] Li H, Hanus R, Polanco C A, Zeidler A, Koblmüller G, Koh Y K, Lindsay L 2020 Phys. Rev. B 102 014313
Google Scholar
[11] Mion C, Muth J F, Preble E A, Hanser D 2006 Appl. Phys. Lett. 89 092123
Google Scholar
[12] Paskov P P, Slomski M, Leach J H, Muth J F, Paskova T 2017 AIP Adv. 7 095302
Google Scholar
[13] Zheng Q, Li C, Rai A, Leach J H, Broido D A, Cahill D G 2019 Phys. Rev. Mater. 3 014601
Google Scholar
[14] Lindsay L, Hua C, Ruan X L, Lee S 2018 Mater. Today Phys. 7 106
Google Scholar
[15] Bao H, Chen J, Gu X, Cao B 2018 ES Energy Environ. 1 16
Google Scholar
[16] Wang T, Carrete J, Mingo N, Madsen G K H 2019 ACS Appl Mater Interfaces 11 8175
Google Scholar
[17] Katre A, Carrete J, Wang T, Madsen Georg K H, Mingo N 2018 Phys. Rev. Mater. 2 050602
Google Scholar
[18] Lindsay L, Broido D A, Reinecke T L 2012 Phys. Rev. Lett. 109 095901
Google Scholar
[19] Yang J, Qin G, Hu M 2016 Appl. Phys. Lett. 109 242103
Google Scholar
[20] Yuan K, Zhang X, Tang D, Hu M 2018 Phys. Rev. B 98 144303
Google Scholar
[21] Togo A, Chaput L, Tanaka I 2015 Phys. Rev. B 91 094306
Google Scholar
[22] Tang D, Qin G, Hu M, Cao B 2020 J. Appl. Phys. 127 035102
Google Scholar
[23] Callaway J 1959 Phys. Rev. 113 1046
Google Scholar
[24] AlShaikhi A, Barman S, Srivastava G P 2010 Phys. Rev. B 81 195320
Google Scholar
[25] Zou J, Kotchetkov D, Balandin A, Florescu D I, Pollak F H 2002 J. Appl. Phys. 92 2534
Google Scholar
[26] Kotchetkov D, Zou J, Balandin A A, Florescu D I, Pollak F H 2001 Appl. Phys. Lett. 79 4316
Google Scholar
[27] Termentzidis K, Isaiev M, Salnikova A, Belabbas I, Lacroix D, Kioseoglou J 2018 Phys. Chem. Chem. Phys. 20 5159
Google Scholar
[28] Klemens P G 1955 Proc. Phys. Soc. A 68 1113
Google Scholar
[29] Klemens P G 1958 Solid State Physics (Vol. 7) (New York: Academic) pp1−98
[30] Gurunathan R, Hanus R, Dylla M, Katre A, Snyder G J 2020 Phys. Rev. Appl. 13 034011
Google Scholar
[31] Morelli D T, Heremans J P, Slack G A 2002 Phys. Rev. B 66 195304
Google Scholar
[32] Carruthers P 1961 Rev. Mod. Phys. 33 92
Google Scholar
[33] Hua Y, Cao B 2017 Nanosc. Microsc. Therm. Eng. 21 159
Google Scholar
[34] Kresse G, Furthmuller J 1996 Phys. Rev. B 54 11169
Google Scholar
[35] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[36] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
Google Scholar
[37] Monkhorst H J, Pack J D 1976 Phys. Rev. B 13 5188
Google Scholar
[38] Togo A, Tanaka I 2015 Scripta Mater. 108 1
Google Scholar
[39] Li W, Carrete J, A. Katcho N, Mingo N 2014 Comput. Phys. Commun. 185 1747
Google Scholar
[40] Ruf T, Serrano J, Cardona M, Pavone P, Pabst M, Krisch M, D'Astuto M, Suski T, Grzegory I, Leszczynski M 2001 Phys. Rev. Lett. 86 906
Google Scholar
[41] Herring C 1954 Phys. Rev. 95 954
Google Scholar
[42] Ma J, Li W, Luo X 2014 Phys. Rev. B 90 035203
Google Scholar
[43] Shannon R D 1976 Acta Crystallogr A 32 751
Google Scholar
[44] Sun Y, Zhou Y, Han J, Liu W, Nan C, Lin Y, Hu M, Xu B 2019 npj Comput. Mater. 5 97
Google Scholar
[45] Hua Y, Cao B 2016 Int. J. Therm. Sci. 101 126
Google Scholar
[46] Hua Y, Cao B 2017 Appl. Therm. Eng. 111 1401
Google Scholar
[47] Hua Y, Cao B 2017 J. Phys. Chem. C 121 5293
Google Scholar
[48] Li H, Cao B 2018 Nanosc. Microsc. Therm. Eng. 23 10
Google Scholar
[49] Hua Y, Cao B 2016 Int. J. Heat Mass Transfer 92 995
Google Scholar
[50] Fujito K, Kubo S, Nagaoka H, Mochizuki T, Namita H, Nagao S 2009 J. Cryst. Growth 311 3011
Google Scholar
[51] Suihkonen S, Pimputkar S, Sintonen S, Tuomisto F 2017 Adv. Electron. Mater. 3 1600496
Google Scholar
下载:
计量
- 文章访问数: 10797
- PDF下载量: 408
- 被引次数: 0
