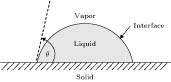
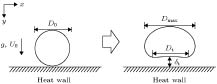
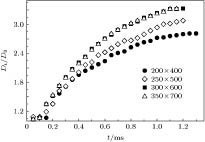
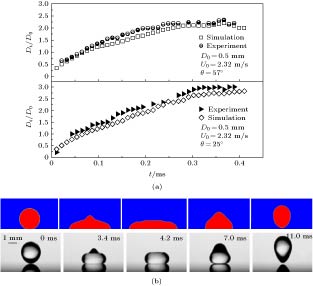
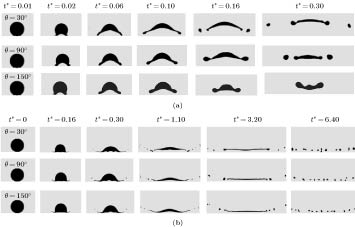
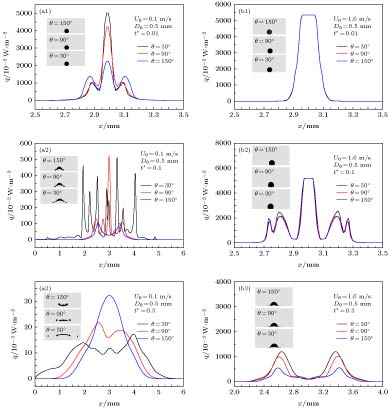
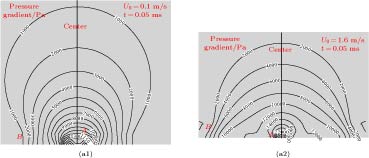
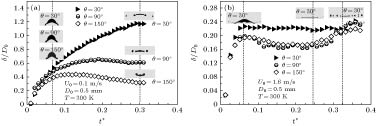
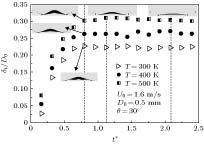
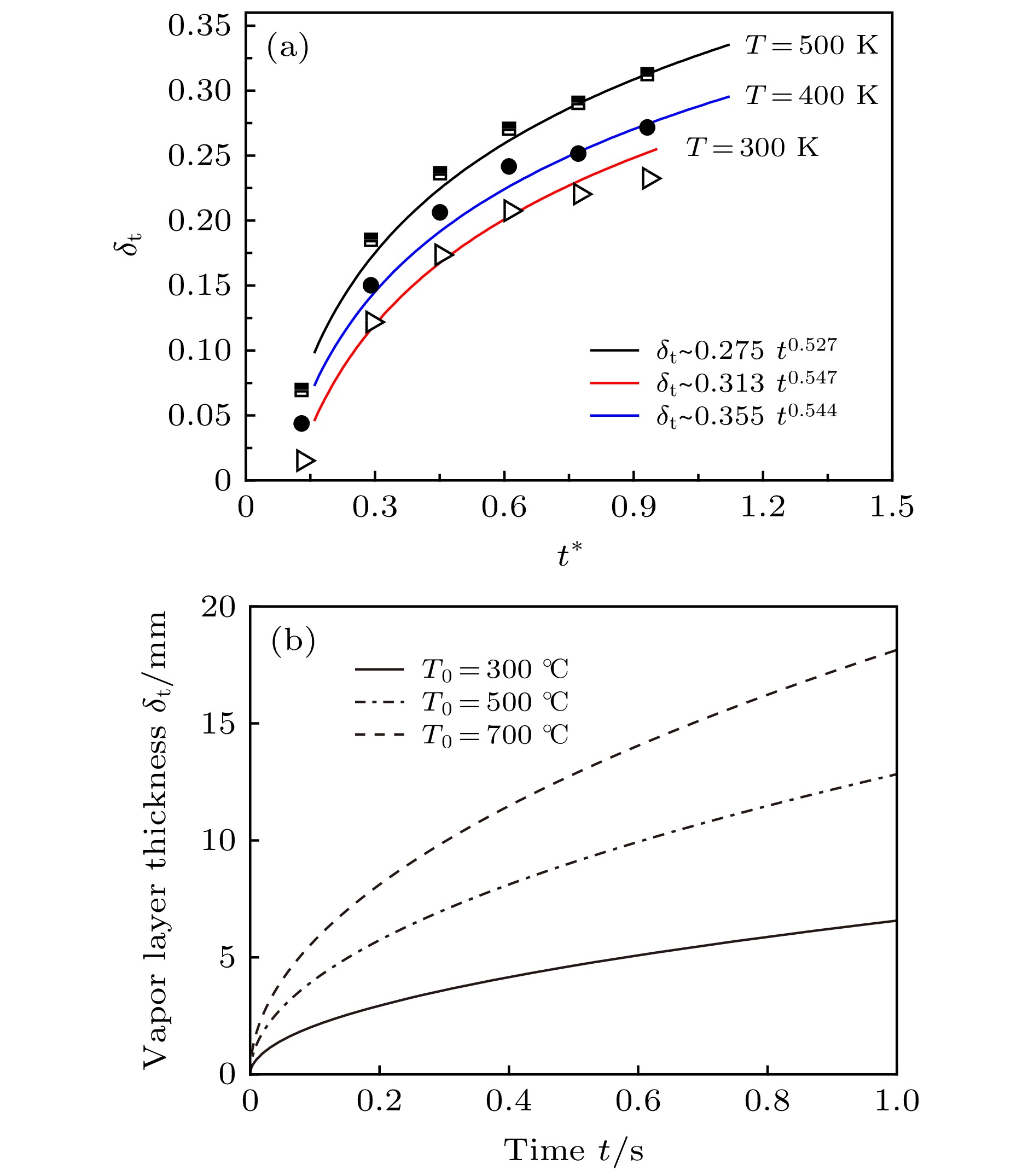
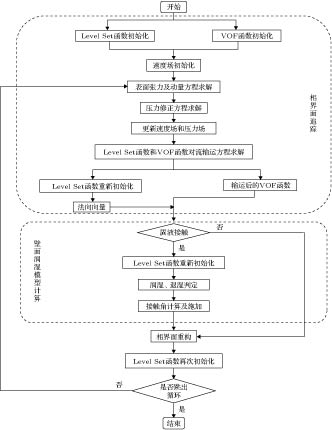
-
采用Level Set-VOF方法建立单液氮滴撞击壁面的数值模型, 探索壁面润湿性(30°—150°)、撞击速度(0.1和1.6 m/s)及壁面温度(300—500 K)对液滴撞壁演化过程中相变行为的影响, 并理论推导了气膜生长数学模型. 结果表明: 增强壁面润湿性、提高撞击速度有利于液滴沿径向铺展, 从而增大了换热面积并降低热阻, 使换热性能得到显著提升; 提高壁面温度增大了换热温差, 热流密度随之上升; 三相接触线处热阻较小导致边缘处热流密度高于中心处, 不同润湿壁面上热流分布的差异性因初始速度的增大而缩小, 呈现明显的速度效应; 在膜沸腾区, 传热过程主要集中在撞击初期, 气膜是主要换热热阻; 基于质量守恒和能量守恒建立气膜生长数值模型, 模型预测结果与本文模拟结果和其他研究结果非常吻合.
-
关键词:
- Leidenfrost /
- 液氮 /
- 液滴撞击 /
- Level Set模拟
The distinct physical properties of liquid nitrogen make liquid nitrogen spray cooling a promising technique in aerospace engineering, the electronic industry, superconductor cooling, cryobiology, etc. In-depth study of the dynamics and thermodynamic behavior of liquid nitrogen droplets impinging on the wall surface is helpful to understand the heat transfer mechanism of spray cooling technology with liquid nitrogen. Therefore, the mathematical model of single-liquid nitrogen droplet impacted solid surface is developed by Level Set-VOF method. The effects of wall wettability (30°－150°), initial velocity (0.1, 1.6 m/s) and wall temperature (300－500 K) on the phase change behavior during the evolution of droplets are investigated, and the mathematical model of film thickness is established. The results show that enhancing the wall wettability and increasing the impact speed facilitate the spreading of the droplets in the radial direction, thereby increasing the heat exchange area and reducing the thermal resistance. Ultimately, the heat exchange performance is significantly improved. Increasing the wall temperature results in an increase in the difference between temperatures of the solid surface and the liquid, thereby significantly increasing the wall heat flux density. The lower thermal resistance at the three-phase contact line results in a higher heat flux density at the edge than in the center; the difference among the heat flux distributions on different wetted walls decreases due to the increase of initial velocity, showing a significant velocity effect. In the film boiling region, the heat transfer process is mainly concentrated in the initial stage of impact, and the gas film is the main heat transfer resistance. Based on conservation of mass and energy, a numerical model of film thickness is developed in this paper. The model predictions are in good agreement with the simulation results of this paper and others.-
Keywords:
- Leidenfrost /
- liquid nitrogen /
- impingement /
- Level Set-VOF simulation
[1] Liang G T, Mudawar I 2017 Int. J. Heat Mass. Tran. 115 1174
Google Scholar
[2] Liang G T, Mudawar I 2017 Int. J. Heat Mass. Tran. 115 1206
Google Scholar
[3] Somasundaram S, Tay A A O 2014 Appl. Therm. Eng. 69 199
Google Scholar
[4] Liu X F, Xue R, Ruan Y X, Chen L, Zhang X Q, Hou Y 2017 Cryogenics 83 57
Google Scholar
[5] Xue R, Ruan Y X, Liu X F, Chen L, Zhang X Q, Hou Y, Chen S T 2018 Appl. Therm. Eng. 142 717
Google Scholar
[6] Kilgore R A, Dress D A 1984 Cryogenics 24 395
Google Scholar
[7] Tran T, Staat H J J, Susarrey-Arce A, Foertsch T C, van Houselt A, Gardeniers H J G E, Prosperetti A, Lohse D, Sun C 2013 Soft Matter. 9 3272
Google Scholar
[8] Celestini F, Kirstetter G 2012 Soft Matter. 8 5992
Google Scholar
[9] 叶学民, 李永康, 李春曦 2016 65 104704
Google Scholar
Ye X M, Li Y K, Li C X 2016 Acta Phys. Sin. 65 104704
Google Scholar
[10] Karl A, Frohn A 2000 Phys. Fluids. 12 785
Google Scholar
[11] Scheller B L, Bousfield D W 1995 AIChE J. 41 1357
Google Scholar
[12] 刘海龙, 沈学峰, 王睿, 曹宇, 王军锋 2018 力学学报 50 1024
Google Scholar
Liu H L, Shen X F, Wang R, Cao Y, Wang J F 2018 Acta Mech. Sin. 50 1024
Google Scholar
[13] 沈胜强, 张洁珊, 梁刚涛 2015 64 134704
Google Scholar
Shen S Q, Zhang J S, Liang G T 2015 Acta Phys. Sin. 64 134704
Google Scholar
[14] Šikalo Š, Wilhelm H D, Roisman I V, Jakirlić S, Tropea C 2005 Phys. Fluids 17 062103
Google Scholar
[15] 梁刚涛, 郭亚丽, 沈胜强 2013 62 184703
Google Scholar
Liang G T, Guo Y L, Shen S Q 2013 Acta Phys. Sin. 62 184703
Google Scholar
[16] Tran T, Staat H J J, Prosperetti A, Sun C, Lohse D 2012 Phys. Rev. Lett. 108 036101
Google Scholar
[17] 梁刚涛, 郭亚丽, 沈胜强 2013 62 024705
Google Scholar
Liang G T, Guo Y L, Shen S Q 2013 Acta Phys. Sin. 62 024705
Google Scholar
[18] Lee W H 1980 A Pressure Iteration Scheme for Two-Phase Flow Modeling (Washington: Hemisphere Publishing) pp407–432
[19] De Schepper S C K, Heynderickx G J, Marin G B 2009 Comput. Chem. Eng. 33 122
Google Scholar
[20] Alizadehdakhel A, Rahimi M, Alsairafi A A 2010 Int. Commun. Heat. Mass. 37 312
Google Scholar
[21] Hatta N, Fujimoto H, Kinoshita K, Takuda H 1997 J. Fluid. Eng. 119 692
Google Scholar
[22] Liang G T, Shen S Q, Guo Y L, Zhang J L 2016 Int. J. Heat Mass. Tran. 100 48
Google Scholar
[23] Chandra S, Aziz S D 1994 J. Heat. Trans. 116 999
Google Scholar
[24] 李大树, 仇性启, 郑志伟, 崔云静, 马培勇 2015 农业机械学报 46 294
Google Scholar
Li D S, Chou X Q, Zheng Z W, Cui Y J, Ma P Y 2015 Transactions of the Chinese Society for Agricultural Machinery 46 294
Google Scholar
[25] Kim H, Kim J 2010 J. Micromech. Microeng. 20 045008
Google Scholar
[26] 李大树, 仇性启, 崔云静, 郑志伟, 马培勇, 祁风雷 2014 农业机械学报 45 25
Google Scholar
Li D S, Chou X Q, Cui Y J, Zheng Z W, Ma P Y, Qi F L 2014 Transactions of the Chinese Society for Agricultural Machinery 45 25
Google Scholar
[27] Chaidron G, Soucemarianadin A, Attané P 1999 Am. Mineral. 84 1235
Google Scholar
[28] Breitenbach J, Roisman I V, Tropea C 2018 Exp. Fluids 59 55
Google Scholar
[29] Manzello S L, Yang J C 2002 P. Roy. Soc. A:-Math. Phys. 458 2417
Google Scholar
[30] 吴苏晨, 张程宾, 陈永平, 施明恒 2018 工程热 39 174
Wu S C, Zhang C B, Chen Y P, Shi M H 2018 J. Eng. Thermophys. 39 174
[31] Aussillous P, Quéré D 2001 Nature 411 924
Google Scholar
[32] Quéré D 2013 Annu. Rev. Fluid. Mech. 45 197
[33] Breitenbach J, Roisman I V, Tropea C 2017 Int. J. Heat. Mass. Tran. 110 34
Google Scholar
-
图 1 复合Level Set-VOF相界面追踪方法计算流程图
Fig. 1. Coupled Level Set-VOF phase interface tracking method calculation flow chart.
图 5 实验结果与模拟结果对比 (a)铺展因子随时间变化; (b)液滴形貌对比
Fig. 5. Comparison of experimental and simulated results: (a) Spreading factor changes with time; (b) comparison of droplet morphology evolution.
图 6 初始速度为(a) 0.1 m/s和(b) 1.6 m/s的液滴撞击不同润湿壁面的形貌演变(D0 = 0.5 mm)
Fig. 6. Morphology evolution of droplets impinging on different wetted walls with initial velocity (D0 = 0.5 mm): (a) 0.1 m/s; (b) 1.6 m/s.
图 7 初始速度为(a) 0.1 m/s和(b) 1.6 m/s的液滴撞击不同润湿壁面的热流分布
Fig. 7. Heat flux density distribution of droplets impinging on different wetted walls with initial velocity: (a) 0.1 m/s; (b) 1.6 m/s.
图 8 不同时刻的温度分布(θ = 30°, U0 = 0.1 m/s, Tw = 300 K)
Fig. 8. Temperature distribution at different times (θ = 30°, U0 = 0.1 m/s, Tw = 300 K).
图 9 不同速度的液滴撞壁后在0.05 ms时刻的压力分布
Fig. 9. Pressure distribution at 0.05 ms after droplets impact wall with different velocities.
图 10 初始速度为(a) 0.1 m/s和(b) 1.6 m/s液滴撞击不同润湿壁面的无量纲气膜厚度随无量纲时间的变化
Fig. 10. Dimensional film thickness with dimensionless time curve of droplets impinging on different wetted walls with initial velocity: (a) 0.1 m/s; (b) 1.6 m/s.
图 11 (a) 0.05 ms和(b) 1.6 ms时不同温度壁面上的热流密度分布
Fig. 11. Heat flux distribution on different temperature walls at (a) 0.05 ms and (b) 1.6 ms.
图 12 (a) 0.05 ms和(b) 1.6 ms不同温度壁面上的液滴温度分布
Fig. 12. Droplets temperature distribution on different temperature wall at (a) 0.05 ms and (b) 1.6 ms.
图 13 不同壁面温度上无量纲气膜厚度随无量纲时间的变化
Fig. 13. Curves of dimensionless film thickness with dimensionless time on different wall temperatures.
表 1 相关参数
Table 1. Related parameters.
参数 数值 液氮密度/kg·m–3 806.88 液氮表面张力系数/N·m–1 8.22 × 10–3 液氮黏度/Pa·s 160.08 × 10–6 液氮滴初始速度/m·s–1 0.1—1.6 液氮滴初始直径/mm 0.5 液氮滴初始温度/K 78 壁面温度/K 300—500 液氮潜热/kJ·kg–1 199.3 氮气导热系数/W·cm–1·K–1 0.002475 氮气黏度/Pa·s 17.54 × 10–6 氮气密度/kg·m–3 1.16 
下载: 导出CSV
-
[1] Liang G T, Mudawar I 2017 Int. J. Heat Mass. Tran. 115 1174
Google Scholar
[2] Liang G T, Mudawar I 2017 Int. J. Heat Mass. Tran. 115 1206
Google Scholar
[3] Somasundaram S, Tay A A O 2014 Appl. Therm. Eng. 69 199
Google Scholar
[4] Liu X F, Xue R, Ruan Y X, Chen L, Zhang X Q, Hou Y 2017 Cryogenics 83 57
Google Scholar
[5] Xue R, Ruan Y X, Liu X F, Chen L, Zhang X Q, Hou Y, Chen S T 2018 Appl. Therm. Eng. 142 717
Google Scholar
[6] Kilgore R A, Dress D A 1984 Cryogenics 24 395
Google Scholar
[7] Tran T, Staat H J J, Susarrey-Arce A, Foertsch T C, van Houselt A, Gardeniers H J G E, Prosperetti A, Lohse D, Sun C 2013 Soft Matter. 9 3272
Google Scholar
[8] Celestini F, Kirstetter G 2012 Soft Matter. 8 5992
Google Scholar
[9] 叶学民, 李永康, 李春曦 2016 65 104704
Google Scholar
Ye X M, Li Y K, Li C X 2016 Acta Phys. Sin. 65 104704
Google Scholar
[10] Karl A, Frohn A 2000 Phys. Fluids. 12 785
Google Scholar
[11] Scheller B L, Bousfield D W 1995 AIChE J. 41 1357
Google Scholar
[12] 刘海龙, 沈学峰, 王睿, 曹宇, 王军锋 2018 力学学报 50 1024
Google Scholar
Liu H L, Shen X F, Wang R, Cao Y, Wang J F 2018 Acta Mech. Sin. 50 1024
Google Scholar
[13] 沈胜强, 张洁珊, 梁刚涛 2015 64 134704
Google Scholar
Shen S Q, Zhang J S, Liang G T 2015 Acta Phys. Sin. 64 134704
Google Scholar
[14] Šikalo Š, Wilhelm H D, Roisman I V, Jakirlić S, Tropea C 2005 Phys. Fluids 17 062103
Google Scholar
[15] 梁刚涛, 郭亚丽, 沈胜强 2013 62 184703
Google Scholar
Liang G T, Guo Y L, Shen S Q 2013 Acta Phys. Sin. 62 184703
Google Scholar
[16] Tran T, Staat H J J, Prosperetti A, Sun C, Lohse D 2012 Phys. Rev. Lett. 108 036101
Google Scholar
[17] 梁刚涛, 郭亚丽, 沈胜强 2013 62 024705
Google Scholar
Liang G T, Guo Y L, Shen S Q 2013 Acta Phys. Sin. 62 024705
Google Scholar
[18] Lee W H 1980 A Pressure Iteration Scheme for Two-Phase Flow Modeling (Washington: Hemisphere Publishing) pp407–432
[19] De Schepper S C K, Heynderickx G J, Marin G B 2009 Comput. Chem. Eng. 33 122
Google Scholar
[20] Alizadehdakhel A, Rahimi M, Alsairafi A A 2010 Int. Commun. Heat. Mass. 37 312
Google Scholar
[21] Hatta N, Fujimoto H, Kinoshita K, Takuda H 1997 J. Fluid. Eng. 119 692
Google Scholar
[22] Liang G T, Shen S Q, Guo Y L, Zhang J L 2016 Int. J. Heat Mass. Tran. 100 48
Google Scholar
[23] Chandra S, Aziz S D 1994 J. Heat. Trans. 116 999
Google Scholar
[24] 李大树, 仇性启, 郑志伟, 崔云静, 马培勇 2015 农业机械学报 46 294
Google Scholar
Li D S, Chou X Q, Zheng Z W, Cui Y J, Ma P Y 2015 Transactions of the Chinese Society for Agricultural Machinery 46 294
Google Scholar
[25] Kim H, Kim J 2010 J. Micromech. Microeng. 20 045008
Google Scholar
[26] 李大树, 仇性启, 崔云静, 郑志伟, 马培勇, 祁风雷 2014 农业机械学报 45 25
Google Scholar
Li D S, Chou X Q, Cui Y J, Zheng Z W, Ma P Y, Qi F L 2014 Transactions of the Chinese Society for Agricultural Machinery 45 25
Google Scholar
[27] Chaidron G, Soucemarianadin A, Attané P 1999 Am. Mineral. 84 1235
Google Scholar
[28] Breitenbach J, Roisman I V, Tropea C 2018 Exp. Fluids 59 55
Google Scholar
[29] Manzello S L, Yang J C 2002 P. Roy. Soc. A:-Math. Phys. 458 2417
Google Scholar
[30] 吴苏晨, 张程宾, 陈永平, 施明恒 2018 工程热 39 174
Wu S C, Zhang C B, Chen Y P, Shi M H 2018 J. Eng. Thermophys. 39 174
[31] Aussillous P, Quéré D 2001 Nature 411 924
Google Scholar
[32] Quéré D 2013 Annu. Rev. Fluid. Mech. 45 197
[33] Breitenbach J, Roisman I V, Tropea C 2017 Int. J. Heat. Mass. Tran. 110 34
Google Scholar
下载:
计量
- 文章访问数: 8639
- PDF下载量: 134
- 被引次数: 0
