-
改善TiAl基合金的高温抗氧化性, 对于拓展其应用领域具有重要意义. 本文采用基于密度泛函理论的第一性原理方法, 从原子平均形成能、弹性常数、间隙O原子的形成能、Ti空位和Al空位的形成能等方面研究了Si和Y替位双掺杂对γ-TiAl基合金抗氧化性的影响. 结果显示, 各个双掺杂γ-TiAl体系的原子平均形成能均为负值, 表明体系具有能量稳定性, 理论预报它们均可以由实验制备, 其中大多数体系的弹性常数满足力学稳定性判据. 对于满足力学稳定性条件的体系, 综合间隙O原子的形成能、Ti空位和Al空位形成能的分析结果, 揭示Si和Y均替位Ti生成体系Ti6SiYAl8对改善抗氧化性效果明显; Y替位Ti且Si替位Al生成体系Ti7YAl7Si, Si替位Ti且Y替位Al生成体系Ti7SiAl7Y对改善抗氧化性具有不确定性; Si和Y均替位Al生成体系Ti8Al6SiY不利于改善抗氧化性.Improving the oxidation resistance of TiAl-based alloys at high temperature has great significance for expanding their application fields. Adding ternary and quaternary elements is one of the effective ways to solve the oxidation problem of this kind of alloys materials. The first-principles method based on density functional theory was used to study the Si and Y substitution co-doping effects on the oxidation resistance of γ-TiAl based alloys from the aspects of atomic average formation energy and elastic constant of system, as well as the formation energies of interstitial O atom, Ti vacancy and Al vacancy in the system. The results indicate that the atomic average formation energies of the Si and Y dual-doped systems are all negative, which imply they possess energy stability and can be prepared by experiments. In addition, the elastic constants of most Si and Y substitution co-doping γ-TiAl systems satisfy the mechanical stability criterion. For the mechanical stable systems, the analysis results about the formation energies of the interstitial O atom, Ti vacancy and Al vacancy reveal that the Ti6SiYAl8 series, in which both Si and Y substitute Ti, have obvious promotion effect on the improvement about oxidation resistance; system Ti7YAl7Si, in which Y substitutes Ti and Si substitutes Al, and system Ti7SiAl7Y, in which Si substitutes Ti and Y substitutes Al, have uncertain influence on improving oxidation resistance; system Ti8Al6SiY, in which both Si and Y substitute Al, is harmful to the improvement about oxidation resistance of the γ-TiAl based alloys. Therefore, the preparation conditions should be controlled moderately so that both Si and Y substitute Ti at the same time to form a large proportion configurations of Ti6SiYAl8 series in the materials. In these configurations, the outward diffusion of Ti atoms and the inward diffusion of interstitial O atoms are suppressed, meanwhile the outward diffusion of the Al atoms is facilitated. In this way, the production of α-Al2O3 is promoted and that of TiO2 is weakened on the surface of co-doping γ-TiAl based alloys. Thus, a scale rich in α-Al2O3, i. e., a continuous, dense, and protective oxide scale can be grown on the surface of Si and Y substitution co-doping γ-TiAl alloys.
-
Keywords:
- co-doping γ-TiAl /
- stability /
- oxidation resistance /
- first-principles
[1] Castillo R M, Nó M L, Jiménez J A, Ruano O A, San Juan J 2016 Acta Mater. 103 46
Google Scholar
[2] Kim S W, Hong J K, Na Y S, Yeom J T, Kim S E 2014 Mater. Des. 54 814
Google Scholar
[3] Chen G, Peng Y B, Zheng G, Qi Z X, Wang M Z, Yu H C, Dong C L, Liu C T 2016 Nature Mater. 15 876
Google Scholar
[4] Pflumm R, Friedle S, Schütze M 2015 Intermetallics 56 1
Google Scholar
[5] Yoshihara M, Kim Y W 2005 Intermetallics 13 952
Google Scholar
[6] Kuranishi T, Habazaki H, Konno H 2005 Surf. Coat. Technol. 200 2438
Google Scholar
[7] 汤守巧, 曲寿江, 冯艾寒, 冯聪, 崔扣彪, 沈军 2017 稀有金属 41 81
Tang S Q, Qu S J, Feng A H, Feng C, Cui K B, Shen J 2017 Rare Metals 41 81
[8] Hu H, Wu X, Wang R, Li W, Liu Q 2016 J. Alloys Compd. 658 689
Google Scholar
[9] Brotzu A, Felli F, Pilone D 2013 Intermetallics 43 131
Google Scholar
[10] 平发平, 胡青苗, 杨锐 2013 金属学报 29 385
Ping F P, Hu Q M, Yang R 2013 Acta Metall. Sin. 29 385
[11] 朱绍祥, 王清江, 刘建荣, 刘羽寅, 杨锐 2010 中国有色金属学报 20 138
Zhu S X, Wang Q J, Liu J R, Liu Y Y, Yang R 2010 Chin. J. Nonferr. Met. 20 138
[12] Izumi T, Yoshioka T, Hayashi S, Narita T 2005 Intermetallics 13 694
Google Scholar
[13] 刘杰, 薛祥义, 杨劼人 2015 稀有金属材料与工程 44 1942
Liu J, Xue X Y, Yang J R 2015 Rare Metal Mat. Eng. 44 1942
[14] Lin J P, Zhao L L, Li G Y, Zhang L Q, Song X P, Ye F, Chen G L 2011 Intermetallics 19 136
[15] 杨忠波, 赵文金, 程竹青, 邱军, 张海, 卓洪 2017 金属学报 53 49
Yang Z B, Zhao W J, Cheng Z Q, Qiu J, Zhang H, Zhuo H 2017 Acta Metall. Sin. 53 49
[16] Zhao L, Zhang Y, Zhu Y, Lin J, Ren Z 2016 Mater. High Temp. 33 234
Google Scholar
[17] Zhao L L, Li G Y, Zhang L Q, Lin J P, Song X P, Ye F, Chen G L 2010 Intermetallics 18 1586
Google Scholar
[18] 周玉俊, 周雪锋, 陈光 2015 热加工工艺 44 93
Zhou Y J, Zhou X F, Chen G 2015 Hot Working Technol. 44 93
[19] 王艳晶, 宋玫锦, 王继杰, 杜兴蒿 2014 稀有金属材料与工程 43 1697
Wang Y J, Song M J, Wang J J, Du X H 2014 Rare Metal Mat. Eng. 43 1697
[20] 董利民, 崔玉友, 杨锐, 王福会 2004 金属学报 40 383
Google Scholar
Dong L M, Cui Y Y, Yang R, Wang F H 2004 Acta Metall. Sin. 40 383
Google Scholar
[21] 肖伟豪, 张亮, 姜惠仁 2004 北京航空航天大学学报 32 365
Google Scholar
Xiao W H, Zhang L, Jiang H R 2004 J. Beijing Univ. Aeron. Astron. 32 365
Google Scholar
[22] 张国英, 刘贵立 2011 现代电子理论在材料设计中的应用(1) (北京: 科学出版社) 第164−167页
Zhang G Y, Liu G L 2011 Application of Modern Electronic Theory in Material Design (Vol. 1) (Beijing: Science Press) pp179−182 (in Chinese)
[23] 吴红丽, 张伟, 宫声凯 2008 化学学报 66 1669
Google Scholar
Wu H L, Zhang W, Gong S K 2008 Acta Chim. Sin. 66 1669
Google Scholar
[24] 陈国良, 林均品 1999有序金属间化合物结构材料物理金属学基础(1)(北京: 冶金工业出版社)第285页
Chen G L, Lin J P 1999 Physicalmetallurgy Basis of Ordered Intermetallic Compound Structure Materials (Vol. 1) (Beijing: Metallurgical Industry Press) p285(in Chinese)
[25] 李虹, 王绍青, 叶恒强 2009 58 224
Google Scholar
Li H, Wang S Q, Ye H Q 2009 Acta Phys. Sin. 58 224
Google Scholar
[26] Segall M D, Lindan P J D, Probert M J 2002 J. Phys.: Condens. Matter 14 2717
Google Scholar
[27] Clark S J, Segall M D, Pickard C J, Hasnip P J, Payne M C 2005 Z. Kristallogr. 220 567
[28] 宋庆功, 姜恩永 2008 57 1823
Google Scholar
Song Q G, Jiang E Y 2008 Acta Phys. Sin. 57 1823
Google Scholar
[29] Born M, Huang K 1954 Dynamical Theory of Crystal Lattices (Oxford: Clarendon Press) pp113－117
-
图 1 γ-TiAl晶胞结构模型 (a) L10型面心四方结构单元; (b)最小结构单元; (c) Sp0(Ti8Al8)结构单元
Fig. 1. Structure models of γ-TiAl: (a) The L10 face-center tetragonal unit cell; (b) the least tetragonal unit cell; (c) the unit of Sp0(Ti8Al8).
图 2 包含O原子的γ-TiAl晶胞结构模型 (a) Sp0-Oa; (b) Sp0-Ob; (c) Sp0-Oc; (d) Sp0-Od
Fig. 2. Structure models of γ-TiAl including O (Ti8Al8O): (a) Sp0-Oa; (b) Sp0-Ob; (c) Sp0-Oc; (d) Sp0-Od
图 3 包含Ti空位或Al空位的γ-TiAl典型结构模型 (a) Sp0-□Ti (Ti7□Al8); (b) Sp0-□Al (Ti8Al7□)
Fig. 3. Typical structure models of γ-TiAl including Ti or Al vacancy: (a) Sp0-□Ti (Ti7□Al8); (b) Sp0-□Al (Ti8Al7□)
图 4 Si和Y替位双掺杂γ-TiAl体系的典型结构模型 (a) Sd1x (Ti6SiYAl8); (b) Sd2 (Ti7YAl7Si); (c) Sd3 (Ti7SiAl7Y); (d) Sd4(Ti8Al6SiY)
Fig. 4. Typical structure models of Si and Y co-doping γ-TiAl: (a) Sd1x (Ti6SiYAl8); (b) Sd2 (Ti7YAl7Si); (c)Sd3 (Ti7SiAl7Y); (d) Sd4(Ti8Al6SiY)
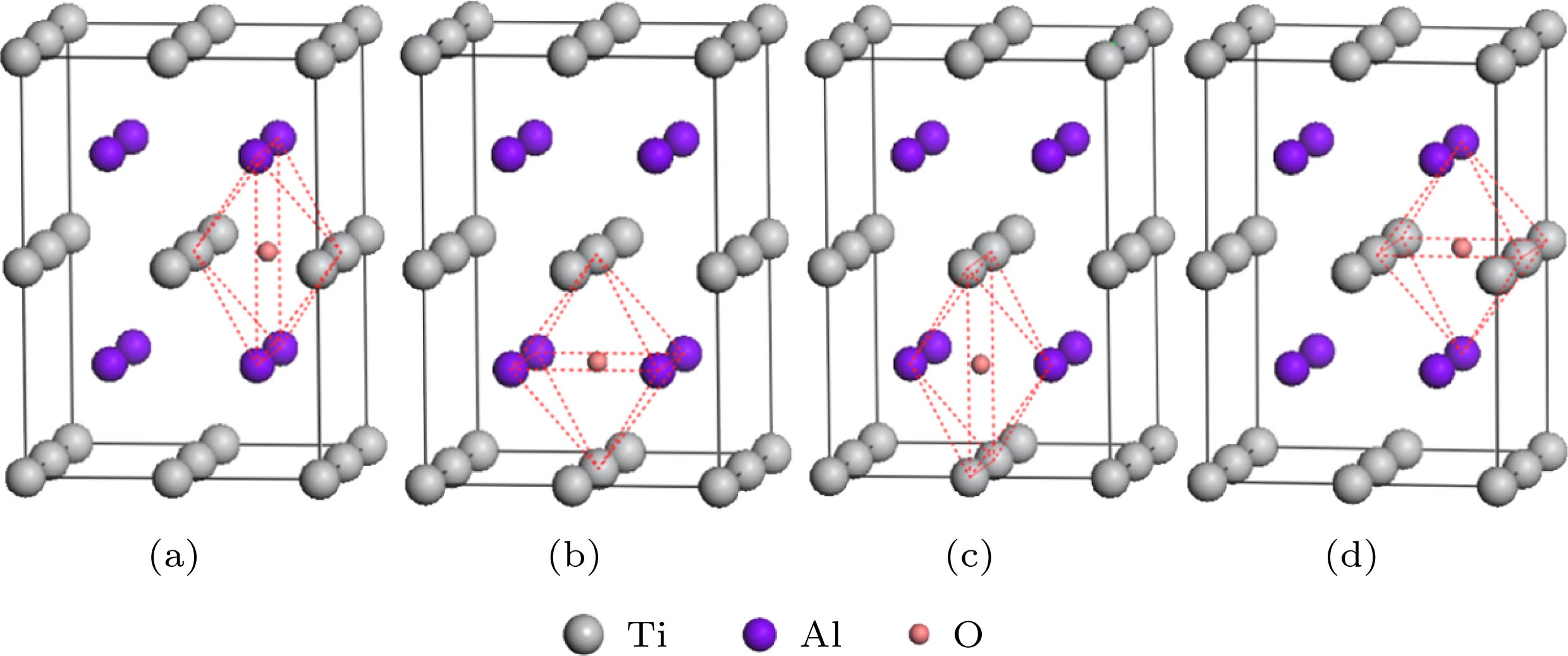
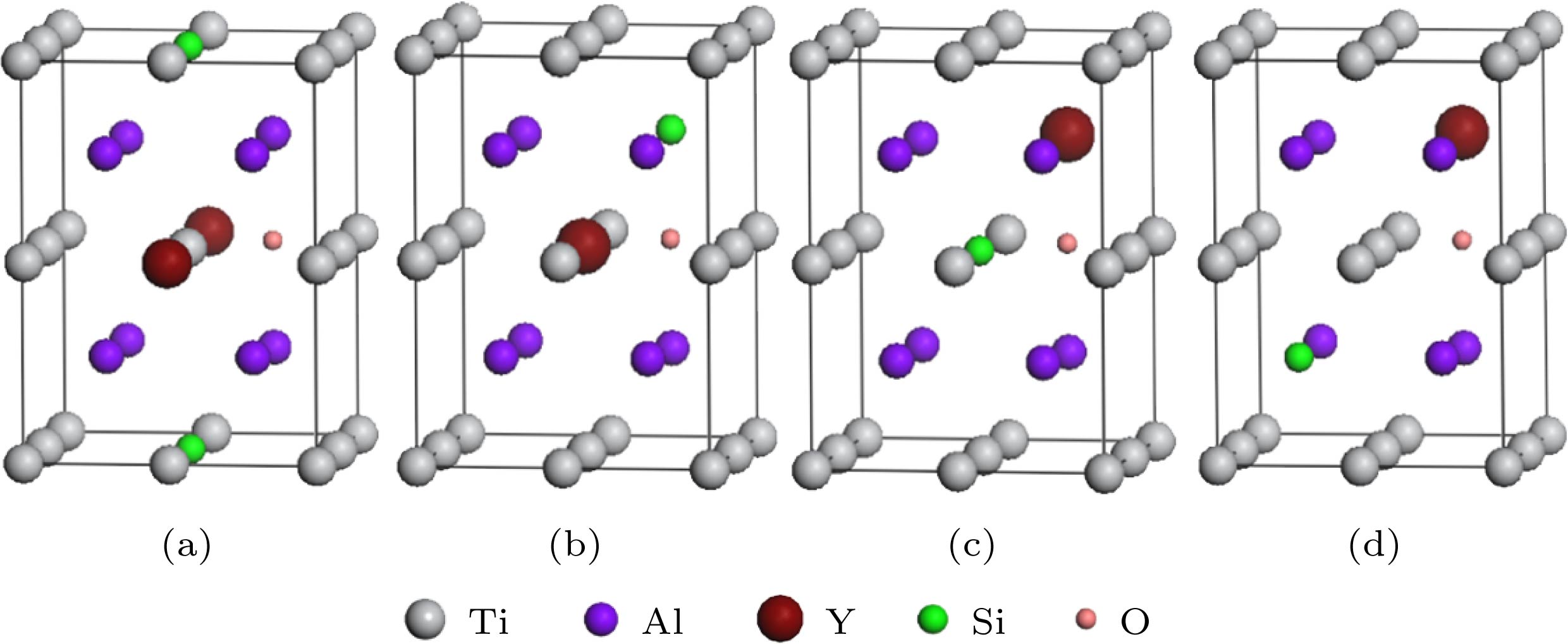
图 5 Si和Y替位双掺杂γ-TiAl含氧体系的典型结构模型 (a) Sd1x-Od (Ti6SiYAl8O); (b) Sd2x-Od (Ti7YAl7SiO); (c) Sd3x-Od (Ti7SiAl7YO); (d) Sd4x-Od(Ti8Al6SiYO)
Fig. 5. Typical structure models of Si and Y co-doping γ-TiAl including O: (a) Sd1x-Od (Ti6SiYAl8O); (b) Sd2x-Od (Ti7YAl7SiO); (c) Sd3x-Od (Ti7SiAl7YO); (d) Sd4x-Od(Ti8Al6SiYO)
图 6 Si和Y替位双掺杂γ-TiAl含空位体系的典型结构模型 (a) Sd1x-□Ti (Ti5SiY□Al8); (b) Sd2x-□Ti (Ti6Y□Al7Si); (c) Sd3x-□Ti(Ti6Si□Al7Y); (d) Sd4x-□Ti(Ti7□Al6SiY); (e) Sd1x-□Al (Ti6SiYAl7□); (f) Sd2x-□Al (Ti7YAl6Si□); (g) Sd3x-□Al(Ti7SiAl6Y□); (h) Sd4x-□Al(Ti8Al5SiY□)
Fig. 6. Typical structure models of Si and Y co-doping γ-TiAl including vacancy: (a)Sd1x-□Ti (Ti5SiY□Al8); (b) Sd2x-□Ti (Ti6Y□Al7Si); (c) Sd3x-□Ti(Ti6Si□Al7Y); (d) Sd4x-□Ti(Ti7□Al6SiY); (e) Sd1x-□Al (Ti6SiYAl7□); (f) Sd2x-□Al (Ti7YAl6Si□); (g) Sd3x-□Al(Ti7SiAl6Y□); (h) Sd4x-□Al(Ti8Al5SiY□)
图 7 Si和Y替位双掺杂γ-TiAl含氧体系中间隙O原子的形成能
Fig. 7. Formation energies of interstitial O atoms in the Si and Y co-doping γ-TiAl systems.
图 8 Si和Y双掺杂γ-TiAl含空位体系中Ti空位和Al空位的形成能
Fig. 8. Formation energies of Ti and Al vacancies in Si and Y co-doping γ-TiAl systems.
表 1 纯γ-TiAl体系与Si和Y替位双掺杂γ-TiAl体系的能量性质
Table 1. Energy properties of pure γ-TiAl and Si and Y co-doping γ-TiAl systems.
体系 能量性质 Et /eV E f/eV Sp0 –13283.2619 –0.3579 Sd17 –10375.5782 –0.2448 Sd19 –10375.3125 –0.2282 Sd2 –11923.1797 –0.3078 Sd3 –11921.0891 –0.1771 Sd4 –13468.5526 –0.2315 
下载: 导出CSV
表 2 纯γ-TiAl体系与Si和Y替位双掺杂γ-TiAl体系中四方晶系的弹性常数
Table 2. Elastic constants of tetragonal systems in pure γ-TiAl and Si and Y co-doping γ-TiAl systems.
体系 C11/GPa C12/GPa C13/GPa C33/GPa C44/GPa C66/GPa Sp0 232.2438 39.8470 69.3592 196.4413 112.3486 44.8147 Sd11 130.8671 75.6434 87.7505 134.2535 70.0558 46.6357 Sd13 136.8296 75.1812 86.9261 134.1158 64.9601 49.0225 Sd14 180.5075 43.1529 81.6884 138.5193 73.0642 19.7364 Sd15 136.6952 74.6364 84.6822 128.5797 67.3934 47.4253 Sd16 160.1225 90.0601 87.6092 137.2518 106.8941 43.0277 Sd19 130.8850 74.3862 78.7846 125.4646 68.9906 56.7747 Sd110 132.4566 95.9060 -40.8881 351.4524 24.8135 42.5965 Sd4 174.4526 59.4503 76.4982 151.9303 82.9168 12.5223
下载: 导出CSV
表 3 Si和Y替位双掺杂γ-TiAl体系Sd17, Sd2和Sd3的弹性常数
Table 3. Elastic constants of Si and Y co-doping γ-TiAl systems Sd17, Sd2 and Sd3.
体系 C11/GPa C12/GPa C13/GPa C15/GPa C22/GPa C23/GPa C25/GPa Sd17 174.0489 51.0128 78.8477 – 178.6799 73.6936 – Sd2 177.5408 69.1504 69.7969 –3.6774 172.7134 65.2400 –1.6610 Sd3 158.9597 58.6004 72.7099 –10.1161 160.3606 73.2300 –0.1101 体系 C33/GPa C35/GPa C44/GPa C46/GPa C55/GPa C66/GPa Sd17 117.2748 – 59.8220 – 64.1452 10.4758 Sd2 170.8100 –1.4732 77.8416 –1.2719 78.4798 38.6156 Sd3 139.6061 –1.4377 67.2411 0.7657 67.6317 26.4129
下载: 导出CSV
表 4 纯γ-TiAl体系及其含氧体系的能量性质
Table 4. Energy properties of pure γ-TiAl and the systems including O.
体系 能量性质 Et /eV E f /eV Sp0 –13283.2619 –0.3579 Sp0-Oa –13718.1429 –0.0716 Sp0-Ob –13720.0413 –0.1832 Sp0-Oc –13720.6086 –0.2166 Sp0-Od –13720.9863 –0.2388
下载: 导出CSV
表 5 Si和Y替位双掺杂γ-TiAl含氧体系中间隙O原子的形成能
Table 5. Formation energies of interstitial O atoms in Si and Y co-doping γ-TiAl systems.
体系 能量性质 Et(SdO)/eV Et (Sd)/eV E f(O)/eV Sp0-Od –13720.9863 –13283.2619 1.6665 Sd11-Od –10812.9929 –10375.4180 1.8160 Sd13-Od –10813.0355 –10375.3811 1.7365 Sd14-Od –10813.1059 –10375.4175 1.7025 Sd15-Od –10813.0129 –10375.3988 1.7768 Sd16-Od –10813.0989 –10375.3983 1.6903 Sd17-Od –10813.1600 –10375.5782 1.8091 Sd19-Od –10813.0084 –10375.3125 1.6950 Sd110-Od –10813.0341 –10375.3121 1.6689 Sd21-Od –12361.2419 –11923.1797 1.3287 Sd22-Od –12361.1930 –11923.1797 1.3776 Sd23-Od –12361.2834 –11923.1797 1.2872 Sd31-Od –12359.6285 –11921.0891 0.8515 Sd32-Od –12360.0430 –11921.0891 0.4370 Sd33-Od –12359.9518 –11921.0891 0.5282 Sd41-Od –13907.6183 –13468.5526 0.3252 Sd42-Od –13906.9550 –13468.5526 0.9885 Sd43-Od –13906.7798 –13468.5526 1.1637
下载: 导出CSV
表 6 Si和Y双掺杂γ-TiAl含空位体系中Ti空位和Al空位的形成能
Table 6. Formation energies of Ti and Al vacancies in Si and Y co-doping γ-TiAl systems.
体系 Et (Sd)/eV Et (Sd□Al)/eV Et (Sd□Ti)/eV E f (□Al)/eV E f (□Ti)/eV Sp0-□ –13283.2619 –13224.0362 –11678.3061 2.6764 1.8132 Sd11-□ –10375.4180 –10317.9824 –8770.2288 0.8863 2.0466 Sd13-□ –10375.3811 –10317.9645 –8770.1941 0.8673 2.0444 Sd14-□ –10375.4175 –10317.9637 –8770.2884 0.9045 1.9865 Sd15-□ –10375.3988 –10317.9620 –8770.2113 0.8875 2.0449 Sd16-□ –10375.3983 –10317.9032 –8770.2649 0.9098 1.9908 Sd17-□ –10375.5782 –10317.4973 –8770.0900 1.5316 2.3456 Sd19-□ –10375.3125 –10318.4246 –8770.2557 0.3386 1.9143 Sd110-□ –10375.3121 –10318.0212 –8770.2609 0.7416 1.9086 Sd2-□ –11923.1797 –11865.3646 –10317.9810 1.2658 2.0561 Sd3-□ –11921.0891 –11865.3095 –10317.6100 –0.7697 0.3365 Sd4-□ –13468.5526 –13409.4369 –11865.3562 2.5664 0.0538
下载: 导出CSV
-
[1] Castillo R M, Nó M L, Jiménez J A, Ruano O A, San Juan J 2016 Acta Mater. 103 46
Google Scholar
[2] Kim S W, Hong J K, Na Y S, Yeom J T, Kim S E 2014 Mater. Des. 54 814
Google Scholar
[3] Chen G, Peng Y B, Zheng G, Qi Z X, Wang M Z, Yu H C, Dong C L, Liu C T 2016 Nature Mater. 15 876
Google Scholar
[4] Pflumm R, Friedle S, Schütze M 2015 Intermetallics 56 1
Google Scholar
[5] Yoshihara M, Kim Y W 2005 Intermetallics 13 952
Google Scholar
[6] Kuranishi T, Habazaki H, Konno H 2005 Surf. Coat. Technol. 200 2438
Google Scholar
[7] 汤守巧, 曲寿江, 冯艾寒, 冯聪, 崔扣彪, 沈军 2017 稀有金属 41 81
Tang S Q, Qu S J, Feng A H, Feng C, Cui K B, Shen J 2017 Rare Metals 41 81
[8] Hu H, Wu X, Wang R, Li W, Liu Q 2016 J. Alloys Compd. 658 689
Google Scholar
[9] Brotzu A, Felli F, Pilone D 2013 Intermetallics 43 131
Google Scholar
[10] 平发平, 胡青苗, 杨锐 2013 金属学报 29 385
Ping F P, Hu Q M, Yang R 2013 Acta Metall. Sin. 29 385
[11] 朱绍祥, 王清江, 刘建荣, 刘羽寅, 杨锐 2010 中国有色金属学报 20 138
Zhu S X, Wang Q J, Liu J R, Liu Y Y, Yang R 2010 Chin. J. Nonferr. Met. 20 138
[12] Izumi T, Yoshioka T, Hayashi S, Narita T 2005 Intermetallics 13 694
Google Scholar
[13] 刘杰, 薛祥义, 杨劼人 2015 稀有金属材料与工程 44 1942
Liu J, Xue X Y, Yang J R 2015 Rare Metal Mat. Eng. 44 1942
[14] Lin J P, Zhao L L, Li G Y, Zhang L Q, Song X P, Ye F, Chen G L 2011 Intermetallics 19 136
[15] 杨忠波, 赵文金, 程竹青, 邱军, 张海, 卓洪 2017 金属学报 53 49
Yang Z B, Zhao W J, Cheng Z Q, Qiu J, Zhang H, Zhuo H 2017 Acta Metall. Sin. 53 49
[16] Zhao L, Zhang Y, Zhu Y, Lin J, Ren Z 2016 Mater. High Temp. 33 234
Google Scholar
[17] Zhao L L, Li G Y, Zhang L Q, Lin J P, Song X P, Ye F, Chen G L 2010 Intermetallics 18 1586
Google Scholar
[18] 周玉俊, 周雪锋, 陈光 2015 热加工工艺 44 93
Zhou Y J, Zhou X F, Chen G 2015 Hot Working Technol. 44 93
[19] 王艳晶, 宋玫锦, 王继杰, 杜兴蒿 2014 稀有金属材料与工程 43 1697
Wang Y J, Song M J, Wang J J, Du X H 2014 Rare Metal Mat. Eng. 43 1697
[20] 董利民, 崔玉友, 杨锐, 王福会 2004 金属学报 40 383
Google Scholar
Dong L M, Cui Y Y, Yang R, Wang F H 2004 Acta Metall. Sin. 40 383
Google Scholar
[21] 肖伟豪, 张亮, 姜惠仁 2004 北京航空航天大学学报 32 365
Google Scholar
Xiao W H, Zhang L, Jiang H R 2004 J. Beijing Univ. Aeron. Astron. 32 365
Google Scholar
[22] 张国英, 刘贵立 2011 现代电子理论在材料设计中的应用(1) (北京: 科学出版社) 第164−167页
Zhang G Y, Liu G L 2011 Application of Modern Electronic Theory in Material Design (Vol. 1) (Beijing: Science Press) pp179−182 (in Chinese)
[23] 吴红丽, 张伟, 宫声凯 2008 化学学报 66 1669
Google Scholar
Wu H L, Zhang W, Gong S K 2008 Acta Chim. Sin. 66 1669
Google Scholar
[24] 陈国良, 林均品 1999有序金属间化合物结构材料物理金属学基础(1)(北京: 冶金工业出版社)第285页
Chen G L, Lin J P 1999 Physicalmetallurgy Basis of Ordered Intermetallic Compound Structure Materials (Vol. 1) (Beijing: Metallurgical Industry Press) p285(in Chinese)
[25] 李虹, 王绍青, 叶恒强 2009 58 224
Google Scholar
Li H, Wang S Q, Ye H Q 2009 Acta Phys. Sin. 58 224
Google Scholar
[26] Segall M D, Lindan P J D, Probert M J 2002 J. Phys.: Condens. Matter 14 2717
Google Scholar
[27] Clark S J, Segall M D, Pickard C J, Hasnip P J, Payne M C 2005 Z. Kristallogr. 220 567
[28] 宋庆功, 姜恩永 2008 57 1823
Google Scholar
Song Q G, Jiang E Y 2008 Acta Phys. Sin. 57 1823
Google Scholar
[29] Born M, Huang K 1954 Dynamical Theory of Crystal Lattices (Oxford: Clarendon Press) pp113－117
下载:
计量
- 文章访问数: 8941
- PDF下载量: 72
- 被引次数: 0
