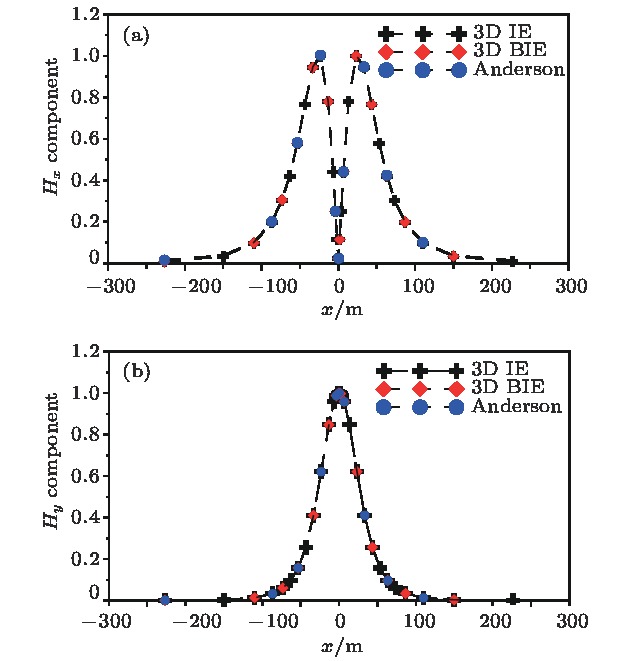
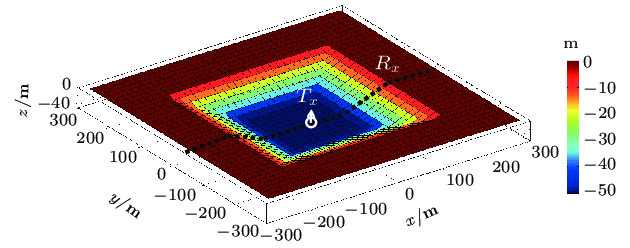
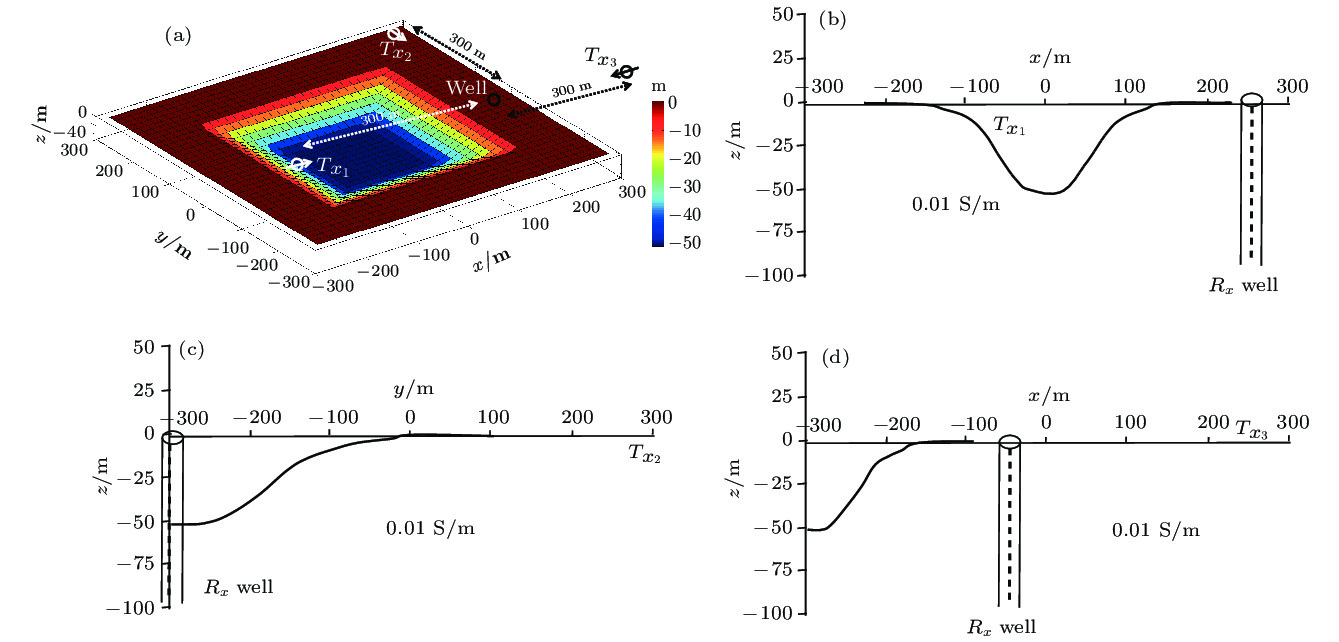
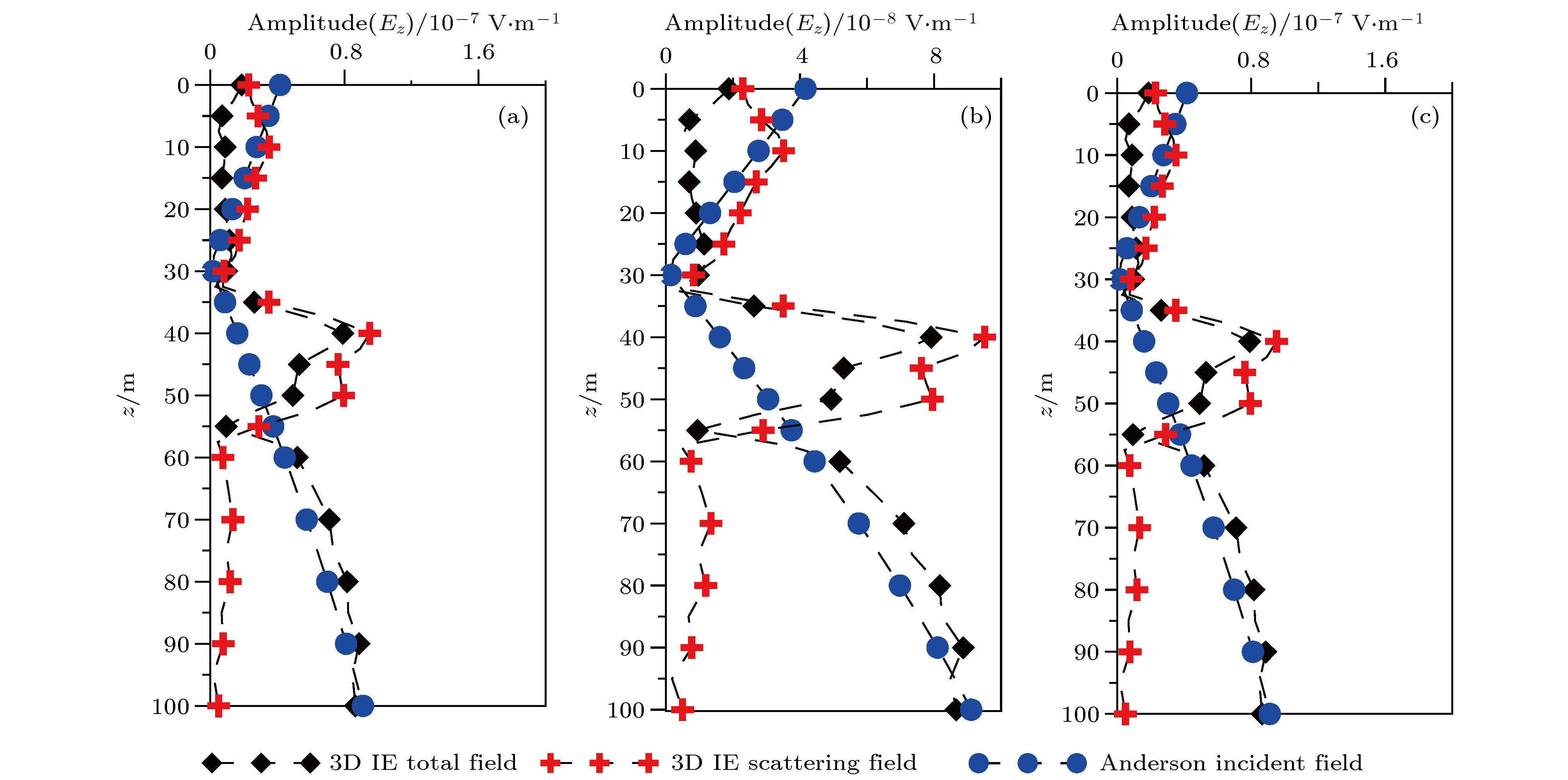
-
井筒电磁法作为一种高效的地球物理勘探技术特别适合我国地形复杂地区(沙漠、高山等)的油气资源勘探. 地形起伏区域对井筒电磁响应的观测具有严重影响, 但到目前为止人们对三维井筒电磁地形效应特征的研究十分有限. 本文提出基于区域划分的积分方程法模拟带地形频率域井筒电磁系统响应, 与基于偏微分方程的有限差分、有限单元法相比, 该方法能更高效地模拟地形响应. 首先根据地形起伏情况定义感应数, 将地形条件下目标体的井筒电磁场模拟区域划分为参考模型、背景介质及目标体介质分布子区域, 针对各子区域的模拟计算特点, 配置Anderson算法、稳定型双共轭梯度-快速傅里叶积分方程算法, 从而获得三维地形频率域井筒电磁场响应. 通过将计算结果与半空间模型的Anderson算法解析解、带山谷地形模型的其他已发表的三维边界积分方程结果进行对比, 检验了本文算法的精度及高效性. 最后, 系统分析了山谷地形对井筒电磁地井观测系统电磁场响应的影响特征. 本文研究结果对三维井筒电磁地形效应的识别和校正具有指导意义.As an efficient geophysical exploration technology, well electromagnetic method is particularly applicable to oil and gas exploration in China's complex terrain areas (deserts, mountains, etc.). A serious influence of topographic relief area on the electromagnetic response of well is inevitable but challenging. To the best of our knowledge, there is no literature on modeling the electromagnetic response of three-dimensional (3D) topography with well electromagnetic method. Based on the domain decomposition, an integral equation method is presented to simulate the electromagnetic response of 3D topography in frequency domain via the well electromagnetic method. Compared with the finite difference and finite element method based on partial differential equation, this method is very efficient in simulating topographic response without huge computation or truncation boundary error accumulation or special boundary condition requirements. Firstly, an induction coefficient is defined according to the topographic relief situation. Then the computational domain consisting of the target body, background medium and 3D topography is divided into reference model, background medium and the distribution of target body medium area. According to the characteristics of each sub-region, Anderson algorithm is an analytic solution based on Gaussian filtering, which is used to provide the primary field from the excited sources in surface. And then, the stable double conjugate gradient-fast Fourier transform is incorporated into integral equation algorithm to obtain the fast 3D terrain shaft frequency domain electromagnetic responses. By comparing the calculation results using the new algorithm presented in this paper with the analytical solutions of Anderson algorithm for half-space model with surface electromagnetic method, the precision and the efficiency of this new algorithm are demonstrated. And the ability to model the electromagnetic responses of 3D topography is shown by comparing with the published results of 3D boundary integral equation. Thus, the high accuracy and high efficiency of the new algorithm presented in this paper are validated. Finally, the influence of 3D valley topography on electromagnetic field response of surface to borehole electromagnetic (SBEM) observation system is presented and analyzed. It is observed that the response of SBEM is seriously disturbed by the field of 3D valley topography which is necessarily removed. The research results presented in this paper are of significance for guiding the identification and correction of electromagnetic topographic effect from 3D SBEM.
-
Keywords:
- domain decomposition based integral equation /
- response of 3-dimensional topography /
- well electromagnetic /
- surface to borehole electromagnetic
[1] Augustin A, Kennedy W, Morrison H 1989 Geophysics 54 90
Google Scholar
[2] 李静和, 何展翔, 吕玉增 2011 工程地球 8 303
Google Scholar
Li J H, He Z X, Lü Y Z 2011 Chin. J. Eng. Geophys. 8 303
Google Scholar
[3] Jahandari H, Ansari S, Farquharson C 2017 J. Appl. Geophys. 138 185
Google Scholar
[4] 汤文武, 柳建新, 叶益信 2018 石油地球物理勘探 53 617
Tang W W, Liu J X, Ye Y X 2018 Oil Geophys. Prosp. 53 617
[5] Nornikman H, Pee N, Ahmad B 2018 JTEC 10 35
[6] 张烨, 林蔺, 陈桂波 2018 地球 61 1639
Google Scholar
Zhang Y, Lin L, Chen G B 2018 Chinese J. Geophys. 61 1639
Google Scholar
[7] 魏宝君, 陈涛, 侯学理 2014 中国石油大学学报 38 57
Google Scholar
Wei B J, Chen T, Hou X L 2014 J. China Uni. Petrol. 38 57
Google Scholar
[8] 郭成豹, 肖昌汉, 刘大明 2008 57 4182
Google Scholar
Guo C B, Xiao C H, Liu D M 2008 Acta Phys. Sin. 57 4182
Google Scholar
[9] 陈桂波, 汪宏年, 姚敬金, 韩子夜 2009 58 3848
Google Scholar
Chen G B, Wang H N, Yao J J, Han Z Y 2009 Acta Phys. Sin. 58 3848
Google Scholar
[10] 李静和, 何展翔 2012 石油地球物理勘探 47 653
Li J H, He Z X 2012 Oil Geophys. Prosp. 47 653
[11] Li J H, He Z X, Xu Y X 2017 Appl. Geophys. 14 559
[12] Kruglyakov M, Kuvshinov A 2018 Geophys. J. Int. 213 1387
Google Scholar
[13] Tiberi G, Monorchio A, Manara G, Mittra R A 2006 IEEE T. Anteen. Propag. 54 2508
Google Scholar
[14] Nie X C, Yuan N, Liu C R 2010 IEEE Geosci. Remote. S. 48 72
Google Scholar
[15] Chobanyan E, Notaros B M, Ilic M M 2014 APSURSI 213 4
[16] 陈桂波, 毕娟, 汪剑波, 陈新邑, 孙贯成, 卢俊 2011 60 094102
Google Scholar
Chen G B, Bi J, Wang J B, Chen X Y, Sun G C, Lu J 2011 Acta Phys. Sin. 60 094102
Google Scholar
[17] Zhdanov M S, Wan L, Gribenko A, Martin C, Key K, Constable S 2011 Geophysics 7 6
[18] 殷长春, 张博, 刘云鹤, 蔡晶 2015 地球 58 1411
Google Scholar
Yin C C, Zhang B, Liu Y H, Cai J 2015 Chinese J. Geophys. 58 1411
Google Scholar
[19] 李先进, 雷霖, 陈涌频, 江明, 荣志, 胡俊 2019 电波科学学报 1 1
Li X J, Lei L, Chen Y P, Jiang M, Rong Z, Hu J 2019 The Chinese J of Rad. Sci. 1 1
[20] Li J H, Song L P, Liu Q H 2016 Pure Appl. Geophys. 173 607
Google Scholar
[21] Anderson W L 1979 Geophysics 44 1287
Google Scholar
[22] 阮百尧, 王有学 2005 地球 48 1197
Google Scholar
Ruan B Y, Wang Y X 2005 Chinese J. Geophys. 48 1197
Google Scholar
-
图 1 积分方程模拟三维地井电磁场观测系统示意图(未显示地形)
Fig. 1. Sketch of 3D (three-dimensional) SBEM (surface to borehole electromagnetic) measurement system using IE (integral equation) without topography.
图 3 观测系统及计算区域划分示意图 (a) 三维地井电磁; (b)参考空间介质; (c) 复杂地质构造背景介质; (d) 油气目标体
Fig. 3. Sketch of domain decomposition and observation system: (a) 3D SBEM; (b) reference model; (c) background model; (d) oil and gas model.
图 4 均匀半空间模型三维积分方程法(3D IE)、三维边界积分法(3D BIE)、Anderson算法模拟结果对比图
Fig. 4. Magnetic field of reference model calculated by 3D IE, 3D BIE and Anderson code.
图 5 三维山谷地形及地面电磁观测系统示意图, Tx为场源位置, Rx为接收点位置
Fig. 5. Sketch of 3D valley terrain with surface electromagnetic. Tx denotes transmitter and Rx is receiver.
图 6 三维山谷地形三维积分方程模拟、三维边界积分模拟地面磁场分量归一化响应及其差值对比图
Fig. 6. Magnetic field of 3D valley terrain calculated by 3D IE and 3D BIE: (a), (b) Total magnetic field; (c), (d) difference of magnetic field between IE and BIE.
图 7 均匀半空间地井电磁观测三维积分方程法、Anderson算法模拟电场响应对比
Fig. 7. Electric field of reference model calculated by 3D IE and Anderson code for 3D SBEM.
图 9 三维山谷地形三方位地井电磁场响应 (a) Tx1场源; (b) Tx2场源; (c) Tx3场源
Fig. 9. Electric field of 3D valley terrain with multi-azimuth SBEM: (a) Tx1; (b) Tx1; (c) Tx1.
表 1 均匀半空间介质地电结构模型的不同算法的电磁场模拟效率对比
Table 1. Comparison of computational effectiveness of modeling electromagnetic field via different algorithms for a half homogeneous medium.
模拟算法 截断边界 剖分方式 网格剖分 耗时/s Anderson 不需要 无 不需要 0.1 三维边界积分 需要 全局剖分 50 × 50 123.0 区域积分 不需要 局部剖分 20 × 20 × 20 86.0 矩量法 不需要 局部剖分 20 × 20 × 20 957.0 
下载: 导出CSV
-
[1] Augustin A, Kennedy W, Morrison H 1989 Geophysics 54 90
Google Scholar
[2] 李静和, 何展翔, 吕玉增 2011 工程地球 8 303
Google Scholar
Li J H, He Z X, Lü Y Z 2011 Chin. J. Eng. Geophys. 8 303
Google Scholar
[3] Jahandari H, Ansari S, Farquharson C 2017 J. Appl. Geophys. 138 185
Google Scholar
[4] 汤文武, 柳建新, 叶益信 2018 石油地球物理勘探 53 617
Tang W W, Liu J X, Ye Y X 2018 Oil Geophys. Prosp. 53 617
[5] Nornikman H, Pee N, Ahmad B 2018 JTEC 10 35
[6] 张烨, 林蔺, 陈桂波 2018 地球 61 1639
Google Scholar
Zhang Y, Lin L, Chen G B 2018 Chinese J. Geophys. 61 1639
Google Scholar
[7] 魏宝君, 陈涛, 侯学理 2014 中国石油大学学报 38 57
Google Scholar
Wei B J, Chen T, Hou X L 2014 J. China Uni. Petrol. 38 57
Google Scholar
[8] 郭成豹, 肖昌汉, 刘大明 2008 57 4182
Google Scholar
Guo C B, Xiao C H, Liu D M 2008 Acta Phys. Sin. 57 4182
Google Scholar
[9] 陈桂波, 汪宏年, 姚敬金, 韩子夜 2009 58 3848
Google Scholar
Chen G B, Wang H N, Yao J J, Han Z Y 2009 Acta Phys. Sin. 58 3848
Google Scholar
[10] 李静和, 何展翔 2012 石油地球物理勘探 47 653
Li J H, He Z X 2012 Oil Geophys. Prosp. 47 653
[11] Li J H, He Z X, Xu Y X 2017 Appl. Geophys. 14 559
[12] Kruglyakov M, Kuvshinov A 2018 Geophys. J. Int. 213 1387
Google Scholar
[13] Tiberi G, Monorchio A, Manara G, Mittra R A 2006 IEEE T. Anteen. Propag. 54 2508
Google Scholar
[14] Nie X C, Yuan N, Liu C R 2010 IEEE Geosci. Remote. S. 48 72
Google Scholar
[15] Chobanyan E, Notaros B M, Ilic M M 2014 APSURSI 213 4
[16] 陈桂波, 毕娟, 汪剑波, 陈新邑, 孙贯成, 卢俊 2011 60 094102
Google Scholar
Chen G B, Bi J, Wang J B, Chen X Y, Sun G C, Lu J 2011 Acta Phys. Sin. 60 094102
Google Scholar
[17] Zhdanov M S, Wan L, Gribenko A, Martin C, Key K, Constable S 2011 Geophysics 7 6
[18] 殷长春, 张博, 刘云鹤, 蔡晶 2015 地球 58 1411
Google Scholar
Yin C C, Zhang B, Liu Y H, Cai J 2015 Chinese J. Geophys. 58 1411
Google Scholar
[19] 李先进, 雷霖, 陈涌频, 江明, 荣志, 胡俊 2019 电波科学学报 1 1
Li X J, Lei L, Chen Y P, Jiang M, Rong Z, Hu J 2019 The Chinese J of Rad. Sci. 1 1
[20] Li J H, Song L P, Liu Q H 2016 Pure Appl. Geophys. 173 607
Google Scholar
[21] Anderson W L 1979 Geophysics 44 1287
Google Scholar
[22] 阮百尧, 王有学 2005 地球 48 1197
Google Scholar
Ruan B Y, Wang Y X 2005 Chinese J. Geophys. 48 1197
Google Scholar
下载:
计量
- 文章访问数: 6334
- PDF下载量: 52
- 被引次数: 0
