-
分子激光冷却与磁光囚禁在超越标准模型的新物理与新机制探索、超冷化学与冷分子碰撞等诸多领域中有着广泛的应用前景. CaH分子的某些态之间具有高度对角化的弗兰克-康登因子, 因此早在2004年就被提出作为激光冷却与磁光囚禁的候选分子之一. 利用速率方程并考虑双频效应的影响, 本文计算了
$ {\mathrm{A}}^{2}{\mathrm{Π}}_{1/2}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ}}^{+} $ 与${\mathrm{B}}^{2}{\mathrm{Σ }}^{+}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ }}^{+}$ 跃迁中CaH分子磁光阱内阻尼力和囚禁力的大小, 分析了四频率组分和多频率组分激光设置下CaH分子磁光囚禁时的冷却和囚禁效果. 结果发现,$ {\mathrm{A}}^{2}{\mathrm{Π}}_{1/2}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ}}^{+} $ 跃迁中, CaH分子在多频率组分激光设置下可获得更大的阻尼力和囚禁力, 从而有利于实现CaH分子磁光阱. 以上工作不仅证明了CaH分子磁光囚禁的可行性以及为实验探索提供了必要的理论支持, 同时也为超冷分子碰撞、极性冷分子BEC、基于极性冷分子的精密测量物理(如电子电偶极矩精密测量)等奠定了重要的研究基础.Laser cooling and magneto-optical trapping of molecules is regarded as one of the state-of-the-art research fields in physics, which possesses broad applications in exploring fundamental physics beyond the Standard Model, quantum many-body physics, cold/ultracold chemistry and collision studies and so forth. Owing to the characteristic of highly diagonal Franck-Condon factors, lower saturation irradiance and larger scattering rate, the CaH molecule has been proposed as a promising candidate for laser cooling and magneto-optical trapping ever since 2004. Taking advantage of the multi-energy-level rate equation as well as the dual frequency effect, we evaluate the damping and trapping forces contained in the optical transitions of$ {\mathrm{A}}^{2}{\mathrm{Π}}_{1/2}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ }}^{+} $ and${\mathrm{B}}^{2}{\mathrm{Σ }}^{+}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ }}^{+}$ , analyze the cooling and trapping performance for different laser polarization sets, power values and detunings of four laser components, and determine the variations in the damping and trapping forces due to an additional frequency component. It is discovered that if the laser polarization is set to be σ-σ+σ+σ+σ+, the detuning for the second laser component is Γwhile the detuning of other components are set to be -2Γ, and the laser power is set to be 150 mW, one can obtain a damping acceleration of 28000 m/s2, and a trapping acceleration of 19000 m/s2 for the transition of $ {\mathrm{A}}^{2}{\mathrm{Π}}_{1/2}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ }}^{+} $ , both of which reach the optimal values under the current scope of the research and exhibit better performance than the CaF molecule. Our results, on one hand, not only offer an ideal method to comprehend the CaH MOT in theory but also help design the CaH MOT experiment or even achieve the Bose-Einstein condensation (BEC) of cold diatomic molecules. On the other hand, alkaline-earth-metal monohydrides (AEMHs) such as CaH, SrH and BaH are well-known for their permanent electric dipole moment, therefore these trapped diatomic molecules can be utilized to untangle the mechanism of dipole-dipole interaction, thus paving the way to realizing the molecular entanglement and quantum computing. More interestingly, current experimental systems for the non-zero measurement of the electron’s electric dipole moment (eEDM), including ThO, YbF and HfF+, still cannot be conducted simultaneously under the laser cooling and magneto-optical trapping technique while maintaining the ease of full polarization and internal co-magnetometry, all of which undoubtedly can increase the coherent measurement time and hence the statistical sensitivity, as well as the immunity to the systematic sensitivity. Previous studies reported that AEMHs share some similar characters with alkaline-earth-metal monofluorides (AEMFs) such as in electron correlation effects, however, the hyperfine energy level structures of AEMHs are relatively simpler than those of AEMFs, and AEMHs are prone to being polarized under the externally applied electric field. All of these lead to the trend that AEMHs may possess the dual character that it can be not only laser cooled and trapped in a MOT but also adopted as an candidate to measure the eEDM. Therefore, our work lays a substantial foundation for the theoretical and experimental study of SrH and BaH that inevitably will contribute to the exploration of the CP violation and new physics beyond the Standard Model on a scientific platform based on cold polar molecules, which is obviously different from large facilities such as the Large Hadron Collider.[1] Hudson E R, Lewandowski H, Sawyer B C, Ye J 2006 Phys. Rev. Lett. 96 143004
Google Scholar
[2] Liu L, Hood J, Yu Y, Zhang J, Hutzler N, Rosenband T, Ni K K 2018 Science 360 900
Google Scholar
[3] Yang T, Thomas A M, Dangi B B, Kaiser R I, Mebel A M, Millar T J 2018 Nat. Commun. 9 1
Google Scholar
[4] Kerman A J, Sage J M, Sainis S, Bergeman T, DeMille D 2004 Phys. Rev. Lett. 92 153001
Google Scholar
[5] Wang D, Qi J, Stone M, Nikolayeva O, Wang H, Hattaway B, Gensemer S, Gould P, Eyler E, Stwalley W 2004 Phys. Rev. Lett. 93 243005
Google Scholar
[6] Ni K K, Ospelkaus S, De Miranda M, Pe'Er A, Neyenhuis B, Zirbel J, Kotochigova S, Julienne P, Jin D, Ye J 2008 Science 322 231
Google Scholar
[7] 陈涛, 颜波 2019 68 043701
Google Scholar
Chen T, Yan B 2019 Acta Phys. Sin. 68 043701
Google Scholar
[8] Bruzewicz C, Gustavsson M, Shimasaki T, DeMille D 2014 New. J. Phys. 16 023018
Google Scholar
[9] Wu C H, Park J W, Ahmadi P, Will S, Zwierlein M W 2012 Phys. Rev. Lett. 109 085301
Google Scholar
[10] Jin D S, Ye J 2011 Phys. Today 64 27
[11] Bethlem H L, Berden G, Meijer G 1999 Phys. Rev. Lett. 83 1558
Google Scholar
[12] Barry J, McCarron D, Norrgard E, Steinecker M, DeMille D 2014 Nature 512 286
Google Scholar
[13] Weinstein J D, Decarvalho R, Amar K, Boca A, Odom B C, Friedrich B, Doyle J M 1998 J. Chem. Phys. 109 2656
Google Scholar
[14] 夏勇, 汪海玲, 许亮, 印建平 2018 47 24
Google Scholar
Xia Y, Wang H L, Xu L, Yin J P 2018 Acta Phys. Sin. 47 24
Google Scholar
[15] Jochim S, Bartenstein M, Altmeyer A, Hendl G, Riedl S, Chin C, Hecker Denschlag J, Grimm R 2003 Science 302 2101
Google Scholar
[16] Greiner M, Regal C A, Jin D S 2003 Nature 426 537
Google Scholar
[17] Zhang Z, Chen L, Yao K X, Chin C 2021 Nature 592 708
Google Scholar
[18] Steinecker M H, McCarron D J, Zhu Y, DeMille D 2016 ChemPhysChem 17 3664
Google Scholar
[19] Tarbutt M, Steimle T 2015 Phys. Rev. A 92 053401
Google Scholar
[20] Truppe S, Williams H, Hambach M, Caldwell L, Fitch N, Hinds E, Sauer B, Tarbutt M 2017 Nat. Phys. 13 1173
Google Scholar
[21] Anderegg L, Augenbraun B L, Chae E, Hemmerling B, Hutzler N R, Ravi A, Collopy A, Ye J, Ketterle W, Doyle J M 2017 Phys. Rev. Lett. 119 103201
Google Scholar
[22] Langin T K, Jorapur V, Zhu Y, Wang Q, DeMille D 2021 Phys. Rev. Lett. 127 163201
Google Scholar
[23] Lu H I, Rasmussen J, Wright M J, Patterson D, Doyle J M 2011 Phys. Chem. Chem. Phys. 13 18986
Google Scholar
[24] 尹俊豪, 杨涛, 印建平 2021 70 163302
Google Scholar
Yin J H, Yang T, Yin J P 2021 Acta Phys. Sin. 70 163302
Google Scholar
[25] Collopy A L, Ding S, Wu Y, Finneran I A, Anderegg L, Augenbraun B L, Doyle J M, Ye J 2018 Phys. Rev. Lett. 121 213201
Google Scholar
[26] Iwata G, McNally R, Zelevinsky T 2017 Phys. Rev. A 96 022509
Google Scholar
[27] Chen T, Bu W, Yan B 2017 Phys. Rev. A 96 053401
Google Scholar
[28] Gu R, Xia M, Yan K, Wu D, Wei J, Xu L, Xia Y, Yin J 2022 J. Quant. Spectrosc. Radiat. Transfer 278 108015
Google Scholar
[29] Kozyryev I, Baum L, Matsuda K, Augenbraun B L, Anderegg L, Sedlack A P, Doyle J M 2017 Phys. Rev. Lett. 118 173201
Google Scholar
[30] Baum L, Vilas N B, Hallas C, Augenbraun B L, Raval S, Mitra D, Doyle J M 2021 Phys. Rev. A 103 043111
Google Scholar
[31] Fazil N, Prasannaa V, Latha K, Abe M, Das B 2018 Phys. Rev. A 98 032511
Google Scholar
[32] Kozyryev I, Hutzler N R 2017 Phys. Rev. Lett. 119 133002
Google Scholar
[33] Barbuy B, Schiavon R, Gregorio-Hetem J, Singh P, Batalha C 1993 Astron. Astrophys. Suppl. Ser. 101 409
[34] Lépine S, Rich R M, Shara M M 2003 Astrophys. J. 591 L49
Google Scholar
[35] Barclay Jr W, Anderson M, Ziurys L M 1993 Astrophys. J. 408 L65
Google Scholar
[36] Steimle T, Chen J, Gengler J 2004 J. Chem. Phys. 121 829
Google Scholar
[37] Weinstein J D, DeCarvalho R, Guillet T, Friedrich B, Doyle J M 1998 Nature 395 148
Google Scholar
[38] Singh V, Hardman K S, Tariq N, Lu M J, Ellis A, Morrison M J, Weinstein J D 2012 Phys. Rev. Lett. 108 203201
Google Scholar
[39] Ramanaiah M, Lakshman S 1982 Physica 113C 263
Google Scholar
[40] Nakagawa J, Domaille P J, Steimle T C, Harris D O 1978 J. Mol. Spectrosc. 70 374
Google Scholar
[41] Shayesteh A, Ram R S, Bernath P F 2013 J. Mol. Spectrosc. 288 46
Google Scholar
[42] Gao Y, Gao T 2014 Phys. Rev. A 90 052506
Google Scholar
[43] Chen J, Gengler J, Steimle T, Brown J M 2006 Phys. Rev. A 73 012502
Google Scholar
[44] Barry J F, McCarron D J, Norrgard E B, Steinecker M H, DeMille D 2014 Nature 512 286
[45] Xu S, Xia M, Gu R, Yin Y, Xu L, Xia Y, Yin J 2019 Phys. Rev. A 99 033408
Google Scholar
[46] Tarbutt M 2015 New. J. Phys. 17 015007
Google Scholar
[47] Liu M, Pauchard T, Sjödin M, Launila O, van der Meulen P, Berg L E 2009 J. Mol. Spectrosc. 257 105
Google Scholar
[48] Xu L, Yin Y, Wei B, Xia Y, Yin J 2016 Phys. Rev. A 93 013408
Google Scholar
[49] Steimle T C, Meyer T P, Al-Ramadin Y, Bernath P 1987 J. Mol. Spectrosc. 125 225
Google Scholar
[50] Williams H, Truppe S, Hambach M, Caldwell L, Fitch N, Hinds E, Sauer B, Tarbutt M 2017 New J. Phys. 19 113035
Google Scholar
-
图 1 CaH分子
${\mathrm{{\rm X}}}^{2}{\mathrm{Σ }}^{+}(\upsilon =0, N=1)$ 电子态的塞曼分裂示意图. F表示总角动量, 括号里的数字是每一个超精细能级的g因子值Fig. 1. The Zeeman level structure for the
${{{\rm X}}}^{2}{{\Sigma }}^{+}(\upsilon = $ $ 0, N=1)$ state of the CaH molecule. F represents the total angular momentum, while numbers in parentheses indicate the g factor for each hyperfine energy level.
图 2 (a) 双频效应原理图. 基态能级Fl = 2, gl = 0.5, 激发态能级Fu = 1, gu = 0.不同偏振的频率分量激发同一能级, 失谐量分别是
$ {\delta }_{1} $ 和$ {\delta }_{2} $ . (b) 囚禁频率与失谐量$ {\delta }_{2} $ 的曲线图; (c)阻尼系数与失谐量$ {\delta }_{2} $ 的曲线图Fig. 2. (a) Illustration of the dual-frequency effect with the ground energy level Fl = 2, gl = 0.5 and the excited energy level Fu = 1, gu = 0. Two transitions with oppositely polarized frequency components were driven, while the detunings are
$ {\delta }_{1} $ and$ {\delta }_{2} $ respectively. (b) Trap frequency versus$ {\delta }_{2} $ ; (c) Damping coefficient versus$ {\delta }_{2} $ .
图 3 CaH分子MOT中
$ \mathrm{A}^{2}{\mathrm{Π}}_{1/2}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ}}^{+} $ 和${\mathrm{B}}^{2}{\mathrm{Σ }}^{+}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ }}^{+}$ 跃迁的频率分布方案Fig. 3. Frequency distribution schemes for
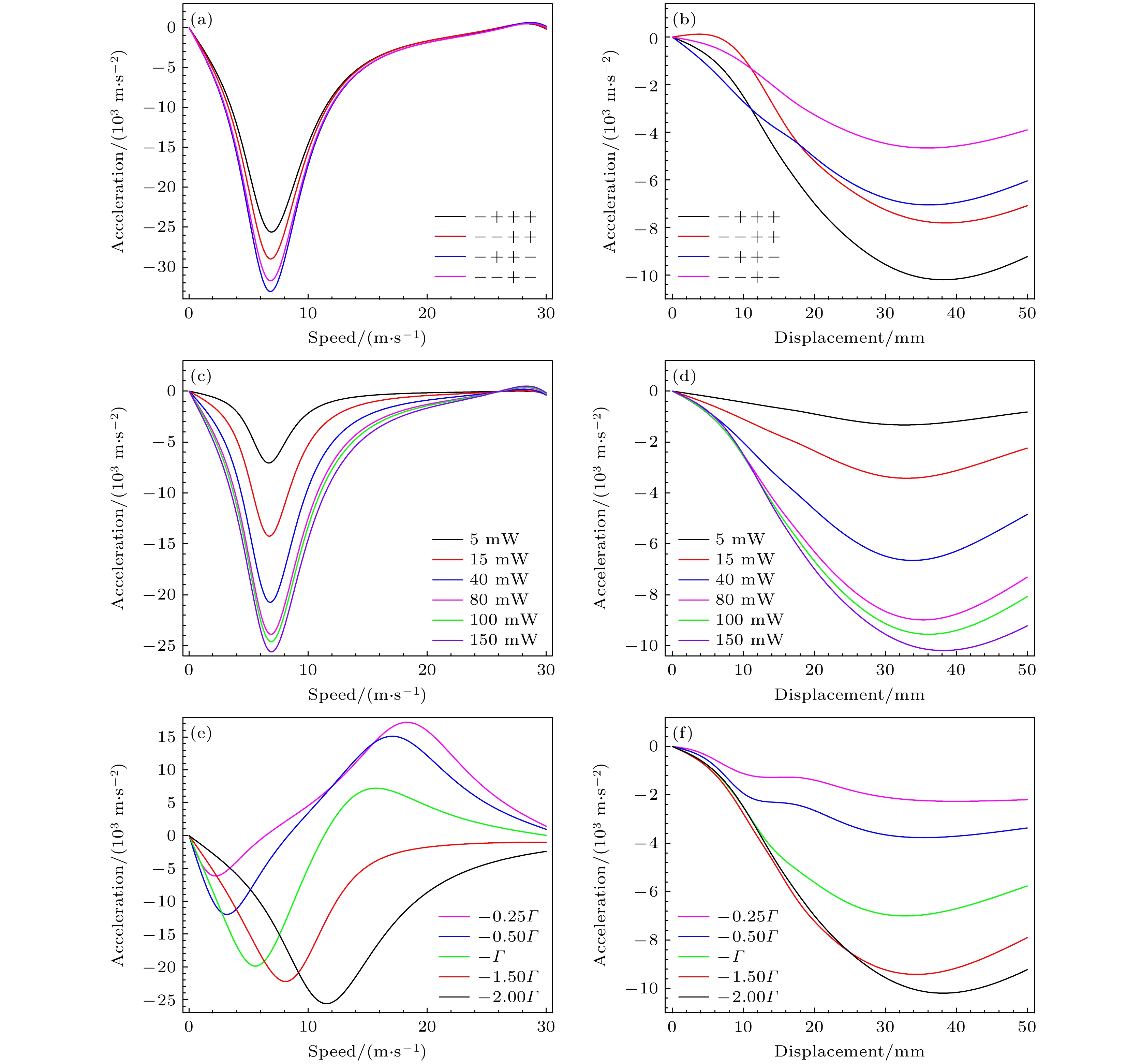
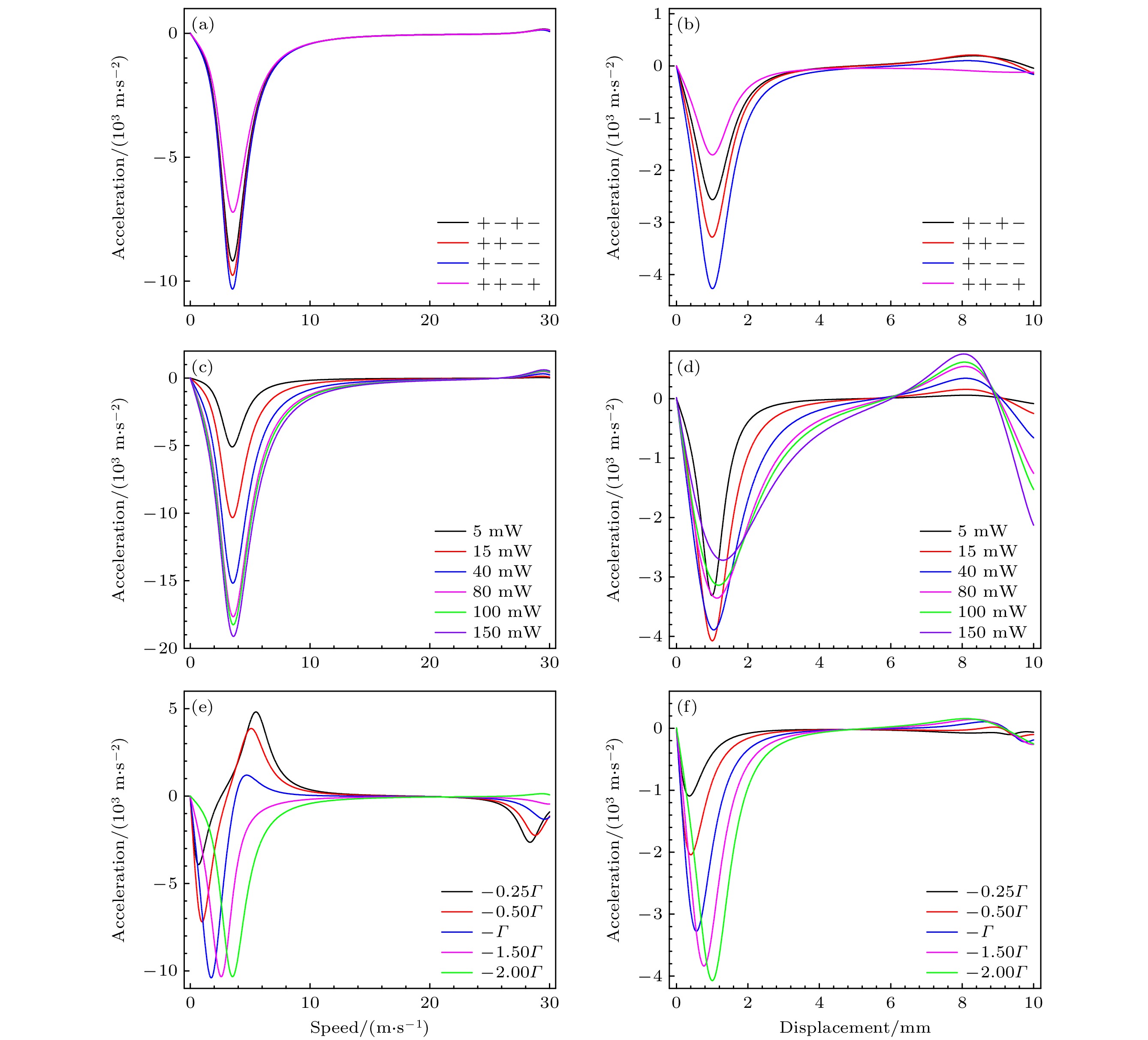
${\mathrm{A}}^{2}{\mathrm{Π}}_{1/2}\leftarrow $ $ {\mathrm{X}}^{2}{\mathrm{Σ }}^{+}$ and${\mathrm{B}}^{2}{\mathrm{Σ }}^{+}\leftarrow {\mathrm{X}}^{2}{\mathrm{Σ }}^{+}$ transitions in a MOT of CaH.
图 4 不同偏振组态下的加速度与(a)速度和(b)位移的关系图. 其中激光功率均为150 mW, 失谐为–2Γ. 不同激光功率下的加速度与(c)速度和(d)位移关系图. 其中频率组分的偏振组态为(–+++), 失谐为–2Γ. 不同失谐量下的加速度与(e)速度和(f)位移关系图. 其中激光功率均为150 mW, 频率组分的偏振组态为(–+++)
Fig. 4. Acceleration versus (a) speed and (b) displacement under different polarization configurations where the power for lasers is set to be 150 mW and the detuning is –2Γ. Acceleration versus (c) speed and (d) displacement on different laser powers. The polarization configuration is (–+++), while the detuning is –2Γ. Acceleration versus (e) speed and (f) displacement on various detunings, where the laser power is set as 150 mW and the polarization configuration is (–+++).
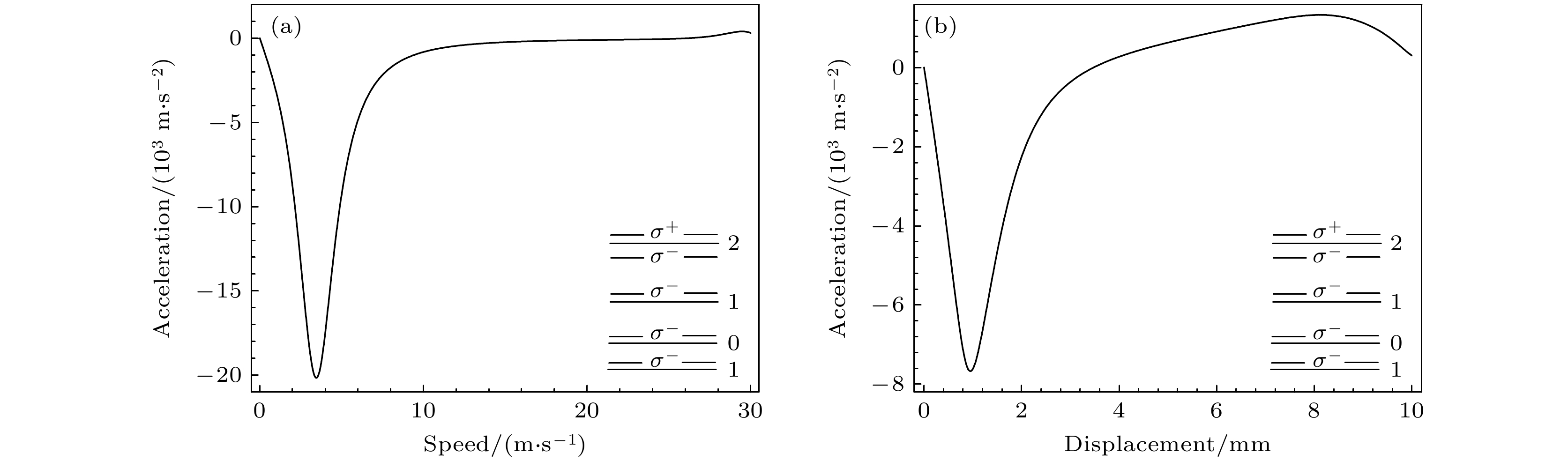
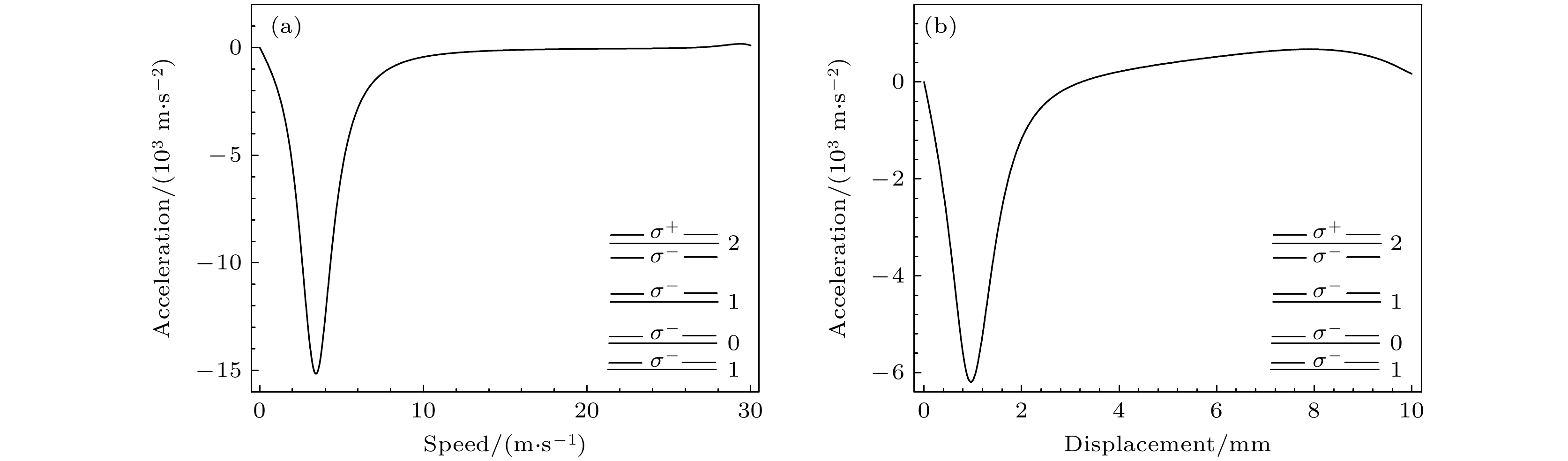
图 5 加速度与(a)速度和(b)位移关系图. 激光频率偏振设置为插图中所示的情况, 其中激光功率均为150 mW. 除了额外加的频率失谐是Γ, 其他频率分量失谐都是–2Γ
Fig. 5. Acceleration versus (a) speed and (b) displacement using the set of detunings and polarizations illustrated in the inset. Here, the laser power is set to be 150 mW. The detuning is –2Γ apart from the additional component of Γ.
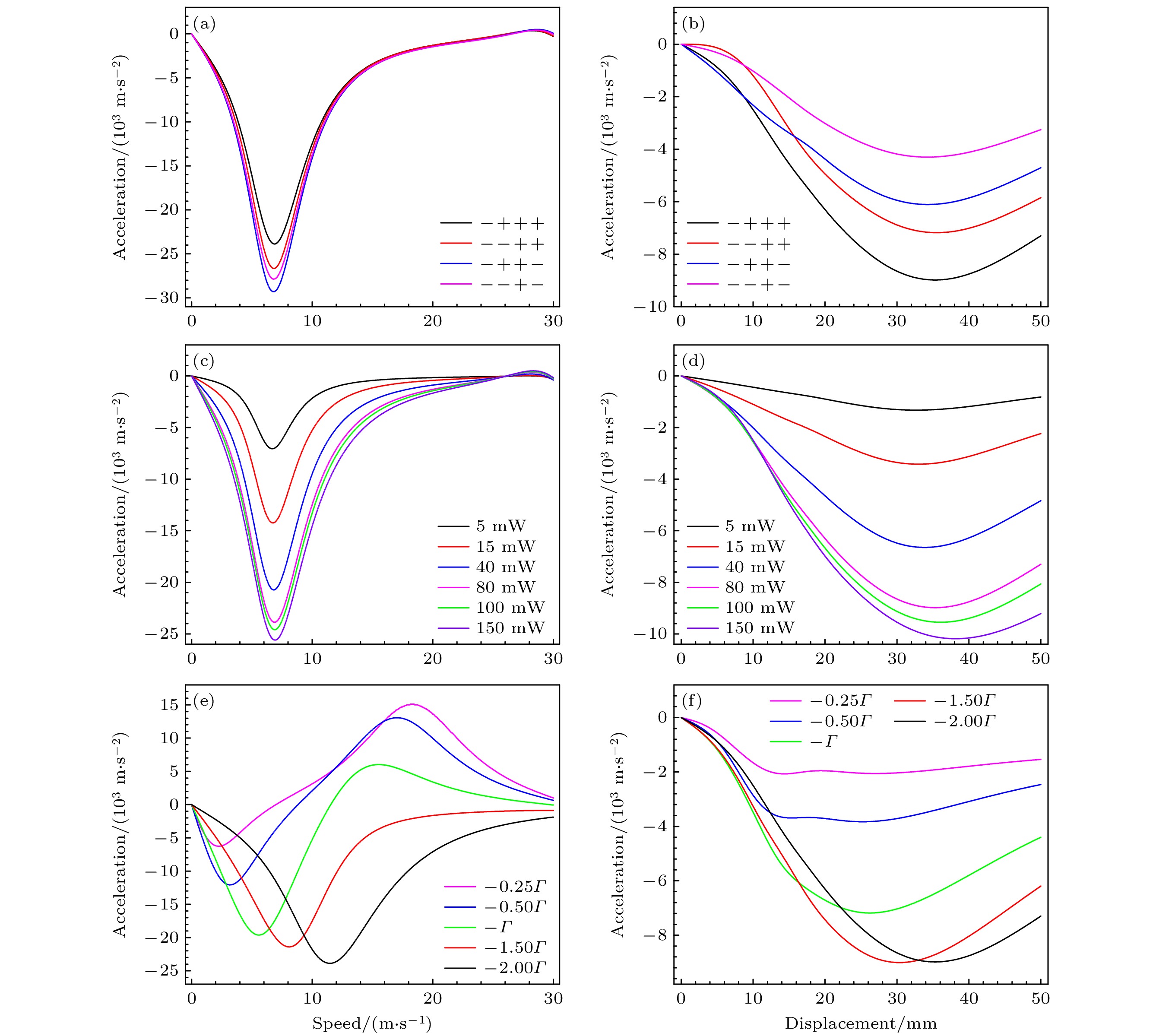
图 6 不同偏振组态下的加速度与(a)速度和(b)位移关系图. 其中激光功率均为40 mW, 失谐为–2Γ. 不同功率下的加速度与(c)和速度(d)位移关系图. 其中偏振组态为(+–––), 失谐为–2Γ. 不同失谐量下的加速度与(e)速度和(f)位移关系图. 其中激光功率均为40 mW, 偏振组态为(+–––)
Fig. 6. Acceleration versus (a) speed and (b) displacement under different polarization configurations where the power for lasers is set to be 40 mW and the detuning is –2Γ. Acceleration versus (c) speed and (d) displacement on different laser powers. The polarization configuration is (+–––), while the detuning is –2Γ. Acceleration versus (e) speed and (f) displacement on various detunings, where the laser power is set as 40 mW and the polarization configuration is (+–––).
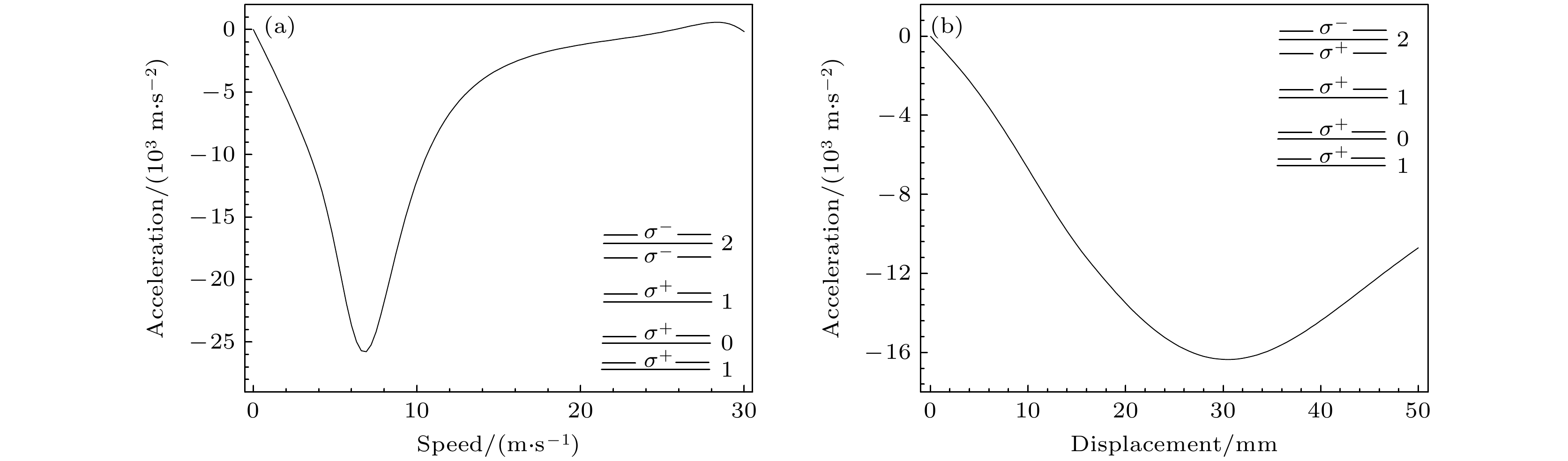
图 7 加速度与(a)速度和(b)位移关系图. 激光频率偏振设置为插图中所示的情况, 其中激光功率均为40 mW. 除了额外加的频率失谐是1.5Γ, 其他频率分量失谐都是–2Γ
Fig. 7. Acceleration versus (a) speed and (b) displacement using the set of detunings and polarizations illustrated in the inset. Here, the laser power is set to be 40 mW. The detuning is –2Γ apart from the additional component of 1.5Γ.
图 A1
${\mathrm{A}}^{2}{{\Pi }}_{1/2}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+}$ 跃迁中不同偏振组态下的加速度与(a)速度和(b)位移的关系图. 其中激光功率均为40 mW, 失谐为–2Γ. 不同激光功率下的加速度与(c)速度和(d)位移关系图. 其中四个频率组分的偏振组态为(–+++), 失谐为–2Γ. 不同失谐量下的加速度与(e)速度和(f)位移关系图. 其中激光功率均为40 mW, 频率组分的偏振组态为(–+++)Fig. A1. Acceleration versus (a) speed and (b) displacement under different polarization configurations in the
$ {\mathrm{A}}^{2}{{\Pi }}_{1/2}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ transition, where the power for lasers is set to be 40 mW and the detuning is –2Γ. Acceleration versus (c) speed and (d) displacement on different laser powers. The polarization configuration is (–+++), while the detuning is –2Γ. Acceleration versus (e) speed and (f) displacement on various detunings, where the laser power is set as 40 mW and the polarization configuration is (–+++).
图 A2
$ {\mathrm{A}}^{2}{{\Pi }}_{1/2}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ 跃迁中加速度与(a)速度和(b)位移关系图. 激光频率偏振设置为插图中所示的情况, 其中激光功率均为40 mW.除了额外加的频率失谐是Γ, 其他频率分量失谐都是–2ΓFig. A2. Acceleration versus (a) speed and (b) displacement in the
$ {\mathrm{A}}^{2}{{\Pi }}_{1/2}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ transition, using the set of detunings and polarizations illustrated in the inset. Here, the laser power is set to be 40 mW. The detuning is –2Γ apart from the additional component of Γ.
图 A3
${\mathrm{A}}^{2}{{\Pi }}_{1/2}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+}$ 跃迁中不同偏振组态下, 加速度与(a)速度和(b)位移的关系图. 其中激光功率均为80 mW, 失谐为–2Γ. 不同激光功率下的加速度与(c)速度和(d)位移关系图. 其中频率组分的偏振组态为(–+++), 失谐为–2Γ. 不同失谐量下的加速度与(e)速度和(f)位移关系图. 其中激光功率均为80 mW, 频率组分的偏振组态为(–+++)Fig. A3. Acceleration versus (a) speed and (b) displacement under different polarization configurations in the
$ {\mathrm{A}}^{2}{{\Pi }}_{1/2}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ transition, where the power for lasers is set to be 80 mW and the detuning is –2Γ. Acceleration versus (c) speed and (d) displacement on different laser powers. The polarization configuration is (–+++), while the detuning is –2Γ. Acceleration versus (e) speed and (f) displacement on various detunings, where the laser power is set as 80 mW and the polarization configuration is (–+++).
图 A4
${\mathrm{A}}^{2}{{\Pi }}_{1/2}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+}$ 跃迁中加速度与(a)速度和(b)位移关系图. 激光频率偏振设置为插图中所示的情况, 其中激光功率均为80 mW. 除了额外加的频率失谐是Γ, 其他频率分量失谐都是–2ΓFig. A4. Acceleration versus (a) speed and (b) displacement in the
${\mathrm{A}}^{2}{{\Pi }}_{1/2}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+}$ transition, using the set of detunings and polarizations illustrated in the inset. Here, the laser power is set to be 80 mW. The detuning is –2Γ apart from the additional component of Γ.
图 A5
$ {\mathrm{B}}^{2}{{\Sigma }}^{+}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ 跃迁中不同偏振组态下的加速度与(a)速度和(b)位移的关系图. 其中激光功率均为15 mW, 失谐为–2Γ. 不同激光功率下的加速度与(c)速度和(d)位移关系图. 其中频率组分的偏振组态为(+–––), 失谐为–2Γ. 不同失谐量下的加速度与(e)速度和(f)位移关系图. 其中激光功率均为15 mW, 频率组分的偏振组态为(+–––)Fig. A5. Acceleration versus (a) speed and (b) displacement under different polarization configurations in the
$ {\mathrm{B}}^{2}{{\Sigma }}^{+}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ transition, where the power for lasers is set to be 15 mW and the detuning is –2Γ. Acceleration versus (c) speed and (d) displacement on different laser powers. The polarization configuration is (+–––), while the detuning is –2Γ. Acceleration versus (e) speed and (f) displacement on various detunings, where the laser power is set as 15 mW and the polarization configuration is (+–––).
图 A6
$ {\mathrm{B}}^{2}{{\Sigma }}^{+}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ 跃迁中加速度与(a)速度和(b)位移关系图. 激光频率偏振设置为插图中所示的情况, 其中激光功率均为15 mW. 除了额外加的频率失谐是1.5Γ, 其他频率分量失谐都是–2ΓFig. A6. Acceleration versus (a) speed and (b) displacement in the
$ {\mathrm{B}}^{2}{{\Sigma }}^{+}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ transition, using the set of detunings and polarizations illustrated in the inset. Here, the laser power is set to be 15 mW. The detuning is –2Γ apart from the additional component of 1.5Γ.
图 A7
$ {\mathrm{B}}^{2}{{\Sigma }}^{+}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ 跃迁中不同偏振组态下的加速度与(a)速度和(b)位移的关系图. 其中激光功率均为80 mW, 失谐为–2Γ. 不同激光功率下的加速度与(c)速度和(d)位移关系图. 其中频率组分的偏振组态为(+–––), 失谐为–2Γ. 不同失谐量下的加速度与(e)速度和(f)位移关系图. 其中激光功率均为80 mW, 频率组分的偏振组态为(+–––)Fig. A7. Acceleration versus (a) speed and (b) displacement under different polarization configurations in the
$ {\mathrm{B}}^{2}{{\Sigma }}^{+}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ transition, where the power for lasers is set to be 80 mW and the detuning is –2Γ. Acceleration versus (c) speed and (d) displacement on different laser powers. The polarization configuration is (+–––), while the detuning is –2Γ. Acceleration versus (e) speed and (f) displacement on various detunings, where the laser power is set as 80 mW and the polarization configuration is (+–––).
图 A8
$ {\mathrm{B}}^{2}{{\Sigma }}^{+}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ 跃迁中加速度与(a)速度和(b)位移关系图. 激光频率偏振设置为插图中所示的情况, 其中激光功率均为80 mW. 除了额外加的频率失谐是1.5Γ, 其他频率分量失谐都是–2ΓFig. A8. Acceleration versus (a) speed and (b) displacement in the
$ {\mathrm{B}}^{2}{{\Sigma }}^{+}\leftarrow {\mathrm{X}}^{2}{{\Sigma }}^{+} $ transition, using the set of detunings and polarizations illustrated in the inset. Here, the laser power is set to be 80 mW. The detuning is –2Γ apart from the additional component of 1.5Γ. -
[1] Hudson E R, Lewandowski H, Sawyer B C, Ye J 2006 Phys. Rev. Lett. 96 143004
Google Scholar
[2] Liu L, Hood J, Yu Y, Zhang J, Hutzler N, Rosenband T, Ni K K 2018 Science 360 900
Google Scholar
[3] Yang T, Thomas A M, Dangi B B, Kaiser R I, Mebel A M, Millar T J 2018 Nat. Commun. 9 1
Google Scholar
[4] Kerman A J, Sage J M, Sainis S, Bergeman T, DeMille D 2004 Phys. Rev. Lett. 92 153001
Google Scholar
[5] Wang D, Qi J, Stone M, Nikolayeva O, Wang H, Hattaway B, Gensemer S, Gould P, Eyler E, Stwalley W 2004 Phys. Rev. Lett. 93 243005
Google Scholar
[6] Ni K K, Ospelkaus S, De Miranda M, Pe'Er A, Neyenhuis B, Zirbel J, Kotochigova S, Julienne P, Jin D, Ye J 2008 Science 322 231
Google Scholar
[7] 陈涛, 颜波 2019 68 043701
Google Scholar
Chen T, Yan B 2019 Acta Phys. Sin. 68 043701
Google Scholar
[8] Bruzewicz C, Gustavsson M, Shimasaki T, DeMille D 2014 New. J. Phys. 16 023018
Google Scholar
[9] Wu C H, Park J W, Ahmadi P, Will S, Zwierlein M W 2012 Phys. Rev. Lett. 109 085301
Google Scholar
[10] Jin D S, Ye J 2011 Phys. Today 64 27
[11] Bethlem H L, Berden G, Meijer G 1999 Phys. Rev. Lett. 83 1558
Google Scholar
[12] Barry J, McCarron D, Norrgard E, Steinecker M, DeMille D 2014 Nature 512 286
Google Scholar
[13] Weinstein J D, Decarvalho R, Amar K, Boca A, Odom B C, Friedrich B, Doyle J M 1998 J. Chem. Phys. 109 2656
Google Scholar
[14] 夏勇, 汪海玲, 许亮, 印建平 2018 47 24
Google Scholar
Xia Y, Wang H L, Xu L, Yin J P 2018 Acta Phys. Sin. 47 24
Google Scholar
[15] Jochim S, Bartenstein M, Altmeyer A, Hendl G, Riedl S, Chin C, Hecker Denschlag J, Grimm R 2003 Science 302 2101
Google Scholar
[16] Greiner M, Regal C A, Jin D S 2003 Nature 426 537
Google Scholar
[17] Zhang Z, Chen L, Yao K X, Chin C 2021 Nature 592 708
Google Scholar
[18] Steinecker M H, McCarron D J, Zhu Y, DeMille D 2016 ChemPhysChem 17 3664
Google Scholar
[19] Tarbutt M, Steimle T 2015 Phys. Rev. A 92 053401
Google Scholar
[20] Truppe S, Williams H, Hambach M, Caldwell L, Fitch N, Hinds E, Sauer B, Tarbutt M 2017 Nat. Phys. 13 1173
Google Scholar
[21] Anderegg L, Augenbraun B L, Chae E, Hemmerling B, Hutzler N R, Ravi A, Collopy A, Ye J, Ketterle W, Doyle J M 2017 Phys. Rev. Lett. 119 103201
Google Scholar
[22] Langin T K, Jorapur V, Zhu Y, Wang Q, DeMille D 2021 Phys. Rev. Lett. 127 163201
Google Scholar
[23] Lu H I, Rasmussen J, Wright M J, Patterson D, Doyle J M 2011 Phys. Chem. Chem. Phys. 13 18986
Google Scholar
[24] 尹俊豪, 杨涛, 印建平 2021 70 163302
Google Scholar
Yin J H, Yang T, Yin J P 2021 Acta Phys. Sin. 70 163302
Google Scholar
[25] Collopy A L, Ding S, Wu Y, Finneran I A, Anderegg L, Augenbraun B L, Doyle J M, Ye J 2018 Phys. Rev. Lett. 121 213201
Google Scholar
[26] Iwata G, McNally R, Zelevinsky T 2017 Phys. Rev. A 96 022509
Google Scholar
[27] Chen T, Bu W, Yan B 2017 Phys. Rev. A 96 053401
Google Scholar
[28] Gu R, Xia M, Yan K, Wu D, Wei J, Xu L, Xia Y, Yin J 2022 J. Quant. Spectrosc. Radiat. Transfer 278 108015
Google Scholar
[29] Kozyryev I, Baum L, Matsuda K, Augenbraun B L, Anderegg L, Sedlack A P, Doyle J M 2017 Phys. Rev. Lett. 118 173201
Google Scholar
[30] Baum L, Vilas N B, Hallas C, Augenbraun B L, Raval S, Mitra D, Doyle J M 2021 Phys. Rev. A 103 043111
Google Scholar
[31] Fazil N, Prasannaa V, Latha K, Abe M, Das B 2018 Phys. Rev. A 98 032511
Google Scholar
[32] Kozyryev I, Hutzler N R 2017 Phys. Rev. Lett. 119 133002
Google Scholar
[33] Barbuy B, Schiavon R, Gregorio-Hetem J, Singh P, Batalha C 1993 Astron. Astrophys. Suppl. Ser. 101 409
[34] Lépine S, Rich R M, Shara M M 2003 Astrophys. J. 591 L49
Google Scholar
[35] Barclay Jr W, Anderson M, Ziurys L M 1993 Astrophys. J. 408 L65
Google Scholar
[36] Steimle T, Chen J, Gengler J 2004 J. Chem. Phys. 121 829
Google Scholar
[37] Weinstein J D, DeCarvalho R, Guillet T, Friedrich B, Doyle J M 1998 Nature 395 148
Google Scholar
[38] Singh V, Hardman K S, Tariq N, Lu M J, Ellis A, Morrison M J, Weinstein J D 2012 Phys. Rev. Lett. 108 203201
Google Scholar
[39] Ramanaiah M, Lakshman S 1982 Physica 113C 263
Google Scholar
[40] Nakagawa J, Domaille P J, Steimle T C, Harris D O 1978 J. Mol. Spectrosc. 70 374
Google Scholar
[41] Shayesteh A, Ram R S, Bernath P F 2013 J. Mol. Spectrosc. 288 46
Google Scholar
[42] Gao Y, Gao T 2014 Phys. Rev. A 90 052506
Google Scholar
[43] Chen J, Gengler J, Steimle T, Brown J M 2006 Phys. Rev. A 73 012502
Google Scholar
[44] Barry J F, McCarron D J, Norrgard E B, Steinecker M H, DeMille D 2014 Nature 512 286
[45] Xu S, Xia M, Gu R, Yin Y, Xu L, Xia Y, Yin J 2019 Phys. Rev. A 99 033408
Google Scholar
[46] Tarbutt M 2015 New. J. Phys. 17 015007
Google Scholar
[47] Liu M, Pauchard T, Sjödin M, Launila O, van der Meulen P, Berg L E 2009 J. Mol. Spectrosc. 257 105
Google Scholar
[48] Xu L, Yin Y, Wei B, Xia Y, Yin J 2016 Phys. Rev. A 93 013408
Google Scholar
[49] Steimle T C, Meyer T P, Al-Ramadin Y, Bernath P 1987 J. Mol. Spectrosc. 125 225
Google Scholar
[50] Williams H, Truppe S, Hambach M, Caldwell L, Fitch N, Hinds E, Sauer B, Tarbutt M 2017 New J. Phys. 19 113035
Google Scholar
-
[1] 白素英, 白景旭, 韩小萱, 焦月春, 赵建明. 超冷长程Rydberg-基态分子. , 2021, 70(12): 123201. doi: 10.7498/aps.70.20202229 [2] 李军依, 叶玉儿, 凌晨, 李林, 刘泱, 夏勇. 超透镜聚焦光环的产生及其在冷分子光学囚禁中的应用. , 2021, 70(16): 167802. doi: 10.7498/aps.70.20210443 [3] 尹俊豪, 杨涛, 印建平. 基于 ${{\bf{A}}}^{{\boldsymbol{2}}}{{{\boldsymbol{\Pi}} }}_{{\boldsymbol{1/2}}}{\boldsymbol{\leftarrow }}{{\bf{X}}}^{{\boldsymbol{2}}}{{{\boldsymbol{\Sigma }}}}_{{\boldsymbol{1/2}}}$ 跃迁的CaH分子激光冷却光谱理论研究. , 2021, 70(16): 163302. doi: 10.7498/aps.70.20210522[4] 鹿博, 王大军. 超冷极性分子. , 2019, 68(4): 043301. doi: 10.7498/aps.68.20182274 [5] 陈涛, 颜波. 极性分子的激光冷却及囚禁技术. , 2019, 68(4): 043701. doi: 10.7498/aps.68.20181655 [6] 秦燕, 栗生长. 基于方波脉冲外场的超冷原子-分子绝热转化. , 2018, 67(20): 203701. doi: 10.7498/aps.67.20180908 [7] 许雪艳, 侯顺永, 印建平. 一种可控的Ioffe型冷分子表面微电阱. , 2018, 67(11): 113701. doi: 10.7498/aps.67.20180206 [8] 李晓云, 孙博文, 许正倩, 陈静, 尹亚玲, 印建平. 基于调制光晶格的中性分子束光学Stark减速与囚禁的理论研究. , 2018, 67(20): 203702. doi: 10.7498/aps.67.20181348 [9] 张云光, 张华, 窦戈, 徐建刚. 激光冷却OH分子的理论研究. , 2017, 66(23): 233101. doi: 10.7498/aps.66.233101 [10] 马杰, 王晓峰, 辛统钰, 刘文良, 李玉清, 武寄洲, 肖连团, 贾锁堂. 超冷铯分子0u+(6P3/2)长程态的高灵敏光缔合光谱研究. , 2015, 64(15): 153303. doi: 10.7498/aps.64.153303 [11] 刘建平, 侯顺永, 魏斌, 印建平. 亚声速NH3分子束静电Stark减速的理论研究. , 2015, 64(17): 173701. doi: 10.7498/aps.64.173701 [12] 赵延霆, 元晋鹏, 姬中华, 李中豪, 孟腾飞, 刘涛, 肖连团, 贾锁堂. 光缔合制备超冷铯分子的温度测量. , 2014, 63(19): 193701. doi: 10.7498/aps.63.193701 [13] 元晋鹏, 姬中华, 杨艳, 张洪山, 赵延霆, 马杰, 汪丽蓉, 肖连团, 贾锁堂. 飞行时间质谱探测磁光阱中超冷分子离子的实验研究. , 2012, 61(18): 183301. doi: 10.7498/aps.61.183301 [14] 陆俊发, 周琦, 纪宪明, 印建平. 实现冷原子、冷分子光学囚禁的组合三光学势阱方案. , 2011, 60(6): 063701. doi: 10.7498/aps.60.063701 [15] 许雪艳, 陈海波, 印建平. 一种实现冷分子囚禁的可控制静电双阱方案. , 2009, 58(3): 1563-1568. doi: 10.7498/aps.58.1563 [16] 沐仁旺, 纪宪明, 印建平. 一种实现冷原子(或冷分子)囚禁的可控制纵向光学双阱. , 2006, 55(11): 5795-5802. doi: 10.7498/aps.55.5795 [17] 陆俊发, 纪宪明, 印建平. 实现冷原子或冷分子囚禁的可控制光学四阱. , 2006, 55(4): 1740-1750. doi: 10.7498/aps.55.1740 [18] 沐仁旺, 李雅丽, 纪宪明, 印建平. 实现冷原子(冷分子)囚禁的可控制光学双阱的产生及其实验研究. , 2006, 55(12): 6333-6341. doi: 10.7498/aps.55.6333 [19] 纪宪明, 陆俊法, 沐仁旺, 印建平. 采用Damman光栅实现冷原子或冷分子囚禁的光阱阵列. , 2006, 55(7): 3396-3402. doi: 10.7498/aps.55.3396 [20] 纪宪明, 印建平. 冷原子或冷分子囚禁的可控制光学双阱. , 2004, 53(12): 4163-4172. doi: 10.7498/aps.53.4163
下载:
计量
- 文章访问数: 3759
- PDF下载量: 61
- 被引次数: 0
