-
The average transverse momentum
$\left\langle p_{\mathrm{T}} \right\rangle$ of final particles is an important observable in high-energy heavy-ion collision experiments. It reflects the properties of soft hadrons and thermonuclear matter, and it can also be used to deduce the information about the evolution of collision systems. By using the phenomenological linear and power-law functions, we study the dependence of the average transverse momentum$\langle p_{\mathrm{T}}\rangle$ at midrapidity in Au + Au and Pb + Pb collisions from the STAR, PHENIX and ALICE Collaborations on four normalized physical quantities, i.e. the collision centrality, the average number of binary collisions per participant pair$\dfrac{2N_{{\mathrm{coll}}}}{N_{{\mathrm{part}}}}$ , the average pseudorapidity density of charged particles per participant pair$\dfrac{2}{N_{{\mathrm{part}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta}$ and the average pseudorapidity density of charged particles per binary collision$\dfrac{1}{N_{{\mathrm{coll}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta} $ . The results show that the average transverse momentum$\langle p_{\mathrm{T}} \rangle$ of identified particles exhibits a good linear relationship with collision centrality, and it follows a nice power-law relationship with the average number of binary collisions per participant pair$\dfrac{2N_{{\mathrm{coll}}}}{N_{{\mathrm{part}}}}$ , the average pseudorapidity density of charged particles per participant pair$\dfrac{2}{N_{{\mathrm{part}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta}$ , and the average pseudorapidity density of charged particles per binary collision$\dfrac{1}{N_{{\mathrm{coll}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta}$ . It is also found that the fitting parameters in the proposed phenomenological functions for the average transverse momentum$\langle p_{\mathrm{T}} \rangle$ with collision centrality and the average number of binary collisions per participant pair follow a power-law function with collision energy, which endows the phenomenological approach with predictive ability. Therefore, the collision centrality and the average number of binary collisions per participant pair are good physical quantities for studying the average transverse momentum of identified particles in high-energy heavy-ion collisions. The results in this study can be used to predict the average transverse momentum of identified particles at other collision energy of which the experimental data are not available so far. The mass ordering of the average transverse momentum of identified particles, i.e.$\text{π}^{-},\;{\mathrm{K}}^{-} $ and$\bar{{\mathrm{p}}}$ , is also discussed and explained by the particle production time related to energy conservation, at a given collision centrality and energy.[1] Hwa R C, Wang X N 2004 Quark-Gluon Plasma 3 (Singapore: World Scientific
[2] Hwa R C, Wang X N 2010 Quark-Gluon Plasma 4 (Singapore: World Scientific
[3] L P Csernai 1994 Introduction to Relativistic Heavy Ion Collisions (New York: Wiley
[4] Adams J, Aggarwal M M, Ahammed Z, et al. 2005 Nucl. Phys. A 757 102
Google Scholar
[5] C Y Wong 1994 Introduction to High-Energy Heavy-Ion Collisions (Singapore: World Scientific
[6] Abelev B I, Adams J, Aggarwal M M, et al. 2007 Phys. Rev. C 75 064901
Google Scholar
[7] Adamczyk L, Adkins J K, Agakishiev G, et al. 2017 Phys. Rev. C 96 044904
Google Scholar
[8] Adam J, Adamczyk L, Adams J R, et al. 2020 Phys. Rev. C 101 024905
Google Scholar
[9] Abelev B I, Aggarwal M M, Ahammed Z, et al. 2009 Phys. Rev. C 79 034909
Google Scholar
[10] Abelev B, Adam J, Adamova D, et al. 2013 Phys. Rev. C 88 044910
Google Scholar
[11] Acharya S, Adamova D, Adhya S P, et al. 2020 Phys. Rev. C 101 044907
Google Scholar
[12] Adams J, Adler C, Aggarwal M M, et al. 2004 Phys. Rev. Lett. 92 052302
Google Scholar
[13] Back B B, Baker M D, Ballintijn M, et al. 2005 Nucl. Phys. A 757 28
Google Scholar
[14] Wang M, Tao J Q, Zheng H, Zhang W C, Zhu L L, Bonasera A 2022 Nucl. Sci. Tech. 33 37
Google Scholar
[15] Abelev B B, Adam J, Adamova D, et al. 2015 JHEP 2015 190
Google Scholar
[16] Adler C, Ahammed Z, Allgower C, et al. 2002 Phys. Rev. Lett. 89 202301
Google Scholar
[17] Chatrchyan S, Khachatryan V, Sirunyan A M, et al. 2014 Phys. Rev. C 90 024908
Google Scholar
[18] Qin G Y, Wang X N 2015 Int. J. Mod. Phys. E 24 1530014
Google Scholar
[19] Cao S S, Wang X N 2021 Rep. Prog. Phys. 84 024301
Google Scholar
[20] Adcox K, Adler S S, Afanasiev S, et al. 2005 Nucl. Phys. A 757 184
Google Scholar
[21] Zhang S L, Liao J, Qin G Y, Wang E, Xing H 2023 Sci. Bull. 68 2003
Google Scholar
[22] Hwa R C, Zhu L 2018 Phys. Rev. C 97 054908
Google Scholar
[23] Zhu L, Zheng H, Da K, Gong H, Ye Z, Liu G, Hwa R C 2023 Phys. Rev. C 107 064907
Google Scholar
[24] Zhu L, Zheng H, Kong R 2019 Eur. Phys. J. A 55 205
Google Scholar
[25] Tao J Q, Wang M, Zheng H, Zhang W C, Zhu L L, Bonasera A 2021 J. Phys. G 48 105102
Google Scholar
[26] Gao Y, Zheng H, Zhu L L, Bonasera A 2017 Eur. Phys. J. A 53 197
Google Scholar
[27] Tao J Q, He H B, Zheng H, Zhang W C, Liu X Q, Zhu L L, Bonasera A 2023 Nucl. Sci. Tech. 34 172
Google Scholar
[28] Zhu L, Zheng H, Hwa R C 2021 Phys. Rev. C 104 014902
Google Scholar
[29] She Z L, Lei A K, Yan Y L, Zhou D M, Zhang W C, Zheng H, Zheng L, Xie Y L, Chen G, Sa B H 2024 Phys. Rev. C 110 014910
Google Scholar
[30] Wu W H, Tao J Q, Zheng H, Zhang W C, Liu X Q, Zhu L L, Bonasera A 2023 Nucl. Sci. Tech. 34 151
Google Scholar
[31] Zhao W, Zhu L, Zheng H, Ko C M, Song H 2018 Phys. Rev. C 98 054905
Google Scholar
[32] Lin Z W, Zheng L 2021 Nucl. Sci. Tech. 32 113
Google Scholar
[33] Fu B, Liu S Y F, Pang L, Song H, Yin Y 2021 Phys. Rev. Lett. 127 142301
Google Scholar
[34] Pang L G, Petersen H, Wang X N 2018 Phys. Rev. C 97 064918
Google Scholar
[35] Ye K F, Wang Q, Shi J H, Qin Z Y, Zhang W C, Lei A K, She Z L, Yan Y L, Sa B H 2024 Phys. Rev. C 109 035201
Google Scholar
[36] Lan S W, Shi S S 2022 Nucl. Sci. Tech. 33 21
Google Scholar
[37] Zheng H, Zhu L 2016 Adv. High Energy Phys. 2016 9632126
Google Scholar
[38] Zheng H, Zhu L 2015 Adv. High Energy Phys. 2015 180491
Google Scholar
[39] Zheng H, Zhu L, Bonasera A 2015 Phys. Rev. D 92 074009
Google Scholar
[40] Zhu L L, Wang B, Wang M, Zheng H 2022 Nucl. Sci. Tech. 33 45
Google Scholar
[41] Zhu L L, Zheng H, Yang C B 2008 Nucl. Phys. A 802 122
Google Scholar
[42] Tao J, Wu W, Wang M, Zheng H, Zhang W, Zhu L, Bonasera A 2022 Particles 5 146
Google Scholar
[43] Wong C Y, Wilk G 2012 Acta Phys. Polon. B 43 2047
Google Scholar
[44] Wong C Y, Wilk G, Cirto L J L, Tsallis C 2015 Phys. Rev. D 91 114027
Google Scholar
[45] Deppman A, Megias E, Menezes D P 2020 Phys. Rev. D 101 034019
Google Scholar
[46] Yang P P, Liu F H, Olimov K K 2023 Entropy 25 1571
Google Scholar
[47] Pradhan G S, Sahu D, Rath R, Sahoo R, Cleymans J 2024 Eur. Phys. J. A 60 52
Google Scholar
[48] Wu J, Lin Y, Li Z, Luo X, Wu Y 2021 Phys. Rev. C 104 034902
Google Scholar
[49] Bernhard J E, Moreland J S, Bass S A 2019 Nat. Phys. 15 1113
Google Scholar
[50] He Y Y, Pang L G, Wang X N 2019 Phys. Rev. Lett. 122 252302
Google Scholar
[51] Heffernan M R, Gale C, Jeon S, Paquet J F 2024 Phys. Rev. C 109 065207
Google Scholar
[52] Feng Y T, Shao F L, Song J 2022 Phys. Rev. C 106 034910
Google Scholar
[53] Van Hove L 1982 Phys. Lett. B 118 138
Google Scholar
[54] Olimov K K, Liu F H, Musaev K A, Olimov A K, Tukhtaev B J, Saidkhanov N S, Yuldashev B S, Olimov K, Gulamov K G 2021 Int. J. Mod. Phys. E 30 2150029
Google Scholar
[55] Olimov K K, Lebedev I A, Tukhtaev B J, Fedosimova A I, Liu F H, Khudoyberdieva S A, Kanokova S Z 2023 Int. J. Mod. Phys. E 32 2350066
Google Scholar
[56] ALICE Publications 2018 https://cds.cern.ch/record/2636623
[57] Aamodt K, Abrahantes Quintana A, Adamova D, et al. 2011 Phys. Rev. Lett. 106 032301
Google Scholar
[58] Adam J, Adamova D, Aggarwal M M, et al. 2016 Phys. Rev. Lett. 116 222302
Google Scholar
[59] Adare A, Afanasiev S, Aidala C, et al. 2016 Phys. Rev. C 93 024901
Google Scholar
-
图 1 采用线性函数公式(1)对不同碰撞能量下, 中心快度区鉴别粒子的平均横动量$ \langle p_{\mathrm{T}} \rangle $与碰撞中心度关系的拟合结果. 金核-金核碰撞能量为7.7 GeV (a); 11.5 GeV (b); 14.5 GeV (c); 19.6 GeV (d); 27 GeV (e); 39 GeV (f); 62.4 GeV (g); 130 GeV (h); 200 GeV (i). 铅核-铅核碰撞能量为2.76 TeV (j); 5.02 TeV (k). 实验数据来自文献[7—11]
Figure 1. Linear fits with Eq. (1) to the experimental midrapidity $ \langle p_{\mathrm{T}} \rangle $ versus centrality for the identified particles in Au + Au collisions at $ \sqrt{s_{{{\rm NN}}}}={\rm{7.7\;GeV}}$ (a), 11.5 GeV (b), 14.5 GeV (c), 19.6 GeV (d), 27 GeV (e), 39 GeV (f), 62.4 GeV (g), 130 GeV (h), 200 GeV (i), and in Pb + Pb collisions at $ \sqrt{s_{{{\rm NN}}}}={\rm{2.76\;TeV}}$ (j), 5.02 TeV (k). The experimental data are taken from Refs. [7–11].
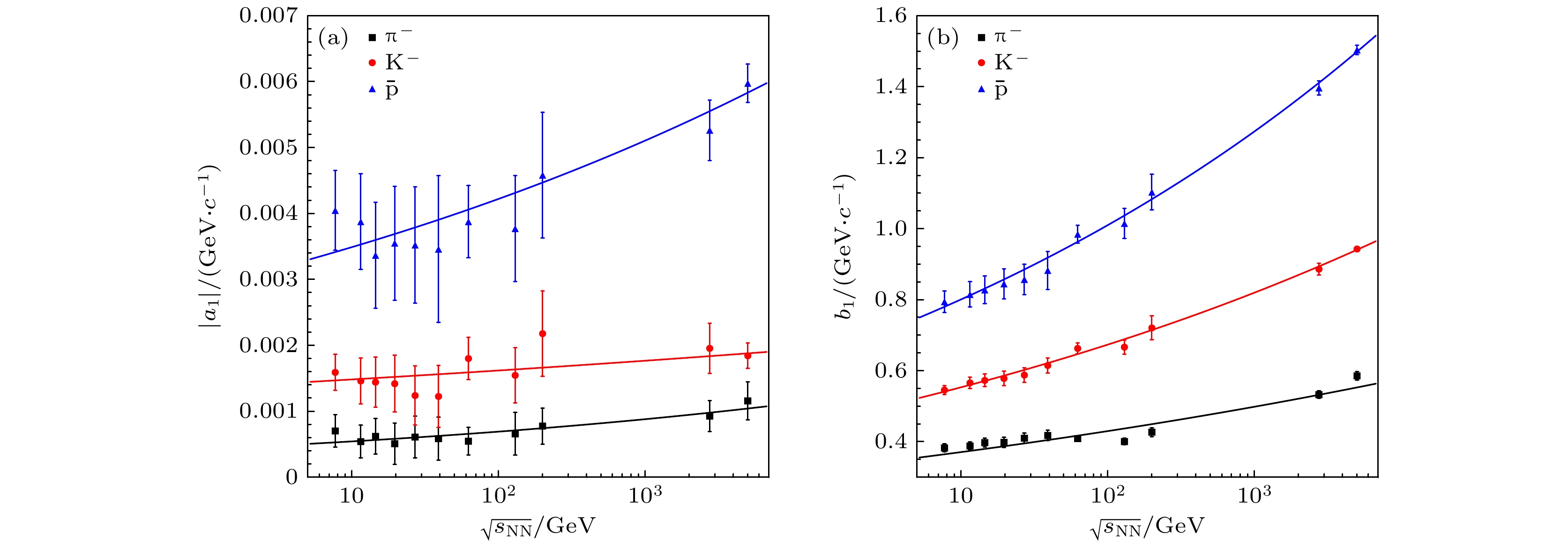
图 2 (1)式拟合鉴别粒子平均横动量$ \langle p_{\mathrm{T}} \rangle $随碰撞中心度关系的拟合参数 (a) 斜率绝对值$ |a_1 | $; (b) 截距 $ b_1 $与每核子对质心碰撞能量$ \sqrt{s_{{{\rm NN}}}} $的关系
Figure 2. The collision energy $ \sqrt{s_{{{\rm NN}}}} $ dependence of the fitting parameters from Eq. (1): (a) For the absolute values of slope $ |a_1 | $; (b) for the intercepts $ b_1 $.
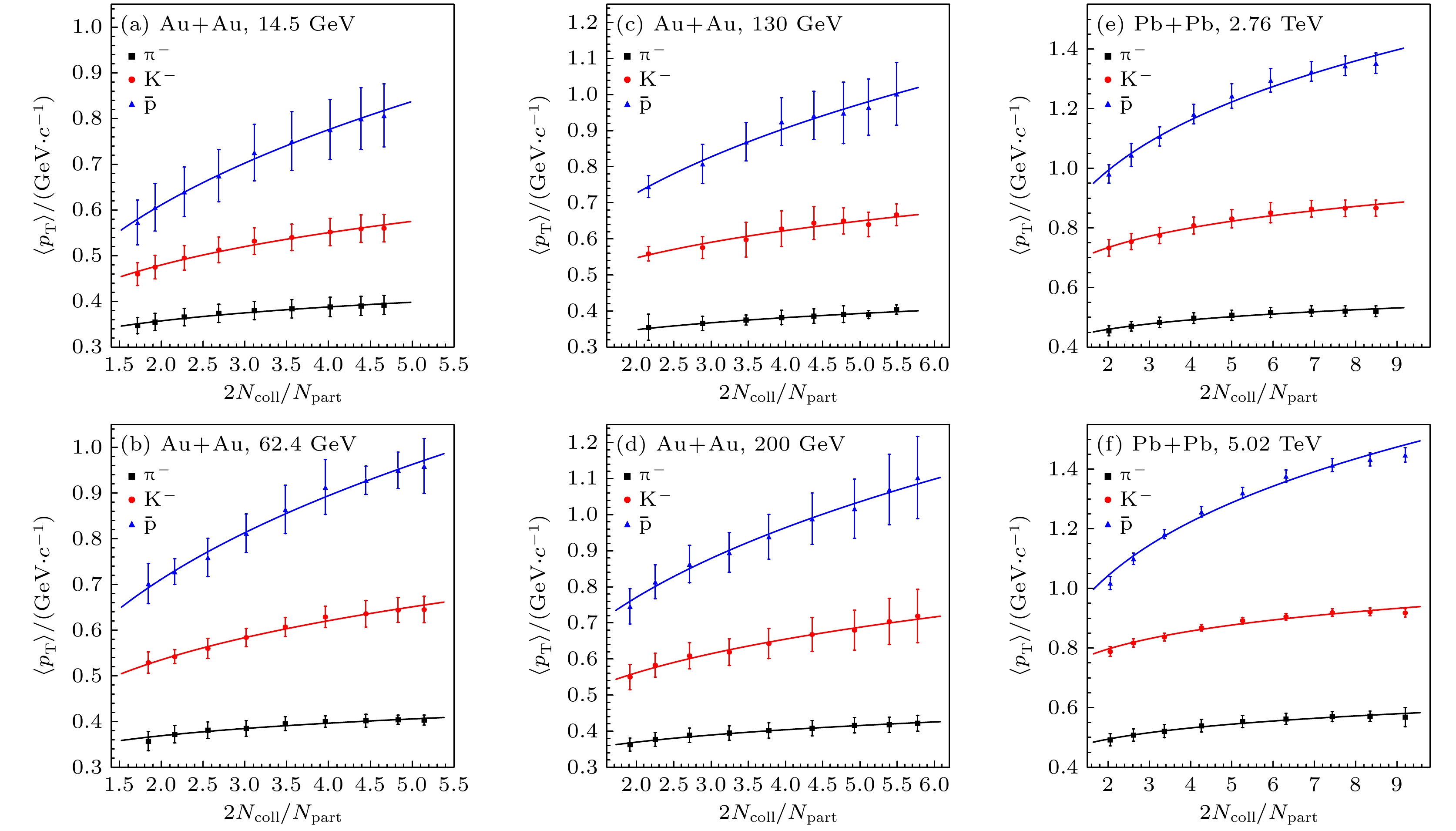
图 3 采用幂律函数公式(2)拟合不同碰撞能量下, 中心快度区鉴别粒子平均横动量$ \langle p_{\mathrm{T}} \rangle $与每核子对的平均碰撞次数关系的拟合结果. 金核-金核碰撞能量为14.5 GeV (a); 62.4 GeV (b); 130 GeV (c); 200 GeV (d). 铅核-铅核碰撞能量为2.76 TeV (e); 5.02 TeV (f). 实验数据来自文献[8—11, 56]
Figure 3. Power-law fits with Eq. (2) to the experimental midrapidity $ \langle p_{\mathrm{T}} \rangle $ versus $ {2 N_{{\mathrm{coll}}}}/{N_{{\mathrm{part}}}} $ for the identified particles in Au + Au collisions at $ \sqrt{s_{{{\rm NN}}}}={\rm{14.5\;GeV}}$ (a), 62.4 GeV (b), 130 GeV (c), 200 GeV (d), and in Pb + Pb collisions at $ \sqrt{s_{{{\rm NN}}}}={\rm{2.76\;TeV}}$ (e), 5.02 TeV (f). The experimental data are taken from Refs. [8–11, 56].
图 5 采用幂律函数公式(3)拟合不同碰撞能量下, 中心快度区的平均横动量$ \langle p_{\mathrm{T}} \rangle $与每核子对平均产生的带电粒子多重数赝快度密度关系的拟合结果. 金核-金核碰撞能量$ \sqrt{s_{{{\rm NN}}}}={\rm{7.7\;GeV}}$ (a); 62.4 GeV (b); 200 GeV (c). 铅核-铅核碰撞能量$ \sqrt{s_{{{\rm NN}}}}= $$ {\rm{2.76\;TeV}}$(d). 实验数据来自文献[7—11, 56—59]
Figure 5. Power-law fits with Eq. (3) to the experimental midrapidity $ \langle p_{\mathrm{T}} \rangle $ versus $ \dfrac{2}{N_{{\mathrm{part}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta} $ for the identified particles in Au + Au collisions at $ \sqrt{s_{{{\rm NN}}}}={\rm{7.7\;GeV}}$ (a), 62.4 GeV (b), 200 GeV (c), and in Pb + Pb collisions at $ \sqrt{s_{{{\rm NN}}}}={\rm{2.76\;TeV}}$ (d). The experimental data are taken from Refs. [7–11, 56–59].
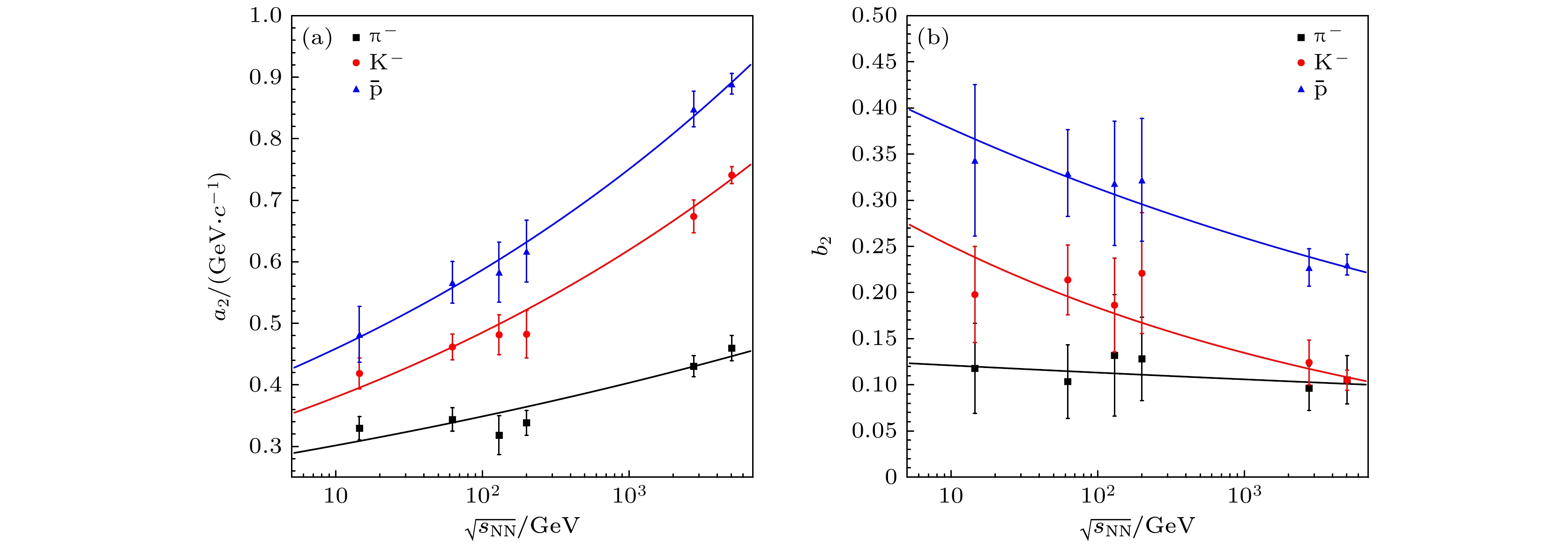
图 6 (3)式拟合鉴别粒子平均横动量$ \langle p_{\mathrm{T}}\rangle $随每核子对平均产生的带电粒子多重数赝快度密度的拟合参数(a)系数$ a_3 $和(b)指数$ b_3 $与每核子对质心碰撞能量$ \sqrt{s_{{{\rm NN}}}} $的关系
Figure 6. Collision energy $ \sqrt{s_{{{\rm NN}}}} $ dependence of the fitting parameters from Eq. (3): (a) For the coefficient $ a_3 $; (b) for the power $ b_3 $
图 7 采用幂律函数公式(4)拟合不同碰撞能量下, 中心快度区的平均横动量$ \langle p_{\mathrm{T}}\rangle $与每次碰撞平均产生的带电粒子多重数赝快度密度关系的拟合结果. 金核-金核碰撞能量$ \sqrt{s_{{{\rm NN}}}}={\rm{14.5\;GeV}}$ (a); 62.4 GeV (b); 130 GeV (c); 200 GeV (d). 铅核-铅核碰撞能量$ \sqrt{s_{{{\rm NN}}}}={\rm{2.76\;TeV}}$ (e); 5.02 TeV (f). 实验数据来自文献[8—11, 56—59]
Figure 7. Power-law fits with Eq. (4) to the experimental midrapidity $ \langle p_{\mathrm{T}}\rangle $ versus $ \frac{1}{N_{{\mathrm{coll}}}}\frac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta} $ for the identified particles in Au + Au collisions at $ \sqrt{s_{{{\rm NN}}}}={\rm{14.5\;GeV}}$ (a), 62.4 GeV (b), 130 GeV (c), 200 GeV (d), and in Pb + Pb collisions at $ \sqrt{s_{{{\rm NN}}}}={\rm{2.76\;TeV}}$ (e), 5.02 TeV (f). The experimental data are taken from Refs. [8–11, 56–59].
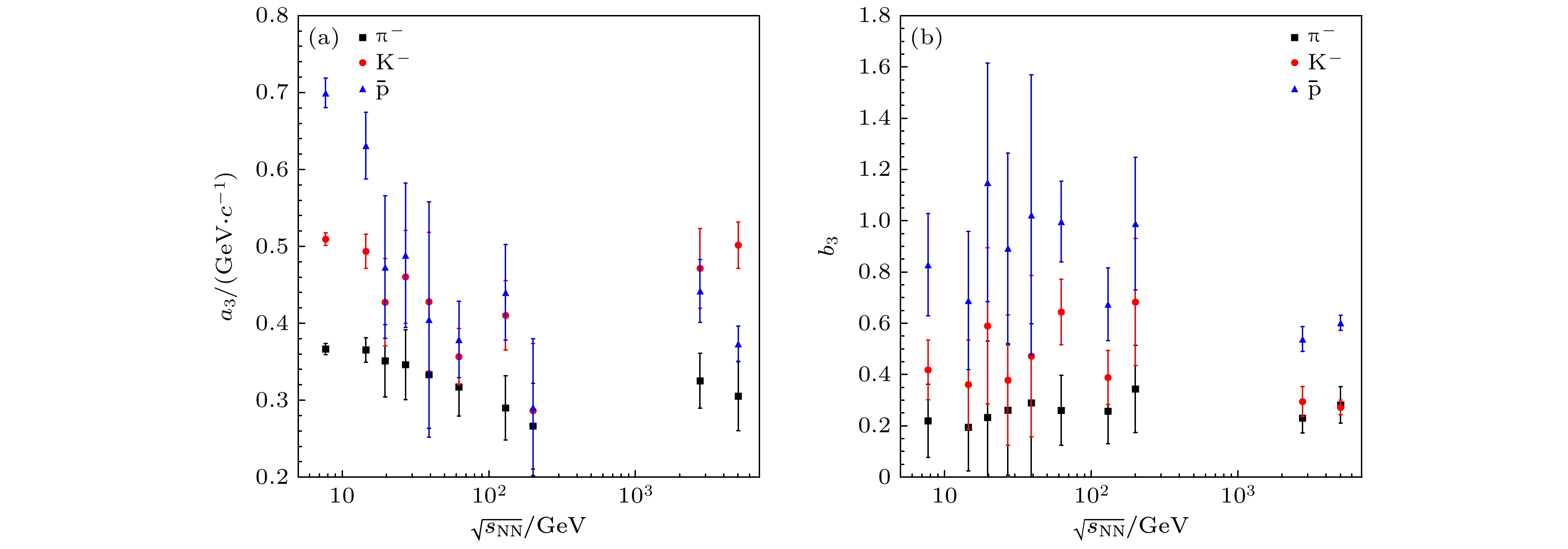
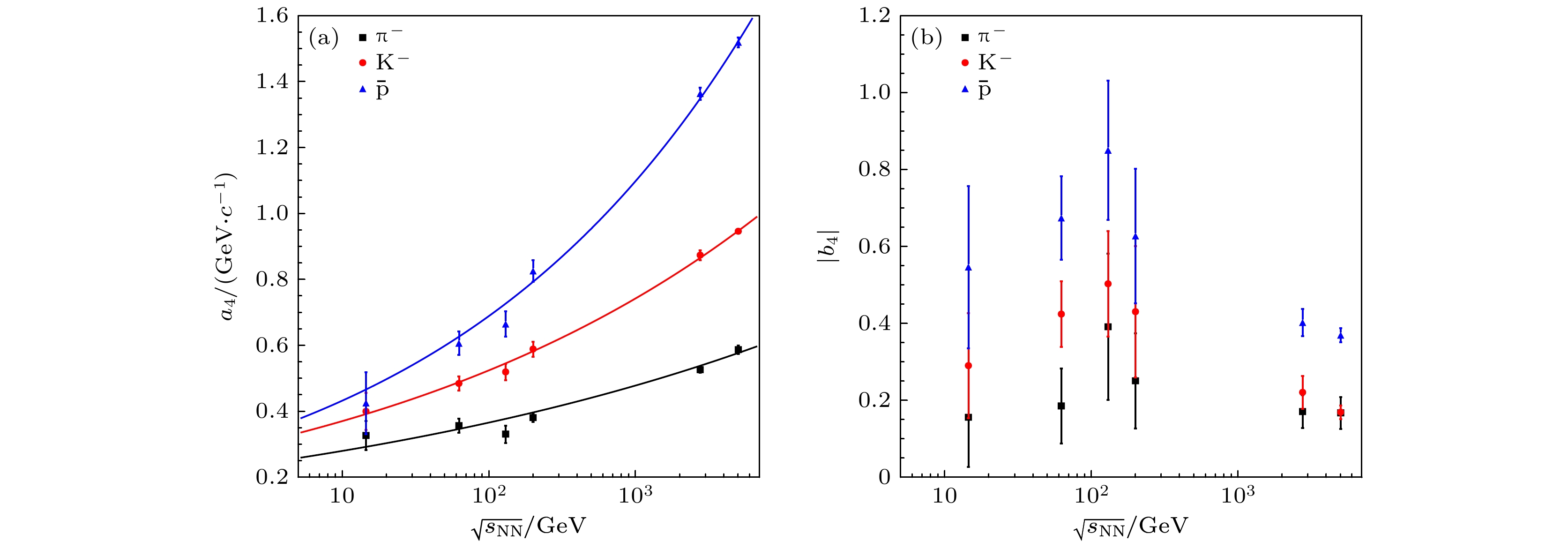
图 8 (4)式拟合鉴别粒子平均横动量$ \langle p_{\mathrm{T}}\rangle $随每次碰撞平均产生的带电粒子多重数赝快度密度的拟合参数(a)系数$ a_4 $和(b)指数$ b_4 $与每核子对质心碰撞能量$ \sqrt{s_{{{\rm NN}}}} $的关系
Figure 8. Collision energy $ \sqrt{s_{{{\rm NN}}}} $ dependence of the fitting parameters from Eq. (4): (a) For the coefficient $ a_4 $; (b) for the power $ b_4 $
表 A1 (1)式拟合鉴别粒子平均横动量$ \langle p_{\mathrm{T}}\rangle $与碰撞中心度C关系的拟合参数及相应的$ \chi^2/{\rm NDF} $
Table A1. Fitting parameters of the $ \langle p_{\mathrm{T}}\rangle $ versus centrality for the identified particles from Eq. (1) and the corresponding $ \chi^2/{\rm NDF} $.
碰撞系统, 碰撞能量 粒子种类 截距$ b_1 /(\mathrm{GeV}\cdot c^{-1}) $ 斜率$ a_1 /(\mathrm{GeV}\cdot c^{-1}) $ $ \chi^2/{\rm NDF} $ $ \text{π}^{-} $ $ 0.382\pm 0.011 $ $ -7.01\times10^{-4} \pm 2.47\times10^{-4} $ 0.311/7 Au+Au, 7.7 GeV $ {\mathrm{K}}^{-} $ $ 0.545 \pm 0.013 $ $ -1.59\times10^{-3}\pm 2.72\times10^{-4} $ 0.337/7 $ \bar{{\mathrm{p}}} $ $ 0.794\pm 0.030 $ $ -4.05\times10^{-3} \pm 6.07\times10^{-4} $ 0.211/7 $ \text{π}^{-} $ $ 0.388\pm 0.011 $ $ -5.39\times10^{-4} \pm 2.50\times10^{-4} $ 0.397/7 Au+Au, 11.5 GeV $ {\mathrm{K}}^{-} $ $ 0.566 \pm 0.016 $ $ -1.46\times10^{-3}\pm 3.47\times10^{-4} $ 0.531/7 $ \bar{{\mathrm{p}}} $ $ 0.815\pm0.036 $ $ -3.87\times10^{-3} \pm 7.24\times10^{-4} $ 0.097/7 $ \text{π}^{-} $ $ 0.397\pm 0.012 $ $ -6.19\times10^{-4} \pm 2.69\times10^{-4} $ 0.238/7 Au+Au, 14.5 GeV $ {\mathrm{K}}^{-} $ $ 0.572 \pm 0.018 $ $ -1.44\times10^{-3}\pm 3.79\times10^{-4} $ 0.323/7 $ \bar{{\mathrm{p}}} $ $ 0.827\pm0.039 $ $ -3.37\times10^{-3} \pm 8.04\times10^{-4} $ 0.122/7 $ \text{π}^{-} $ $ 0.398\pm 0.014 $ $ -5.08\times10^{-4} \pm 3.12\times10^{-4} $ 0.195/7 Au+Au, 19.6 GeV $ {\mathrm{K}}^{-} $ $ 0.578 \pm 0.020 $ $ -1.42\times10^{-3}\pm 4.30\times10^{-4} $ 0.149/7 $ \bar{{\mathrm{p}}} $ $ 0.845\pm0.042 $ $ -3.55\times10^{-3} \pm 8.64\times10^{-4} $ 0.066/7 $ \text{π}^{-} $ $ 0.410\pm 0.014 $ $ -6.08\times10^{-4} \pm 3.19\times10^{-4} $ 0.093/7 Au+Au, 27 GeV $ {\mathrm{K}}^{-} $ $ 0.588 \pm 0.020 $ $ -1.24\times10^{-3}\pm 4.48\times10^{-4} $ 0.179/7 $ \bar{{\mathrm{p}}} $ $ 0.857\pm0.043 $ $ -3.52\times10^{-3} \pm 8.81\times10^{-4} $ 0.134/7 $ \text{π}^{-} $ $ 0.417\pm 0.015 $ $ -5.84\times10^{-4} \pm3.25\times10^{-4} $ 0.151/7 Au+Au, 39 GeV $ {\mathrm{K}}^{-} $ $ 0.615 \pm 0.021 $ $ -1.22\times10^{-3}\pm 4.71\times10^{-4} $ 0.138/7 $ \bar{{\mathrm{p}}} $ $ 0.882\pm 0.054 $ $ -3.46\times10^{-3} \pm 1.11\times10^{-3} $ 0.091/7 $ \text{π}^{-} $ $ 0.409\pm 0.007 $ $ -5.46\times10^{-4} \pm 2.11\times10^{-4} $ 0.755/7 Au+Au, 62.4 GeV $ {\mathrm{K}}^{-} $ $ 0.663\pm0.016 $ $ -1.80\times10^{-3}\pm 3.20\times10^{-4} $ 0.712/7 $ \bar{{\mathrm{p}}} $ $ 0.984\pm 0.025 $ $ -3.87\times10^{-3} \pm 5.46\times10^{-4} $ 0.501/7 $ \text{π}^{-} $ $ 0.400\pm0.009 $ $ -6.57\times10^{-4} \pm 3.24\times10^{-4} $ 0.384/6 Au+Au, 130 GeV $ {\mathrm{K}}^{-} $ $ 0.666 \pm 0.020 $ $ -1.54\times10^{-3} \pm 4.19\times10^{-4} $ 0.478/6 $ \bar{{\mathrm{p}}} $ $ 1.01\pm 0.042 $ $ -3.77\times10^{-3}\pm8.05\times10^{-4} $ 0.275/6 $ \text{π}^{-} $ $ 0.427\pm0.012 $ $ -7.75\times10^{-4} \pm 2.73\times10^{-4} $ 0.234/7 Au+Au, 200 GeV $ {\mathrm{K}}^{-} $ $ 0.720\pm0.033 $ $ -2.18 \times10^{-3} \pm 6.49\times10^{-4} $ 0.145/7 $ \bar{{\mathrm{p}}} $ $ 1.10\pm0.050 $ $ -4.58\times10^{-3}\pm 9.55\times10^{-4} $ 0.222/7 $ \text{π}^{-} $ $ 0.532\pm0.010 $ $ -9.28\times10^{-4} \pm 2.34\times10^{-4} $ 1.099/7 Pb+Pb, 2.76 TeV $ {\mathrm{K}}^{-} $ $ 0.886 \pm 0.017 $ $ -1.95\times10^{-3} \pm 3.80\times10^{-4} $ 0.960/7 $ \bar{{\mathrm{p}}} $ $ 1.40\pm 0.020 $ $ -5.26\times10^{-3}\pm 4.58\times10^{-4} $ 3.124/7 $ \text{π}^{-} $ $ 0.586\pm0.012 $ $ -1.16\times10^{-3} \pm 2.88\times10^{-4} $ 0.707/7 Pb+Pb, 5.02 TeV $ {\mathrm{K}}^{-} $ $ 0.943 \pm 0.008 $ $ -1.84\times10^{-3} \pm 1.93\times10^{-4} $ 6.723/7 $ \bar{{\mathrm{p}}} $ $ 1.50\pm 0.013 $ $ -5.97\times10^{-3}\pm 2.91\times10^{-4} $ 12.752/7 
DownLoad: CSV
表 A2 (2)式拟合鉴别粒子平均横动量$ \langle p_{\mathrm{T}}\rangle $与每核子对的平均碰撞次数$ {2 N_{{\mathrm{coll}}}}/{N_{{\mathrm{part}}}} $关系的拟合参数及相应的$ \chi^2/{\rm NDF} $
Table A2. Fitting parameters of the $ \langle p_{\mathrm{T}}\rangle $ versus $ {2 N_{{\mathrm{coll}}}}/{N_{{\mathrm{part}}}} $ for the identified particles from Eq. (2) and the corresponding $ \chi^2/{\rm NDF} $.
碰撞系统, 碰撞能量 粒子种类 系数$ a_2/(\mathrm{GeV}\cdot c^{-1})$ 指数$ b_2 $ $ \chi^2/{\rm NDF} $ $ \text{π}^{-} $ $ 0.330\pm0.019 $ $ 0.118\pm 0.049 $ 0.180/7 Au+Au, 14.5 GeV $ {\mathrm{K}}^{-} $ $ 0.418\pm0.025 $ $ 0.198\pm 0.052 $ 0.235/7 $ \bar{{\mathrm{p}}} $ $ 0.482\pm 0.045 $ $ 0.343\pm 0.082 $ 0.110/7 $ \text{π}^{-} $ $ 0.344\pm0.019 $ $ 0.104\pm 0.040 $ 0.519/7 Au+Au, 62.4 GeV $ {\mathrm{K}}^{-} $ $ 0.462\pm0.021 $ $ 0.214\pm 0.038 $ 0.413/7 $ \bar{{\mathrm{p}}} $ $ 0.566\pm 0.034 $ $ 0.330\pm 0.047 $ 0.352/7 $ \text{π}^{-} $ $ 0.318\pm0.032 $ $ 0.132\pm 0.066 $ 0.375/6 Au+Au, 130 GeV $ {\mathrm{K}}^{-} $ $ 0.481\pm 0.033 $ $ 0.186 \pm0.051 $ 0.448/6 $ \bar{{\mathrm{p}}} $ $ 0.583\pm 0.049 $ $ 0.318\pm 0.067 $ 0.215/6 $ \text{π}^{-} $ $ 0.338\pm0.020 $ $ 0.128 \pm 0.045 $ 0.149/7 Au+Au, 200 GeV $ {\mathrm{K}}^{-} $ $ 0.482\pm0.038 $ $ 0.221\pm 0.065 $ 0.184/7 $ \bar{{\mathrm{p}}} $ $ 0.617\pm 0.050 $ $ 0.322 \pm 0.066 $ 0.304/7 $ \text{π}^{-} $ $ 0.430\pm0.017 $ $ 0.096 \pm 0.024 $ 0.623/7 Pb+Pb, 2.76 TeV $ {\mathrm{K}}^{-} $ $ 0.674 \pm 0.027 $ $ 0.124 \pm 0.024 $ 0.527/7 $ \bar{{\mathrm{p}}} $ $ 0.848\pm 0.029 $ $ 0.227\pm 0.020 $ 1.731/7 $ \text{π}^{-} $ $ 0.460\pm 0.020 $ $ 0.105\pm 0.026 $ 0.405/7 Pb+Pb, 5.02 TeV $ {\mathrm{K}}^{-} $ $ 0.741 \pm 0.014 $ $ 0.105\pm 0.011 $ 3.765/7 $ \bar{{\mathrm{p}}} $ $ 0.889\pm 0.017 $ $ 0.230\pm 0.011 $ 7.564/7
DownLoad: CSV
表 A3 (3)式拟合鉴别粒子平均横动量$ \langle p_{\mathrm{T}}\rangle $随每核子对平均产生的带电粒子多重数赝快度密度$ \dfrac{2}{N_{{\mathrm{part}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta} $的拟合参数及相应的$ \chi^2/{\rm NDF} $
Table A3. Fitting parameters of the $ \langle p_{\mathrm{T}}\rangle $ versus $ \dfrac{2}{N_{{\mathrm{part}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta} $ for the identified particles from Eq. (3) and the corresponding $ \chi^2/{\rm NDF} $.
碰撞系统, 碰撞能量 粒子种类 系数$ a_3 /(\mathrm{GeV}\cdot c^{-1})$ 指数$ b_3 $ $ \chi^2/{\rm NDF} $ $ \text{π}^{-} $ $ 0.366\pm0.007 $ $ 0.220\pm 0.142 $ 0.263/5 Au+Au, 7.7 GeV $ {\mathrm{K}}^{-} $ $ 0.509\pm 0.008 $ $ 0.418\pm 0.117 $ 0.551/5 $ \bar{{\mathrm{p}}} $ $ 0.700\pm 0.019 $ $ 0.828 \pm 0.200 $ 0.548/5 $ \text{π}^{-} $ $ 0.366\pm0.01 $6 $ 0.195\pm 0.170 $ 0.063/5 Au+Au, 14.5 GeV $ {\mathrm{K}}^{-} $ $ 0.494\pm 0.022 $ $ 0.361\pm 0.174 $ 0.237/5 $ \bar{{\mathrm{p}}} $ $ 0.631\pm 0.044 $ $ 0.689 \pm 0.269 $ 0.235/5 $ \text{π}^{-} $ $ 0.351\pm0.047 $ $ 0.232\pm 0.299 $ 0.105/5 Au+Au, 19.6 GeV $ {\mathrm{K}}^{-} $ $ 0.427\pm 0.057 $ $ 0.590\pm 0.304 $ 0.462/5 $ \bar{{\mathrm{p}}} $ $ 0.473\pm 0.093 $ $ 1.15 \pm 0.465 $ 0.621/5 $ \text{π}^{-} $ $ 0.346\pm0.045 $ $ 0.261\pm 0.254 $ 0.081/5 Au+Au, 27 GeV $ {\mathrm{K}}^{-} $ $ 0.460\pm0.060 $ $ 0.378\pm 0.254 $ 0.123/5 $ \bar{{\mathrm{p}}} $ $ 0.489\pm 0.094 $ $ 0.893 \pm 0.371 $ 0.245/5 $ \text{π}^{-} $ $ 0.333\pm0.070 $ $ 0.290\pm 0.309 $ 0.083/5 Au+Au, 39 GeV $ {\mathrm{K}}^{-} $ $ 0.428\pm 0.090 $ $ 0.472\pm 0.315 $ 0.146/5 $ \bar{{\mathrm{p}}} $ $ 0.405\pm 0.153 $ $ 1.02 \pm 0.546 $ 0.142/5 $ \text{π}^{-} $ $ 0.317\pm0.038 $ $ 0.260\pm 0.136 $ 0.331/6 Au+Au, 62.4 GeV $ {\mathrm{K}}^{-} $ $ 0.357\pm 0.036 $ $ 0.644\pm 0.127 $ 0.662/6 $ \bar{{\mathrm{p}}} $ $ 0.379\pm 0.050 $ $ 0.997 \pm 0.158 $ 0.507/6 $ \text{π}^{-} $ $ 0.290\pm0.042 $ $ 0.257\pm 0.127 $ 0.356/6 Au+Au, 130 GeV $ {\mathrm{K}}^{-} $ $ 0.410\pm 0.045 $ $ 0.388\pm 0.105 $ 0.452/6 $ \bar{{\mathrm{p}}} $ $ 0.440\pm 0.062 $ $ 0.674 \pm 0.142 $ 0.481/6 $ \text{π}^{-} $ $ 0.266\pm0.056 $ $ 0.344 \pm 0.171 $ 0.278/6 Au+Au, 200 GeV $ {\mathrm{K}}^{-} $ $ 0.286\pm0.087 $ $ 0.683\pm 0.247 $ 0.190/6 $ \bar{{\mathrm{p}}} $ $ 0.291\pm 0.089 $ $ 0.989 \pm0.259 $ 0.383/6 $ \text{π}^{-} $ $ 0.325\pm 0.036 $ $ 0.230 \pm 0.058 $ 0.862/7 Pb+Pb, 2.76 TeV $ {\mathrm{K}}^{-} $ $ 0.471\pm0.052 $ $ 0.295\pm 0.058 $ 1.182/7 $ \bar{{\mathrm{p}}} $ $ 0.442\pm0.041 $ $ 0.538\pm 0.048 $ 3.699/7 $ \text{π}^{-} $ $ 0.305\pm0.045 $ $ 0.282\pm 0.071 $ 0.924/7 Pb+Pb, 5.02 TeV $ {\mathrm{K}}^{-} $ $ 0.502\pm 0.030 $ $ 0.272\pm 0.029 $ 8.162/7 $ \bar{{\mathrm{p}}} $ $ 0.373\pm0.023 $ $ 0.602\pm 0.030 $ 20.985/7
DownLoad: CSV
表 A4 (4)式拟合鉴别粒子平均横动量$ \langle p_{\mathrm{T}}\rangle $随每次碰撞平均产生的带电粒子多重数赝快度密度$ \dfrac{1}{N_{{\mathrm{coll}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta} $的拟合参数及相应的$ \chi^2/{\rm NDF} $
Table A4. Fitting parameters of the $ \langle p_{\mathrm{T}}\rangle $ versus $ \dfrac{1}{N_{{\mathrm{coll}}}}\dfrac{{\mathrm{d}}N_{{\mathrm{ch}}}}{{\mathrm{d}}\eta} $ for the identified particles from Eq. (4) and the corresponding $ \chi^2/{\rm NDF} $.
碰撞系统, 碰撞能量 粒子种类 系数$ a_4 /(\mathrm{GeV}\cdot c^{-1}) $ 指数$ b_4 $ $ \chi^2/{\rm NDF} $ $ \text{π}^{-} $ $ 0.326\pm 0.044 $ $ -0.156\pm 0.128 $ 0.001/5 Au+Au, 14.5 GeV $ {\mathrm{K}}^{-} $ $ 0.400\pm0.056 $ $ -0.290\pm 0.137 $ 0.026/5 $ \bar{{\mathrm{p}}} $ $ 0.425\pm0.094 $ $ -0.547\pm 0.211 $ 0.052/5 $ \text{π}^{-} $ $ 0.357\pm0.021 $ $ -0.185\pm 0.098 $ 0.411/6 Au+Au, 62.4 GeV $ {\mathrm{K}}^{-} $ $ 0.484\pm0.021 $ $ -0.424\pm 0.086 $ 1.108/6 $ \bar{{\mathrm{p}}} $ $ 0.606\pm 0.036 $ $ -0.674 \pm 0.109 $ 1.402/6 $ \text{π}^{-} $ $ 0.331\pm0.026 $ $ -0.391 \pm 0.190 $ 0.347/6 Au+Au, 130 GeV $ {\mathrm{K}}^{-} $ $ 0.519\pm0.025 $ $ -0.503\pm 0.137 $ 0.720/6 $ \bar{{\mathrm{p}}} $ $ 0.665\pm 0.038 $ $ -0.850\pm 0.181 $ 0.384/6 $ \text{π}^{-} $ $ 0.381\pm0.013 $ $ -0.251 \pm 0.124 $ 0.053/6 Au+Au, 200 GeV $ {\mathrm{K}}^{-} $ $ 0.589\pm0.023 $ $ -0.430\pm 0.170 $ 0.420/6 $ \bar{{\mathrm{p}}} $ $ 0.826\pm 0.033 $ $ -0.627\pm 0.174 $ 0.704/6 $ \text{π}^{-} $ $ 0.526\pm 0.009 $ $ -0.171\pm 0.042 $ 0.511/7 Pb+Pb, 2.76 TeV $ {\mathrm{K}}^{-} $ $ 0.874 \pm0.015 $ $ -0.221 \pm 0.043 $ 0.353/7 $ \bar{{\mathrm{p}}} $ $ 1.36 \pm 0.018 $ $ -0.402\pm 0.036 $ 1.187/7 $ \text{π}^{-} $ $ 0.587\pm 0.013 $ $ -0.167\pm 0.041 $ 0.204/7 Pb+Pb, 5.02 TeV $ {\mathrm{K}}^{-} $ $ 0.946\pm 0.008 $ $ -0.169\pm 0.018 $ 1.997/7 $ \bar{{\mathrm{p}}} $ $ 1.52\pm 0.014 $ $ -0.369\pm 0.018 $ 2.886/7
DownLoad: CSV
-
[1] Hwa R C, Wang X N 2004 Quark-Gluon Plasma 3 (Singapore: World Scientific
[2] Hwa R C, Wang X N 2010 Quark-Gluon Plasma 4 (Singapore: World Scientific
[3] L P Csernai 1994 Introduction to Relativistic Heavy Ion Collisions (New York: Wiley
[4] Adams J, Aggarwal M M, Ahammed Z, et al. 2005 Nucl. Phys. A 757 102
Google Scholar
[5] C Y Wong 1994 Introduction to High-Energy Heavy-Ion Collisions (Singapore: World Scientific
[6] Abelev B I, Adams J, Aggarwal M M, et al. 2007 Phys. Rev. C 75 064901
Google Scholar
[7] Adamczyk L, Adkins J K, Agakishiev G, et al. 2017 Phys. Rev. C 96 044904
Google Scholar
[8] Adam J, Adamczyk L, Adams J R, et al. 2020 Phys. Rev. C 101 024905
Google Scholar
[9] Abelev B I, Aggarwal M M, Ahammed Z, et al. 2009 Phys. Rev. C 79 034909
Google Scholar
[10] Abelev B, Adam J, Adamova D, et al. 2013 Phys. Rev. C 88 044910
Google Scholar
[11] Acharya S, Adamova D, Adhya S P, et al. 2020 Phys. Rev. C 101 044907
Google Scholar
[12] Adams J, Adler C, Aggarwal M M, et al. 2004 Phys. Rev. Lett. 92 052302
Google Scholar
[13] Back B B, Baker M D, Ballintijn M, et al. 2005 Nucl. Phys. A 757 28
Google Scholar
[14] Wang M, Tao J Q, Zheng H, Zhang W C, Zhu L L, Bonasera A 2022 Nucl. Sci. Tech. 33 37
Google Scholar
[15] Abelev B B, Adam J, Adamova D, et al. 2015 JHEP 2015 190
Google Scholar
[16] Adler C, Ahammed Z, Allgower C, et al. 2002 Phys. Rev. Lett. 89 202301
Google Scholar
[17] Chatrchyan S, Khachatryan V, Sirunyan A M, et al. 2014 Phys. Rev. C 90 024908
Google Scholar
[18] Qin G Y, Wang X N 2015 Int. J. Mod. Phys. E 24 1530014
Google Scholar
[19] Cao S S, Wang X N 2021 Rep. Prog. Phys. 84 024301
Google Scholar
[20] Adcox K, Adler S S, Afanasiev S, et al. 2005 Nucl. Phys. A 757 184
Google Scholar
[21] Zhang S L, Liao J, Qin G Y, Wang E, Xing H 2023 Sci. Bull. 68 2003
Google Scholar
[22] Hwa R C, Zhu L 2018 Phys. Rev. C 97 054908
Google Scholar
[23] Zhu L, Zheng H, Da K, Gong H, Ye Z, Liu G, Hwa R C 2023 Phys. Rev. C 107 064907
Google Scholar
[24] Zhu L, Zheng H, Kong R 2019 Eur. Phys. J. A 55 205
Google Scholar
[25] Tao J Q, Wang M, Zheng H, Zhang W C, Zhu L L, Bonasera A 2021 J. Phys. G 48 105102
Google Scholar
[26] Gao Y, Zheng H, Zhu L L, Bonasera A 2017 Eur. Phys. J. A 53 197
Google Scholar
[27] Tao J Q, He H B, Zheng H, Zhang W C, Liu X Q, Zhu L L, Bonasera A 2023 Nucl. Sci. Tech. 34 172
Google Scholar
[28] Zhu L, Zheng H, Hwa R C 2021 Phys. Rev. C 104 014902
Google Scholar
[29] She Z L, Lei A K, Yan Y L, Zhou D M, Zhang W C, Zheng H, Zheng L, Xie Y L, Chen G, Sa B H 2024 Phys. Rev. C 110 014910
Google Scholar
[30] Wu W H, Tao J Q, Zheng H, Zhang W C, Liu X Q, Zhu L L, Bonasera A 2023 Nucl. Sci. Tech. 34 151
Google Scholar
[31] Zhao W, Zhu L, Zheng H, Ko C M, Song H 2018 Phys. Rev. C 98 054905
Google Scholar
[32] Lin Z W, Zheng L 2021 Nucl. Sci. Tech. 32 113
Google Scholar
[33] Fu B, Liu S Y F, Pang L, Song H, Yin Y 2021 Phys. Rev. Lett. 127 142301
Google Scholar
[34] Pang L G, Petersen H, Wang X N 2018 Phys. Rev. C 97 064918
Google Scholar
[35] Ye K F, Wang Q, Shi J H, Qin Z Y, Zhang W C, Lei A K, She Z L, Yan Y L, Sa B H 2024 Phys. Rev. C 109 035201
Google Scholar
[36] Lan S W, Shi S S 2022 Nucl. Sci. Tech. 33 21
Google Scholar
[37] Zheng H, Zhu L 2016 Adv. High Energy Phys. 2016 9632126
Google Scholar
[38] Zheng H, Zhu L 2015 Adv. High Energy Phys. 2015 180491
Google Scholar
[39] Zheng H, Zhu L, Bonasera A 2015 Phys. Rev. D 92 074009
Google Scholar
[40] Zhu L L, Wang B, Wang M, Zheng H 2022 Nucl. Sci. Tech. 33 45
Google Scholar
[41] Zhu L L, Zheng H, Yang C B 2008 Nucl. Phys. A 802 122
Google Scholar
[42] Tao J, Wu W, Wang M, Zheng H, Zhang W, Zhu L, Bonasera A 2022 Particles 5 146
Google Scholar
[43] Wong C Y, Wilk G 2012 Acta Phys. Polon. B 43 2047
Google Scholar
[44] Wong C Y, Wilk G, Cirto L J L, Tsallis C 2015 Phys. Rev. D 91 114027
Google Scholar
[45] Deppman A, Megias E, Menezes D P 2020 Phys. Rev. D 101 034019
Google Scholar
[46] Yang P P, Liu F H, Olimov K K 2023 Entropy 25 1571
Google Scholar
[47] Pradhan G S, Sahu D, Rath R, Sahoo R, Cleymans J 2024 Eur. Phys. J. A 60 52
Google Scholar
[48] Wu J, Lin Y, Li Z, Luo X, Wu Y 2021 Phys. Rev. C 104 034902
Google Scholar
[49] Bernhard J E, Moreland J S, Bass S A 2019 Nat. Phys. 15 1113
Google Scholar
[50] He Y Y, Pang L G, Wang X N 2019 Phys. Rev. Lett. 122 252302
Google Scholar
[51] Heffernan M R, Gale C, Jeon S, Paquet J F 2024 Phys. Rev. C 109 065207
Google Scholar
[52] Feng Y T, Shao F L, Song J 2022 Phys. Rev. C 106 034910
Google Scholar
[53] Van Hove L 1982 Phys. Lett. B 118 138
Google Scholar
[54] Olimov K K, Liu F H, Musaev K A, Olimov A K, Tukhtaev B J, Saidkhanov N S, Yuldashev B S, Olimov K, Gulamov K G 2021 Int. J. Mod. Phys. E 30 2150029
Google Scholar
[55] Olimov K K, Lebedev I A, Tukhtaev B J, Fedosimova A I, Liu F H, Khudoyberdieva S A, Kanokova S Z 2023 Int. J. Mod. Phys. E 32 2350066
Google Scholar
[56] ALICE Publications 2018 https://cds.cern.ch/record/2636623
[57] Aamodt K, Abrahantes Quintana A, Adamova D, et al. 2011 Phys. Rev. Lett. 106 032301
Google Scholar
[58] Adam J, Adamova D, Aggarwal M M, et al. 2016 Phys. Rev. Lett. 116 222302
Google Scholar
[59] Adare A, Afanasiev S, Aidala C, et al. 2016 Phys. Rev. C 93 024901
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 1640
- PDF Downloads: 51
- Cited By: 0
