-
According to the recent experimental progress of simulating energy band topology and dynamic quantum phase transitions (DQPTs) in ultracold atomic systems, we develop a periodically driven one-dimensional (1D) Raman lattice system to simulate dynamic topological phenomena. By utilizing amplitude-periodically modulated Raman beams to couple the
$ \{^1{{\mathrm{S}}}_0, {}^3{{\mathrm{P}}}_1\} $ manifolds of alkaline-earth-like atoms$^{171}{\rm{Yb}}$ , we can realize the desired periodically driven Raman lattice. Utilizing the single-band, tight-binding Hamiltonian of the time- periodic system, we analytically determine the effective Floquet Hamiltonian and the micromotion operator. These allow us to investigate the conditions under which Floquet dynamic quantum phase transitions and dynamic skyrmion structures emerge at any driving frequency in the 1D Raman lattice. When the corresponding vector trajectory of the effective Floquet Hamiltonian has a non-zero winding number ($\nu \neq 0$ ), the system exhibits both Floquet dynamic quantum phase transitions and dynamic skyrmion structures. For$\nu =0$ , Floquet dynamic quantum phase transitions may still occur, but dynamic skyrmion structures will definitely disappear. Therefore, the topologically nontrivial nature of the effective Floquet Hamiltonian is a sufficient but not necessary condition for the onset of the Floquet dynamic quantum phase transitions. But it is a necessary and sufficient condition for the onset of the dynamical skyrmion structures.
[1] Aidelsburger M, Atala M, Lohse M, Barreiro J T, Paredes B, Bloch I 2013 Phys. Rev. Lett. 111 185301
Google Scholar
[2] Jotzu G, Messer M, Desbuquois R, Lebrat M, Uehlinger T, Greif D, Esslinger T 2014 Nature 515 237
Google Scholar
[3] Fläschner N, Rem B S, Tarnowski M, Vogel D, Lühmann D S, Sengstock K, Weitenberg C 2016 Science 352 1091
Google Scholar
[4] Wu Z, Zhang L, Sun W, Xu X T, Wang B Z, Ji S C, Deng Y, Chen S, Liu X J, Pan J W 2016 Science 354 83
Google Scholar
[5] Tai M E, Lukin A, Rispoli M, et al. 2017 Nature 546 519
Google Scholar
[6] Xie D, Deng T S, Xiao T, Gou W, Chen T, Yi W, Yan B 2020 Phys. Rev. Lett. 124 050502
Google Scholar
[7] Kitagawa T, Broome M A, Fedrizzi A, et al. 2012 Nat. Commun. 3 882
Google Scholar
[8] Rechtsman M C, Zeuner J M, Plotnik Y, et al. 2013 Nature 496 196
Google Scholar
[9] Xu X Y, Wang Q Q, Pan W W, et al. 2018 Phys. Rev. Lett. 120 260501
Google Scholar
[10] Wang B, Chen T, Zhang X 2018 Phys. Rev. Lett. 121 100501
Google Scholar
[11] Chen C, Ding X, Qin J, et al. 2018 Phys. Rev. Lett. 121 100502
Google Scholar
[12] Yao N Y, Laumann C R, Gopalakrishnan S, Knap M, Müller M, Demler E A, Lukin M D 2014 Phys. Rev. Lett. 113 243002
Google Scholar
[13] Shu Z, Liu Y, Cao Q, Yang P, Zhang S, Plenio M B, Jelezko F, Cai J 2018 Phys. Rev. Lett. 121 210501
Google Scholar
[14] Roushan P, Neill C, Megrant A, et al. 2017 Nat. Phys. 13 146
Google Scholar
[15] Yan Z, Zhang Y R, Gong M, et al. 2019 Science 364 753
Google Scholar
[16] Heyl M, Polkovnikov A, Kehrein S 2013 Phys. Rev. Lett. 110 135704
Google Scholar
[17] Budich J C, Heyl M 2016 Phys. Rev. B 93 085416
Google Scholar
[18] Huang Z, Balatsky A V 2016 Phys. Rev. Lett. 117 086802
Google Scholar
[19] Wang C, Zhang P, Chen X, Yu J, Zhai H 2017 Phys. Rev. Lett. 118 185701
Google Scholar
[20] Heyl M 2018 Rep. Prog. Phys. 81 054001
Google Scholar
[21] Fläschner N, Vogel D, Tarnowski M, et al. 2018 Nat. Phys. 14 265
Google Scholar
[22] Zhou L W, Wang Q H, Wang H L 2018 Phys. Rev. A 98 022129
Google Scholar
[23] Qiu X, Deng T S, Guo G C, Yi W 2018 Phys. Rev. A 98 021601
Google Scholar
[24] Tarnowski M, Ünal F N, Fläschner N, Rem B S, Eckardt A, Sengstock K, Weitenberg C 2019 Nat. Commun. 10 1728
Google Scholar
[25] 邓天舒, 易为 2019 68 040303
Google Scholar
Deng T S, Yi W 2019 Acta Phys. Sin. 68 040303
Google Scholar
[26] Zhang L, Zhang L, Niu S, Liu X J 2018 Science Bulletin 63 1385
Google Scholar
[27] Zhang L, Zhang L, Liu X J 2019 Phys. Rev. A 99 053606
Google Scholar
[28] Yang C, Li L, Chen S 2018 Phys. Rev. B 97 060304
Google Scholar
[29] Gong Z, Ueda M 2018 Phys. Rev. Lett. 121 250601
Google Scholar
[30] Qiu X, Deng T S, Hu Y, Xue P, Yi W 2019 iScience 20 392
Google Scholar
[31] Sun W, Yi C R, Wang B Z, et al. 2018 Phys. Rev. Lett. 121 250403
Google Scholar
[32] Defenu N, Enss T, Halimeh J C 2019 Phys. Rev. B 100 014434
Google Scholar
[33] Ünal F N, Bouhon A, Slager R J 2020 Phys. Rev. Lett. 125 053601
Google Scholar
[34] Uhrich P, Defenu N, Jafari R, Halimeh J C 2020 Phys. Rev. B 101 245148
Google Scholar
[35] Kitagawa T, Berg E, Rudner M, Demler E 2010 Phys. Rev. B 82 235114
Google Scholar
[36] Rudner M S, Lindner N H, Berg E, Levin M 2013 Phys. Rev. X 3 031005
[37] Eckardt A 2017 Rev. Mod. Phys. 89 011004
Google Scholar
[38] Wang K, Qiu X, Xiao L, Zhan X, Bina Z, Yi W, Xue P 2019 Phys. Rev. Lett. 122 020501
Google Scholar
[39] Yang K, Zhou L, Ma W, Kong X, Wang P, Qin X, Rong X, Wang Y, Shi F, Gong J, Du J 2019 Phys. Rev. B 100 085308
Google Scholar
[40] Wang K, Qiu X, Xiao L, Zhan X, Bian Z, Sanders B C, Yi W, Xue P 2019 Nat. Commun. 10 2293
Google Scholar
[41] Cheng Q, Pan Y, Wang H, Zhang C, Yu D, Gover A, Zhang H, Li T, Zhou L, Zhu S 2019 Phys. Rev. Lett. 122 173901
Google Scholar
[42] Mukherjee S, Rechtsman M C 2020 Science 368 856
Google Scholar
[43] Zamani S, Jafari R, Langari A 2020 Phys. Rev. B 102 144306
Google Scholar
[44] Jafari R, Akbari A 2021 Phys. Rev. A 103 012204
Google Scholar
[45] Cai D H, Yi W 2022 Phys. Rev. A 105 042812
Google Scholar
[46] Liu X J, Liu Z X, Cheng M 2013 Phys. Rev. Lett. 110 076401
Google Scholar
[47] Liu X J, Law K T, Ng T K 2014 Phys. Rev. Lett. 112 086401
Google Scholar
[48] Pan J S, Liu X J, Zhang W, Yi W, Guo G C 2015 Phys. Rev. Lett. 115 045303
Google Scholar
-
图 1 激光与类碱土$ ^{171} {\rm{Yb}}$原子相互耦合实现时间周期拉曼晶格的原理图. F和$ F' $分别是标记超精细基态和激发态的量子数, $ {\varDelta}_{\rm{s}} $是超精细能级间距, x轴为量子化轴
Figure 1. Schematic illustration of a time-periodic Raman lattice realized by laser coupling to alkaline-earth-like atoms $ ^{171} {\rm{Yb}}$. F and $ F' $ are the quantum numbers labeling the hyperfine ground states and excited states respectively, $ {\varDelta}_{\rm{s}} $ is the hyperfine manifolds, and the x axis is the quantization axis.
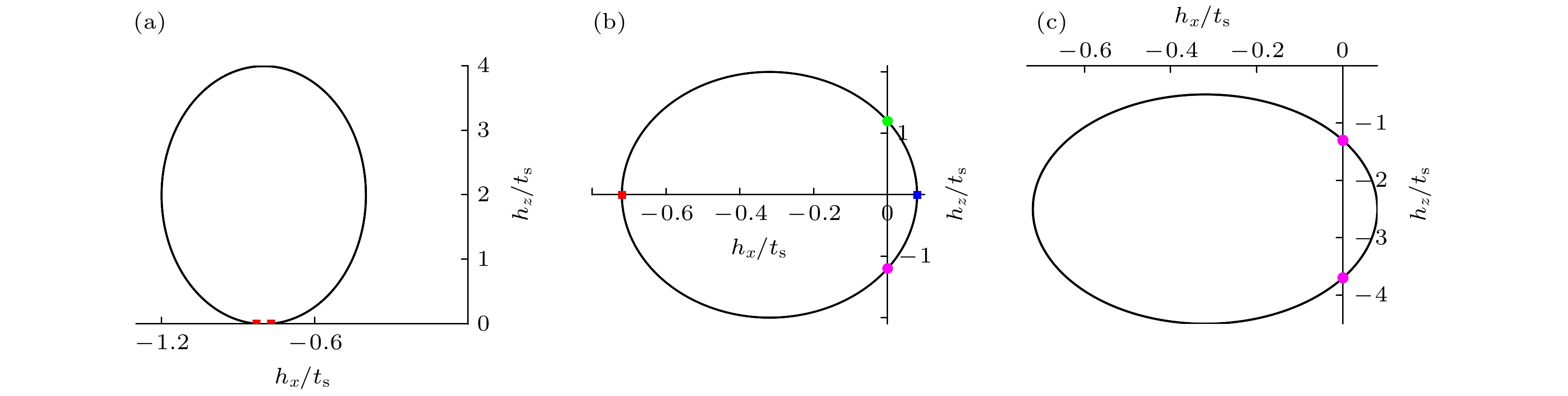
图 2 随无量纲的准动量k遍历整个布里渊区过程中矢量h形成的椭圆轨迹 (a)椭圆轨迹的绕数$ \nu = 0 $, 相应的参数为$ m_z = 2.05 t_{\rm{s}} $,$ t_{\rm{so}} = 0.2 t_{\rm{s}} $, $ t''_{\rm{so}} = 0.8 t_{\rm{s}} $, $ \omega = 0.1 t_{\rm{s}} $; (b) 椭圆轨迹的绕数$ \nu = 1 $, 相应的参数为$ m_z = 2.5 t_{\rm{s}} $, $ t_{\rm{so}} = 0.2 t_{\rm{s}} $, $ t''_{\rm{so}} = 0.32 t_{\rm{s}} $, $ \omega = 5 t_{\rm{s}} $; (c) 椭圆轨迹的绕数$ \nu = 0 $, 相应的参数为$ m_z = 0 $, $ t_{\rm{so}} = 0.2 t_{\rm{s}} $, $ t''_{\rm{so}} = 0.32 t_{\rm{s}} $, $ \omega = 5 t_{\rm{s}} $. 品红色和绿色的圆点对应于两种不同类型的不动点, 红色和蓝色的方点对应于两种不同类型的临界动量$ k_{\mathrm{c}} $
Figure 2. The elliptic trajectory formed by h as the dimensionless quasimomentum k traverses the Brillouin zone: (a) Trajectory for $ \nu = 0 $, with $ m_z = 2.05 t_{\rm{s}} $, $ t_{\rm{so}} = 0.2 t_{\rm{s}} $, $ t''_{\rm{so}} = 0.8 t_{\rm{s}} $, $ \omega = 0.1 t_{\rm{s}} $; (b) trajectory for $ \nu = 1 $, with $ m_z = 2.5 t_{\rm{s}} $, $ t_{\rm{so}} = 0.2 t_{\rm{s}} $, $ t''_{\rm{so}} = $$ 0.32 t_{\rm{s}} $, $ \omega = 5 t_{\rm{s}} $; (c) trajectory for $ \nu = 0 $, with $ m_z = 0 $, $ t_{\rm{so}} = 0.2 t_{\rm{s}} $, $ t''_{\rm{so}} = 0.32 t_{\rm{s}} $, $ \omega = 5 t_{\rm{s}} $. The magenta and green dots correspond to two different types of fixed points, and the red and blue square dots correspond to critical quasimomenta $ k_{\mathrm{c}} $.
图 3 单位矢量$ {\boldsymbol{n}}_{h}(k, t) $在Bloch球上的动力学演化 (a)任意k指标下$ {\boldsymbol{n}}_{h}(k, t) $绕z轴进动, $ k_m $指标下矢量$ {\boldsymbol{n}}_{h}(k, t) $指向Bloch球的北极(球上绿点)或者南极(球上品红色点), 这些点对应于图2中椭圆轨迹与z轴交点; (b)在临界动量$ k_{\mathrm{c}} $指标下矢量$ {\boldsymbol{n}}_{h}(k, t) $落在赤道面上, Floquet动力学经历半个周期以后单位矢量运动至相反方向, 此时的量子态与初态正交. 这些$ k_{\mathrm{c}} $点对应于图2中椭圆轨迹与x轴的交点
Figure 3. Dynamical evolution of the unit vector $ {\boldsymbol{n}}_{h}(k, t) $ on the Bloch sphere: (a) $ {\boldsymbol{n}}_{h}(k, t) $ rotates around the z-axis at any k-index. $ {\boldsymbol{n}}_{h}(k, t) $ at the $ k_m $-index points to the north pole (the green point) or the south pole (the magenta point) of the Bloch sphere, which corresponds to the intersections of elliptical trajectories with the z-axis in Fig. 2; (b) $ {\boldsymbol{n}}_{h}(k, t) $ lies on the equator at the critical momentum $ k_{\mathrm{c}} $-index, and the Floquet dynamics evolves half a cycle after which the unit vector moves to the opposite direction, at which point the quantum state is orthogonal to the initial state. These $ k_{\mathrm{c}} $-index correspond to the intersections of the elliptical trajectories with the x axis in Fig. 2.
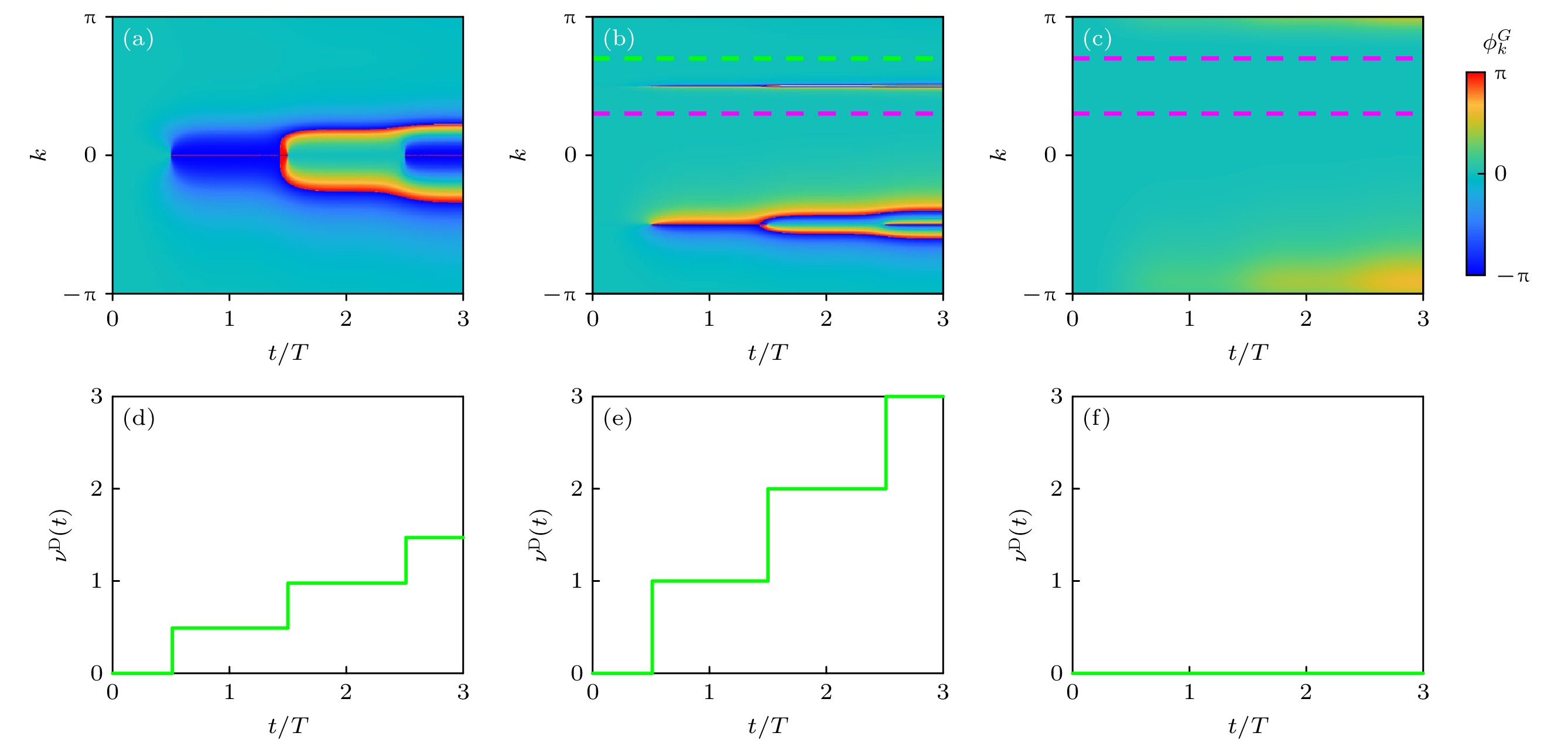
图 4 Loschmidt回波$ |G^{-}_{k}(t)|^2 $和相应的速率函数$ g_-(t) $ (a)—(c) $ |G^{-}_{k}(t)|^2 $随时间$ t $和无量纲准动量k变化的密度图, 这里的数值计算分别采用与图2(a)—(c)中相同的参数, 相应的速率函数如图(d)—(f)所示; 图(a)和(b)中出现了Floquet动力学量子相变, 每个周期内存在两个临界动量, 相应的速率函数在临界时间处出现非解析性. 图中品红色和绿色水平虚线分别表示两种不同类型的不动点出现的位置
Figure 4. Loschmidt eco $ |G^{-}_{k}(t)|^2 $ and the corresponding rate functions $ g_-(t) $: (a)–(c) The density plot of $ |G^{-}_{k}(t)|^2 $ versus $ t $ and k, where the numerical calculations are performed using the same parameters as in Figs. 2(a)-(c), respectively. The corresponding rate functions are shown in (d)–(f); Floquet dynamic quantum pahse transitions occur in (a), (b), where two critical quasimomentas are identified in each period, and the corresponding rate functions appear nonanalytic at the critical time. The magenta and green dashed horizontal lines indicate the locations where two different types of fixed points appear, respectively.
图 5 Pancharatnam几何相位和动力学拓扑序参量 (a)—(c) Pancharatnam几何相位随时间$ t $和无量纲的准动量k变化的密度图, 这里的数值计算分别采用与图2(a)—(c)中相同的参数, 相应的动力学拓扑序参量分别如图(d)—(f)所示. 图中品红色和绿色虚线分别表示两种不同类型的不动点出现的位置
Figure 5. Pancharatnam geometric phases and dynamic topological order parameter: (a)–(c) The density plot of the Pancharatnam geometric phases with time t and k, where the numerical calculations are performed using the same parameters as in Figs. 2(a)-(c), respectively. The corresponding dynamic topological order parameter are shown in (d)–(f). The magenta and green dashed horizontal lines indicate the locations where two different types of fixed points appear, respectively.
图 6 动量时间域k-t上的自旋结构$ {\boldsymbol{n}}_h(k, t) $ (a)存在动力学量子相变但不存在不动点; (b)系统出现斯格明子结构说明存在分立化的动力学陈数, 品红色和绿色虚线分别表示两种不同类型的不动点; (c)两条品红色虚线表示两个同种类型的不动点, 此时不存在动力学量子相变; 图(a)和(b)中红色方点表示动力学量子相变出现的位置
Figure 6. Spin texture $ {\boldsymbol{n}}_h(k, t)$ in the k-t domain: (a) Floquet dynamic quantum phase transitions exist but there are not fixed points; (b) the appearance of skyrmion substructure indicates the existence of quantized Chern number; the magenta and green dashed horizontal lines indicate the locations where two different types of fixed points appear, respectively; (c) two magenta dashed horizontal lines represent two fixed points of the same type, where there are no Floquet dynamic quantum phase transitions; the red square dots in (a) and (b) indicate the location where dynamic quantum phase transitions occur.
-
[1] Aidelsburger M, Atala M, Lohse M, Barreiro J T, Paredes B, Bloch I 2013 Phys. Rev. Lett. 111 185301
Google Scholar
[2] Jotzu G, Messer M, Desbuquois R, Lebrat M, Uehlinger T, Greif D, Esslinger T 2014 Nature 515 237
Google Scholar
[3] Fläschner N, Rem B S, Tarnowski M, Vogel D, Lühmann D S, Sengstock K, Weitenberg C 2016 Science 352 1091
Google Scholar
[4] Wu Z, Zhang L, Sun W, Xu X T, Wang B Z, Ji S C, Deng Y, Chen S, Liu X J, Pan J W 2016 Science 354 83
Google Scholar
[5] Tai M E, Lukin A, Rispoli M, et al. 2017 Nature 546 519
Google Scholar
[6] Xie D, Deng T S, Xiao T, Gou W, Chen T, Yi W, Yan B 2020 Phys. Rev. Lett. 124 050502
Google Scholar
[7] Kitagawa T, Broome M A, Fedrizzi A, et al. 2012 Nat. Commun. 3 882
Google Scholar
[8] Rechtsman M C, Zeuner J M, Plotnik Y, et al. 2013 Nature 496 196
Google Scholar
[9] Xu X Y, Wang Q Q, Pan W W, et al. 2018 Phys. Rev. Lett. 120 260501
Google Scholar
[10] Wang B, Chen T, Zhang X 2018 Phys. Rev. Lett. 121 100501
Google Scholar
[11] Chen C, Ding X, Qin J, et al. 2018 Phys. Rev. Lett. 121 100502
Google Scholar
[12] Yao N Y, Laumann C R, Gopalakrishnan S, Knap M, Müller M, Demler E A, Lukin M D 2014 Phys. Rev. Lett. 113 243002
Google Scholar
[13] Shu Z, Liu Y, Cao Q, Yang P, Zhang S, Plenio M B, Jelezko F, Cai J 2018 Phys. Rev. Lett. 121 210501
Google Scholar
[14] Roushan P, Neill C, Megrant A, et al. 2017 Nat. Phys. 13 146
Google Scholar
[15] Yan Z, Zhang Y R, Gong M, et al. 2019 Science 364 753
Google Scholar
[16] Heyl M, Polkovnikov A, Kehrein S 2013 Phys. Rev. Lett. 110 135704
Google Scholar
[17] Budich J C, Heyl M 2016 Phys. Rev. B 93 085416
Google Scholar
[18] Huang Z, Balatsky A V 2016 Phys. Rev. Lett. 117 086802
Google Scholar
[19] Wang C, Zhang P, Chen X, Yu J, Zhai H 2017 Phys. Rev. Lett. 118 185701
Google Scholar
[20] Heyl M 2018 Rep. Prog. Phys. 81 054001
Google Scholar
[21] Fläschner N, Vogel D, Tarnowski M, et al. 2018 Nat. Phys. 14 265
Google Scholar
[22] Zhou L W, Wang Q H, Wang H L 2018 Phys. Rev. A 98 022129
Google Scholar
[23] Qiu X, Deng T S, Guo G C, Yi W 2018 Phys. Rev. A 98 021601
Google Scholar
[24] Tarnowski M, Ünal F N, Fläschner N, Rem B S, Eckardt A, Sengstock K, Weitenberg C 2019 Nat. Commun. 10 1728
Google Scholar
[25] 邓天舒, 易为 2019 68 040303
Google Scholar
Deng T S, Yi W 2019 Acta Phys. Sin. 68 040303
Google Scholar
[26] Zhang L, Zhang L, Niu S, Liu X J 2018 Science Bulletin 63 1385
Google Scholar
[27] Zhang L, Zhang L, Liu X J 2019 Phys. Rev. A 99 053606
Google Scholar
[28] Yang C, Li L, Chen S 2018 Phys. Rev. B 97 060304
Google Scholar
[29] Gong Z, Ueda M 2018 Phys. Rev. Lett. 121 250601
Google Scholar
[30] Qiu X, Deng T S, Hu Y, Xue P, Yi W 2019 iScience 20 392
Google Scholar
[31] Sun W, Yi C R, Wang B Z, et al. 2018 Phys. Rev. Lett. 121 250403
Google Scholar
[32] Defenu N, Enss T, Halimeh J C 2019 Phys. Rev. B 100 014434
Google Scholar
[33] Ünal F N, Bouhon A, Slager R J 2020 Phys. Rev. Lett. 125 053601
Google Scholar
[34] Uhrich P, Defenu N, Jafari R, Halimeh J C 2020 Phys. Rev. B 101 245148
Google Scholar
[35] Kitagawa T, Berg E, Rudner M, Demler E 2010 Phys. Rev. B 82 235114
Google Scholar
[36] Rudner M S, Lindner N H, Berg E, Levin M 2013 Phys. Rev. X 3 031005
[37] Eckardt A 2017 Rev. Mod. Phys. 89 011004
Google Scholar
[38] Wang K, Qiu X, Xiao L, Zhan X, Bina Z, Yi W, Xue P 2019 Phys. Rev. Lett. 122 020501
Google Scholar
[39] Yang K, Zhou L, Ma W, Kong X, Wang P, Qin X, Rong X, Wang Y, Shi F, Gong J, Du J 2019 Phys. Rev. B 100 085308
Google Scholar
[40] Wang K, Qiu X, Xiao L, Zhan X, Bian Z, Sanders B C, Yi W, Xue P 2019 Nat. Commun. 10 2293
Google Scholar
[41] Cheng Q, Pan Y, Wang H, Zhang C, Yu D, Gover A, Zhang H, Li T, Zhou L, Zhu S 2019 Phys. Rev. Lett. 122 173901
Google Scholar
[42] Mukherjee S, Rechtsman M C 2020 Science 368 856
Google Scholar
[43] Zamani S, Jafari R, Langari A 2020 Phys. Rev. B 102 144306
Google Scholar
[44] Jafari R, Akbari A 2021 Phys. Rev. A 103 012204
Google Scholar
[45] Cai D H, Yi W 2022 Phys. Rev. A 105 042812
Google Scholar
[46] Liu X J, Liu Z X, Cheng M 2013 Phys. Rev. Lett. 110 076401
Google Scholar
[47] Liu X J, Law K T, Ng T K 2014 Phys. Rev. Lett. 112 086401
Google Scholar
[48] Pan J S, Liu X J, Zhang W, Yi W, Guo G C 2015 Phys. Rev. Lett. 115 045303
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 1359
- PDF Downloads: 62
- Cited By: 0
