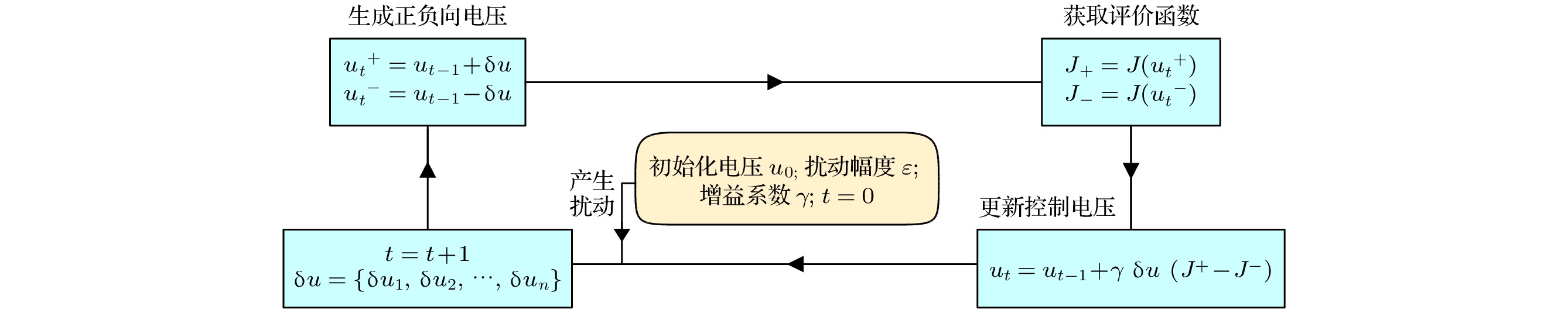
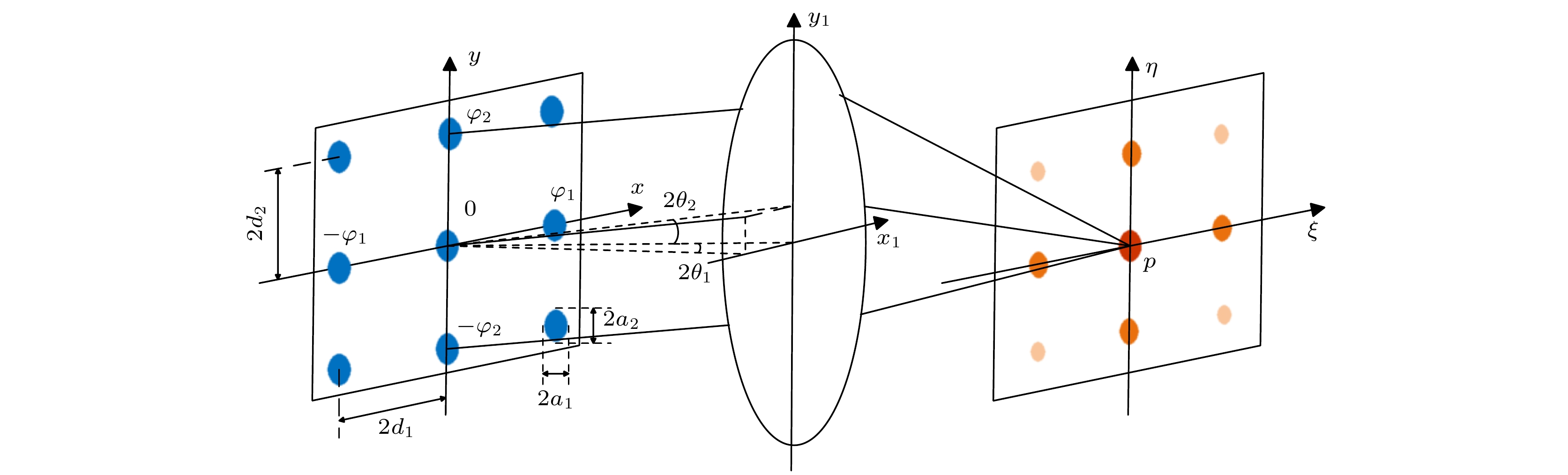
-
Optical phased array (OPA) technology, as a pivotal component of laser detection and ranging (LiDAR) systems, plays a crucial role in augmenting the application efficiency in fields such as autonomous driving, precision measurement, and remote sensing detection. With the escalating demands for high-resolution imaging, the array size of OPAs is continuously expanding, imposing higher requirements on the calibration precision and efficiency of the output beam. Existing calibration algorithms, such as the simultaneous perturbation stochastic gradient descent (SPGD) and the Gerchberg-Saxton (GS) algorithm, often face challenges of prolonging calibration times and insufficient precision when dealing with large-scale OPA systems. In order to address this problem, our study introduces the Adam optimization algorithm, renowned for its adaptive learning rate feature, into the calibration process of OPA output beams. Through simulation modeling and experimental validation, this work comprehensively examines the differences in performance between the Adam algorithm and conventional SPGD and GS algorithms in beam calibration, especially under various OPA array configurations. For a 16×16 OPA array, the application of the Adam algorithm significantly enhances the peak side lobe ratio (PSLR) to over 15.98 dB, while notably reducing the number of iterations to less than 600, thereby shortening the calibration cycle and improving calibration precision effectively. Furthermore, this work provides an in-depth analysis of parameter selection, convergence speed, and stability of the Adam algorithm in OPA calibration, offering detailed guidance for achieving more efficient and high-quality beam calibration. Through comparative analysis, this work not only demonstrates the substantial advantages of the Adam algorithm in enhancing OPA calibration efficiency, reducing calibration duration, and optimizing output beam quality but also emphasizes its critical role in advancing OPA technology. The main contribution of this work lies in providing an innovative algorithmic approach for achieving efficient calibration of OPA output beams, which has important theoretical and practical significance for advancing the LiDAR technology, particularly in the field of high-precision beam control. Moreover, by applying optimized algorithms, this study not only improves the performance of OPA technology within existing domains but also paves new ways for its application in emerging fields such as optical communication, optical networking, and high-resolution imaging. 
-
Keywords:
- optical phased array /
- calibration algorithm /
- Adam algorithm
[1] Barker S, Rebeiz G M 1998 IEEE T. Microw. Theory 46 1881
Google Scholar
[2] Duewer B E, Wilson J M, Winick D A, Franzon P D 1999 Proceedings of SPIE-The International Society for Optical Engineering Gold Coast, Australia, October 8, 1999 p262
[3] Hobbs R H, Cantor A J, Grantham D H, Shuskus A J, Berak J M, Cowher M E, Farina J D, Hoffman N N, Black J F, Drake G W, Brown R T, Holton C E, Silverman B B, Leonberger F J, Demaria A J 2002 Conference Proceedings LEOS Lasers and Electro-Optics Society Santa Clara, CA, USA, February 4, 1988 p94
[4] Konforti N, Marom E, Wu S T 1988 Opt. Lett. 13 251
Google Scholar
[5] Malczewski A, Eshelman S, Pillans B, Ehmke J, Goldsmith C 1999 IEEE Microw. Guided W. 9 517
Google Scholar
[6] Pillans B, Eshelman S, Malczewski A, Ehmke J, Goldsmith C 2002 Radio Frequency Integrated Circuits (RFIC) Symposium: Digest of Papers Boston, MA, USA, June 11–13, 2000 p195
[7] Meyer R A 1972 Appl. Opt. 11 613
Google Scholar
[8] Sun J, Hosseini E S, Yaacobi A, Cole D B, Leake G, Coolbaugh D, Watts M R 2014 Opt. Lett. 39 367
Google Scholar
[9] Sun J, Timurdogan E, Yaacobi A, Hosseini E S, Watts M R 2013 Nature 493 195
Google Scholar
[10] Sun J, Timurdogan E, Yaacobi A, Su Z, Hosseini E S, Cole D B, Watts M R 2013 IEEE J. Sel. Top. Quant. 20 264
Google Scholar
[11] Yaacobi A, Sun J, Moresco M, Leake G, Coolbaugh D, Watts M R 2014 Opt. Lett. 39 4575
Google Scholar
[12] Mahon R, Preussner M W, Rabinovich W S, Goetz P G, Kozak D A, Ferraro M S, Murphy J L 2016 Free-Space Laser Communication and Atmospheric Propagation XXVIII San Francisco, California, USA, March 15, 2016 p224
[13] Kim S H, You J B, Ha Y G, Kang G, Lee D S, Yoon H, Yoo D E, Lee D W, Yu K, Youn C H, Park H H 2019 Opt. Lett. 44 411
Google Scholar
[14] 丁亚军, 钱盛友, 胡继文, 邹孝 2012 61 144301
Google Scholar
Ding Y J, Qian Y S, Hu J W, Zou X 2012 Acta Phys. Sin. 61 144301
Google Scholar
[15] 李明飞, 袁梓豪, 刘院省, 邓意成, 王学锋 2021 70 084205
Google Scholar
Li M F, Yuan Z H, Liu Y X, Deng Y C, Wang X F 2021 Acta Phys. Sin. 70 084205
Google Scholar
[16] Zhang H Y, Zhang Z X, Peng C, Hu W W 2020 IEEE Photonics J. 12 6600210
Google Scholar
[17] Leng L M, Zeng Z B, Wu G H, Lin Z Z, Ji X, Shi Z Y, Jiang W 2022 Photonics Res. 10 347
Google Scholar
[18] Notaros J, Poulton C V, Raval M, Watts M R 2018 J. Lightwave Technol. 36 5912
Google Scholar
[19] Raptakis A, Gounaridis L, Weigel M, Kleinert M, Georgiopoulos M, Mylonas E, Groumas P, Tsokos C, Keil N, Avramopoulos H 2021 J. Lightwave Technol. 39 6509
Google Scholar
[20] Tyler N A, Fowler D, Malhouitre S, Garcia S, Grosse P, Rabaud W, Szelag B 2019 Opt. Express 27 5851
Google Scholar
[21] Wang P F, Luo G Z, Xu Y, Li Y J, Su Y M, Ma J B, Wang R T, Yang Z X, Zhou X L, Zhang Y J, Pan J Q 2020 Photonics Res. 8 912
Google Scholar
[22] Zhou P, Liu Z, Wang X, Ma Y, Xu X 2009 Acta Opt. Sin. 29 2232
[23] Gerchberg R W 1972 Optik 35 237
[24] Chen C C, Miao J, Wang C, Lee T 2007 Phys. Rev. B 76 064113
Google Scholar
[25] Kingma D P, Ba J 2014 arXiv: 1412.6980 [cs.LG]
[26] Reyad M, Sarhan A M, Arafa M 2023 Neural Comput. Appl. 35 17095
Google Scholar
[27] 石顺祥, 张海兴, 刘劲松 2000 物理光学与应用光学(第三版) (西安: 西安电子科技大学出版社) 第139—149页
Shi S X, Zhang H X, Liu J S 2000 Physical Optics and Applied Optics (3rd Ed.) (Xi’an: Xidian University Press) pp139–149
[28] Wang Z H, Wu B B, Liao J L, Li X F, Wang C, Sun Y L, Jin L, Feng J B, Cao C Q 2023 Opt. Laser Technol. 157 108743
Google Scholar
-
图 1 OPA夫琅禾费衍射示意图
Figure 1. Schematic diagram of Fraunhofer diffraction in OPA.
图 2 OPA输出光束校准的Adam算法流程图
Figure 2. Flowchart of the Adam algorithm for calibrating the OPA output beam.
图 3 OPA输出光束校准的SPGD算法流程图
Figure 3. Flowchart of the SPGD algorithm for calibrating the OPA output beam.
图 4 使用Adam算法对不同阵列规模OPA输出光束校准结果 (a) 4×4阵列校准结果; (b) 8×8阵列校准结果; (c) 16×16阵列校准结果
Figure 4. Different adjusting results of output beam in serial OPA with Adam algorithm: (a) Adjusting results of 4×4 array; (b) adjusting results of 8×8 array; (c) adjusting results of 16×16 array.
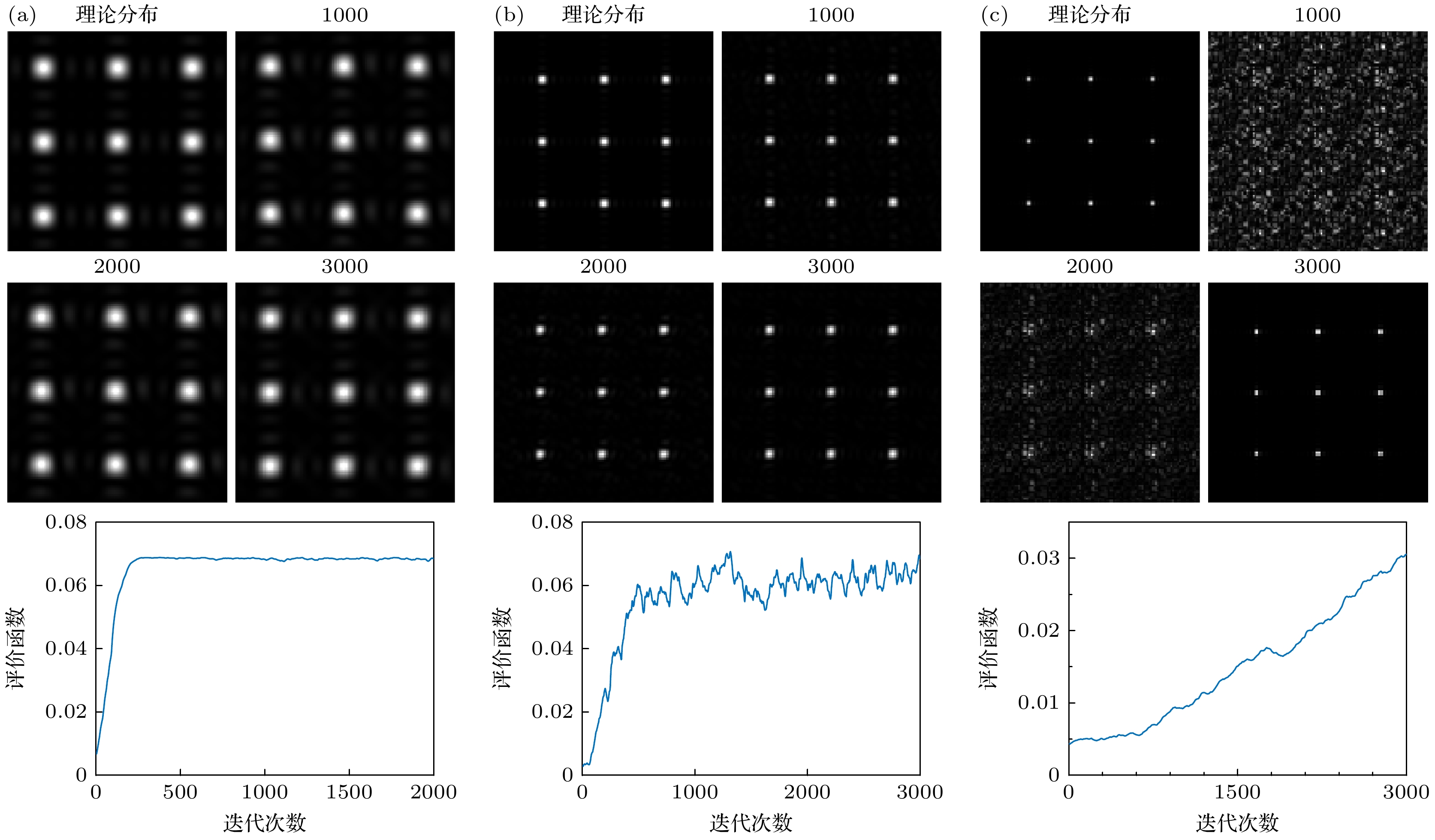
图 5 使用SPGD, GS, Adam算法对4×4规模OPA输出光束校准结果 (a)不同算法优化仿真结果; (b) 优化迭代1000次不同算法评价函数曲线图汇总; (c) 优化不限次数不同算法评价函数曲线图汇总
Figure 5. Different adjusting results of output beam with SPGD, GS, Adam algorithm in 4×4 OPA: (a) Simulation results with different algorithms; (b) collection of curve graphs of evaluation function when iterating 1000 times with different algorithms; (c) collection of curve graphs of evaluation function when iterating unlimited times with different algorithms.
图 6 实验系统流程图
Figure 6. Flow diagram of experiment system.
图 7 仿真理论分布、Adam算法及SPGD算法输出光场三维图
Figure 7. 3D diagram of output light field with simulation model, Adam algorithm and SPGD algorithm.
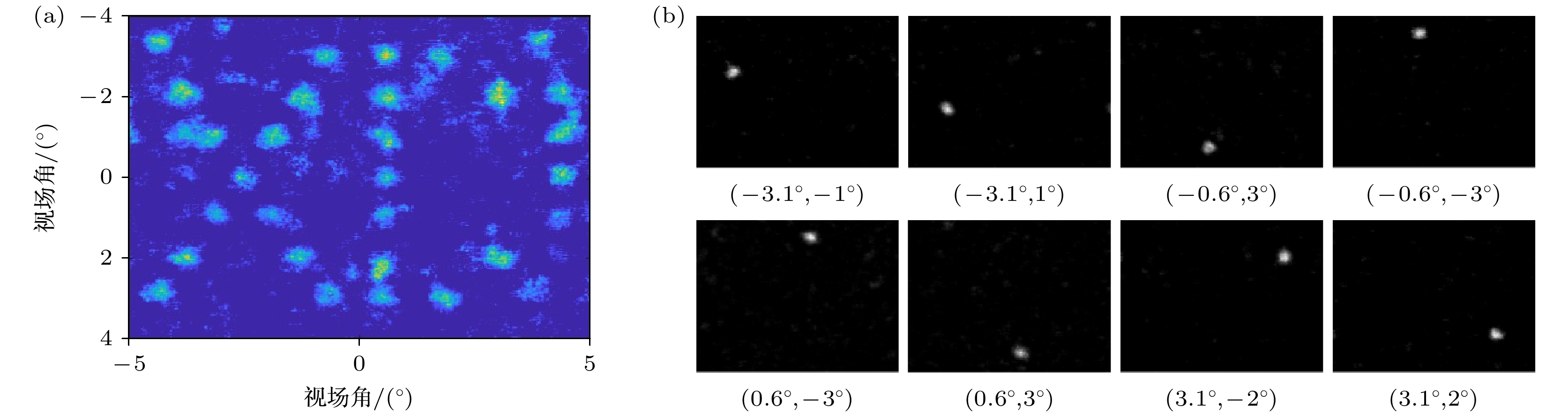
图 8 SPGD算法及Adam算法光束校准效果图 (a)不同迭代次数下Adam算法光场灰度图; (b) 不同迭代次数下SPGD算法光场灰度图; (c) Adam及SPGD算法评价函数曲线图
Figure 8. Adjusting results of beam with SPGD and Adam algorithm: (a) Grey-scale map of different iterative times with Adam algorithm; (b) grey-scale map of different iterative times with SPGD algorithm; (c) curve graph of evaluation function with Adam and SPGD algorithm.
图 9 (a)优化光场叠加图; (b)不同位置优化光场
Figure 9. (a) Superposed figure of optimized light field; (b) optimized light field in different positions.
-
[1] Barker S, Rebeiz G M 1998 IEEE T. Microw. Theory 46 1881
Google Scholar
[2] Duewer B E, Wilson J M, Winick D A, Franzon P D 1999 Proceedings of SPIE-The International Society for Optical Engineering Gold Coast, Australia, October 8, 1999 p262
[3] Hobbs R H, Cantor A J, Grantham D H, Shuskus A J, Berak J M, Cowher M E, Farina J D, Hoffman N N, Black J F, Drake G W, Brown R T, Holton C E, Silverman B B, Leonberger F J, Demaria A J 2002 Conference Proceedings LEOS Lasers and Electro-Optics Society Santa Clara, CA, USA, February 4, 1988 p94
[4] Konforti N, Marom E, Wu S T 1988 Opt. Lett. 13 251
Google Scholar
[5] Malczewski A, Eshelman S, Pillans B, Ehmke J, Goldsmith C 1999 IEEE Microw. Guided W. 9 517
Google Scholar
[6] Pillans B, Eshelman S, Malczewski A, Ehmke J, Goldsmith C 2002 Radio Frequency Integrated Circuits (RFIC) Symposium: Digest of Papers Boston, MA, USA, June 11–13, 2000 p195
[7] Meyer R A 1972 Appl. Opt. 11 613
Google Scholar
[8] Sun J, Hosseini E S, Yaacobi A, Cole D B, Leake G, Coolbaugh D, Watts M R 2014 Opt. Lett. 39 367
Google Scholar
[9] Sun J, Timurdogan E, Yaacobi A, Hosseini E S, Watts M R 2013 Nature 493 195
Google Scholar
[10] Sun J, Timurdogan E, Yaacobi A, Su Z, Hosseini E S, Cole D B, Watts M R 2013 IEEE J. Sel. Top. Quant. 20 264
Google Scholar
[11] Yaacobi A, Sun J, Moresco M, Leake G, Coolbaugh D, Watts M R 2014 Opt. Lett. 39 4575
Google Scholar
[12] Mahon R, Preussner M W, Rabinovich W S, Goetz P G, Kozak D A, Ferraro M S, Murphy J L 2016 Free-Space Laser Communication and Atmospheric Propagation XXVIII San Francisco, California, USA, March 15, 2016 p224
[13] Kim S H, You J B, Ha Y G, Kang G, Lee D S, Yoon H, Yoo D E, Lee D W, Yu K, Youn C H, Park H H 2019 Opt. Lett. 44 411
Google Scholar
[14] 丁亚军, 钱盛友, 胡继文, 邹孝 2012 61 144301
Google Scholar
Ding Y J, Qian Y S, Hu J W, Zou X 2012 Acta Phys. Sin. 61 144301
Google Scholar
[15] 李明飞, 袁梓豪, 刘院省, 邓意成, 王学锋 2021 70 084205
Google Scholar
Li M F, Yuan Z H, Liu Y X, Deng Y C, Wang X F 2021 Acta Phys. Sin. 70 084205
Google Scholar
[16] Zhang H Y, Zhang Z X, Peng C, Hu W W 2020 IEEE Photonics J. 12 6600210
Google Scholar
[17] Leng L M, Zeng Z B, Wu G H, Lin Z Z, Ji X, Shi Z Y, Jiang W 2022 Photonics Res. 10 347
Google Scholar
[18] Notaros J, Poulton C V, Raval M, Watts M R 2018 J. Lightwave Technol. 36 5912
Google Scholar
[19] Raptakis A, Gounaridis L, Weigel M, Kleinert M, Georgiopoulos M, Mylonas E, Groumas P, Tsokos C, Keil N, Avramopoulos H 2021 J. Lightwave Technol. 39 6509
Google Scholar
[20] Tyler N A, Fowler D, Malhouitre S, Garcia S, Grosse P, Rabaud W, Szelag B 2019 Opt. Express 27 5851
Google Scholar
[21] Wang P F, Luo G Z, Xu Y, Li Y J, Su Y M, Ma J B, Wang R T, Yang Z X, Zhou X L, Zhang Y J, Pan J Q 2020 Photonics Res. 8 912
Google Scholar
[22] Zhou P, Liu Z, Wang X, Ma Y, Xu X 2009 Acta Opt. Sin. 29 2232
[23] Gerchberg R W 1972 Optik 35 237
[24] Chen C C, Miao J, Wang C, Lee T 2007 Phys. Rev. B 76 064113
Google Scholar
[25] Kingma D P, Ba J 2014 arXiv: 1412.6980 [cs.LG]
[26] Reyad M, Sarhan A M, Arafa M 2023 Neural Comput. Appl. 35 17095
Google Scholar
[27] 石顺祥, 张海兴, 刘劲松 2000 物理光学与应用光学(第三版) (西安: 西安电子科技大学出版社) 第139—149页
Shi S X, Zhang H X, Liu J S 2000 Physical Optics and Applied Optics (3rd Ed.) (Xi’an: Xidian University Press) pp139–149
[28] Wang Z H, Wu B B, Liao J L, Li X F, Wang C, Sun Y L, Jin L, Feng J B, Cao C Q 2023 Opt. Laser Technol. 157 108743
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 1668
- PDF Downloads: 46
- Cited By: 0
