Theoretical investigation on extreme ultraviolet radiative opacity and emissivity of Sn plasmas at local-thermodynamic equilibrium

Gao Cheng; Liu Yan-Peng; Yan Guan-Peng; Yan Jie; Chen Xiao-Qi; Hou Yong; Jin Feng-Tao; Wu Jian-Hua; Zeng Jiao-Long; Yuan Jian-Min

doi:10.7498/aps.72.20230455

Abstract

Sn is the material for an extreme ultraviolet (EUV) light source working at 13.5 nm, therefore the radiative properties of Sn plasma are of great importance in designing light source. The radiative opacity and emissivity of Sn plasma at local thermodynamic equilibrium are investigated by using a detailed-level-accounting model. In order to obtain precise atomic data, a multi-configuration Dirac-Fock method is used to calculate energy levels and oscillator strengths of ${\rm{Sn}}^{6+}$-${\rm{Sn}}^{14+}$. The electronic correlation effects of $4{\rm d}^m\text{-}4{\rm f}^m$($m=1, 2, 3, 4$) and $ 4\mathrm{p}^n\text{-}4\mathrm{d}^n $($n=1, 2, 3$) are mainly considered, which dominate the radiation near 13.5 nm. The number of fine-structure levels reaches about 200000 for each ionization stage in the present large-scale configuration interaction calculations. For the large oscillator strengths (> 0.01), the length form is in accord with the velocity form and their relative difference is about 20%–30%. The calculated transmission spectra of Sn plasma at 30 eV and 0.01 g/cm³ are compared with the experimental result, respectively, showing that they have both good consistency. The radiative opacity and emissivity of Sn plasma at the temperature in a range of 16–30 eV and density in a scope of of 0.0001–0.1 g/cm³ are investigated systematically. The effects of the plasma temperature and plasma density on radiation characteristics are studied. The results show that the radiative properties near 13.5 nm are broadened with the increase of density at a specific temperature, while it is narrowed with the increase of temperature for a specific density. The present investigation should be helpful in designing and studying EUV light source in the future.

Keywords:

Authors and contacts

1.
College of Science, National University of Defense Technology, Changsha 410073, China
2.
State Key Laboratory of Laser Interaction with Matter, Northwest Institute of Nuclear Technology, Xi’an 710000, China
3.
College of Science, Zhejiang University of Technology, Hangzhou 310023, China
4.
Graduate School of China Academy of Engineering Physics, Beijing 100193, China

Corresponding author: Gao Cheng, gaocheng@nudt.edu.cn ; Wu Jian-Hua, wujh@nudt.edu.cn ;

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12074430, 11974423) and the Foundation Research of the State Key Laboratory of Laser Interaction with Matter, China (Grant No. SKLIM2008).

FullText HTML : translate this paragraph

References

[1]	Pirati A, Van Shoot J, Troost K, van Ballegoij R, Krabbendam P, Stoeldraijer J, Loopstra E, Benschop J, Finders J, Meiling H, van Setten E, Mika N, Driedonkx J, Stamm U 2017 Proc. SPIE 10143 101430G Google Scholar
[2]	Hutcheson G D 2018 Proc. SPIE 10583 1058303
[3]	David A 2007 Soft x-rays and Extreme Ultraviolet Radiation: Principles and Applications (Cambridge: Cambridge University Press) pp17–20
[4]	宗楠, 胡蔚敏, 王志敏, 王小军, 张申金, 薄勇, 彭钦军, 许祖彦 2020 中国光学 13 28 Google Scholar Zong N, Hu W M, Wang Z M, Wang X J, Zhang S J, Bo Y, Peng Q J, Xu Z Y 2020 Chin. Opt. 13 28 Google Scholar
[5]	Versolato O O 2019 Plasma Sources Sci. Technol. 28 083001 Google Scholar
[6]	Kieft E R, Garloff K, van der Mullen J J A M, Banine V 2005 Phys. Rev. E 71 036402 Google Scholar
[7]	Ueno Y, Soumagne G, Sumitani A, Endo A, Higashiguchi T 2007 Appl. Phys. Lett. 91 231501 Google Scholar
[8]	Svendsen W, O’Sullivan G 1994 Phys. Rev. A 50 3710 Google Scholar
[9]	Churilov S S, Ryabtsev A N 2006 Opt. Spectrosc. 101 169 Google Scholar
[10]	D'Arcy R, Ohashi H, Suda S, Tanuma H, Fujioka S, Nishimura H, Nishihara K, Suzuki C, Kato T, Koike F, White J, O’Sullivan G 2009 Phys. Rev. A 79 042509 Google Scholar
[11]	Ohashi H, Suda S, Tanuma H, Fujioka S, Nishimura H, Sasaki A, Nishihara K 2010 J. Phys. B: At. Mol. Opt. Phys. 43 065204 Google Scholar
[12]	O'Sullivan G, Li B, D’Arcy R, Dunne P, Hayden P, Kilbane D, McCormack T, Ohashi H, O'Reilly F, Sheridan P, Sokell E, Suzuki C, Higashiguchi T 2015 J. Phys. B: At. Mol. Opt. Phys. 48 144025 Google Scholar
[13]	Windberger A, Torretti F, Borschevsky A, Ryabtsev A, Dobrodey S, Bekker H, Eliav E, Kaldor U, Ubachs W, Hoekstra R, Crespo López-Urrutia J R, Versolato O O 2016 Phys. Rev. A 94 012506 Google Scholar
[14]	Torretti F, Windberger A, Ryabtsev A, Dobrodey S, Bekker H, Ubachs W, Hoekstra R, Kahl E V, Berengut J C, Crespo López-Urrutia J R, Versolato O O 2017 Phys. Rev. A 95 042503 Google Scholar
[15]	Torretti F, Schupp R, Kurilovich D, Bayerle A, Scheers J, Ubachs W, Hoekstra R, Versolato O O 2018 J. Phys. B: At. Mol. Opt. Phys. 51 045005 Google Scholar
[16]	de Gaufridy de Dortan F 2007 J. Phys. B: At. Mol. Opt. Phys. 40 599 Google Scholar
[17]	Koike F, Fritzsche S 2007 Radiat. Phys. Chem. 76 404 Google Scholar
[18]	Kagawa T, Tanuma H, Ohashi H, Nishihara K 2007 J. Phys.: Conf. Ser. 58 149 Google Scholar
[19]	Eliav E, Kaldor U, Ishikawa Y 1994 Phys. Rev. A 49 1724 Google Scholar
[20]	Berengut J C, Flambaum V V, Kozlov M G 2006 Phys. Rev. A 73 012504 Google Scholar
[21]	Lysaght M, Kilbane D, Murphy N, Cummings A, Dunne P, O’Sullivan G 2005 Phys. Rev. A 72 014502 Google Scholar
[22]	Fujioka S, Nishimura H, Nishihara K, Sasaki A, Sunahara A, Okuno T, Ueda N, Ando T, Tao Y, Shimada Y, Hashimoto K, Yamaura M, Shigemori K, Nakai M, Nagai K, Norimatsu T, Nishikawa T, Miyanaga N, Izawa Y, Mima K 2005 Phys. Rev. Lett. 95 235004 Google Scholar
[23]	蔡懿, 王文涛, 杨明, 刘建胜, 陆培祥, 李儒新, 徐至展 2008 57 5100 Google Scholar Cai Y, Wang W T, Yang M, Liu J S, Lu P X, Li R X, Xu Z Z 2008 Acta Phys. Sin. 57 5100 Google Scholar
[24]	陈鸿, 兰慧, 陈子琪, 刘璐宁, 吴涛, 左都罗, 陆培祥, 王新兵 2015 64 075202 Google Scholar Chen H, Lan H, Chen Z Q, Liu L N, Wu T, Zuo D L, Lu P X, Wang X B 2015 Acta Phys. Sin. 64 075202 Google Scholar
[25]	Su M G, Min Q, Cao S Q, Sun D X, Hayden P, O’Sullivan G, Dong C Z 2017 Sci. Rep. 7 45212 Google Scholar
[26]	Schupp R, Torretti F, Meijer R A, Bayraktar M, Sheil J, Scheers J, Kurilovich D, Bayerle A, Schafgans A A, Purvis M, Eikema K S E, Witte S, Ubachs W, Hoekstra R, Versolato O O 2019 Appl. Phys. Lett. 115 124101 Google Scholar
[27]	Zeng J, Gao C, Yuan J 2010 Phys. Rev. E 82 026409 Google Scholar
[28]	Colgan J, Kilcrease D P, Jr. Abdallah J, Sherrill M E, Fontes C J, Hakel P, Armstrong G S J 2017 High Energy Density Phys. 23 133 Google Scholar
[29]	Torretti F, Sheil J, Schupp R, Basko M M, Bayraktar M, Meijer R A, Witte S, Ubachs W, Hoekstra R, Versolato O O, Neukirch A J, Colgan J 2020 Nat. Commun. 11 2334 Google Scholar
[30]	Sheil J, Versolato O O, Neukirch A J, Colgan J 2021 J. Phys. B: At. Mol. Opt. Phys. 54 035002 Google Scholar
[31]	Cowan R D 1981 The Theory of Atomic Structure and Spectra (Berkeley: University of California Press
[32]	Jönson P, Gaigalas G, Bieroń, Froese Fischer C, Grant I P 2013 Comput. Phys. Commun. 184 2197 Google Scholar
[33]	Zeng J L, Yuan J M 2006 Phys. Rev. E 74 025401(R Google Scholar
[34]	Gao C, Zeng J, Jin F, Yuan J 2013 High Energy Density Phys. 9 419 Google Scholar
[35]	Zeng J L, Gao C, Liu P F, Li Y J, Meng C S, Hou Y, Kang D D, Yuan J M 2022 Sci. China-Phys. Mech. Astron. 65 233011 Google Scholar
[36]	Stewart J C, Pyatt K D Jr. 1966 Astrophys. J. 144 1203 Google Scholar
[37]	李世昌 1992 高温辐射物理与量子辐射理论 (北京: 国防工业出版社) 第6页 Li S C 1992 High Temperature Radiation Physics and Quantum Radaition Theory (Beijing: National Defense Industry Press) p6
[38]	Zeng J L, Zhao G, Yuan J M 2006 J. Quant. Spectrosc. Radiat. Transfer 102 172 Google Scholar
[39]	Wu Z W, Tian Z Q, An Y H, Dong C Z 2021 Astrophys. J. 910 142 Google Scholar
[40]	Kühn S, Cheung C, Oreshkina N S, Steinbrügge, Togawa M, Bernitt S, Berger L, Buck J, Hoesch M, Seltmann J, Trinter F, Keitel C H, Kozlov M G, Porsev S G, Gu M F, Porter F S, Pfeifer T, Leutenegger M A, Harman Z, Safronova M S, López-Urrutia J R C, Shah C 2022 Phys. Rev. Lett. 129 245001 Google Scholar
[41]	Colgan J, Kilcrease D P, Magee N H, Sherrill M E, Abdallah Jr. J, Hakel P, Fontes C J, Guzik J A, Mussack K A 2016 Astrophys. J. 817 116 Google Scholar
[42]	兰慧 2016 博士学位论文 (武汉: 华中科技大学) Lan H 2016 Ph. D. Dissertation (Wuhan: Huazhong University of Science and Technology
[43]	Hansen S B, Chung H K, Fontes C J, Ralchenko Yu, Scott H A, Stambulchik E 2020 High Energy Density Phys. 35 100693 Google Scholar
[44]	Gao C, Zeng J, Li Y, Jin F, Yuan J 2013 High Energy Density Phys. 9 583 Google Scholar

Cited By

图 1 (a) ${\rm{Sn}}^{5+}$, (b) ${\rm{Sn}}^{8+}$, (c) ${\rm{Sn}}^{10+}$和(d) ${\rm{Sn}}^{13+}$离子的4s, 4p, 4d和4f轨道波函数

Figure 1. Radial wavefunctions of 4s, 4p, 4d and 4f belonging to (a) ${\rm{Sn}}^{5+}$, (b) ${\rm{Sn}}^{8+}$, (c) ${\rm{Sn}}^{10+}$ and (d) ${\rm{Sn}}^{13+}$.

DownLoad: Full-Size Img PowerPoint

图 2 ${\rm{Sn}}^{10+}$束缚组态, 其中长条表示相应组态分裂而成的精细能级的能量范围, 虚线表示电离阈值

Figure 2. Bound configurations of ${\rm{Sn}}^{10+}$. Each bar represents the energy range of fine-structure levels belonging to the corresponding configuration. The dashed line represents ionization threshold.

DownLoad: Full-Size Img PowerPoint

图 3 ${\rm{Sn}}^{10+}$振子强度　(a) 振子强度的长度和速度表示, 红色虚线斜率为1; (b) 波长11—20 nm的振子强度长度和速度表示的比值, 上下两条红色虚线分别表示比值为1和0.8

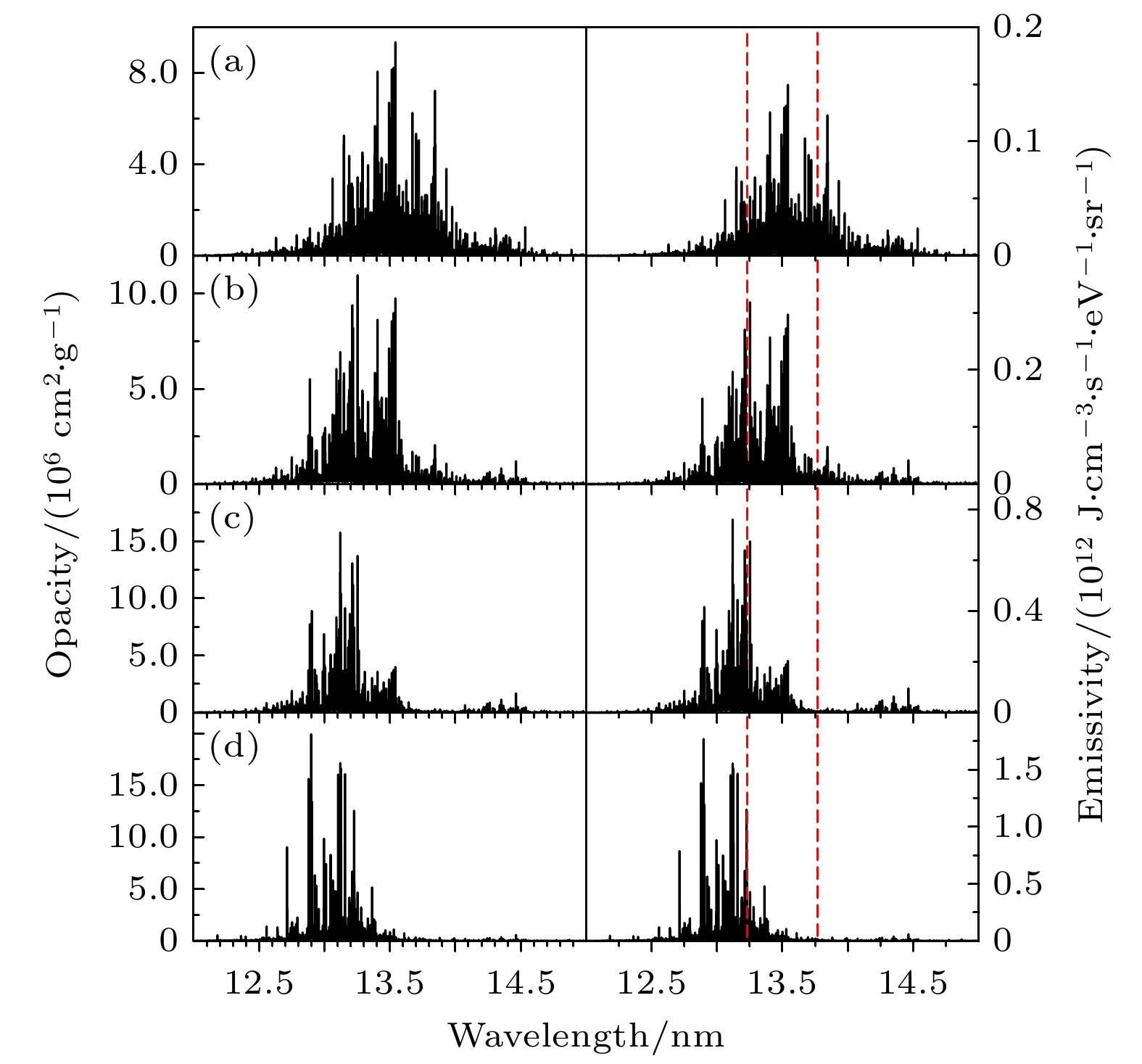
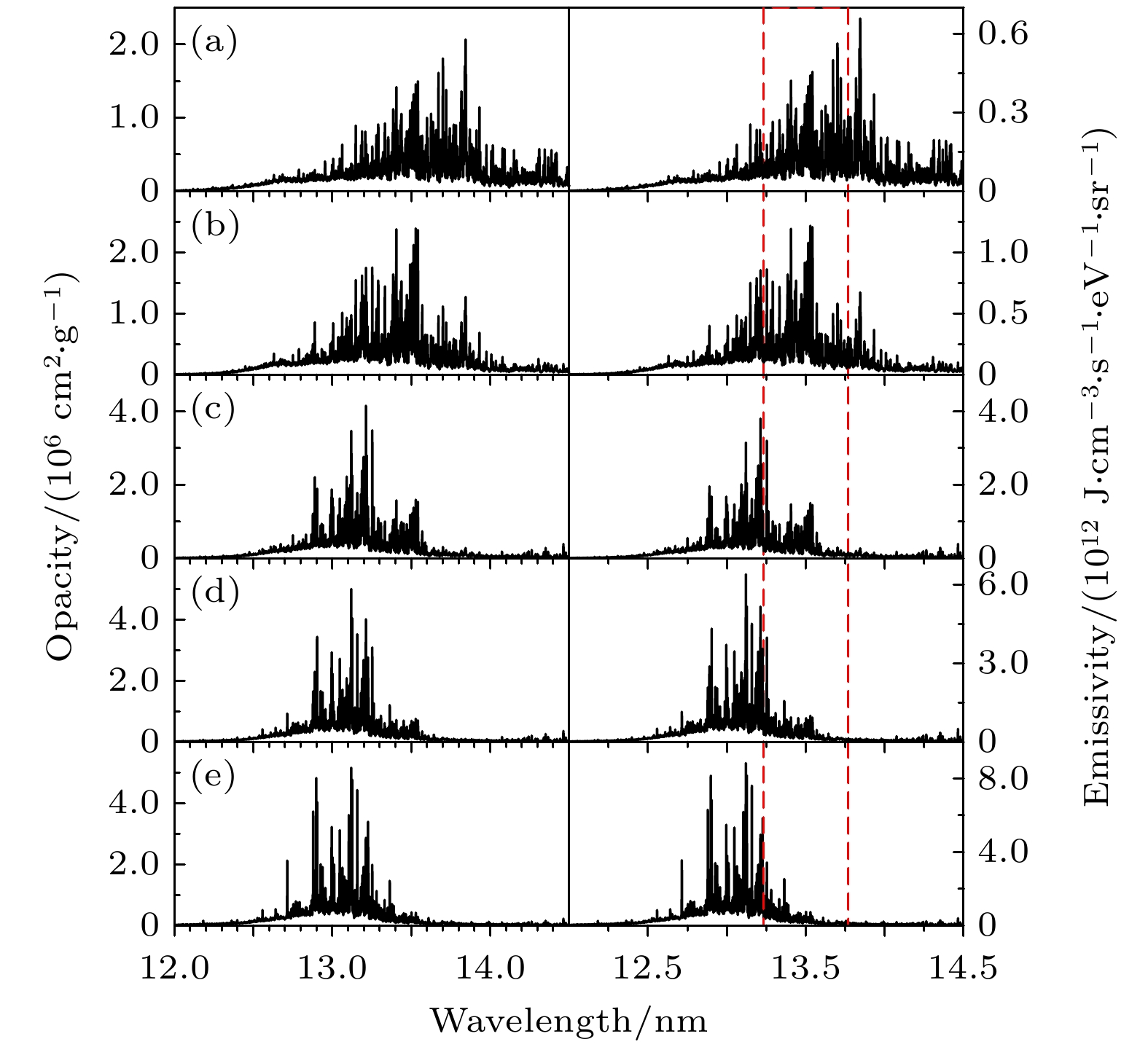
Figure 3. Oscillator strengths of ${\rm{Sn}}^{10+}$: (a) Length and velocity forms of oscillator strengths; the slope of the red dashed line is 1; (b) ratio of length form to velocity form. The upper and lower red dashed lines represent 1 and 0.8, respectively.

DownLoad: Full-Size Img PowerPoint

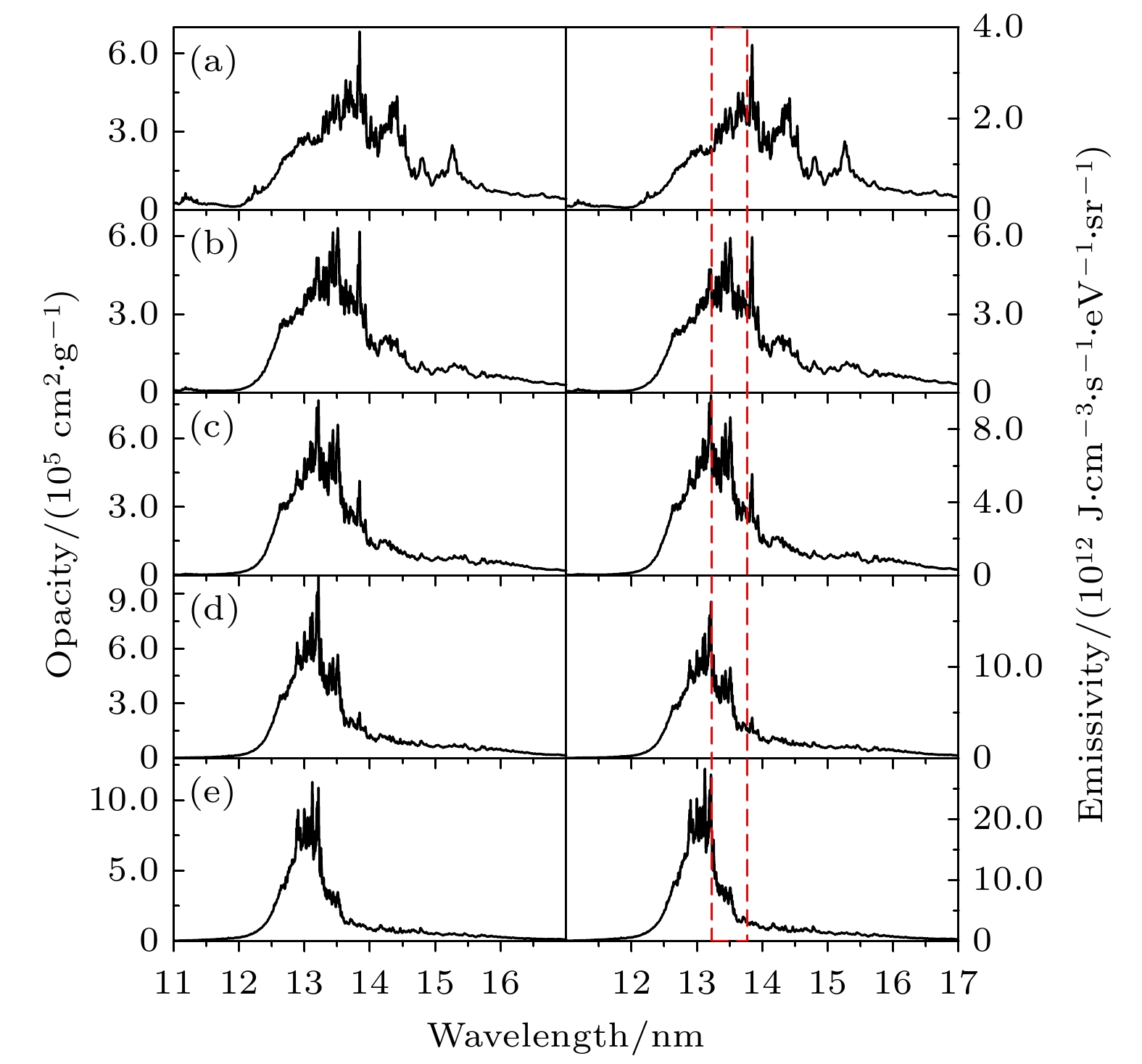
图 4 温度30 eV, 密度0.01 g/cm³条件下Sn等离子体中不同电荷态对辐射不透明度的贡献　(a) ${\rm{Sn}}^{9+}$; (b) ${\rm{Sn}}^{10+}$; (c) ${\rm{Sn}}^{11+}$; (d) ${\rm{Sn}}^{12+}$; (e)总不透明度, 红线为只包含基组态和单电子激发组态的结果, 绿线为包括了基组态、单电子和双电子激发组态的结果

Figure 4. Radiative opacity of Sn at a temperature of 30 eV and a density of 0.01 g/cm³ contributed by different ionization stages: (a) ${\rm{Sn}}^{9+}$; (b) ${\rm{Sn}}^{10+}$; (c) ${\rm{Sn}}^{11+}$; (d) ${\rm{Sn}}^{12+}$; (e) total opacity. The red line represents the result including only ground and singly excited configurations. The green line represents the result including ground, singly and doubly excited configurations

DownLoad: Full-Size Img PowerPoint

图 5 Sn等离子体透射谱　(a)温度30 eV, 密度0.01 g/cm³时本文计算、ATOMIC^[28]与实验^[22]结果; (b) 本文计算的密度0.01 g/cm³, 温度为25, 27, 30和32 eV的Sn等离子体透射谱. 本文计算取仪器展宽为0.5 eV

Figure 5. Transmission spectra of Sn plasmas: (a) Present calculation, ATOMIC^[28] and experimental results^[22] of Sn at a temperature of 30 eV and a density of 0.01 g/cm³; (b) present calculated transmission spectra of Sn at a density of 0.01 g/cm³ and temperatures of 25, 27, 30 and 32 eV. The instrumental broadening in the present calculation is set to be 0.5 eV

DownLoad: Full-Size Img PowerPoint

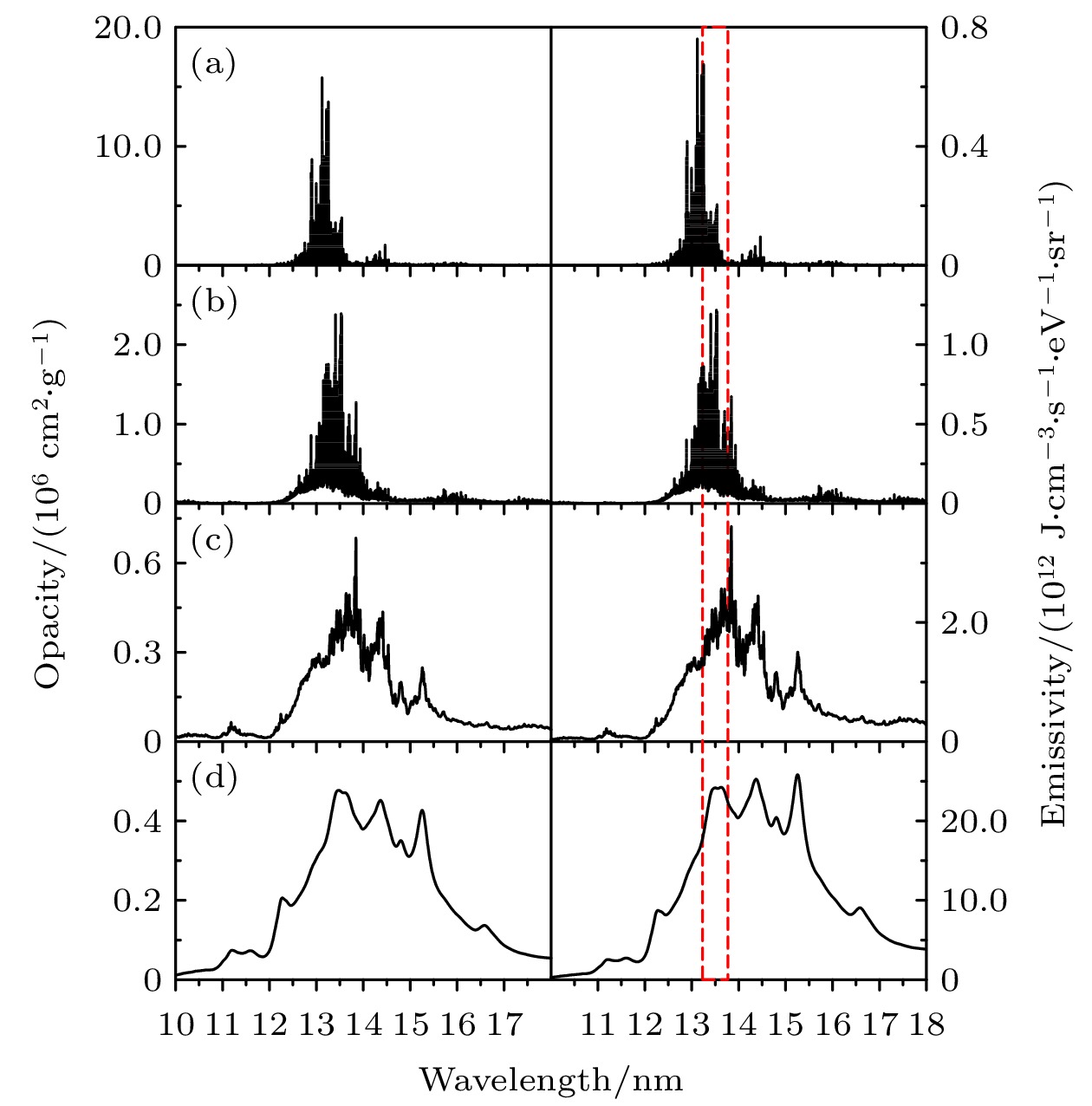
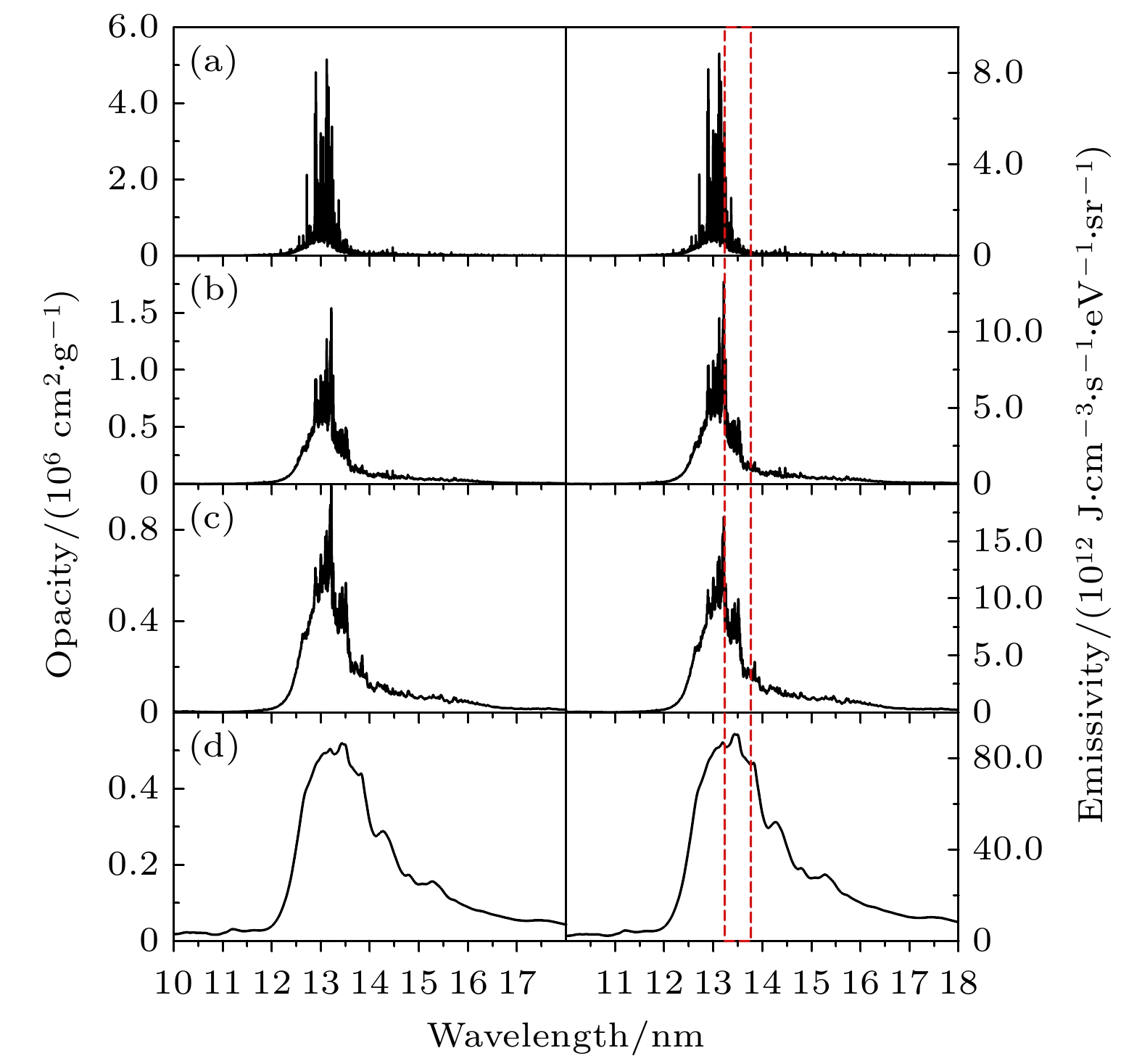
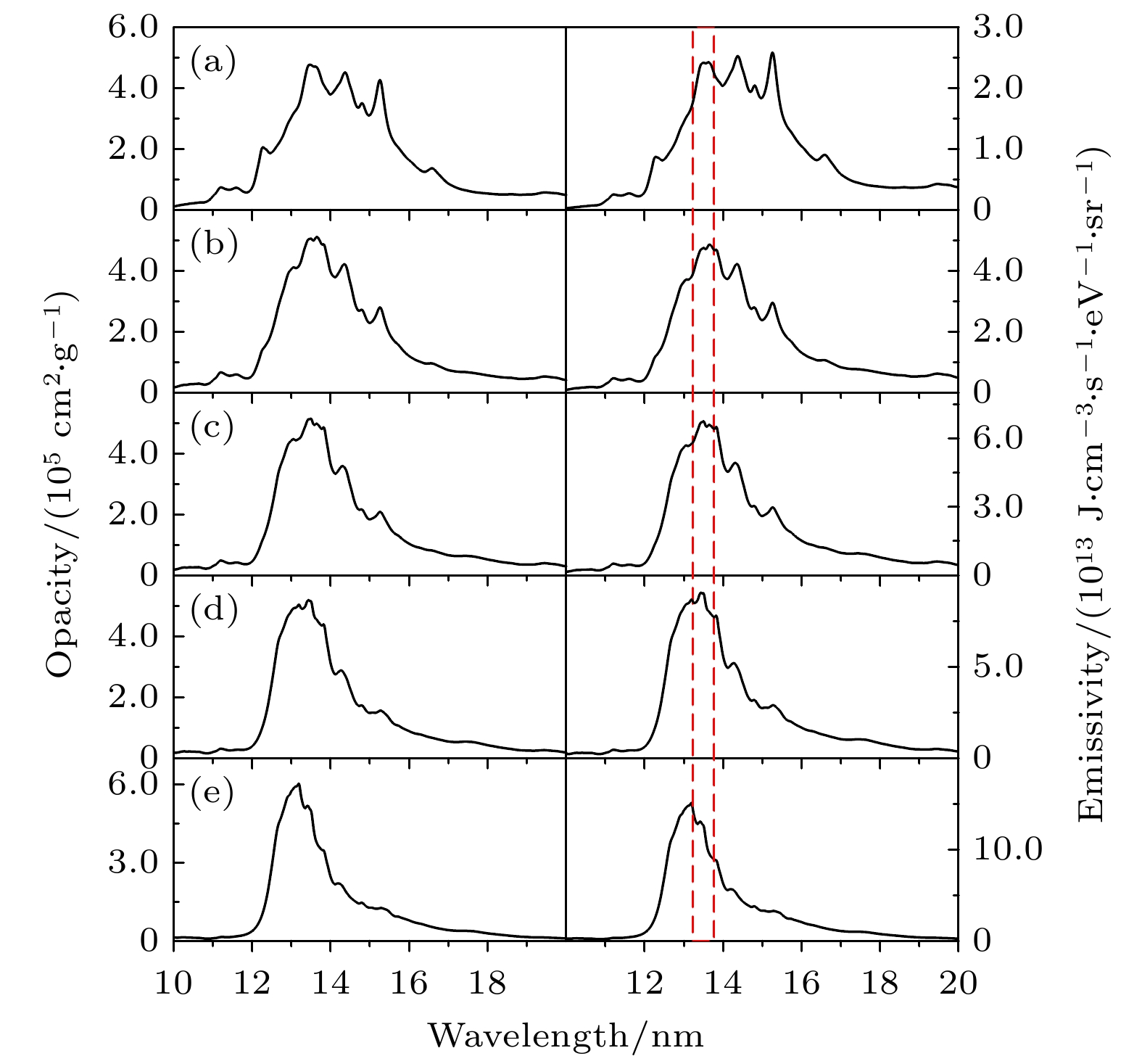
图 6 温度为20 eV, 密度为(a) 0.0001, (b) 0.001, (c) 0.01和(d) 0.1 g/cm³的Sn等离子体EUV辐射不透明度(左图)和发射系数(右图). 平均电离度分别为10.96, 9.63, 8.36和7.16, 自由电子密度分别为5.56 × 10¹⁸, 4.89 × 10¹⁹, 4.24 × 10²⁰和3.63 × 10²¹ cm^–3. 红色虚线表示中心波长13.5 nm, 带宽2%的波长范围

Figure 6. EUV Radiative opacity (left) and emissivity (right) of Sn plasmas at a temperature of 20 eV and densities of (a) 0.0001, (b) 0.001, (c) 0.01 and (d) 0.1 g/cm³. The average ionization is 10.96, 9.63, 8.36 and 7.16, respectively. The free electron density is 5.56 × 10¹⁸, 4.89 × 10¹⁹, 4.24 × 10²⁰ and 3.63 × 10²¹ cm^–3, respectively. The red-dashed lines represent the 2% wavelength region centered at 13.5 nm

DownLoad: Full-Size Img PowerPoint

图 7 温度为23 eV, 密度为(a) 0.001, (b) 0.005, (c) 0.01和(d) 0.1 g/cm³的Sn等离子体EUV辐射不透明度(左图)和发射系数(右图)

Figure 7. EUV Radiative opacity (left panel) and emissivity (right panel) of Sn plasmas at a temperature of 23 eV and densities of (a) 0.001, (b) 0.005, (c) 0.01 and (d) 0.1 g/cm³

DownLoad: Full-Size Img PowerPoint

图 8 温度为27 eV, 密度为(a) 0.001, (b) 0.005, (c) 0.01和(d) 0.1 g/cm³的Sn等离子体EUV辐射不透明度(左图)和发射系数(右图)

Figure 8. EUV Radiative opacity (left panel) and emissivity (right panel) of Sn plasmas at a temperature of 27 eV and densities of (a) 0.001, (b) 0.005, (c) 0.01 and (d) 0.1 g/cm³

DownLoad: Full-Size Img PowerPoint

图 9 密度为0.0001 g/cm³, 温度为(a) 16, (b) 18, (c) 20和(d) 23 eV的Sn等离子体EUV辐射不透明度(左图)和发射系数(右图)

Figure 9. EUV radiative opacity (left panel) and emissivity (right panel) of Sn plasmas at a density of 0.0001 g/cm³ and temperatures of (a) 16, (b) 18, (c) 20 and (d) 23 eV

DownLoad: Full-Size Img PowerPoint

图 10 密度为0.001 g/cm³, 温度为(a) 18, (b) 20, (c) 23, (d) 25和(e) 27 eV的Sn等离子体EUV辐射不透明度(左图)和发射系数(右图)

Figure 10. EUV radiative opacity (left panel) and emissivity (right panel) of Sn plasmas at a density of 0.001 g/cm³ and temperatures of (a) 18, (b) 20, (c) 23, (d) 25 and (e) 27 eV

DownLoad: Full-Size Img PowerPoint

图 12 密度为0.1 g/cm³, 温度为(a) 20, (b) 23, (c) 25, (d) 27和(e) 30 eV的Sn等离子体EUV辐射不透明度(左图)和发射系数(右图)

Figure 12. EUV radiative opacity (left panel) and emissivity (right panel) of Sn plasmas at a density of 0.1 g/cm³ and temperatures of (a) 20, (b) 23, (c) 25, (d) 27 and (e) 30 eV

DownLoad: Full-Size Img PowerPoint

图 11 密度为0.01 g/cm³, 温度为(a) 20, (b) 23, (c) 25, (d) 27和(e) 30 eV的Sn等离子体EUV辐射不透明度(左图)和发射系数(右图)

Figure 11. EUV radiative opacity (left panel) and emissivity (right panel) of Sn plasmas at a density of 0.01 g/cm³ and temperatures of (a) 20, (b) 23, (c) 25, (d) 27 and (e) 30 eV

DownLoad: Full-Size Img PowerPoint

表 1 ${\rm{Sn}}^{10+}$基组态4s²4p⁶4d⁴的精细能级(单位: eV), 能级符号中省略了满壳层的4s和4p轨道.

Table 1. Fine-structure levels belonging to the ground configuration 4s²4p⁶4d⁴ of ${\rm{Sn}}^{10+}$ (Unit: eV), where the fully occupied 4s and 4p orbitals are omitted.

	能级	J	本文(MCDF)	实验^[14]	CI+MBPT ^[14]
1	${\rm{4d}}_{3/2}^4$	0	0.00	0.00	0.00
2	$(\text{4d}_{3/2}^3)_{3/2}\text{4d}_{5/2}$	1	0.36	0.38	0.39
3	$(\text{4d}_{3/2}^2)_{2}(\text{4d}_{5/2}^2)_4$	2	0.79	0.82	0.83
4	$(\text{4d}_{3/2}^2)_{2}(\text{4d}_{5/2}^2)_4$	3	1.22	1.25	1.27
5	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{9/2}$	4	1.64	1.65	1.68
6	$(\text{4d}_{3/2}^2)_{2}(\text{4d}_{5/2}^2)_2$	0	3.48	3.32	3.28
7	$(\text{4d}_{3/2}^3)_{3/2}\text{4d}_{5/2}$	4	3.98	3.67	3.60
8	$(\text{4d}_{3/2}^2)_2(\text{4d}_{5/2}^2)_2$	3	4.58	4.33	4.29
9	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{9/2}$	5	4.61	4.36	4.30
10	$(\text{4d}_{3/2}^2)_2(\text{4d}_{5/2}^2)_2$	1	4.68	4.39	4.39
11	$(\text{4d}_{3/2}^3)_{3/2}\text{4d}_{5/2}$	2	4.80	4.55	4.54
12	$(\text{4d}_{3/2}^2)_2(\text{4d}_{5/2}^2)_4$	6	5.09	4.74	4.67
13	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{9/2}$	4	5.29	5.02	4.99
14	$(\text{4d}_{3/2}^2)_2(\text{4d}_{5/2}^2)_2$	2	5.61	5.38	5.40
15	$(\text{4d}_{3/2}^2)_2(\text{4d}_{5/2}^2)_2$	4	5.72	5.43	5.40
16	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{3/2}$	3	5.87	5.60	5.60
17	$(\text{4d}_{3/2}^2)_2(\text{4d}_{5/2}^2)_2$	3	6.27	5.99	5.99
18	$(\text{4d}_{3/2}^2)_2(\text{4d}_{5/2}^2)_4$	5	6.36	6.06	6.03
19	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{3/2}$	2	6.69	6.42	6.43
20	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{3/2}$	1	6.81	6.55	6.56
21	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{9/2}$	6	7.15	6.68	6.60
22	$\text{4d}_{5/2}^4$	4	7.72	7.40	7.38
23	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{3/2}$	0	7.77	7.55	7.57
24	$\text{4d}_{5/2}^4$	2	8.41	8.01	8.00
25	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{3/2}$	3	8.89	8.43	8.42
26	$(\text{4d}_{3/2}^2)_0(\text{4d}_{5/2}^2)_2$	2	9.86	—	9.40
27	$(\text{4d}_{3/2}^2)_0(\text{4d}_{5/2}^2)_4$	4	10.27	9.77	9.78
28	$(\text{4d}_{3/2}^2)_2(\text{4d}_{5/2}^2)_0$	2	10.48	9.97	9.98
29	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{5/2}$	3	10.66	10.15	10.16
30	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{5/2}$	1	10.77	—	10.30
31	$\text{4d}_{5/2}^4$	0	11.23	—	10.77
32	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{5/2}$	4	11.79	—	11.11
33	$\text{4d}_{3/2}(\text{4d}_{5/2}^3)_{5/2}$	2	14.70	—	13.95
34	$(\text{4d}_{3/2}^2)_0(\text{4d}_{5/2}^2)_0$	0	18.87	—	17.98

DownLoad: CSV

表 2 不同温度和密度条件下, Sn等离子体在(13.5 ± 2%) nm波长范围的总发射功率. a(b)表示a × $10^{b}$

Table 2. Total emissivity of Sn plasmas in (13.5 ± 2%) nm wavelength region at a variety of temperature and density. a(b) represents a × $10^{b}$

$T_{\rm{e}}$/eV	ρ/(g·cm^–3)	$j_{{\rm{tot}}}$/(W·cm^–3)
20	0.0001	4.52(10)
	0.001	7.86(11)
	0.01	6.68(12)
	0.1	8.36(13)
23	0.0001	5.50(10)
	0.001	1.01(12)
	0.01	1.42(13)
	0.1	1.69(14)
27	0.001	1.13(12)
	0.005	9.07(12)
	0.01	2.24(13)
	0.1	3.11(14)

DownLoad: CSV

map

[1]	Pirati A, Van Shoot J, Troost K, van Ballegoij R, Krabbendam P, Stoeldraijer J, Loopstra E, Benschop J, Finders J, Meiling H, van Setten E, Mika N, Driedonkx J, Stamm U 2017 Proc. SPIE 10143 101430G Google Scholar
[2]	Hutcheson G D 2018 Proc. SPIE 10583 1058303
[3]	David A 2007 Soft x-rays and Extreme Ultraviolet Radiation: Principles and Applications (Cambridge: Cambridge University Press) pp17–20
[4]	宗楠, 胡蔚敏, 王志敏, 王小军, 张申金, 薄勇, 彭钦军, 许祖彦 2020 中国光学 13 28 Google Scholar Zong N, Hu W M, Wang Z M, Wang X J, Zhang S J, Bo Y, Peng Q J, Xu Z Y 2020 Chin. Opt. 13 28 Google Scholar
[5]	Versolato O O 2019 Plasma Sources Sci. Technol. 28 083001 Google Scholar
[6]	Kieft E R, Garloff K, van der Mullen J J A M, Banine V 2005 Phys. Rev. E 71 036402 Google Scholar
[7]	Ueno Y, Soumagne G, Sumitani A, Endo A, Higashiguchi T 2007 Appl. Phys. Lett. 91 231501 Google Scholar
[8]	Svendsen W, O’Sullivan G 1994 Phys. Rev. A 50 3710 Google Scholar
[9]	Churilov S S, Ryabtsev A N 2006 Opt. Spectrosc. 101 169 Google Scholar
[10]	D'Arcy R, Ohashi H, Suda S, Tanuma H, Fujioka S, Nishimura H, Nishihara K, Suzuki C, Kato T, Koike F, White J, O’Sullivan G 2009 Phys. Rev. A 79 042509 Google Scholar
[11]	Ohashi H, Suda S, Tanuma H, Fujioka S, Nishimura H, Sasaki A, Nishihara K 2010 J. Phys. B: At. Mol. Opt. Phys. 43 065204 Google Scholar
[12]	O'Sullivan G, Li B, D’Arcy R, Dunne P, Hayden P, Kilbane D, McCormack T, Ohashi H, O'Reilly F, Sheridan P, Sokell E, Suzuki C, Higashiguchi T 2015 J. Phys. B: At. Mol. Opt. Phys. 48 144025 Google Scholar
[13]	Windberger A, Torretti F, Borschevsky A, Ryabtsev A, Dobrodey S, Bekker H, Eliav E, Kaldor U, Ubachs W, Hoekstra R, Crespo López-Urrutia J R, Versolato O O 2016 Phys. Rev. A 94 012506 Google Scholar
[14]	Torretti F, Windberger A, Ryabtsev A, Dobrodey S, Bekker H, Ubachs W, Hoekstra R, Kahl E V, Berengut J C, Crespo López-Urrutia J R, Versolato O O 2017 Phys. Rev. A 95 042503 Google Scholar
[15]	Torretti F, Schupp R, Kurilovich D, Bayerle A, Scheers J, Ubachs W, Hoekstra R, Versolato O O 2018 J. Phys. B: At. Mol. Opt. Phys. 51 045005 Google Scholar
[16]	de Gaufridy de Dortan F 2007 J. Phys. B: At. Mol. Opt. Phys. 40 599 Google Scholar
[17]	Koike F, Fritzsche S 2007 Radiat. Phys. Chem. 76 404 Google Scholar
[18]	Kagawa T, Tanuma H, Ohashi H, Nishihara K 2007 J. Phys.: Conf. Ser. 58 149 Google Scholar
[19]	Eliav E, Kaldor U, Ishikawa Y 1994 Phys. Rev. A 49 1724 Google Scholar
[20]	Berengut J C, Flambaum V V, Kozlov M G 2006 Phys. Rev. A 73 012504 Google Scholar
[21]	Lysaght M, Kilbane D, Murphy N, Cummings A, Dunne P, O’Sullivan G 2005 Phys. Rev. A 72 014502 Google Scholar
[22]	Fujioka S, Nishimura H, Nishihara K, Sasaki A, Sunahara A, Okuno T, Ueda N, Ando T, Tao Y, Shimada Y, Hashimoto K, Yamaura M, Shigemori K, Nakai M, Nagai K, Norimatsu T, Nishikawa T, Miyanaga N, Izawa Y, Mima K 2005 Phys. Rev. Lett. 95 235004 Google Scholar
[23]	蔡懿, 王文涛, 杨明, 刘建胜, 陆培祥, 李儒新, 徐至展 2008 57 5100 Google Scholar Cai Y, Wang W T, Yang M, Liu J S, Lu P X, Li R X, Xu Z Z 2008 Acta Phys. Sin. 57 5100 Google Scholar
[24]	陈鸿, 兰慧, 陈子琪, 刘璐宁, 吴涛, 左都罗, 陆培祥, 王新兵 2015 64 075202 Google Scholar Chen H, Lan H, Chen Z Q, Liu L N, Wu T, Zuo D L, Lu P X, Wang X B 2015 Acta Phys. Sin. 64 075202 Google Scholar
[25]	Su M G, Min Q, Cao S Q, Sun D X, Hayden P, O’Sullivan G, Dong C Z 2017 Sci. Rep. 7 45212 Google Scholar
[26]	Schupp R, Torretti F, Meijer R A, Bayraktar M, Sheil J, Scheers J, Kurilovich D, Bayerle A, Schafgans A A, Purvis M, Eikema K S E, Witte S, Ubachs W, Hoekstra R, Versolato O O 2019 Appl. Phys. Lett. 115 124101 Google Scholar
[27]	Zeng J, Gao C, Yuan J 2010 Phys. Rev. E 82 026409 Google Scholar
[28]	Colgan J, Kilcrease D P, Jr. Abdallah J, Sherrill M E, Fontes C J, Hakel P, Armstrong G S J 2017 High Energy Density Phys. 23 133 Google Scholar
[29]	Torretti F, Sheil J, Schupp R, Basko M M, Bayraktar M, Meijer R A, Witte S, Ubachs W, Hoekstra R, Versolato O O, Neukirch A J, Colgan J 2020 Nat. Commun. 11 2334 Google Scholar
[30]	Sheil J, Versolato O O, Neukirch A J, Colgan J 2021 J. Phys. B: At. Mol. Opt. Phys. 54 035002 Google Scholar
[31]	Cowan R D 1981 The Theory of Atomic Structure and Spectra (Berkeley: University of California Press
[32]	Jönson P, Gaigalas G, Bieroń, Froese Fischer C, Grant I P 2013 Comput. Phys. Commun. 184 2197 Google Scholar
[33]	Zeng J L, Yuan J M 2006 Phys. Rev. E 74 025401(R Google Scholar
[34]	Gao C, Zeng J, Jin F, Yuan J 2013 High Energy Density Phys. 9 419 Google Scholar
[35]	Zeng J L, Gao C, Liu P F, Li Y J, Meng C S, Hou Y, Kang D D, Yuan J M 2022 Sci. China-Phys. Mech. Astron. 65 233011 Google Scholar
[36]	Stewart J C, Pyatt K D Jr. 1966 Astrophys. J. 144 1203 Google Scholar
[37]	李世昌 1992 高温辐射物理与量子辐射理论 (北京: 国防工业出版社) 第6页 Li S C 1992 High Temperature Radiation Physics and Quantum Radaition Theory (Beijing: National Defense Industry Press) p6
[38]	Zeng J L, Zhao G, Yuan J M 2006 J. Quant. Spectrosc. Radiat. Transfer 102 172 Google Scholar
[39]	Wu Z W, Tian Z Q, An Y H, Dong C Z 2021 Astrophys. J. 910 142 Google Scholar
[40]	Kühn S, Cheung C, Oreshkina N S, Steinbrügge, Togawa M, Bernitt S, Berger L, Buck J, Hoesch M, Seltmann J, Trinter F, Keitel C H, Kozlov M G, Porsev S G, Gu M F, Porter F S, Pfeifer T, Leutenegger M A, Harman Z, Safronova M S, López-Urrutia J R C, Shah C 2022 Phys. Rev. Lett. 129 245001 Google Scholar
[41]	Colgan J, Kilcrease D P, Magee N H, Sherrill M E, Abdallah Jr. J, Hakel P, Fontes C J, Guzik J A, Mussack K A 2016 Astrophys. J. 817 116 Google Scholar
[42]	兰慧 2016 博士学位论文 (武汉: 华中科技大学) Lan H 2016 Ph. D. Dissertation (Wuhan: Huazhong University of Science and Technology
[43]	Hansen S B, Chung H K, Fontes C J, Ralchenko Yu, Scott H A, Stambulchik E 2020 High Energy Density Phys. 35 100693 Google Scholar
[44]	Gao C, Zeng J, Li Y, Jin F, Yuan J 2013 High Energy Density Phys. 9 583 Google Scholar

[1]	Luo Yan, Yu Xuan, Lei Jian-Ting, Tao Chen-Yu, Zhang Shao-Feng, Zhu Xiao-Long, Ma Xin-Wen, Yan Shun-Cheng, Zhao Xiao-Hui. Fragmentation mechanism of methane dehydrogenation channel induced by extreme ultraviolet and high charge ions. Acta Physica Sinica, 2024, 73(4): 044101. doi: 10.7498/aps.73.20231377
[2]	Li Hui, Tan Fang-Rui, Yin Hao-Yu, Ma Yue-Yang, Wu Xiao-Bin. Simulation study of decoherence and light intensity uniformization for extreme ultraviolet of uniform light pipe. Acta Physica Sinica, 2024, 73(11): 114201. doi: 10.7498/aps.73.20240335
[3]	Si Ming-Qi, Wen Zhi-Lin, Zhang Qi-Jin, Dou Yin-Ping, Li Bo-Chao, Song Xiao-Wei, Xie Zhuo, Lin Jing-Quan. Radiation of extreme ultraviolet source and out-of-band from laser-irradiated low-density SnO₂ target. Acta Physica Sinica, 2023, 72(6): 065201. doi: 10.7498/aps.72.20222385
[4]	Xie Zhuo, Wen Zhi-Lin, Si Ming-Qi, Dou Yin-Ping, Song Xiao-Wei, Lin Jing-Quan. Characteristics of extreme ultraviolet emission from Gd plasma produced by dual pulse laser. Acta Physica Sinica, 2022, 71(3): 035202. doi: 10.7498/aps.71.20211450
[5]	. The characteristics of extreme ultraviolet emission from Gd plasma produced by dual pulse laser. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20211450
[6]	Hai Bang, Zhang Shao-Feng, Zhang Min, Dong Da-Pu, Lei Jian-Ting, Zhao Dong-Mei, Ma Xin-Wen. A tabletop experimental system for investigating ultrafast atomic dynamics based on femtosecond extreme ultraviolet photons. Acta Physica Sinica, 2020, 69(23): 234208. doi: 10.7498/aps.69.20201035
[7]	Yu Geng-Hua, Yan Hui, Gao Dang-Li, Zhao Peng-Yi, Liu Hong, Zhu Xiao-Ling, Yang Wei. Calculationof isotope shift of Mg+ ion by using the relativistic multi-configuration interaction method. Acta Physica Sinica, 2018, 67(1): 013101. doi: 10.7498/aps.67.20171817
[8]	Zhou Rui, Li Chuan-Liang, He Xiao-Hu, Qiu Xuan-Bing, Meng Hui-Yan, Li Ya-Chao, Lai Yun-Zhong, Wei Ji-Lin, Deng Lun-Hua. Spectroscopic properties of low-lying excited electronic states for CF- anion based on ab initio calculation. Acta Physica Sinica, 2017, 66(2): 023101. doi: 10.7498/aps.66.023101
[9]	Dou Yin-Ping, Xie Zhuo, Song Xiao-Lin, Tian Yong, Lin Jing-Quan. Experimental research on laser-produced Gd target plasma source for 6.7 nm lithography. Acta Physica Sinica, 2015, 64(23): 235202. doi: 10.7498/aps.64.235202
[10]	Chen Hong, Lan Hui, Chen Zi-Qi, Liu Lu-Ning, Wu Tao, Zuo Du-Luo, Lu Pei-Xiang, Wang Xin-Bing. Experimental study on laser produced tin droplet plasma extreme ultraviolet light source. Acta Physica Sinica, 2015, 64(7): 075202. doi: 10.7498/aps.64.075202
[11]	Xiong Zhuang, Wang Zhen-Xin, Naoum C. Bacalis. Accuracy study for excited atoms (ions)：A new variational method. Acta Physica Sinica, 2014, 63(5): 053104. doi: 10.7498/aps.63.053104
[12]	Zhao Yong-Peng, Xu Qiang, Xiao De-Long, Ding Ning, Xie Yao, Li Qi, Wang Qi. Time behavior and optimum conditions for the Xe gas extreme ultraviolet source. Acta Physica Sinica, 2013, 62(24): 245204. doi: 10.7498/aps.62.245204
[13]	Li Zun-Mao, Xiong Zhuang, Dai Li-Li. Calculation of geometrically active atomic state. Acta Physica Sinica, 2010, 59(11): 7824-7829. doi: 10.7498/aps.59.7824
[14]	Cai Yi, Wang Wen-Tao, Yang Ming, Liu Jian-Sheng, Lu Pei-Xiang, Li Ru-Xin, Xu Zhi-Zhan. Experimental study on extreme ultraviolet light generation from high power laser-irradiated tin slab. Acta Physica Sinica, 2008, 57(8): 5100-5104. doi: 10.7498/aps.57.5100
[15]	Yuan Wei-Guo, Dai Chang-Jian, Jin Song, Zhao Hong-Ying, Guan Feng. Study of Ba 6pnd(J=1, 3)autoionizing states. Acta Physica Sinica, 2008, 57(7): 4076-4082. doi: 10.7498/aps.57.4076
[16]	Wan Jian-Jie, Xie Lu-You, Dong Chen-Zhong, Jiang Jun, Yan Jun. Theoretical study of forbidden M1, M2, E2 transitions for highly charged Ni-like ions. Acta Physica Sinica, 2007, 56(1): 152-159. doi: 10.7498/aps.56.152
[17]	Liu Ling-Tao, Wang Min-Sheng, Han Xiao-Ying, Li Jia-Ming. Photonionization and radiative recombination of Br——Comparison of rate coefficients deduced form the average atom and detailed configuration models. Acta Physica Sinica, 2006, 55(5): 2322-2327. doi: 10.7498/aps.55.2322
[18]	Huang Chun-Jia, He Hui-Yong, Li Jiang-Fan, Zhou Ming. . Acta Physica Sinica, 2002, 51(5): 1049-1053. doi: 10.7498/aps.51.1049
[19]	GAO YUN-FENG, FENG JIAN, SHI SHU-REN. CAVITY FIELD SPECTRA OF AN ATOM INTERACTING WITH TWO-MODE FIELD THROUGH RAMAN PROCESS. Acta Physica Sinica, 2001, 50(8): 1496-1500. doi: 10.7498/aps.50.1496
[20]	QI JING-BO, CHEN CHONG-YANG, WANG YAN-SEN. ELECTRON IMPACT IONIZATION CROSS SECTIONS FOR THE Na-LIKE IONS. Acta Physica Sinica, 2001, 50(8): 1475-1480. doi: 10.7498/aps.50.1475

Catalog

Vol. 72, Issue 18 - 2023-09-20

Metrics

Abstract views: 3720
PDF Downloads: 128
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板