-
The coupling of ballooning mode and peeling mode forms the so-called peeling-ballooning mode, which is widely used in the physical explanation of the edge localized mode (ELM). The nonlinear platform simulation based on the non-ideal peeling-ballooning mode model successfully explained the ELM experimental results. Therefore, exploring the influences of various non-ideal effects on the ballooning mode in the edge transport barrier is very important in controlling the ELM in the future fusion reactors. Among the reports on non-ideal effects, there are few reports involving the effect of hyper-resistivity caused by anomalous electron viscosity on ballooning mode. It has been found that the hyper-resistivity has a destabilizing effect on the ballooning mode, but the associated physical mechanism is still unclear. Therefore, it is necessary to systematically explore the influence of hyper-resistivity on the ballooning mode theoretically by introducing hyper-resistivity into the ballooning mode model. The linear growth rate of ideal and non-ideal ballooning mode are solved by the shooting method for the derived eigenvalue equation of non-ideal ballooning mode containing hyper-resistivity, finite resistivity and diamagnetic drift effects, and the dependence of ballooning mode on hyper-resistivity is also explored under different conditions. The results show that the hyper-resistivity may destabilize the ballooning mode, and the physical mechanism is that the current diffusion effect caused by the hyper-resistivity weakens the stabilizing effect of the magnetic field line bending on the ballooning mode. When both the resistivity and hyper-resistivity are considered, they are in a competitive relationship. When the ratio of hyper-resistivity to resistivity is relatively high, hyper-resistivity plays a dominant role, and the destabilizing effect of resistivity will be shielded by hyper-resistivity, and vice versa. The destabilization effect of hyper-resistivity on ballooning modes is enhanced with the increase of the toroidal mode number. The hyper-resistivity will destabilize the original stable modes once the toroidal mode number exceeds a certain threshold. Further studies show that the threshold is inversely proportional to the ratio of hyper-resistivity to resistivity. The research results have important reference value for the control of edge localized modes in low-collisionality edge plasma in future fusion reactors.
-
Keywords:
- tokamak /
- magnetohydrodynamic instability /
- ballooning modes /
- hyper-resistivity
[1] La Haye R J 2006 Phys. Plasmas 13 055501
Google Scholar
[2] Connor J W, Hastie R J, Taylor J B 1978 Phys. Rev. Lett. 40 396
Google Scholar
[3] Glenn Bateman, Nelson D B 1978 Phys. Rev. Lett. 41 1804
Google Scholar
[4] Strauss H R 1981 Phys. Fluids 24 2004
Google Scholar
[5] Dark J F, Antonsen Jr T M 1985 Phys. Fluids 28 544
Google Scholar
[6] Lortz D, Nuhrenberg J 1978 Phys. Lett. A 68 49
Google Scholar
[7] Coppi B, Ferreira A, Ramos J 1980 Phys. Rev. Lett. 44 990
Google Scholar
[8] Strauss H R, Park W, Monticello D A, White R B 1980 Nucl. Fusion 20 638
[9] Snyder P B, Wilson H R, Ferron J R, Lao L L, Leonard A W, Osborne T H, Turnbull A D, Mossessian D, Murakami M, Xu X Q 2002 Phys. Plasmas 9 2037
Google Scholar
[10] Strauss H R 1986 Phys. Fluids 29 3668
Google Scholar
[11] Kaw P K, Valeo E J, Rutherford P H 1979 Phys. Rev. Lett. 43 1398
Google Scholar
[12] Wu N, Chen S Y, Mou M L, Tang C J 2018 Phys. Plasmas 25 092305
Google Scholar
[13] Connor J W, Hastie R J, Wilson H R 1998 Phys. Plasmas 5 2687
Google Scholar
[14] Xu X Q, Dudson B, Snyder P B, Umansky M V, Wilson H 2010 Phys. Rev. Lett. 105 175005
Google Scholar
[15] Rhee T, Park G Y, Jhang H, Kim S S, Singh R 2017 Phys. Plasmas 24 072504
Google Scholar
[16] Rhee T, Kim S S, Jhang H, Park G Y, Singh R 2015 Nucl. Fusion 55 032004
Google Scholar
[17] Jhang H, Kaang H H, Kim S S, Rhee T, Singh R, Hahm T S 2017 Nucl. Fusion 57 022006
Google Scholar
[18] Miller R L, Chu M S, Greene J M, Lin-Liu Y R, Waltz R E 1998 Phys. Plasmas 5 973
Google Scholar
[19] Mou M L, Jhang H, Rhee T, Chen S Y, Tang C J 2018 Phys. Plasmas 25 082518
Google Scholar
[20] Xia T Y, Xu X Q, Dudson B D, Li J 2012 Contrib. Plasma Phys. 52 353
Google Scholar
[21] Tang W M, Dewar R L, Manickam J 1982 Nucl. Fusion 22 1079
Google Scholar
-
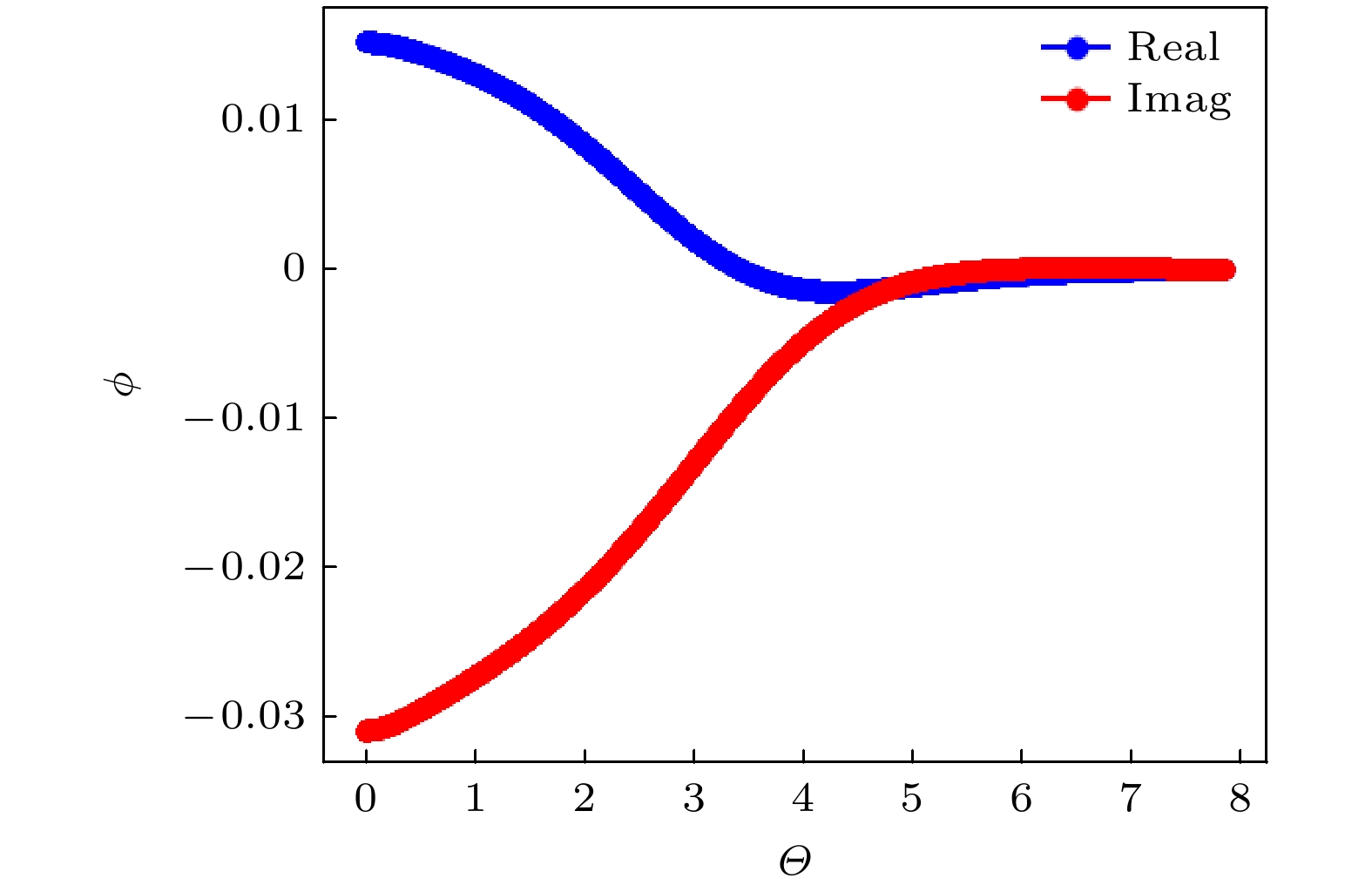
图 1 考虑超电阻的非理想气球模本征方程函数解(
$R= $ $ 3.52\;{\rm{m}}$ ,$r=1.24\;{\rm{m}}$ ,$ q=2.35 $ ,$s=2.59 $ ,$ \alpha =0.46 $ ,$n= $ $ 35$ ,$\eta ={10}^{-7}$ ,$ {\eta }_{{\rm{H}}}={9\times 10}^{-15} $ )Figure 1. Eigen-functions of the ballooning model with hyper-resistivity (
$R=3.52\;{\rm{m}}$ ,$r=1.24\;{\rm{m}}$ ,$ q=2.35 $ ,$s=2.59 $ ,$ \alpha =0.46 $ ,$ n=35 $ ,$\eta ={10}^{-7}$ ,$ {\eta }_{{\rm{H}}}={9\times 10}^{-15} $ ).
图 2 超电阻对理想气球模线性增长率的影响, 其中横坐标
$ n $ 表示环向模数, 纵坐标为归一化气球模线性增长率Figure 2. Effect of hyper-resistivity on the linear growth rate of ideal ballooning modes, where the x-coordinate represents the toroidal mode number, and the y-coordinate is the linear growth rate of ballooning modes.
图 3 不同电阻和超电阻条件下气球模线性增长率随环向模数的变化, 其中超电阻和电阻的比值
$ {\alpha }_{{\rm{H}}}={10}^{-7} $ 保持不变Figure 3. Linear growth rate of ballooning modes varies with toroidal mode number under different resistivity and hyper-resistivity, the ratio of hyper-resistivity to resistivity remain unchanged, where
$ {\alpha }_{{\rm{H}}}={10}^{-7} $ .
图 4 保持超电阻大小不变(
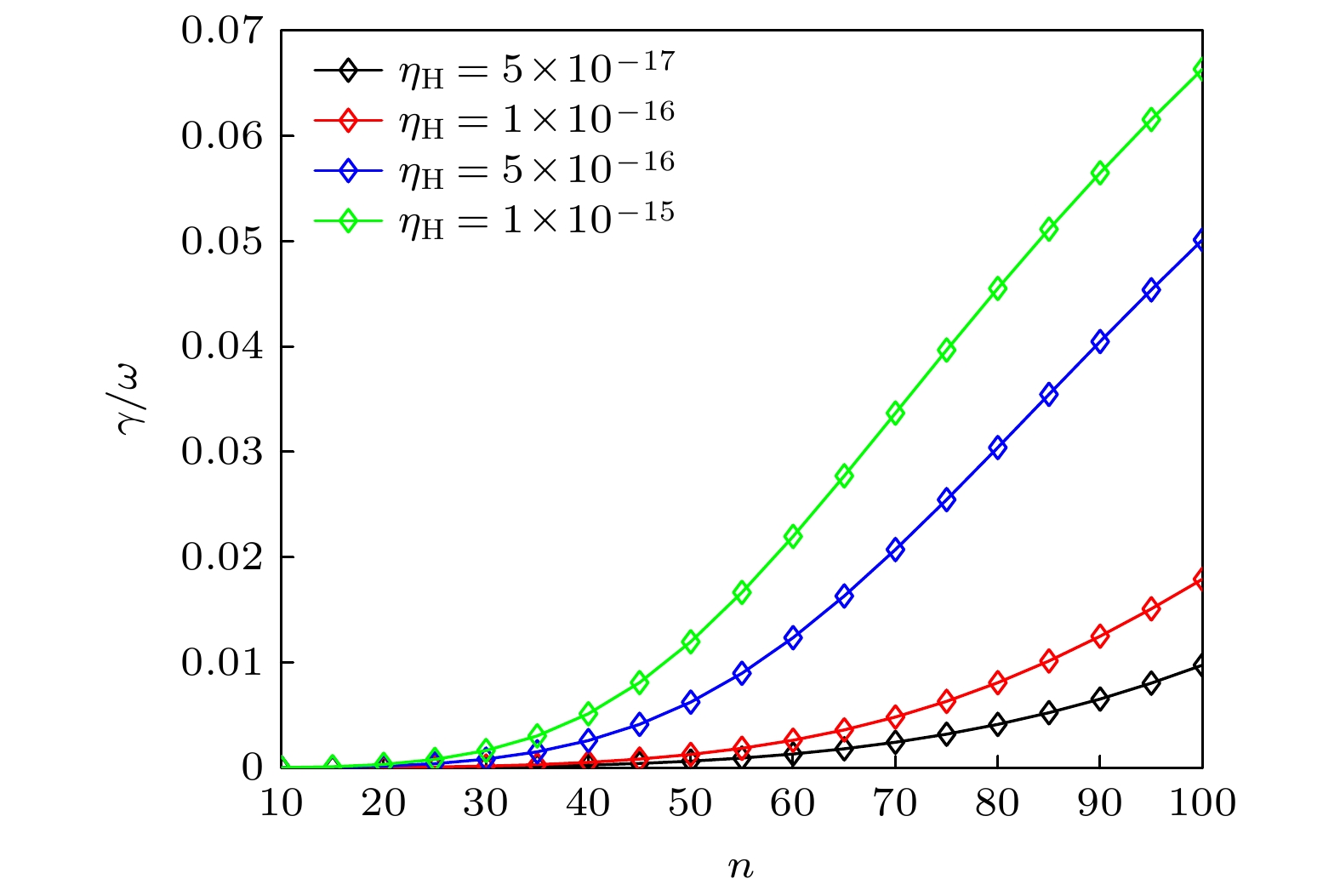
$ {\eta }_{{\rm{H}}}={10}^{-16} $ ), 不同电阻条件下气球模线性增长率随环向模数的变化Figure 4. The linear growth rate of the ballooning mode varies with the toroidal mode number under different resistivity conditions, keeping the values of the hyper-resistivity unchanged, where
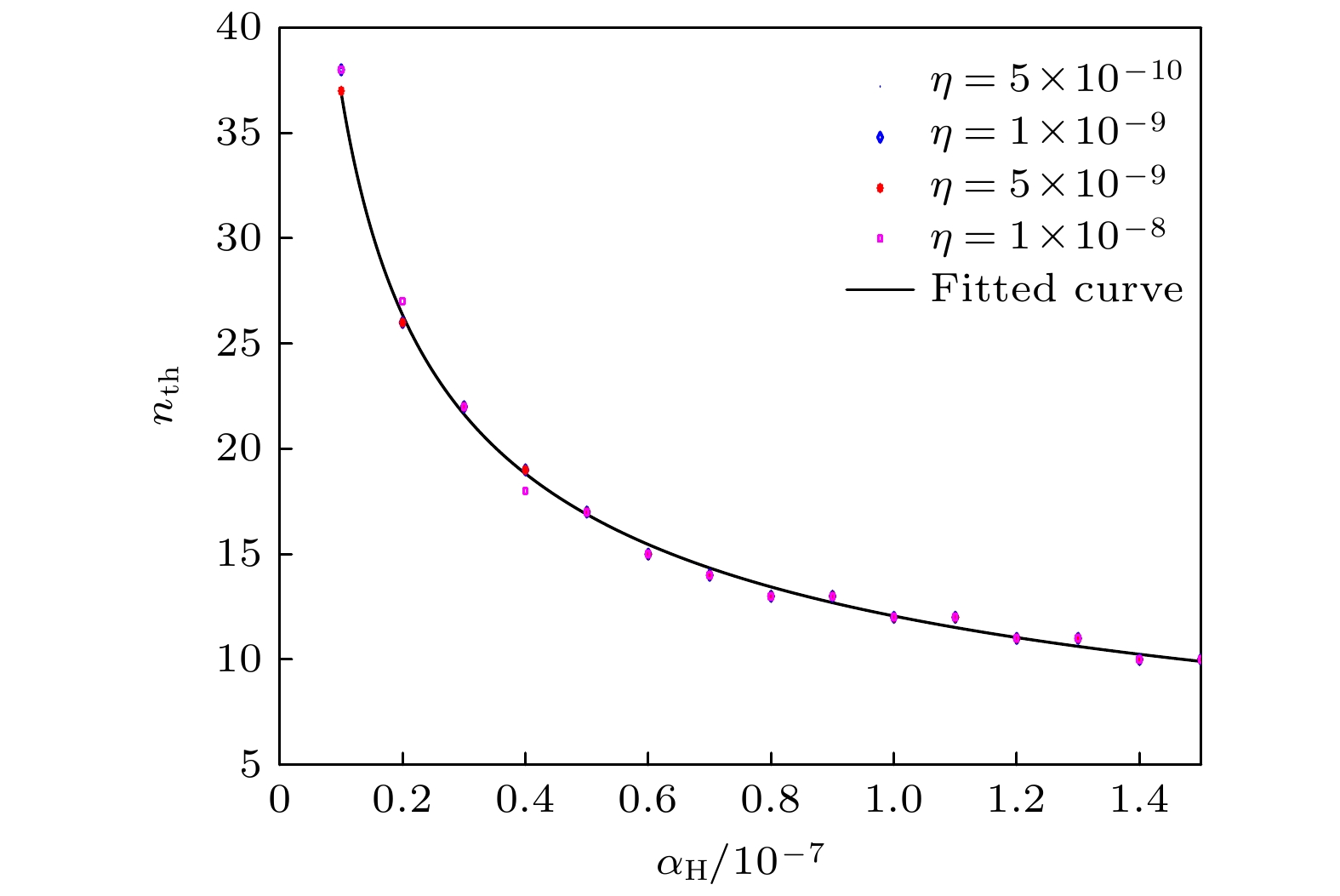
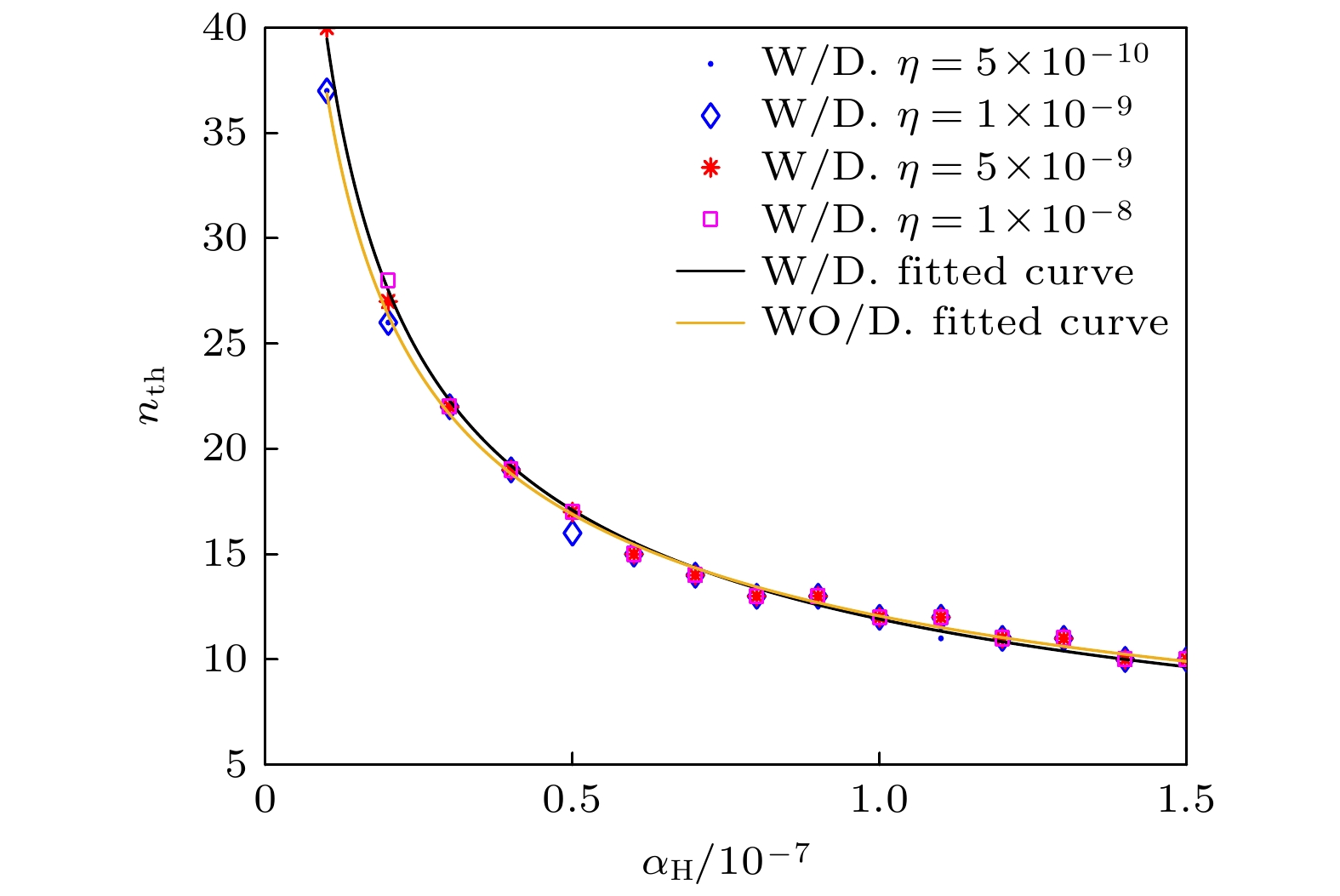
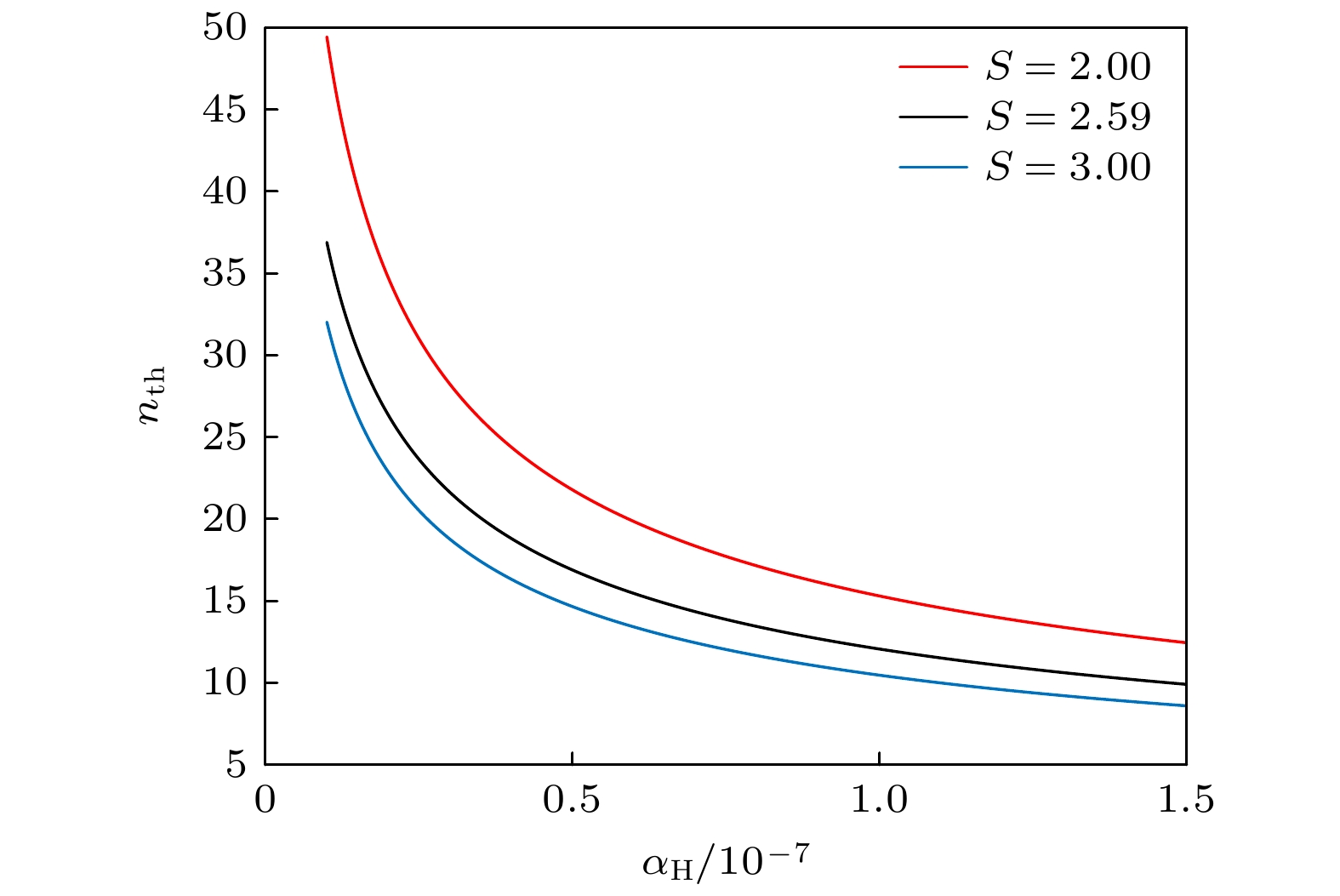
$ {\eta }_{{\rm{H}}}={10}^{-16} $ .
图 5 不同
$ {\alpha }_{{\rm{H}}} $ 条件下, 气球模线性增长率随环向模数的变化情况, 其中电阻$ \eta ={10}^{-8} $ 保持不变Figure 5. The linear growth rate of the ballooning mode varies with the toroidal mode number under different ratio of the hyper-resistivity to the resistivity, where the value of resistivity is a constant.
图 6 不同电阻条件下, 超电阻对气球模线性增长率起作用的环向模数阈值与超电阻和电阻比值之间的关系, 横坐标为超电阻与电阻的比值
$ {\alpha }_{{\rm{H}}} $ , 纵坐标为环向模数阈值$ {n}_{{\rm{t}}{\rm{h}}} $ Figure 6. The threshold value of toroidal mode number varies with the ratio of hyper-resistivity to resistivity when the hyper-resistivity plays a role in the linear growth rate of the ballooning mode by changing the resistivity values. The x-coordinate is the ratio of the hyper-resistivity to the resistivity, and the y-coordinate is the threshold value of toroidal mode number.
图 7 考虑抗磁效应条件下, 超电阻对气球模线性增长率的影响
Figure 7. Effect of hyper-resistivity on the linear growth rate of ideal ballooning modes with diamagnetic effect.
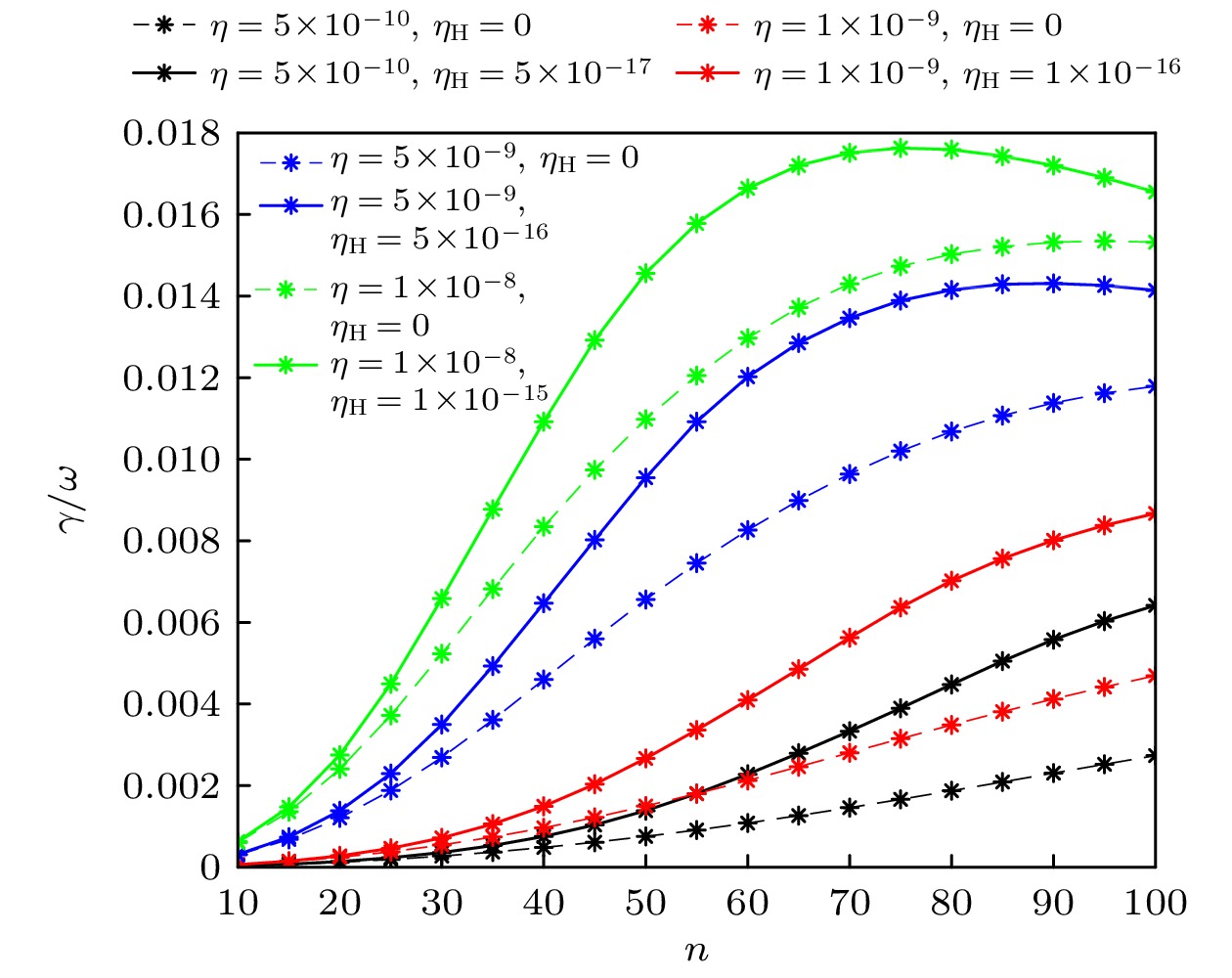
图 8 同时考虑抗磁效应、电阻和超电阻条件下, 气球模线性增长率随环向模数的变化, 其中
$ {\alpha }_{{\rm{H}}}={10}^{-7} $ 保持不变Figure 8. With diamagnetic effect, the linear growth rate of ballooning modes varies with toroidal mode number under different resistivity and hyper-resistivity, keeping the ratio of hyper-resistivity to resistivity unchanged, where
${\alpha }_{{\rm{H}}}= $ $ {10}^{-7}$ .
图 9 同时考虑抗磁、电阻和超电阻效应条件下, 气球模线性增长率随环向模数的变化, 其中超电阻大小(
${\eta }_{{\rm{H}}}= $ $ {10}^{-16}$ )保持不变Figure 9. With diamagnetic effect, the linear growth rate of the ballooning mode varies with the toroidal mode number under different resistivity conditions, keeping the values of hyper-resistivity unchanged, where
$ {\eta }_{{\rm{H}}}={10}^{-16} $ .
图 10 考虑抗磁效应时, 环向模数阈值随超电阻与电阻比值的变化
Figure 10. With diamagnetic effect, the threshold value of toroidal mode number varies with the ratio of hyper-resistivity to resistivity.
图 11 气球模线性增长率随磁剪切的变化关系(取参数
$\alpha =0.46,\; n=50, \;\eta ={10}^{-8},\; {\eta }_{{\rm{H}}}={1\times 10}^{-15}$ )Figure 11. Linear growth rate of ballooning modes varies with the growth of magnetic shear, with
$\alpha =0.46,\; n=50, $ $ \; \eta ={10}^{-8}, \;{\eta }_{{\rm{H}}}={1\times 10}^{-15}$ .
图 12 不同磁剪切条件下, 环向模数阈值随超电阻与电阻比值的变化关系
Figure 12. With different magnetic shear, the threshold value of toroidal mode number varies with the ratio of hyper-resistivity to resistivity.
-
[1] La Haye R J 2006 Phys. Plasmas 13 055501
Google Scholar
[2] Connor J W, Hastie R J, Taylor J B 1978 Phys. Rev. Lett. 40 396
Google Scholar
[3] Glenn Bateman, Nelson D B 1978 Phys. Rev. Lett. 41 1804
Google Scholar
[4] Strauss H R 1981 Phys. Fluids 24 2004
Google Scholar
[5] Dark J F, Antonsen Jr T M 1985 Phys. Fluids 28 544
Google Scholar
[6] Lortz D, Nuhrenberg J 1978 Phys. Lett. A 68 49
Google Scholar
[7] Coppi B, Ferreira A, Ramos J 1980 Phys. Rev. Lett. 44 990
Google Scholar
[8] Strauss H R, Park W, Monticello D A, White R B 1980 Nucl. Fusion 20 638
[9] Snyder P B, Wilson H R, Ferron J R, Lao L L, Leonard A W, Osborne T H, Turnbull A D, Mossessian D, Murakami M, Xu X Q 2002 Phys. Plasmas 9 2037
Google Scholar
[10] Strauss H R 1986 Phys. Fluids 29 3668
Google Scholar
[11] Kaw P K, Valeo E J, Rutherford P H 1979 Phys. Rev. Lett. 43 1398
Google Scholar
[12] Wu N, Chen S Y, Mou M L, Tang C J 2018 Phys. Plasmas 25 092305
Google Scholar
[13] Connor J W, Hastie R J, Wilson H R 1998 Phys. Plasmas 5 2687
Google Scholar
[14] Xu X Q, Dudson B, Snyder P B, Umansky M V, Wilson H 2010 Phys. Rev. Lett. 105 175005
Google Scholar
[15] Rhee T, Park G Y, Jhang H, Kim S S, Singh R 2017 Phys. Plasmas 24 072504
Google Scholar
[16] Rhee T, Kim S S, Jhang H, Park G Y, Singh R 2015 Nucl. Fusion 55 032004
Google Scholar
[17] Jhang H, Kaang H H, Kim S S, Rhee T, Singh R, Hahm T S 2017 Nucl. Fusion 57 022006
Google Scholar
[18] Miller R L, Chu M S, Greene J M, Lin-Liu Y R, Waltz R E 1998 Phys. Plasmas 5 973
Google Scholar
[19] Mou M L, Jhang H, Rhee T, Chen S Y, Tang C J 2018 Phys. Plasmas 25 082518
Google Scholar
[20] Xia T Y, Xu X Q, Dudson B D, Li J 2012 Contrib. Plasma Phys. 52 353
Google Scholar
[21] Tang W M, Dewar R L, Manickam J 1982 Nucl. Fusion 22 1079
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 2736
- PDF Downloads: 60
- Cited By: 0
