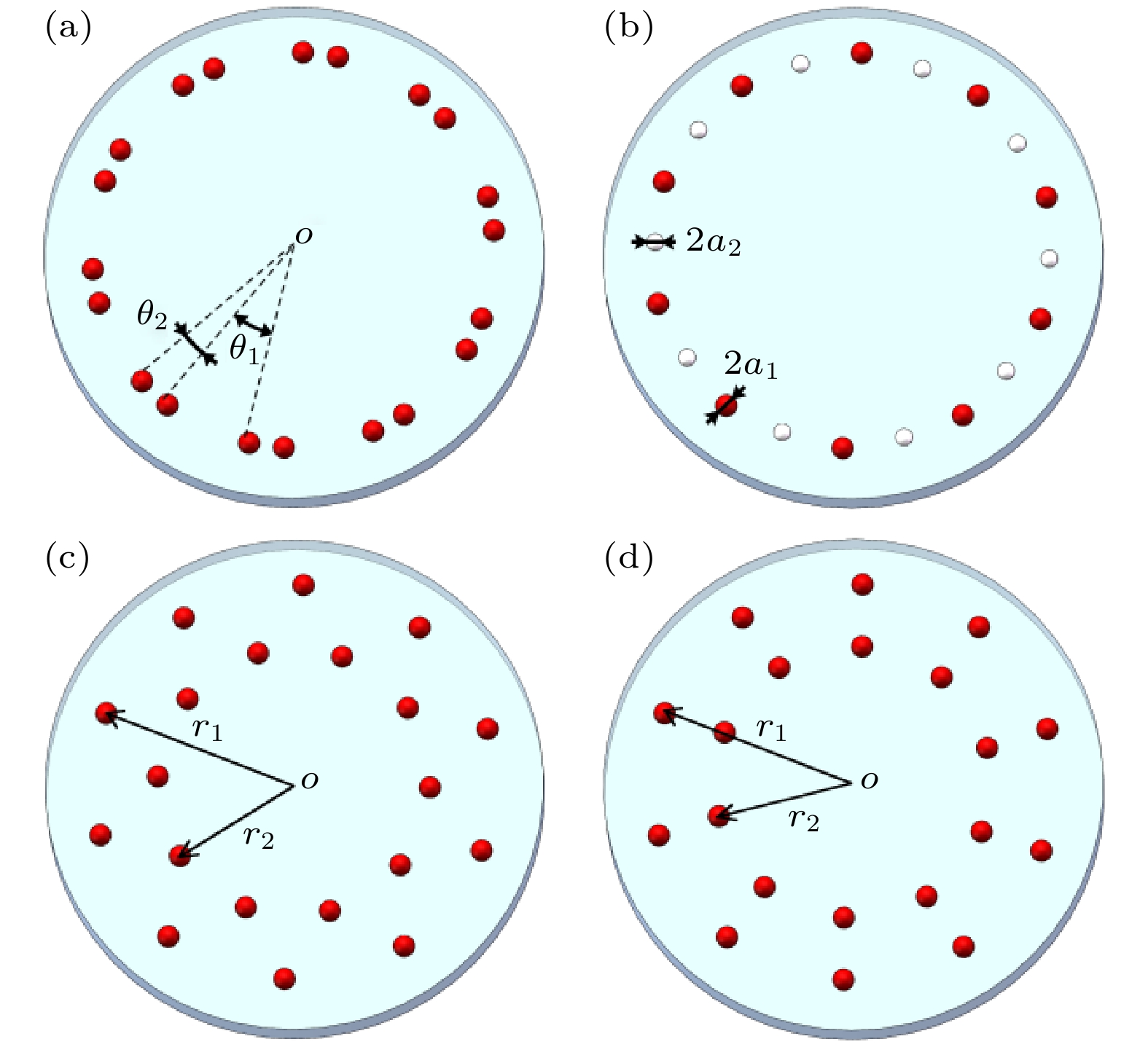
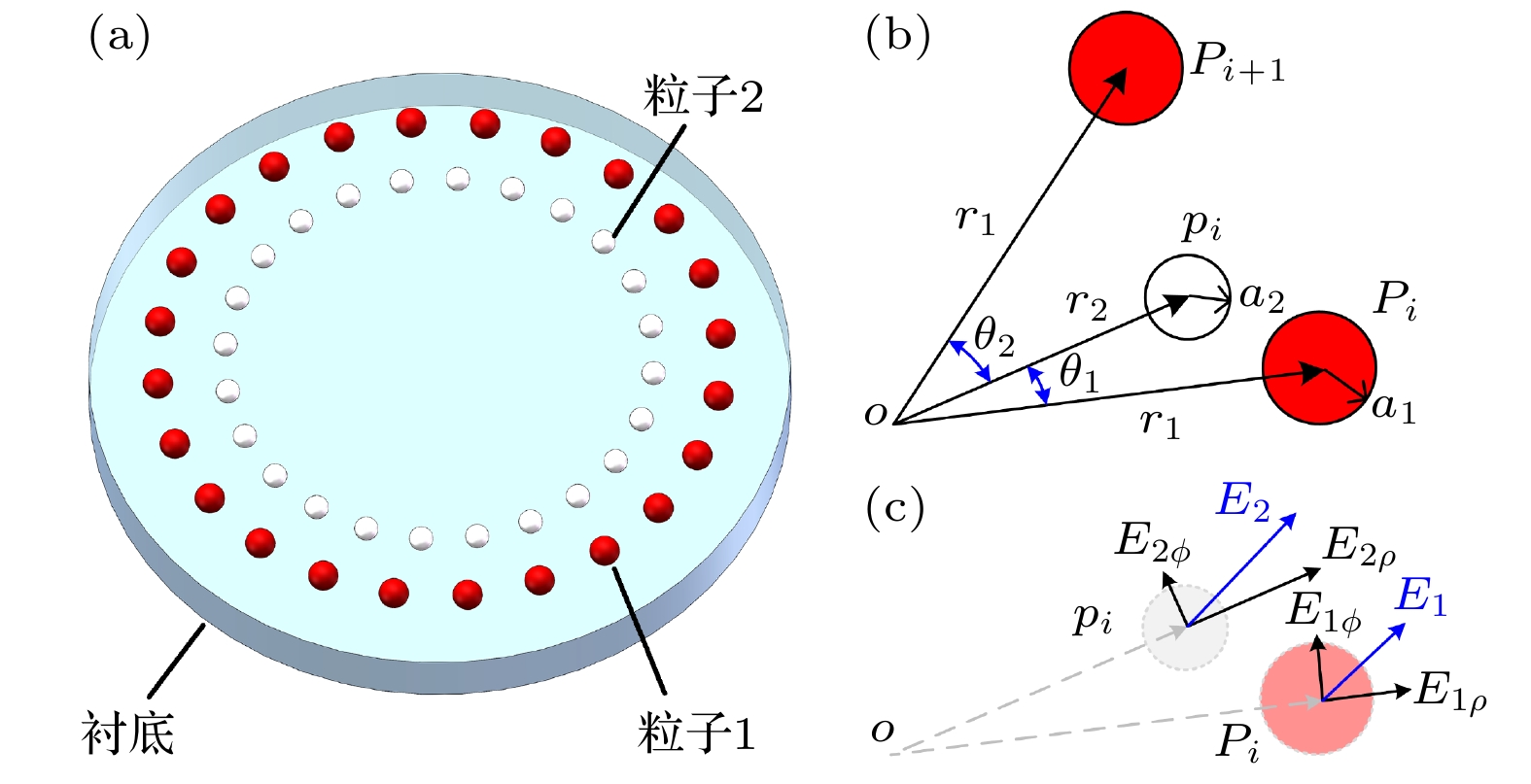
-
Surface lattice resonances due to regular periodic array of metallic nanoparticles can be attributed to the mutual coupling between the localized surface plasmon resonances of different nanoparticles. A comparison of resonant effect between the single particle and the array shows that the resonance line width can be significantly reduced. In this paper, we extend the coupled dipole approximation to solving the electromagnetic characteristics of the particle ring structures with rotational symmetry, and propose an analytical model for the double ring array of metallic nano-particles. Furthermore, we derive the general resonant condition of the double ring array and investigate some concrete cases in detail. It shows that the full resonance of the whole array depends crucially on the structural parameters, whose enhancement factor can be extremely high. But a slight change in the structural parameter willlead the enhancement factor to decrease sharply. We also find that the radiation field of the full resonance effect will be independent of the external field, which provides us a simple approach to producing a localized optical field with complex space distribution. This proposed structure can possess potential applications in various fields such as metasurface, optoelectronics, optical manipulation, communication, and biosensing.
-
Keywords:
- surface plasmon resonance /
- nanoarray /
- metal nano-particles
[1] Wang Q, Ren Z H, Zhao W M, Wang L, Yan X, Zhu A S, Qiu F M, Zhang K K 2022 Nanoscale 14 564
Google Scholar
[2] Philip A, Kumar A R 2022 Coord. Chem. Rev. 458 214424
Google Scholar
[3] Dong L, Zhang C Y, Yan L, Zhang B B, Chen H, Mi X H, Fu Z K, Zhang Z L, Zheng H R 2021 Chin. Phys. B 30 077301
Google Scholar
[4] Wu Y, Niu J, Danesh M, Liu J, Chen Y, Ke L, Qiu C, Yang H 2016 Appl. Phys. Lett. 109 041106
Google Scholar
[5] Hutter E, Fendler J H 2004 Adv. Mater. 16 1685
Google Scholar
[6] Kravets V G, Kabashin A V, Barnes W L, Grigorenko A N 2018 Chem. Rev. 118 5912
Google Scholar
[7] Wang F, Shen Y R 2006 Phys. Rev. Lett. 97 206806
Google Scholar
[8] Wang L, Wang Q, Wang T Q, Zhao W M, Yin X Y, Jiang J X, Zhang S S 2022 Nanoscale 14 6144
Google Scholar
[9] Huang X, Zhang B, Yu B, Zhang H, Shao G 2022 Nano Technol. 33 225206
[10] Hua Y, Fumani A K, Odom T W 2019 ACS Photonics 6 322
Google Scholar
[11] Kataja M, Hakala T, Julku A, Huttunen M, van Dijken S, Törmä P 2015 Nat. Commun. 6 7072
Google Scholar
[12] Bauer C, Kobiela G, Giessen H 2011 Phys. Rev. B 84 193104
Google Scholar
[13] Utikal T, Zentgraf T, Paul T, Rockstuhl C, Lederer F, Lippitz M, Giessen H 2011 Phys. Rev. Lett. 106 133901
Google Scholar
[14] Thackray B D, Thomas P A, Auton G H, Rodriguez F J, Marshall O P, Kravets V G, Grigorenko A N 2015 Nano Lett. 15 3519
Google Scholar
[15] 殷澄, 许田, 陈秉岩, 韩庆邦 2015 64 164202
Google Scholar
Yin C, Xu T, Chen B Y, Han Q B 2015 Acta Phys. Sin. 64 164202
Google Scholar
[16] 殷澄, 陆成杰, 笪婧, 张瑞耕, 阚雪芬, 韩庆邦, 许田 2021 70 024201
Google Scholar
Yin C, Lu C J, Da J, Zhang R G, Kan X F, Han Q B, Xu T 2021 Acta Phys. Sin. 70 024201
Google Scholar
[17] Alexander M 2009 JOSA B 26 517
Google Scholar
[18] Humphrey A D, Barnes W L 2014 Phys. Rev. B 90 075404
Google Scholar
[19] Cai W, Shalaev V 2010 Optical Metamaterials (New York: Springer) p22
[20] Martin S 2017 Chiral Nanophotonics (Switzerland: Springer) p43
-
图 1 (a) 金属纳米粒子的双圆环阵列示意图; (b) 阵列结构参数图; (c) 激发场参数图
Figure 1. (a) Schematic diagram of the double ring array of metallic nanoparticles; (b) structure diagram and the parameter definitions; (c) definition of the excitation field.
图 2 4种特殊的双圆环金属纳米粒子阵列结构
Figure 2. Four special arrangement of the double ring array of metallic particles.
图 3 (a) 由单圆环粒子阵列构造双圆环粒子阵列; (b) 双圆环阵列的归一化增强因子随结构参数
$ {{\Delta \theta } \mathord{\left/ {\vphantom {{\Delta \theta } \theta }} \right. } \theta } $ 和$ {{\Delta r} \mathord{\left/ {\vphantom {{\Delta r} r}} \right. } r} $ 的变化, 图中展现出两种共振结构(用红色虚线标出)随着$ {{\Delta \theta } \mathord{\left/ {\vphantom {{\Delta \theta } \theta }} \right. } \theta } $ 变化而逐渐简并的情形. 在仿真中, 金属纳米粒子使用的是半径为40 nm的银球, 激发波长为650 nm, 其他参数为N = 12和r = 200 nmFigure 3. (a) Transform to double ring structure from single ring array by varying two parameters
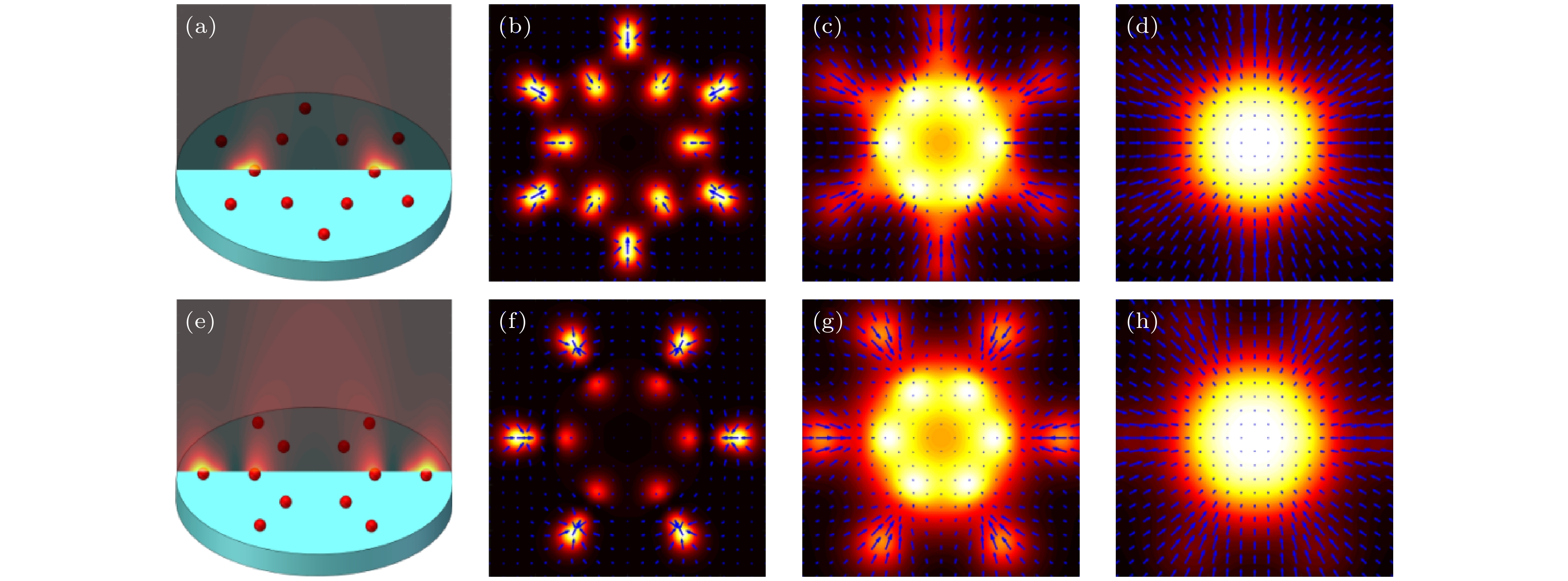
$ \Delta \theta /\theta $ and$ {{\Delta r} \mathord{\left/ {\vphantom {{\Delta r} r}} \right. } r} $ ; (b) normalized enhancement factor ($\beta $ ) as a function of the two parameters. Two resonant structures are displayed via red dashed arrows, which gradually degenerate as the$ \Delta \theta /\theta $ turns large. In the calculation, the metallic particles are silver sphere of 40 nm in radius, and the incident wavelength is 650 nm, other parameters are N = 12 and r = 200 nm.
图 6 两种双圆环粒子阵列结构的局域场分布 (a), (c) xz平面上的场分布, 最大传输距离为150 nm; (b), (f) 距离阵列40 nm处xy平面上的场分布; (c), (g) 距离阵列80 nm处xy平面上的场分布; (d), (h) 距离阵列120 nm处xy平面上的场分布. 仿真区域的边长为600 nm, 蓝色箭头标识了横向能流的方向
Figure 6. Radiation field of two double ring array: (a), (c) Field distribution on the xz plane, where the largest propagation distance is 150 nm; (b), (f) field distribution plot the transverse distribution on the xy plane of 40 nm away from the array; (c), (g) field distribution on the xy plane at 80 nm from the array; (d), (h) field distribution on the xy plane at 120 nm distance from the array. The simulation area is a square of 600 nm wide, and the blue arrows show the transversal energy flux.
图 7 双圆环粒子阵列的共振效应 (a) 完全共振结构与作为对比的部分共振结构参数; (b) 完全共振结构的增强因子; (c) 在不同激发外场下, 两种共振结构在不同传输距离处的局域场分布与横向能流分布
Figure 7. Resonant characteristics of the double ring particle array: (a) Structural parameters of the full resonant and partial resonant array; (b) enhancement factor of the full resonant structure; (c) radiation field of the two structures at different propagation distance under different excitation.
-
[1] Wang Q, Ren Z H, Zhao W M, Wang L, Yan X, Zhu A S, Qiu F M, Zhang K K 2022 Nanoscale 14 564
Google Scholar
[2] Philip A, Kumar A R 2022 Coord. Chem. Rev. 458 214424
Google Scholar
[3] Dong L, Zhang C Y, Yan L, Zhang B B, Chen H, Mi X H, Fu Z K, Zhang Z L, Zheng H R 2021 Chin. Phys. B 30 077301
Google Scholar
[4] Wu Y, Niu J, Danesh M, Liu J, Chen Y, Ke L, Qiu C, Yang H 2016 Appl. Phys. Lett. 109 041106
Google Scholar
[5] Hutter E, Fendler J H 2004 Adv. Mater. 16 1685
Google Scholar
[6] Kravets V G, Kabashin A V, Barnes W L, Grigorenko A N 2018 Chem. Rev. 118 5912
Google Scholar
[7] Wang F, Shen Y R 2006 Phys. Rev. Lett. 97 206806
Google Scholar
[8] Wang L, Wang Q, Wang T Q, Zhao W M, Yin X Y, Jiang J X, Zhang S S 2022 Nanoscale 14 6144
Google Scholar
[9] Huang X, Zhang B, Yu B, Zhang H, Shao G 2022 Nano Technol. 33 225206
[10] Hua Y, Fumani A K, Odom T W 2019 ACS Photonics 6 322
Google Scholar
[11] Kataja M, Hakala T, Julku A, Huttunen M, van Dijken S, Törmä P 2015 Nat. Commun. 6 7072
Google Scholar
[12] Bauer C, Kobiela G, Giessen H 2011 Phys. Rev. B 84 193104
Google Scholar
[13] Utikal T, Zentgraf T, Paul T, Rockstuhl C, Lederer F, Lippitz M, Giessen H 2011 Phys. Rev. Lett. 106 133901
Google Scholar
[14] Thackray B D, Thomas P A, Auton G H, Rodriguez F J, Marshall O P, Kravets V G, Grigorenko A N 2015 Nano Lett. 15 3519
Google Scholar
[15] 殷澄, 许田, 陈秉岩, 韩庆邦 2015 64 164202
Google Scholar
Yin C, Xu T, Chen B Y, Han Q B 2015 Acta Phys. Sin. 64 164202
Google Scholar
[16] 殷澄, 陆成杰, 笪婧, 张瑞耕, 阚雪芬, 韩庆邦, 许田 2021 70 024201
Google Scholar
Yin C, Lu C J, Da J, Zhang R G, Kan X F, Han Q B, Xu T 2021 Acta Phys. Sin. 70 024201
Google Scholar
[17] Alexander M 2009 JOSA B 26 517
Google Scholar
[18] Humphrey A D, Barnes W L 2014 Phys. Rev. B 90 075404
Google Scholar
[19] Cai W, Shalaev V 2010 Optical Metamaterials (New York: Springer) p22
[20] Martin S 2017 Chiral Nanophotonics (Switzerland: Springer) p43
DownLoad:
Catalog
Metrics
- Abstract views: 3712
- PDF Downloads: 106
- Cited By: 0
