On theoretical calculation method for two-dimensional planar shock wave refractions

Jia Lei-Ming; Wang Zhi-Huan; Wang Shu-Fei; Zhong Wei; Tian Zhou

doi:10.7498/aps.72.20222042

Abstract

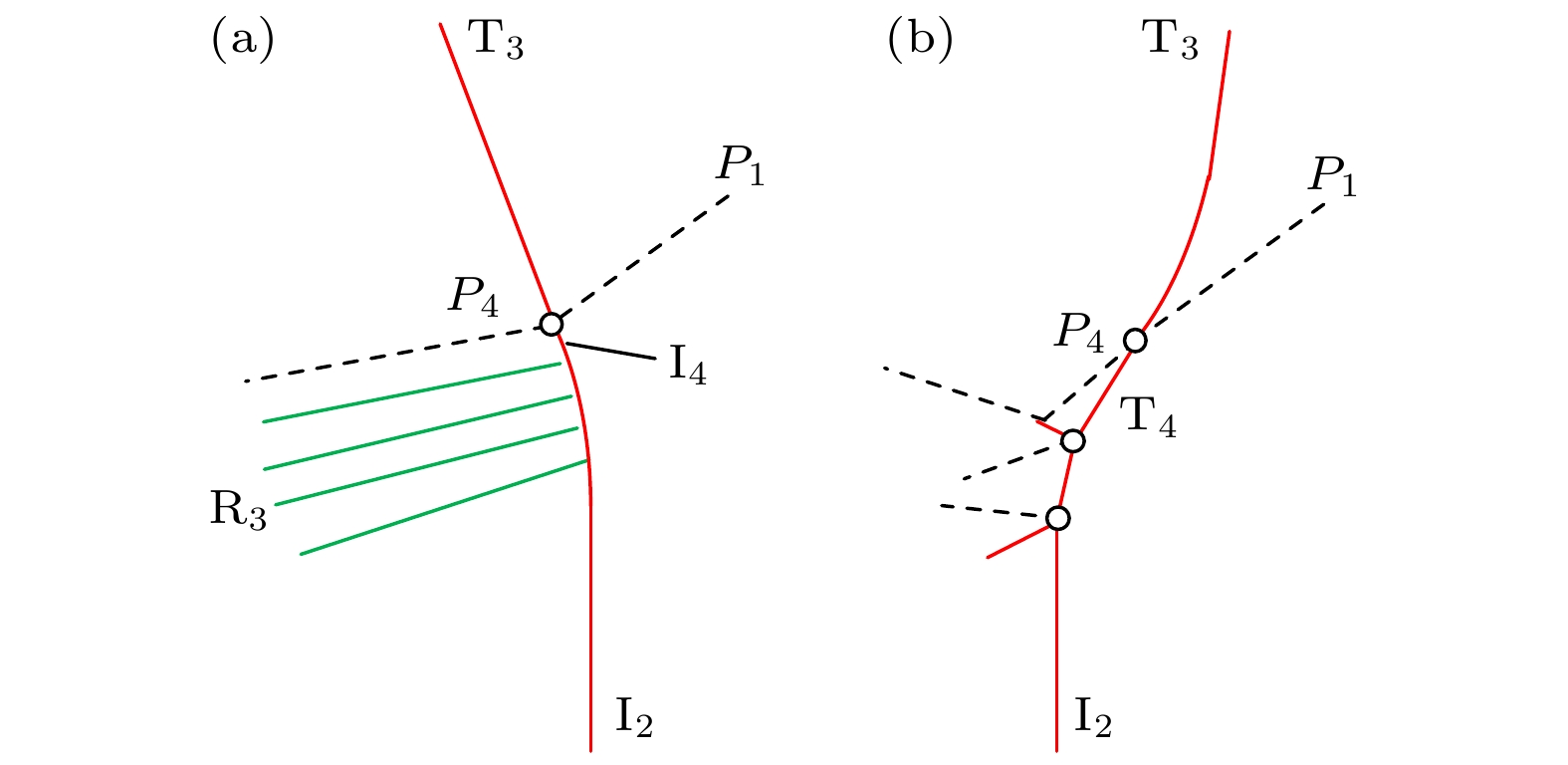
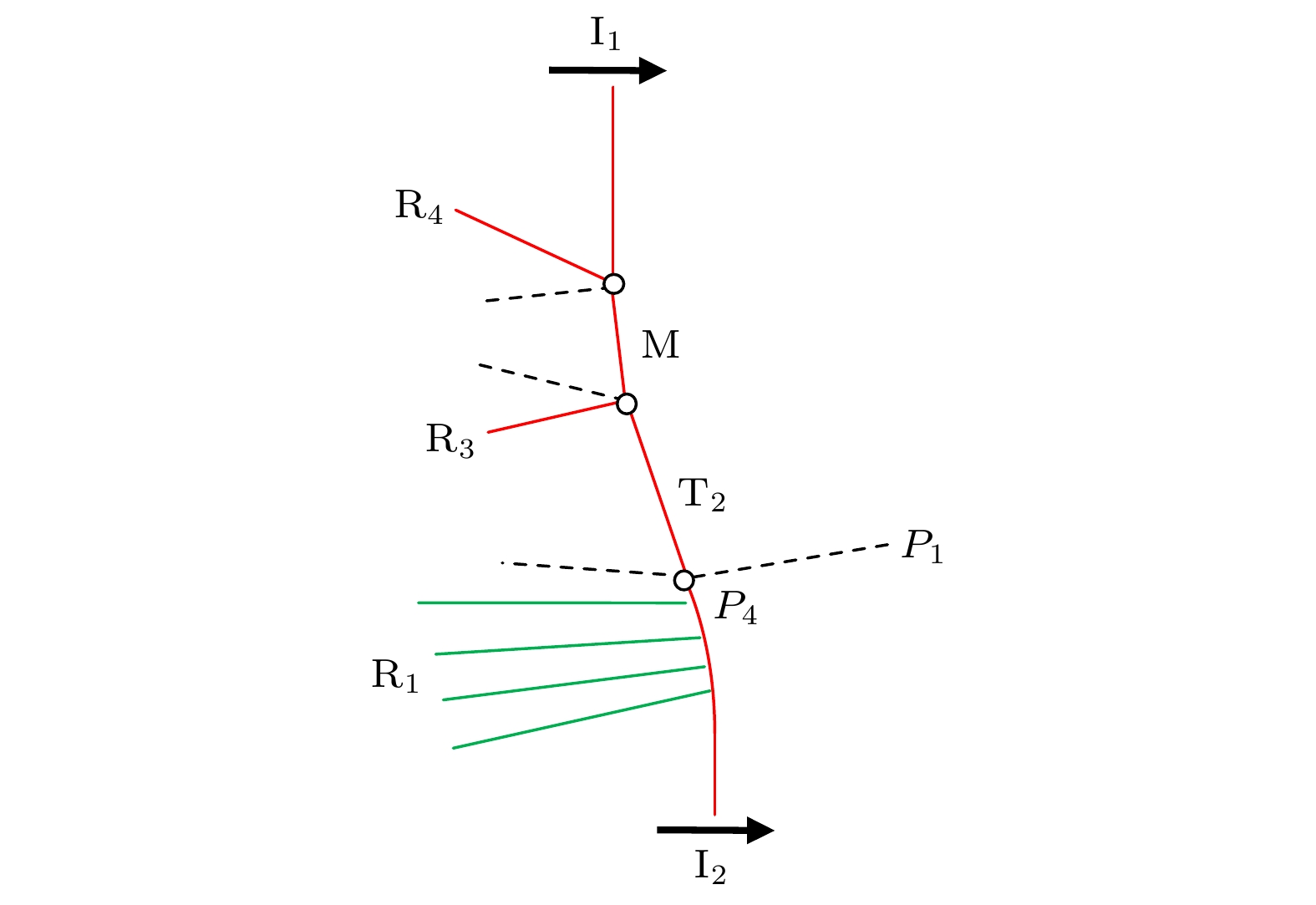
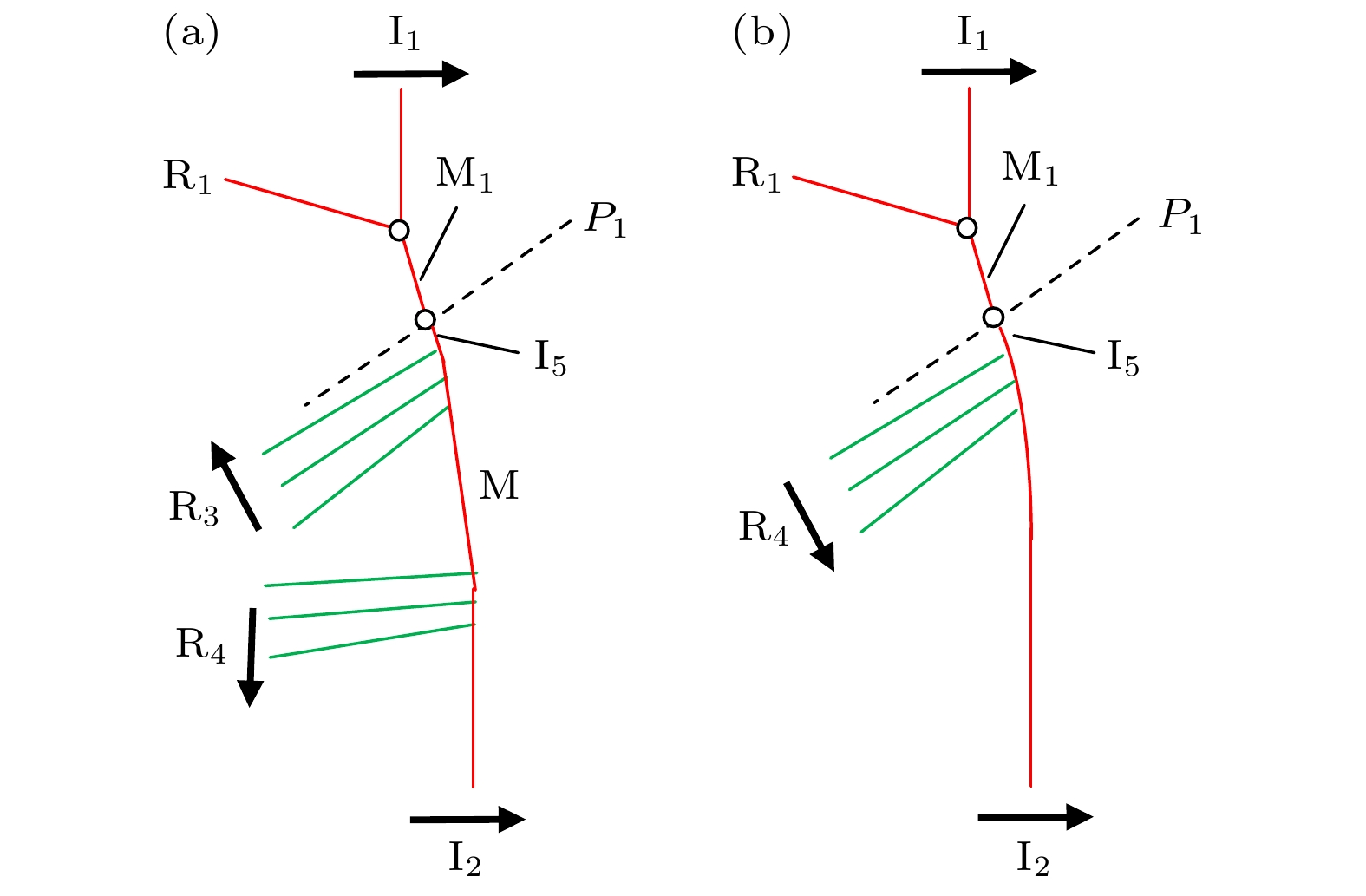
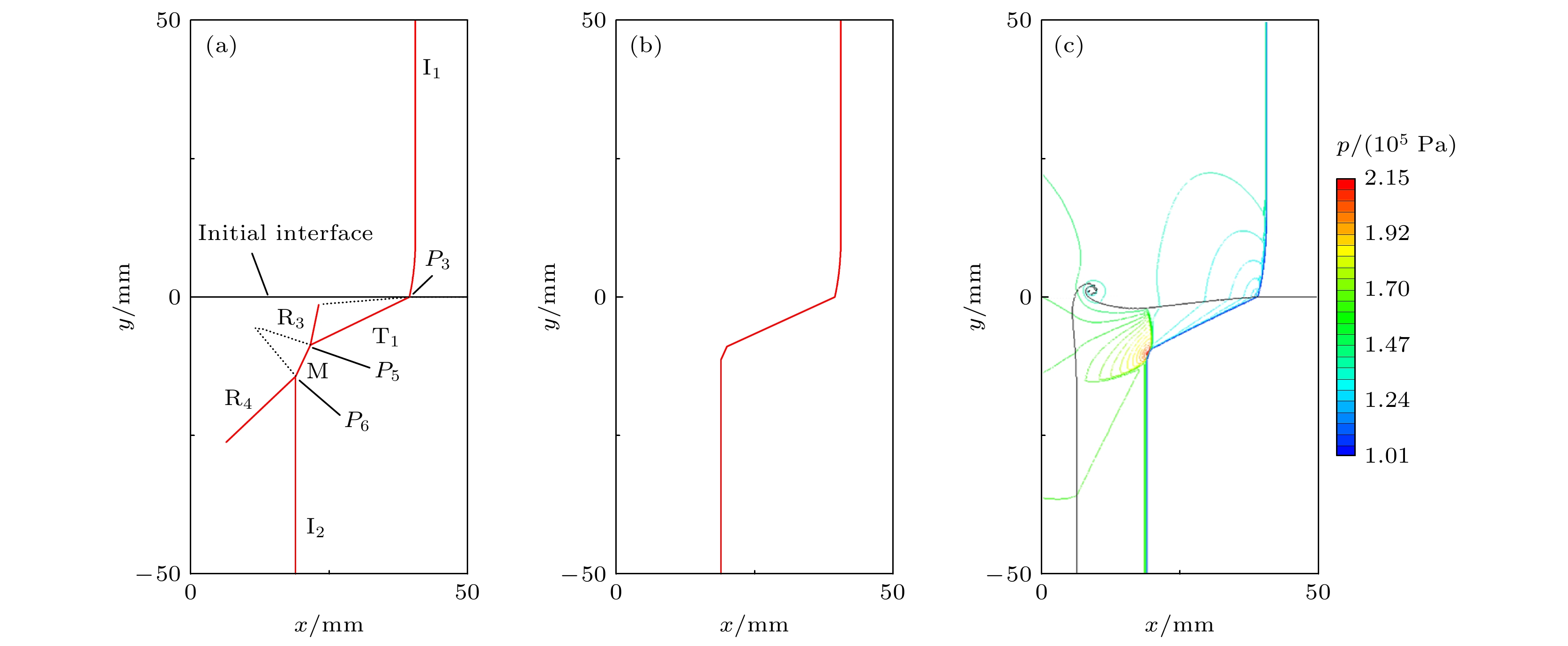
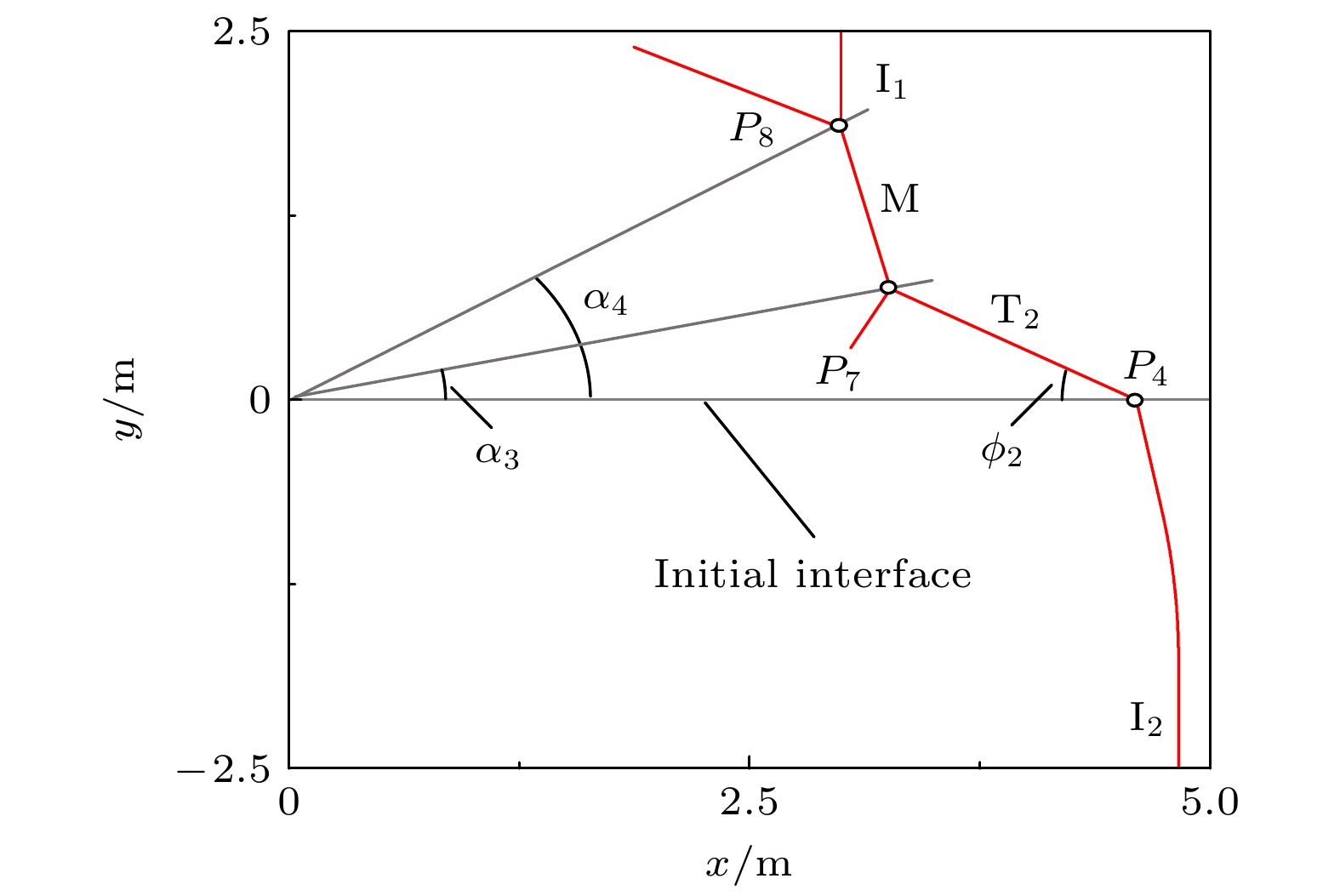
A theoretical calculation method for wave structures in the flow resulting from the interaction between the two-dimensional planar shock and the material interface is developed. First, the propagation of the shock wave on either side of the interface is analyzed, and two regular refraction types and three irregular ones are identified. Then, according to the relative speed of the perturbations on either side, three different interaction cases are established. Compared with the existing Catherasoo’s method, this method is improved in the following aspects: 1) the influence of the perturbation in the post-shock flow field on the interaction is taken into account, including its type and whether it can catch up and interact with the shock front; 2) the interactions between different waves are calculated mostly based on the exact solutions of the Euler equations, except those involving post-shock subsonic rarefaction waves. This method has been used to investigate the interaction of a Mach number 1.17 shock with an air/SF₆ interface, and give wave structures that accord with numerical results and existing experimental data. The angle between the transmitted shock and the horizontal direction is obtained to be in better agreement with experimental data than Catherasoo’s result, and more parameters are obtained, such as the reflected wave and the interface deflection angle. For cases involving a Mach number 2.00 shock with different material density ratios and interface inclination angles, comparisons between theoretical and numerical results show that our method can obtain the type of wave structure more accurately than Catherasoo’s method, and identify a refraction type in which the post-shock strong perturbation catches up with the shock front and a three-wave structure is formed, whereas Catherasoo’s method cannot handle this case. Thus, the results show that the improved method in this work has better applicability and higher accuracy than the existing method in identifying the type of wave structure, and can also provide more information about the wave structures.

Keywords:

Authors and contacts

1.
Northwest Institute of Nuclear Technology, Xi’an 710024, China
2.
Department of Engineering Physics, Tsinghua University, Beijing 100084, China

Corresponding author: Jia Lei-Ming, jialeiming@nint.ac.cn

FullText HTML : translate this paragraph

References

[1]	Taub A H 1947 Phys. Rev. 72 51 Google Scholar
[2]	Polachek H, Seeger R J 1951 Phys. Rev. 84 922 Google Scholar
[3]	Henderson L F 1966 J. Fluid Mech. 26 607 Google Scholar
[4]	Ben-Dor G, Igra O, Elperin T 2001 Handbook of Shock Waves (Vol. 2) (London: Academic Press) pp71,72
[5]	Jahn R G 1956 J. Fluid Mech. 1 457 Google Scholar
[6]	Abd-El-Fattah A M, Henderson L F, Lozzi A 1976 J. Fluid Mech. 76 157 Google Scholar
[7]	Flores J, Holt M 1982 Phys. Fluids 25 238 Google Scholar
[8]	Henderson L F, Jia-Huan M, Akira S, Kazuyoshi T 1990 Fluid Dyn. Res. 5 337 Google Scholar
[9]	Henderson L F 1992 Shock Waves 2 103 Google Scholar
[10]	Abd-El-Fattah A M, Henderson L F 1978 J. Fluid Mech. 86 15 Google Scholar
[11]	Abd-El-Fattah A M, Henderson L F 1978 J. Fluid Mech. 89 79 Google Scholar
[12]	Henderson L F 1989 J. Fluid Mech. 198 365 Google Scholar
[13]	Henderson L F, Colella P, Puckett E G 1991 J. Fluid Mech. 224 1 Google Scholar
[14]	Henderson L F, Puckett E G 2014 Shock Waves 24 553 Google Scholar
[15]	Nourgaliev R R, Sushchikh S Y, Dinh T N, Theofanous T G 2005 Int. J. Multiphas. Flow 31 969 Google Scholar
[16]	Zhai Z G, Wang M H, Si T, Luo X S 2014 J. Fluid Mech. 757 800 Google Scholar
[17]	Wang M H, Si T, Luo X S 2015 Shock Waves 25 347 Google Scholar
[18]	Luo X S, Wang M H, Si T, Zhai Z G 2015 J. Fluid Mech. 773 366 Google Scholar
[19]	Zhai Z G, Li W, Si T, Luo X S, Yang J M, Lu X Y 2017 Phys. Fluids 29 016102 Google Scholar
[20]	Igra D, Igra O 2018 Phys. Fluids 30 056104 Google Scholar
[21]	Sadin D V, Davidchuk V A 2020 J. Eng. Phys. Thermophys. 93 474 Google Scholar
[22]	Onwuegbu S, Yang Z 2022 AIP Adv. 12 025215 Google Scholar
[23]	Georgievskii P, Levin V, Sutyrin O 2010 Fluid Dyn. 45 281 Google Scholar
[24]	Georgievskiy P, Levin V, Sutyrin O 2012 Proceedings of the 15th International Symposium on Flow Visualization Minsk, Belarus, June 25-28, 2012 p1
[25]	Catherasoo C J, Sturtevant B 1983 J. Fluid Mech. 127 539 Google Scholar
[26]	Whitham G B 1957 J. Fluid Mech. 2 145 Google Scholar
[27]	Whitham G B 1959 J. Fluid Mech. 5 369 Google Scholar
[28]	Schwendeman D W 1988 J. Fluid Mech. 188 383 Google Scholar
[29]	Toro E F 2009 Riemann Solvers and Numerical Methods for Fluid Dynamics (3rd Ed.) (Berlin, Heidelberg: Springers-Verlag) pp115–151

Cited By

图 1 二维平面激波与物质界面作用示意图　(a) t < 0; (b) t > 0

Figure 1. Schematic diagram of the interaction of two-dimensional planar shock wave with material interface: (a) t < 0; (b) t > 0.

DownLoad: Full-Size Img PowerPoint

图 2 波I₁沿界面OP₁的折射类型　(a) RRS; (b) RRE; (c) IRS; (d) IRE; (e) IRP

Figure 2. Various refraction types of shock wave I₁ along the interface OP₁: (a) RRS; (b) RRE; (c) IRS; (d) IRE; (e) IRP

DownLoad: Full-Size Img PowerPoint

图 3 波I₁, R₁和M₁组成三波结构

Figure 3. Three wave structures of I₁, R₁ and M₁.

DownLoad: Full-Size Img PowerPoint

图 4 不同时刻波I₁与R₁作用

Figure 4. Interaction between I₁ and R₁ at different times.

DownLoad: Full-Size Img PowerPoint

图 5 波I₂沿界面OP₁的折射类型　(a) IRE; (b) IRP

Figure 5. Various refraction types of shock wave I₂ along the interface OP₁: (a) IRE; (b) IRP.

DownLoad: Full-Size Img PowerPoint

图 6 第一种情形下波I₁, I₂沿界面OP₁的相互作用

Figure 6. Interaction of I₁ and I₂ along the interface OP₁ under case 1.

DownLoad: Full-Size Img PowerPoint

图 7 第二种情形下波I₁, I₂沿界面OP₁的相互作用

Figure 7. Interaction of I₁ and I₂ along the interface OP₁ under case 2.

DownLoad: Full-Size Img PowerPoint

图 8 第三种情形下波I₁, I₂沿界面OP₁的相互作用

Figure 8. Interaction of I₁ and I₂ along the interface OP₁ under case 3.

DownLoad: Full-Size Img PowerPoint

图 9 t = 0.10 ms时刻波系结构　(a) 本文方法; (b) Catherasoo方法; (c) 数值结果

Figure 9. Wave structure at t = 0.10 ms: (a) Our own method; (b) Catherasoo’s method; (c) numerical results.

DownLoad: Full-Size Img PowerPoint

图 10 波系结构参量随时间变化　(a) 波系结构; (b) ϕ₁; (c) α₁; (d) α₂

Figure 10. Variation of wave structure parameters with time: (a) Wave structure; (b) ϕ₁; (c) α₁; (d) α₂.

DownLoad: Full-Size Img PowerPoint

DownLoad: Full-Size Img PowerPoint

图 12 不同φ值下的波系结构(Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25)　(a)—(i) 分别对应φ = 0°, 10°, ···, 80°

Figure 12. Wave structures for different φ (Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25). Panels (a)–(i) correspond to φ = 0°, 10°, ···, 80°, respectively.

DownLoad: Full-Size Img PowerPoint

图 13 波系结构变量示意图

Figure 13. Diagram for wave structure parameters.

DownLoad: Full-Size Img PowerPoint

图 14 t = 4.00 ms时流场压力分布数值结果(Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25)　(a) φ = 40°; (b) φ = 50°

Figure 14. Numerical results for field pressure at t = 4.00 ms (Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25): (a) φ = 40°; (b) φ = 50°.

DownLoad: Full-Size Img PowerPoint

图 15 偏差绝对值随φ的变化(Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25)　(a) ϕ₂, α₃和r₄; (b) α₄和d_7-8.

Figure 15. Variation of absolute value of deviations with φ (Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25): (a) ϕ₂, α₃ and r₄; (b) α₄ and d_7-8.

DownLoad: Full-Size Img PowerPoint

图 16 波系结构类型随φ的变化(Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25)

Figure 16. Variation of wave structure types with φ (Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25).

DownLoad: Full-Size Img PowerPoint

图 17 不同φ值下的波系结构(Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00)　(a)—(i) 分别对应φ = 0°, 10°, ···, 80°

Figure 17. Wave structures for different φ (Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00). Panels (a)–(i) correspond to φ = 0°, 10°, ···, 80°, respectively.

DownLoad: Full-Size Img PowerPoint

图 18 t = 6.00 ms时流场压力分布数值结果(Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00)　(a) φ = 20°; (b) φ = 30°

Figure 18. Numerical results for field pressure at t = 6.00 ms (Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00): (a) φ = 20°; (b) φ = 30°.

DownLoad: Full-Size Img PowerPoint

图 19 偏差绝对值随φ的变化(Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00)　(a) ϕ₁, α₁和d_5-6; (b) α₂和r₃

Figure 19. Variation of absolute value of deviations with φ (Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00): (a) ϕ₁, α₁ and d_5-6; (b) α₂ and r₃.

DownLoad: Full-Size Img PowerPoint

图 20 波系结构类型随φ的变化(Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00)

Figure 20. Variation of wave structure types with φ (Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00).

DownLoad: Full-Size Img PowerPoint

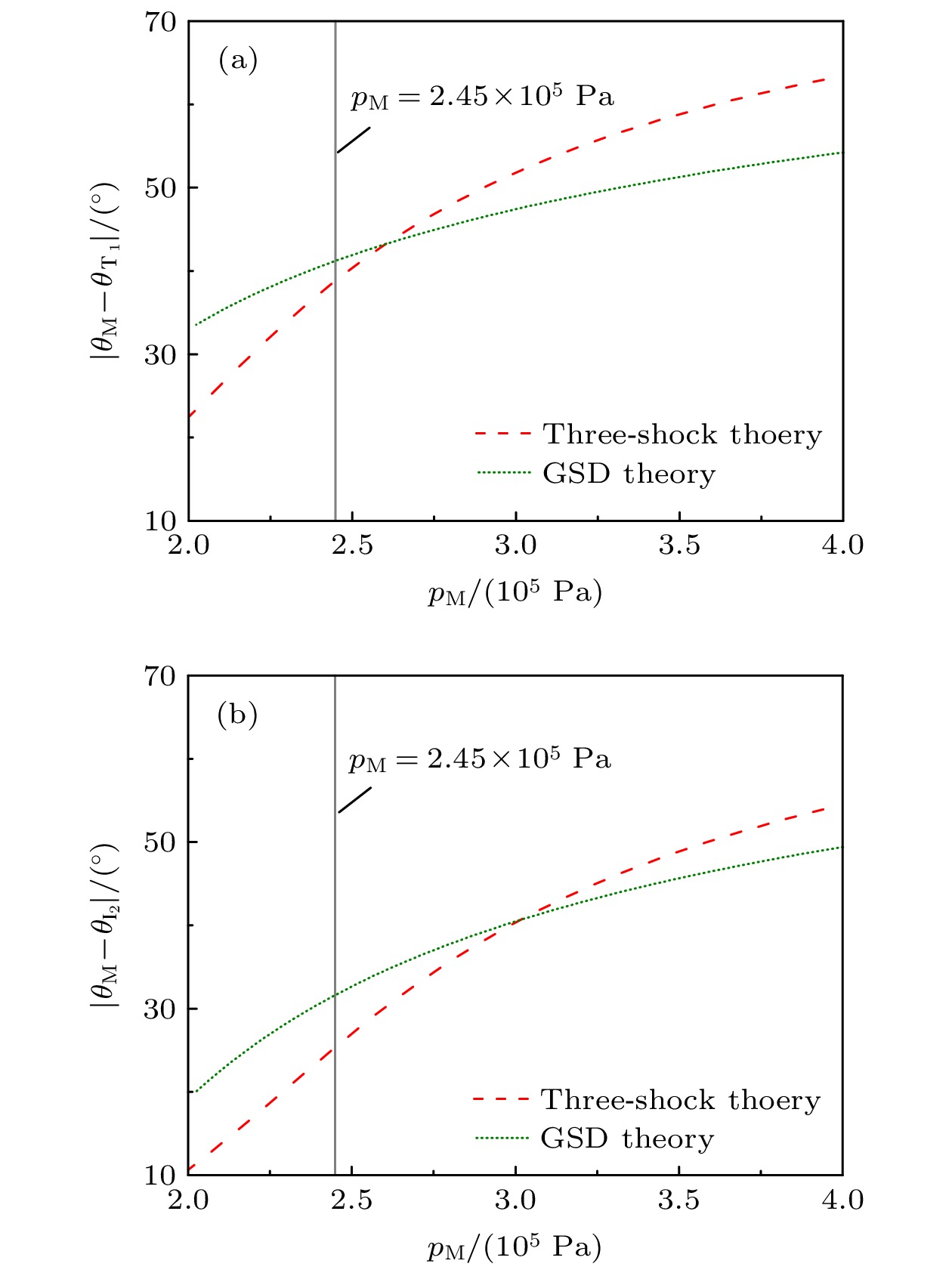
表 1 波T₁参数的理论计算结果

Table 1. Theoretical retuslts for parameters of T₁.

计算方法	p_T1/(10⁵ Pa)	u_T1/(m·s^–1)	v_T1/(m·s^–1)
本文	1.32	15.8	–32.6
Catherasoo	1.21	9.95	–21.6

DownLoad: CSV

表 2 波T₁与I₂作用的理论计算结果

Table 2. Theoretical retuslts for interaction between T₁ and I₂.

波T₁参数	计算方法	α₁/(°)	α₂/(°)	p_M/(10⁵ Pa)
p_T1 = 1.32×10⁵ Pa	本文	21.8	37.2	2.45
p_T1 = 1.32×10⁵ Pa	Catherasoo	24.9	32.0	2.23
p_T1 = 1.21×10⁵ Pa	本文	21.3	36.6	2.37
p_T1 = 1.21×10⁵ Pa	Catherasoo	24.1	30.8	2.17

DownLoad: CSV

表 3 不同φ值下波系结构变量(Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25)

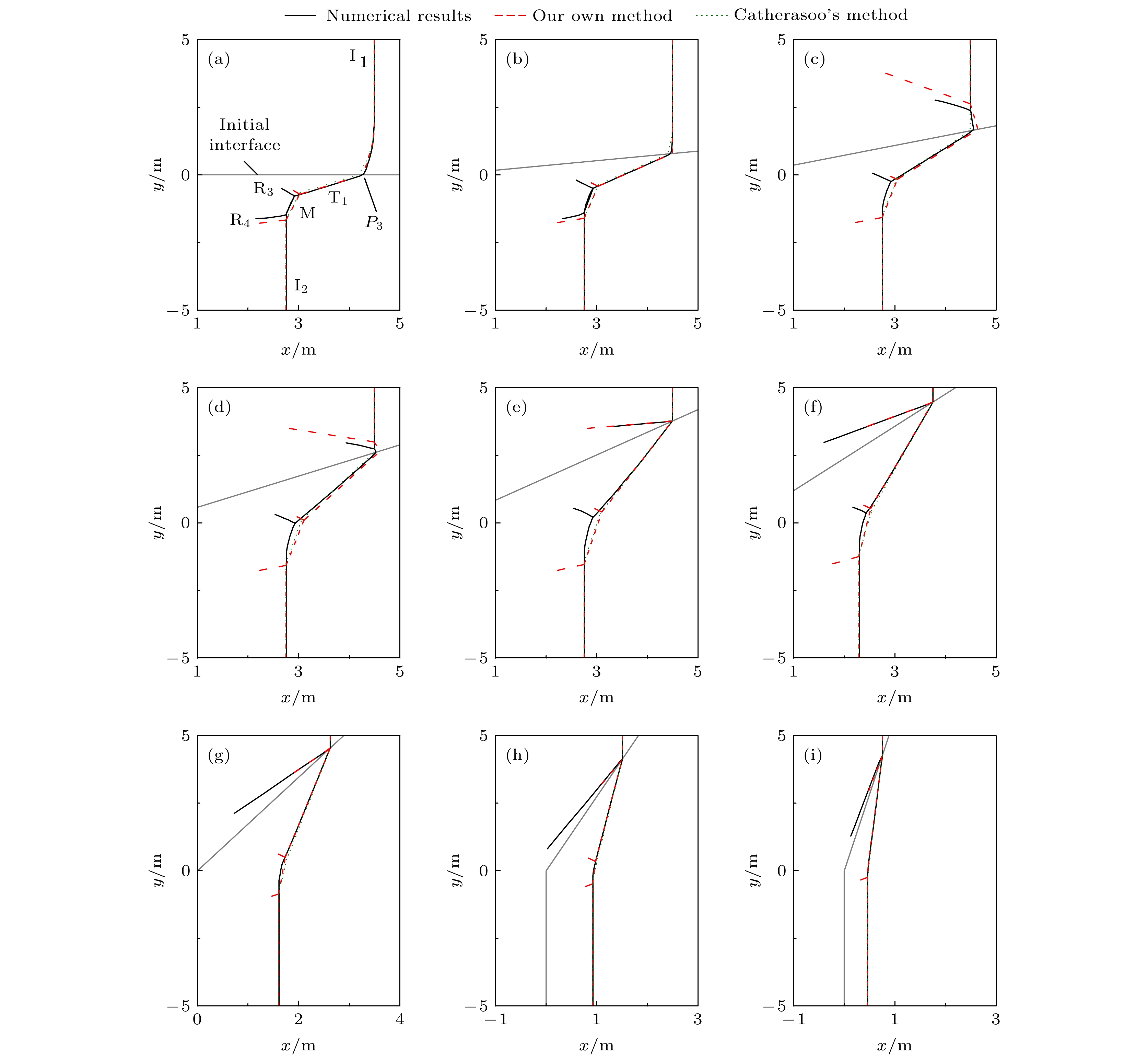
Table 3. Wave structure parameters for different φ (Ma_I1 = 2.00, ρ₂/ρ₁ = 0.25).

φ/(°)	计算方法	ϕ₂		α₃		α₄		d_7–8		r₄
φ/(°)	计算方法	结果/(°)	偏差/%	结果/(°)	偏差/%	结果/(°)	偏差/%	结果/m	偏差/%	结果/m	偏差/%
0	本文	29.4	–4.42	13.0	–10.1	31.7	10.6	1.13	34.2	4.60	0.86
0	Catherasoo	28.4	–7.89	12.2	–15.6	29.5	3.01	1.02	22.0	4.54	–0.31
10	本文	29.4	–4.62	8.97	–14.3	22.6	8.86	0.84	34.0	4.33	–0.51
10	Catherasoo	28.2	–8.54	7.89	–24.6	22.1	6.53	0.87	38.9	4.29	–1.33
20	本文	29.4	–5.12	5.33	–25.4	13.9	2.43	0.54	33.0	4.09	–3.34
20	Catherasoo	28.1	–9.28	3.97	–44.4	15.4	12.8	0.72	77.5	4.06	–3.89
30	本文	29.4	–9.64	2.01	–54.1	5.74	–27.5	0.24	2.59	3.88	–8.68
30	Catherasoo	28.1	–13.7	0.37	–91.7	9.12	15.0	0.59	148.7	3.86	–9.00
40	本文	—	—	—	—	4.17	25.9	—	—	4.16	–5.15
40	Catherasoo	—	—	—	—	5.02	51.4	—	—	4.22	–3.88
50	本文	—	—	—	—	0.82	22.7	—	—	4.74	–1.27
50	Catherasoo	—	—	—	—	1.99	197.9	—	—	4.86	1.22
60	本文	—	—	—	—	—	—	—	—	4.94	–0.06
60	Catherasoo	—	—	—	—	0.71	—	—	—	5.05	2.12
70	本文	—	—	—	—	—	—	—	—	4.60	–0.85
70	Catherasoo	—	—	—	—	—	—	—	—	4.60	–0.85
80	本文	—	—	—	—	—	—	—	—	4.31	–0.98
80	Catherasoo	—	—	—	—	—	—	—	—	4.31	–0.98

DownLoad: CSV

表 4 不同φ值下波系结构变量(Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00)

Table 4. Wave structure parameters for different φ (Ma_I1 = 2.00, ρ₂/ρ₁ = 4.00).

φ/(°)	计算方法	ϕ₁		α₁		α₂		d_5–6		r₃
φ/(°)	计算方法	结果/(°)	偏差/%	结果/(°)	偏差/%	结果/(°)	偏差/%	结果/m	偏差/%	结果/m	偏差/%
0	本文	29.6	–2.32	13.3	–11.4	31.2	11.3	0.99	40.4	4.27	0.83
0	Catherasoo	29.4	–3.02	12.0	–20.0	31.0	10.5	1.04	48.1	4.17	–1.61
10	本文	29.5	–2.64	17.8	–9.35	40.2	8.18	1.22	31.2	4.56	0.69
10	Catherasoo	29.8	–1.64	16.8	–14.2	39.6	6.55	1.23	32.5	4.46	–1.51
20	本文	29.4	–1.14	23.0	–6.63	49.8	—	1.44	—	4.94	1.78
20	Catherasoo	30.2	1.73	22.0	–10.9	48.8	—	1.44	—	4.78	–1.53
30	本文	29.5	3.20	28.0	–6.96	59.7	—	1.71	—	5.32	1.96
30	Catherasoo	29.9	4.53	27.4	–9.10	58.4	—	1.66	—	5.19	–0.68
40	本文	27.5	3.74	32.6	–9.07	69.2	—	1.97	—	5.86	0.14
40	Catherasoo	27.4	3.07	33.4	–6.81	67.9	—	1.84	—	5.86	0.14
50	本文	22.8	1.86	38.1	–8.40	78.5	—	1.79	—	5.82	–0.16
50	Catherasoo	23.3	4.49	39.9	–3.99	77.2	—	1.65	—	5.82	–0.16
60	本文	17.6	0.12	44.0	—	88.0	—	1.36	—	5.24	0.01
60	Catherasoo	18.3	3.71	46.8	—	86.5	—	1.22	—	5.24	0.01
70	本文	12.0	0.36	50.1	—	97.7	—	0.84	—	4.38	–0.45
70	Catherasoo	12.5	4.35	54.0	—	95.8	—	0.73	—	4.38	–0.45
80	本文	6.10	–0.64	56.4	—	107.5	—	0.45	—	4.31	–0.73
80	Catherasoo	6.35	3.47	60.9	—	105.0	—	0.39	—	4.31	–0.73

DownLoad: CSV

map

[1]	Taub A H 1947 Phys. Rev. 72 51 Google Scholar
[2]	Polachek H, Seeger R J 1951 Phys. Rev. 84 922 Google Scholar
[3]	Henderson L F 1966 J. Fluid Mech. 26 607 Google Scholar
[4]	Ben-Dor G, Igra O, Elperin T 2001 Handbook of Shock Waves (Vol. 2) (London: Academic Press) pp71,72
[5]	Jahn R G 1956 J. Fluid Mech. 1 457 Google Scholar
[6]	Abd-El-Fattah A M, Henderson L F, Lozzi A 1976 J. Fluid Mech. 76 157 Google Scholar
[7]	Flores J, Holt M 1982 Phys. Fluids 25 238 Google Scholar
[8]	Henderson L F, Jia-Huan M, Akira S, Kazuyoshi T 1990 Fluid Dyn. Res. 5 337 Google Scholar
[9]	Henderson L F 1992 Shock Waves 2 103 Google Scholar
[10]	Abd-El-Fattah A M, Henderson L F 1978 J. Fluid Mech. 86 15 Google Scholar
[11]	Abd-El-Fattah A M, Henderson L F 1978 J. Fluid Mech. 89 79 Google Scholar
[12]	Henderson L F 1989 J. Fluid Mech. 198 365 Google Scholar
[13]	Henderson L F, Colella P, Puckett E G 1991 J. Fluid Mech. 224 1 Google Scholar
[14]	Henderson L F, Puckett E G 2014 Shock Waves 24 553 Google Scholar
[15]	Nourgaliev R R, Sushchikh S Y, Dinh T N, Theofanous T G 2005 Int. J. Multiphas. Flow 31 969 Google Scholar
[16]	Zhai Z G, Wang M H, Si T, Luo X S 2014 J. Fluid Mech. 757 800 Google Scholar
[17]	Wang M H, Si T, Luo X S 2015 Shock Waves 25 347 Google Scholar
[18]	Luo X S, Wang M H, Si T, Zhai Z G 2015 J. Fluid Mech. 773 366 Google Scholar
[19]	Zhai Z G, Li W, Si T, Luo X S, Yang J M, Lu X Y 2017 Phys. Fluids 29 016102 Google Scholar
[20]	Igra D, Igra O 2018 Phys. Fluids 30 056104 Google Scholar
[21]	Sadin D V, Davidchuk V A 2020 J. Eng. Phys. Thermophys. 93 474 Google Scholar
[22]	Onwuegbu S, Yang Z 2022 AIP Adv. 12 025215 Google Scholar
[23]	Georgievskii P, Levin V, Sutyrin O 2010 Fluid Dyn. 45 281 Google Scholar
[24]	Georgievskiy P, Levin V, Sutyrin O 2012 Proceedings of the 15th International Symposium on Flow Visualization Minsk, Belarus, June 25-28, 2012 p1
[25]	Catherasoo C J, Sturtevant B 1983 J. Fluid Mech. 127 539 Google Scholar
[26]	Whitham G B 1957 J. Fluid Mech. 2 145 Google Scholar
[27]	Whitham G B 1959 J. Fluid Mech. 5 369 Google Scholar
[28]	Schwendeman D W 1988 J. Fluid Mech. 188 383 Google Scholar
[29]	Toro E F 2009 Riemann Solvers and Numerical Methods for Fluid Dynamics (3rd Ed.) (Berlin, Heidelberg: Springers-Verlag) pp115–151

[1]	Zhang Sheng-Bo, Zhang Huan-Hao, Zhang Jun, Mao Yong-Jian, Chen Zhi-Hua, Shi Qi-Chen, Zheng Chun. Magnetic field suppression characteristics in interaction process between shock wave and light gas cylinder. Acta Physica Sinica, 2024, 73(8): 084701. doi: 10.7498/aps.73.20231916
[2]	Zhang Sheng-Bo, Zhang Huan-Hao, Chen Zhi-Hua, Zheng Chun. Influence of different interface component distributions on Richtmyer-Meshkov instability. Acta Physica Sinica, 2023, 72(10): 105202. doi: 10.7498/aps.72.20222090
[3]	Sha Sha, Zhang Huan-Hao, Chen Zhi-Hua, Zheng Chun, Wu Wei-Tao, Shi Qi-Chen. Mechanism of longitudinal magnetic field suppressed Richtmyer-Meshkov instability. Acta Physica Sinica, 2020, 69(18): 184701. doi: 10.7498/aps.69.20200363
[4]	Peng Xu, Li Bin, Wang Shun-Yao, Rao Guo-Ning, Chen Wang-Hua. Gas-liquid two-phase flow of liquid film breaking process under shock wave. Acta Physica Sinica, 2020, 69(24): 244702. doi: 10.7498/aps.69.20201051
[5]	Chen Zhe, Wu Jiu-Hui, Chen Xin, Lei Hao, Hou Jie-Jie. Experimental study on screech tone mode switching of supersonic jet flowing through rectangular nozzles. Acta Physica Sinica, 2015, 64(5): 054703. doi: 10.7498/aps.64.054703
[6]	Sun Xiao-Yan, Zhu Jun-Fang. Study of the shock wave induced by closing partial road in traffic flow. Acta Physica Sinica, 2015, 64(11): 114502. doi: 10.7498/aps.64.114502
[7]	Yi Shi-He, Chen Zhi. Review of recent experimental studies of the shock train flow field in the isolator. Acta Physica Sinica, 2015, 64(19): 199401. doi: 10.7498/aps.64.199401
[8]	Chen Zhi, Yi Shi-He, Zhu Yang-Zhu, He Lin, Quan Peng-Cheng. Experimental study on of dynamics of particles in the flow filed with intensive gradients. Acta Physica Sinica, 2014, 63(18): 188301. doi: 10.7498/aps.63.188301
[9]	Sha Sha, Chen Zhi-Hua, Xue Da-Wen, Zhang Hui. Richtmyer-Meshkov instability induced by the interaction between shock wave and SF6 isosceles trapezoid cylinders. Acta Physica Sinica, 2014, 63(8): 085205. doi: 10.7498/aps.63.085205
[10]	Zhang Qiang, Chen Xin, He Li-Ming, Rong Kang. An experimental study of rectangular under-expanded supersonic jets collision. Acta Physica Sinica, 2013, 62(8): 084706. doi: 10.7498/aps.62.084706
[11]	Zhang Yang, Gu Shu-Lin, Ye Jian-Dong, Huang Shi-Min, Gu Ran, Chen Bin, Zhu Shun-Ming, Zhen You-Dou. Two-dimensional electron Gas in ZnMgO/ZnO heterostructures. Acta Physica Sinica, 2013, 62(15): 150202. doi: 10.7498/aps.62.150202
[12]	Sha Sha, Chen Zhi-Hua, Zhang Huan-Hao, Jiang Xiao-Hai. Numerical investigations on the Schardin's problem. Acta Physica Sinica, 2012, 61(6): 064702. doi: 10.7498/aps.61.064702
[13]	Wang Jian, Li Ying-Hong, Cheng Bang-Qin, Su Chang-Bing, Song Hui-Min, Wu Yun. The mechanism investigation on shock wave controlled by plasma aerodynamic actuation. Acta Physica Sinica, 2009, 58(8): 5513-5519. doi: 10.7498/aps.58.5513
[14]	Wu Qin-Kuan. The shock solution for a class of the nonlinear equations by the Sinc-Galerkin method. Acta Physica Sinica, 2006, 55(4): 1561-1564. doi: 10.7498/aps.55.1561
[15]	Wu Qin-Kuan. The indirect matching solution for a class of shock problems. Acta Physica Sinica, 2005, 54(6): 2510-2513. doi: 10.7498/aps.54.2510
[16]	Zhang Wei-Jia, Wang Tian-Min, Zhong Li-Zhi, Wu Xiao-Wen, Cui Min. Theoretical study of infrared emissivity of indium tin oxide films. Acta Physica Sinica, 2005, 54(9): 4439-4444. doi: 10.7498/aps.54.4439
[17]	Zhou Xiao-Feng, Tao Shu-Fen, Liu Zuo-Quan, Kan Jia-De, Li Hai-Yang. . Acta Physica Sinica, 2002, 51(2): 322-325. doi: 10.7498/aps.51.322
[18]	He Feng, Yang Jing-Long, Shen Meng-Yu. . Acta Physica Sinica, 2002, 51(9): 1918-1922. doi: 10.7498/aps.51.1918
[19]	Lü XIAO-YANG, KONG LING-JIANG, LIU MU-REN. ANALYSIS ON MACROSCOPIC EQUATION TO ONE-DIMENSIONAL RANDOM TRAFFIC FLOW MODELS. Acta Physica Sinica, 2001, 50(7): 1255-1259. doi: 10.7498/aps.50.1255
[20]	ZHANG SHU-DONG, ZHANG WEI-JUN. VELOCITY OF EMISSION PARTICLES AND SHOCKWAVE PRODUCED BY LASER-ABLATED Al TARGET. Acta Physica Sinica, 2001, 50(8): 1512-1516. doi: 10.7498/aps.50.1512

Catalog

Vol. 72, Issue 6 - 2023-03-20

Metrics

Abstract views: 3939
PDF Downloads: 70
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板