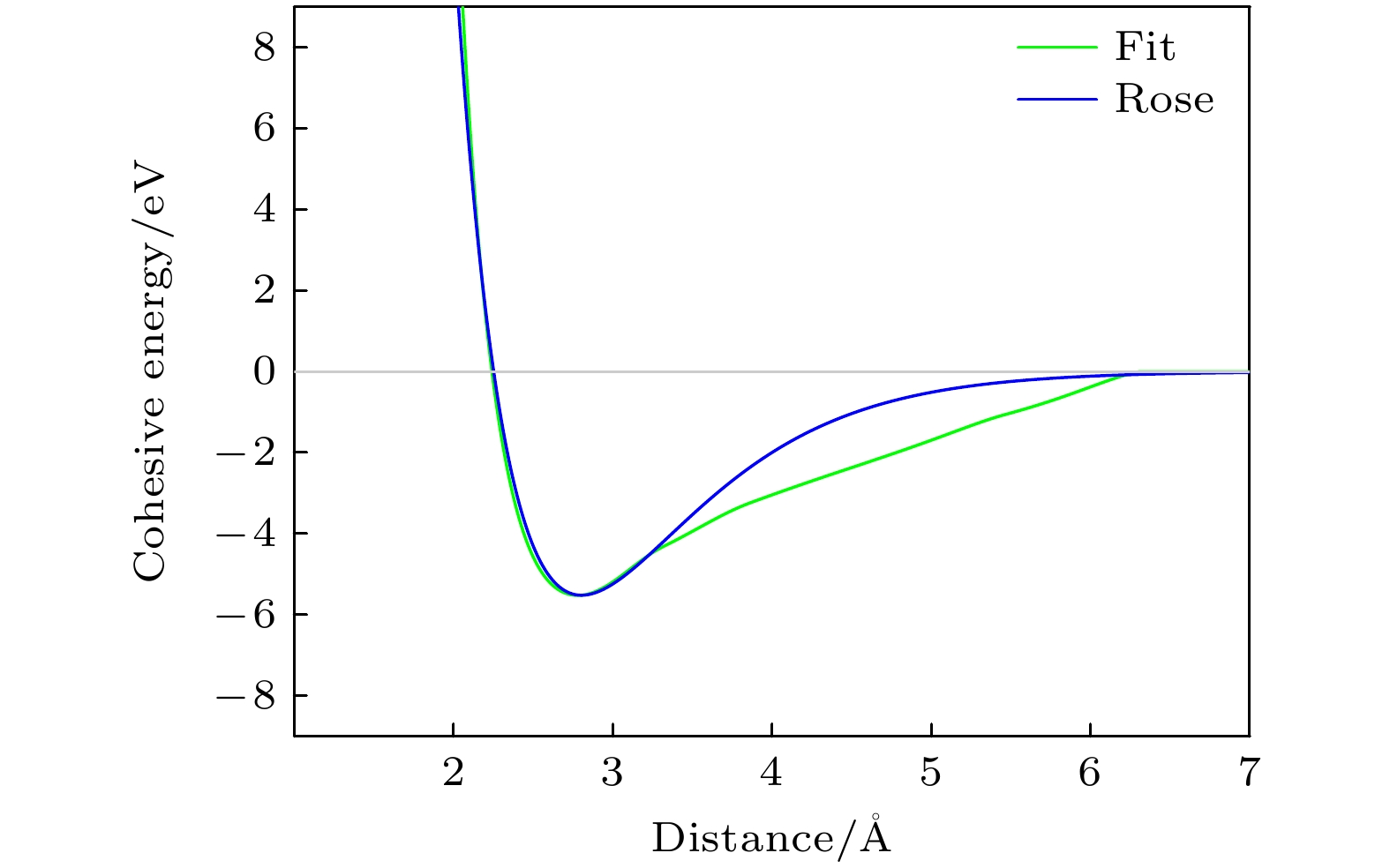
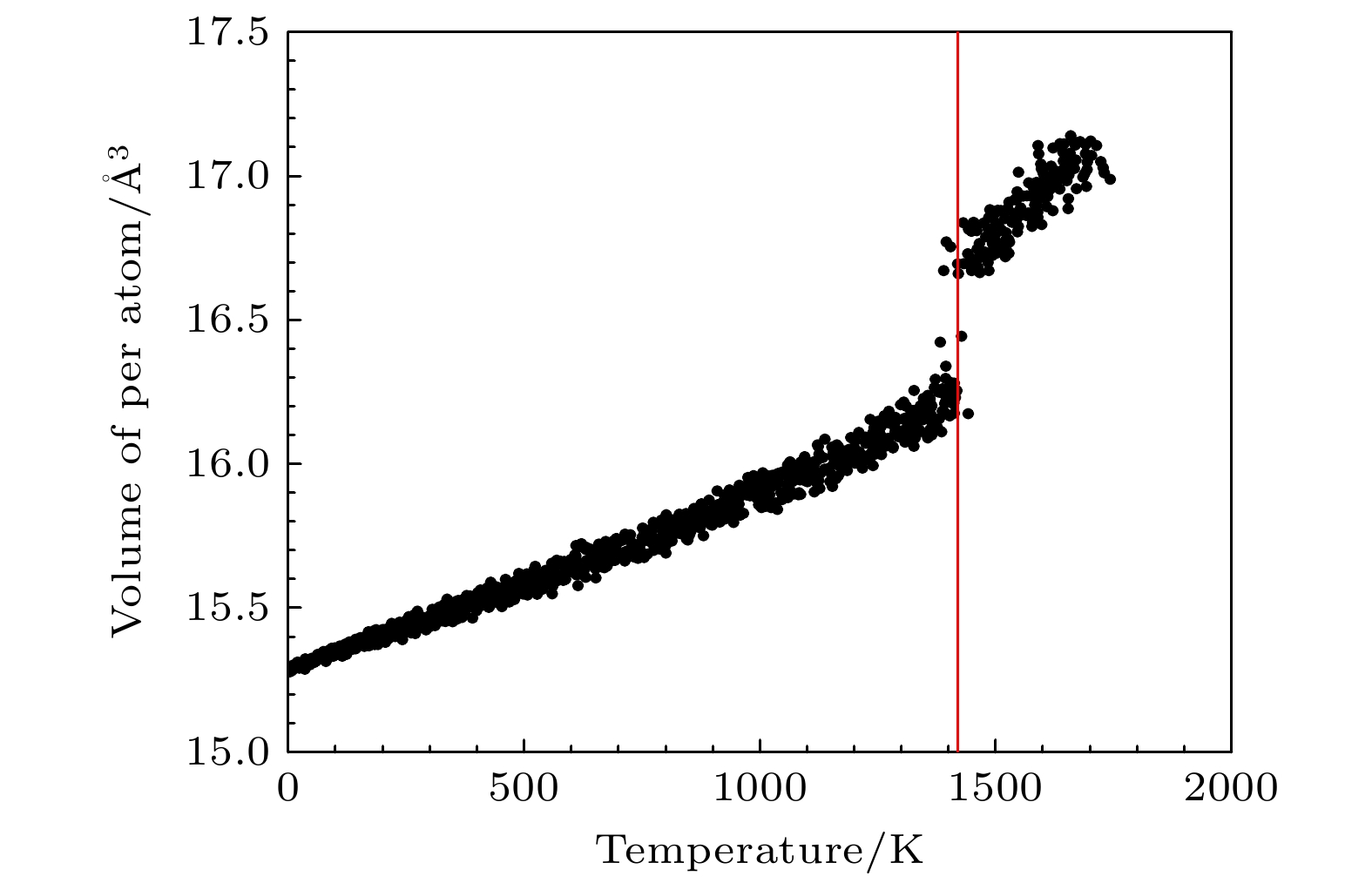
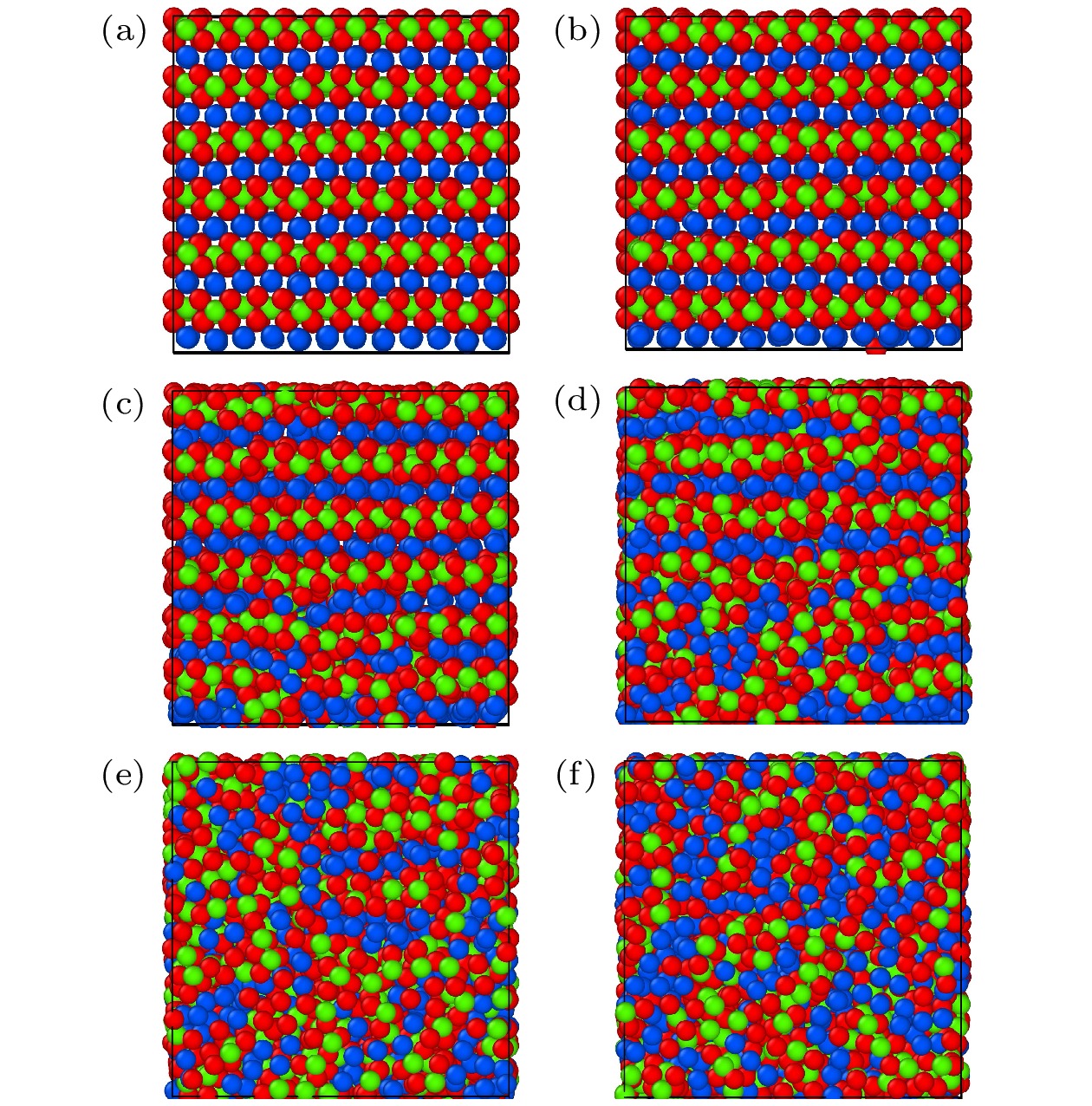
-
Molecular dynamics simulation is an effective computer simulation method. However, owing to the lack of suitable interatomic potential of multicomponent alloys, the application of molecular dynamics simulation is limited. The development of interatomic potential of multicomponent alloys has always been challenging. In this work, under the framework of EAM model, a construction method of interatomic potential suitable for ternary ordered alloys is proposed, and a new interatomic potential of Ti2AlNb alloys suitable for atomic-scale mechanical behavior simulation is developed. The potential can well reproduce the elastic constants of B2-Ti2AlNb, unrelaxation vacancy formation energy, substitutional atom formation energy, transposition atom formation energy, surface energy and cohesive energy of three ordered phase (B2, D019 and O phases ) in different volumes. To further test the potential functions, 1) the E-V curve of B2 phase is calculated, and the result is well consistent with Rose curve; 2) the melting transformation process of B2 phase is studied by molecular dynamics simulation, and the results roughly reflect the experimental fact. The present work provides a way to develop the interatomic potential of multicomponent alloys, and a option for the workers who simulate and calculate the Ti2AlNb alloys as well.
-
Keywords:
- embedded atom method potential /
- Ti2AlNb alloys /
- defects
[1] Deringer V, Csanyi G 2017 Phys. Rev. B 95 094203
Google Scholar
[2] Alizadeh Z, Mohammadizadeh M R 2019 Physica C 558 7
Google Scholar
[3] Jordan M I, Mitchell T M 2015 Science 349 255
Google Scholar
[4] Smith J S, Nebgen B, Mathew N, Chen J, Lubbers N, Burakovsky L, Tretiak S, Nam H A, Germann T, Fensin S, Barros K 2021 Nat. Commun. 12 1257
Google Scholar
[5] Artrith N, Urban A 2016 Comput. Mater. Sci. 114 135
Google Scholar
[6] Kumpfert J 2001 Adv. Eng. Mater. 3 851
Google Scholar
[7] Boehlert C J, Majumdar B S 1999 Metall. Mater. Trans. A 30 2305
Google Scholar
[8] 冯艾寒, 李渤渤, 沈军 2011 材料与冶金学报 10 30
Google Scholar
Feng A H, Li B B, Shen J 2011 J. Mater. Metall. 10 30
Google Scholar
[9] Gogia T K, Nandy T K, Banerjee D, Carisey T, Strudel J L, Franchet J M 1998 Intermetallics 6 741
Google Scholar
[10] Banerjee D, Gogia A K, Nandi T K, Joshi V A 1988 Acta Metall. 36 871
Google Scholar
[11] Pathak A, Singh A K 2015 Solid State Commun. 204 9
Google Scholar
[12] Daw M S, Baskes M I 1984 Phys. Rev. B 29 6443
Google Scholar
[13] Cheng C, Ma Y L, Bao Q L, Wang X, Sun J X, Zhou G, Wang H, Liu Y X, Xu D S 2019 Comput. Mater. Sci. 173 109432
Google Scholar
[14] Johnson R A 1989 Phys. Rev. B 39 12554
Google Scholar
[15] Voter A F 1994 Intermetallic Compounds (New York: Wiley) pp77—80
[16] Mishin Y, Mehl M J 2002 Phys. Rev. B 65 224114
Google Scholar
[17] Ravi C, Vajeeston P, Mathijaya S, Asokamani R 1999 Phys. Rev. B:Condens. Matter. 60 683
Google Scholar
[18] Kittel C 1976 Introduction to Solid State Physics (New Jersey: John Wiley & Sons) pp57–58
[19] Weast R C 1984 Handbook of Chemistry and Physics (Boca Raton: Chemical Rubber) p64
[20] Johnson R A 1972 Phys. Rev. B 6 2094
Google Scholar
[21] Oh D J, Johnson R A 2011 Mater. Res. 3 471
Google Scholar
[22] Bolef D I 1961 Appl. Phys. 32 100
Google Scholar
[23] Agrawal A, Mishra R, Ward L, Flores K M, Windl W 2013 Modell. Simul. Mater. Sci. Eng. 21 085001
Google Scholar
[24] Farkas D 1999 Modell. Simul Mater. Sci. Eng. 4 23
Google Scholar
[25] Rose J H, Smith J R, Guinea F, Ferrante J 1984 Phys. Rev. B 29 2963
Google Scholar
[26] Julius C S, Martin P 2006 J. Phase Equilib. Diffus. 27 255
Google Scholar
[27] Vasudevan V K, Yang J, Woodfield A P 1996 Scr. Mater. 35 1033
Google Scholar
-
图 1 Ti2AlNb的EAM势 (a) 电子密度分布函数; (b) 嵌入能函数; (c) 同种原子间的对势; (d) 异种原子间的对势
Figure 1. EAM potential for Ti2AlNb: (a) Electron density function; (b) embedding energy function; (c) pair potentials between same types of atoms; (d) pair potentials between different types of atoms.
图 2 B2-Ti2AlNb单空位形成能与双空位形成能
Figure 2. Mono-vacancy formation energies and di-vacancy formation energies for B2-Ti2AlNb.
图 3 B2-Ti2AlNb原子位置换位示意图
Figure 3. Atom position transposition diagram for B2-Ti2AlNb.
图 4 B2-Ti2AlNb置换原子形成能的误差
Figure 4. Deviations of substitutional atom formation energies for B2-Ti2AlNb.
图 5 B2-Ti2AlNb换位原子形成能的误差
Figure 5. Deviations of transposition atom formation energies for B2-Ti2AlNb.
图 6 B2-Ti2AlNb的E-V曲线与Rose曲线
Figure 6. E-V curve and Rose curve for B2-Ti2AlNb.
图 7 Ti2AlNb合金的E-V曲线 (a) B2相; (b) D019相; (c) O相
Figure 7. E-V curve of Ti2AlNb alloys: (a) B2 phase; (b) D019 phase; (c) O phase.
图 8 B2相的每个原子的体积随温度的变化
Figure 8. Volume variation of each atom for B2 phase as functions of temperature.
图 9 B2相随温度变化的截面快照 (a) 296 K; (b) 754 K; (c) 1428 K; (d) 1390 K; (e) 1449 K; (f) 1728 K
Figure 9. Cross-section snapshots for
B2 phase as functions of temperature: (a) 296 K; (b) 754 K; (c) 1428 K; (d) 1390 K; (e) 1449 K; (f) 1728 K. 表 1 B2-Ti2AlNb的晶格常数和生成焓(单位: eV)
Table 1. Lattice constants and formation enthalpy for B2-Ti2AlNb (in eV).
DownLoad: CSV
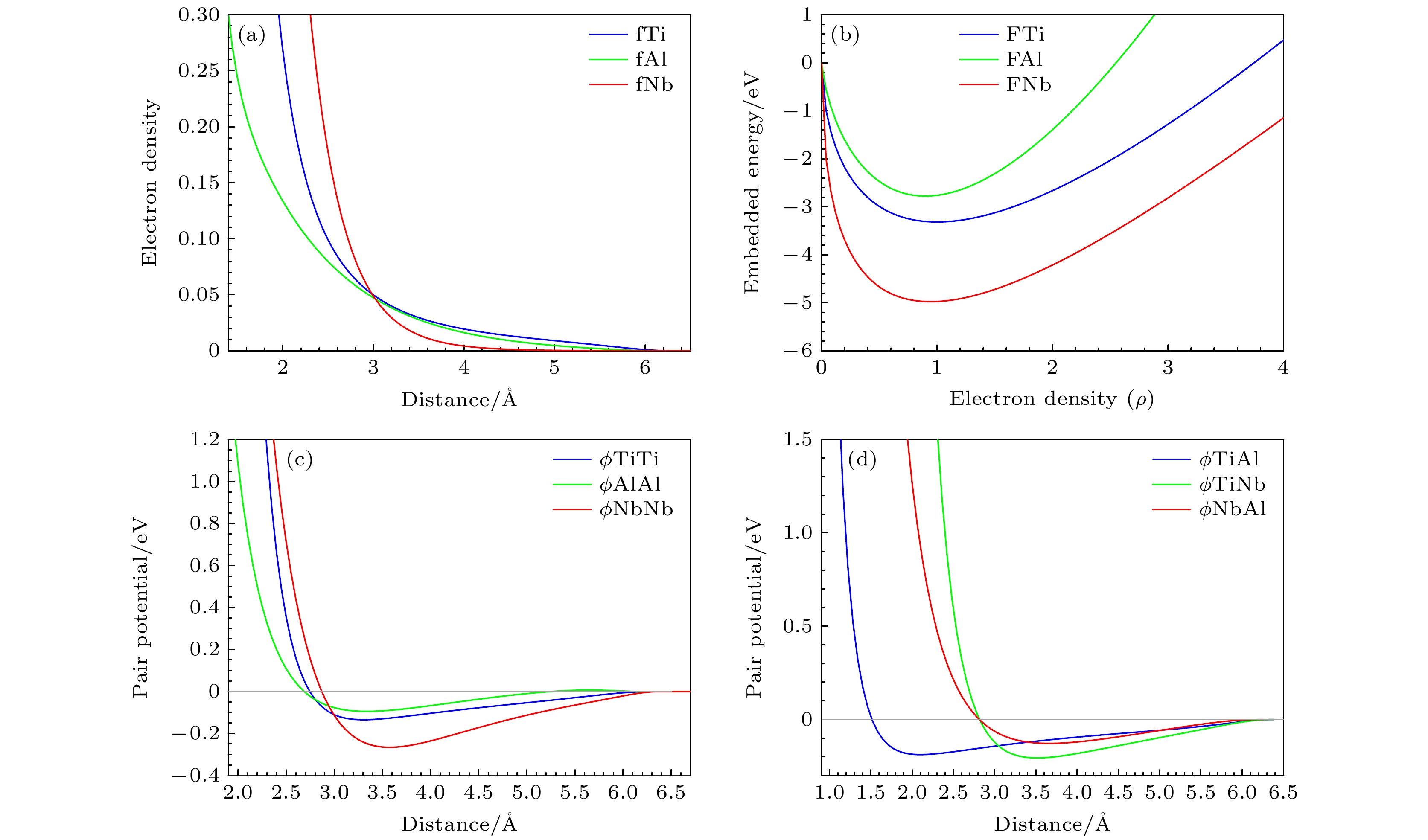
表 3 Ti2AlNb合金EAM势的拟合参数
Table 3. Fitting parameters of EAM potential for Ti2AlNb alloys.
$ \phi \left({r}_{\mathrm{T}\mathrm{i}\mathrm{A}\mathrm{l}}\right) $ $ \phi \left({r}_{\mathrm{T}\mathrm{i}\mathrm{N}\mathrm{b}}\right) $ $ \phi \left({r}_{\mathrm{N}\mathrm{b}\mathrm{A}\mathrm{l}}\right) $ Parameter value Parameter value Parameter value $ \alpha $ –2.1129 $ \alpha $ –4.2151 $ \alpha $ –0.2797 $ \beta $ –1.3742 $ \beta $ –6.6808 $ \beta $ –2.5122 $ \gamma $ 0.2705 $ \gamma $ 1.4339 $ \gamma $ 1.6140 $ \epsilon $ 0.1758 $ \epsilon $ –0.0008 $ \epsilon $ 0.1140 $ \sigma $ –2.3191 $ \sigma $ –1.4062 $ \sigma $ –1.6132 $ \mu $ 0.0516 $ \mu $ 0.2694 $ \mu $ 0.4012
DownLoad: CSV
表 4 B2-Ti2AlNb的弹性常数(单位: GPa)
Table 4. Elastic constants for B2-Ti2AlNb (in GPa).
C11 C33 C44 C66 C12 C13 ABINIT[11] 136 — 81 — 100 — DFT 153.20 147.83 69.36 71.86 133.42 97.62 This work 195.82 178.95 64.35 66.83 136.92 127.04
DownLoad: CSV
表 5 B2-Ti2AlNb单空位形成能和双空位形成能(单位: eV)
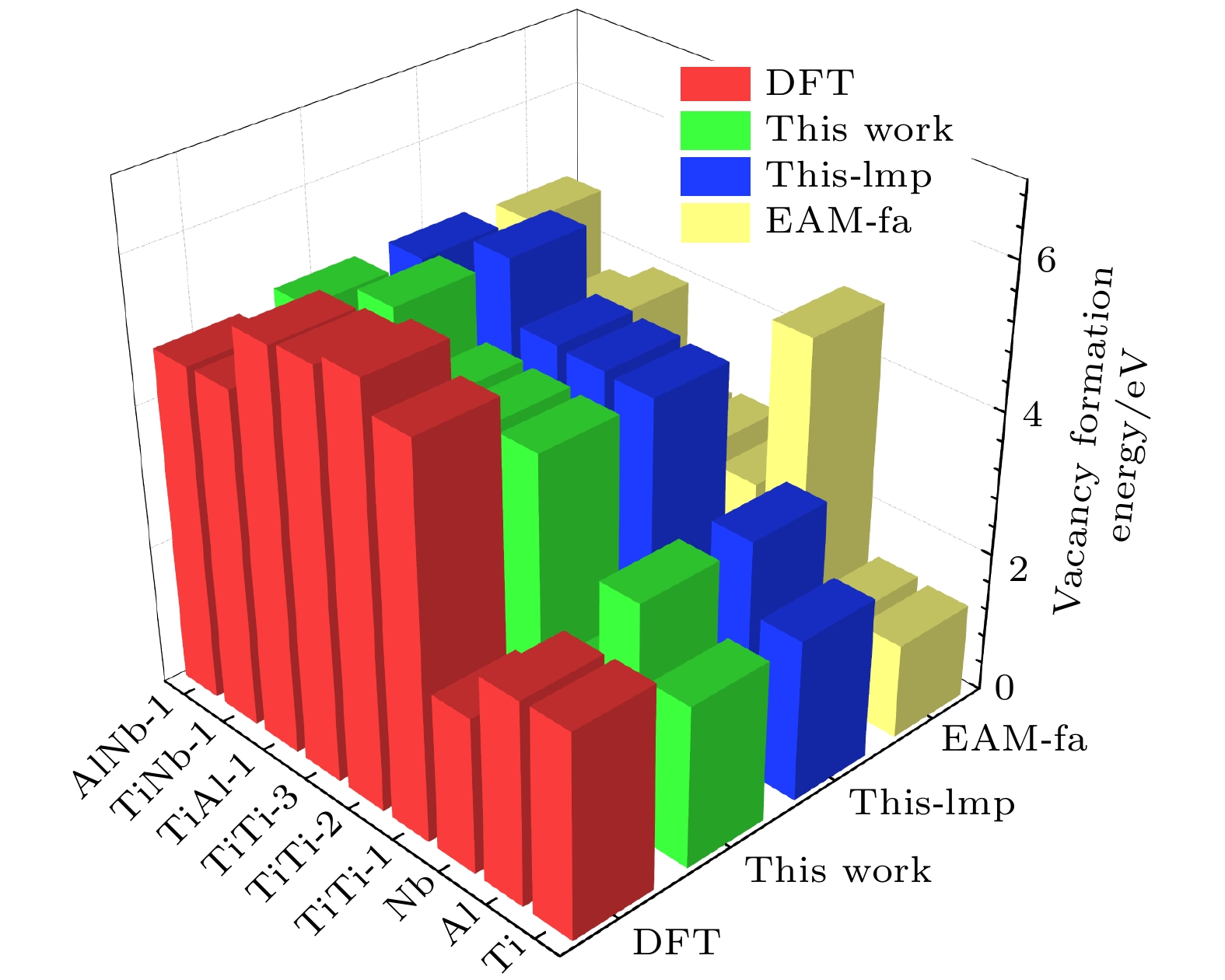
Table 5. Mono-vacancy formation energies and di-vacancy formation energies for B2-Ti2AlNb (in eV).
DFT This work This-lmp EAM-fa $ {E}_{\mathrm{v}}^{\mathrm{f}} $ Ti 2.879 2.283 2.283 1.343 Al 2.859 3.265 3.265 1.391 Nb 2.194 1.763 1.657 4.919 $ {E}_{\mathrm{d}}^{\mathrm{f}} $ TiTi-1 5.454 4.503 4.498 2.562 TiTi-2 5.854 4.539 4.533 2.777 TiTi-3 5.688 4.570 4.567 2.724 TiAl-1 5.623 5.434 5.434 4.014 TiNb-1 4.731 3.980 3.980 3.699 AlNb-1 4.710 4.854 4.854 4.777
DownLoad: CSV
表 6 B2-Ti2AlNb置换原子和换位原子形成能(单位: eV)
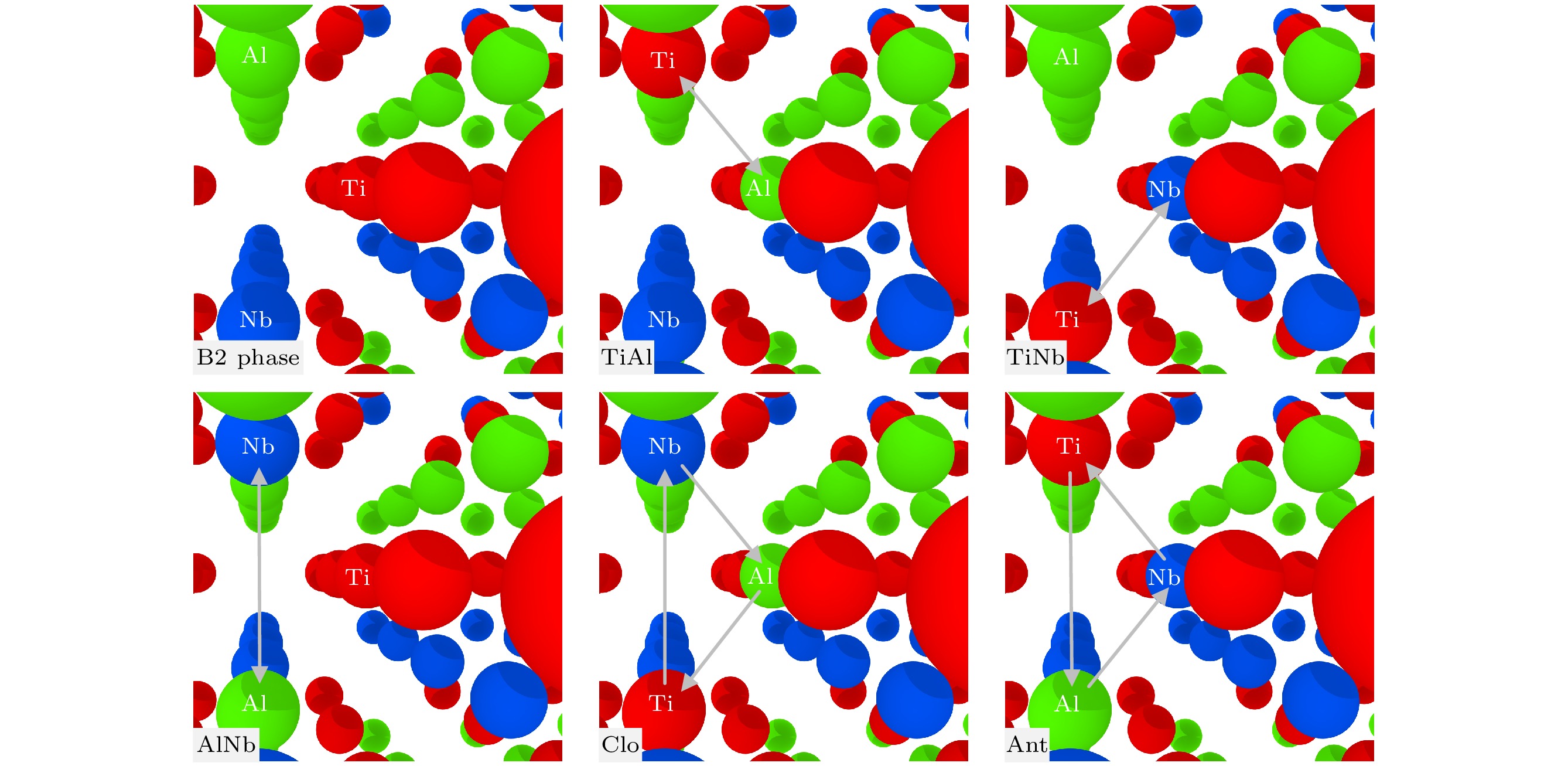
Table 6. Substitutional atom and transposition atom formation energies for B2-Ti2AlNb (in eV).
DFT This work This-lmp EAM-fa $ {E}_{\mathrm{o}}^{\mathrm{f}} $ $ {\mathrm{T}\mathrm{i}}_{\mathrm{A}\mathrm{l}} $ 1.046 0.976 0.976 –2.863 $ {\mathrm{T}\mathrm{i}}_{\mathrm{N}\mathrm{b}} $ –0.384 –0.418 –0.418 –2.440 $ {\mathrm{A}\mathrm{l}}_{\mathrm{T}\mathrm{i}} $ 0.268 0.433 0.433 –1.164 $ {\mathrm{A}\mathrm{l}}_{\mathrm{N}\mathrm{b}} $ –1.310 –1.216 –1.216 –1.113 $ {\mathrm{N}\mathrm{b}}_{\mathrm{T}\mathrm{i}} $ 0.638 0.624 0.624 –7.439 $ {\mathrm{N}\mathrm{b}}_{\mathrm{A}\mathrm{l}} $ 1.522 1.289 1.289 0.911 $ {E}_{\mathrm{e}}^{\mathrm{f}} $ TiAl 1.097 1.181 1.181 –4.142 TiNb 0.270 0.191 0.191 –9.213 AlNb 0.123 0.172 0.172 –0.571 Clo 1.078 1.132 1.132 –3.169 Ant 0.453 0.413 0.413 –10.752
DownLoad: CSV
表 7 B2-Ti2AlNb的表面能(单位: eV)
Table 7. Surface energies for
B2-Ti2AlNb (in eV). DFT This work This-lmp EAM-fa $ {E}_{\mathrm{s}\mathrm{u}\mathrm{r}}^{\mathrm{f}} $ (100) 2.056 2.298 2.273 1.705 (110) 1.937 2.194 2.170 1.620 (100)′ 1.844 — 1.923 0.484 (110)′ 1.661 — 1.750 –11.000
DownLoad: CSV
表 8 Ti2AlNb合金的内聚能(单位: eV)
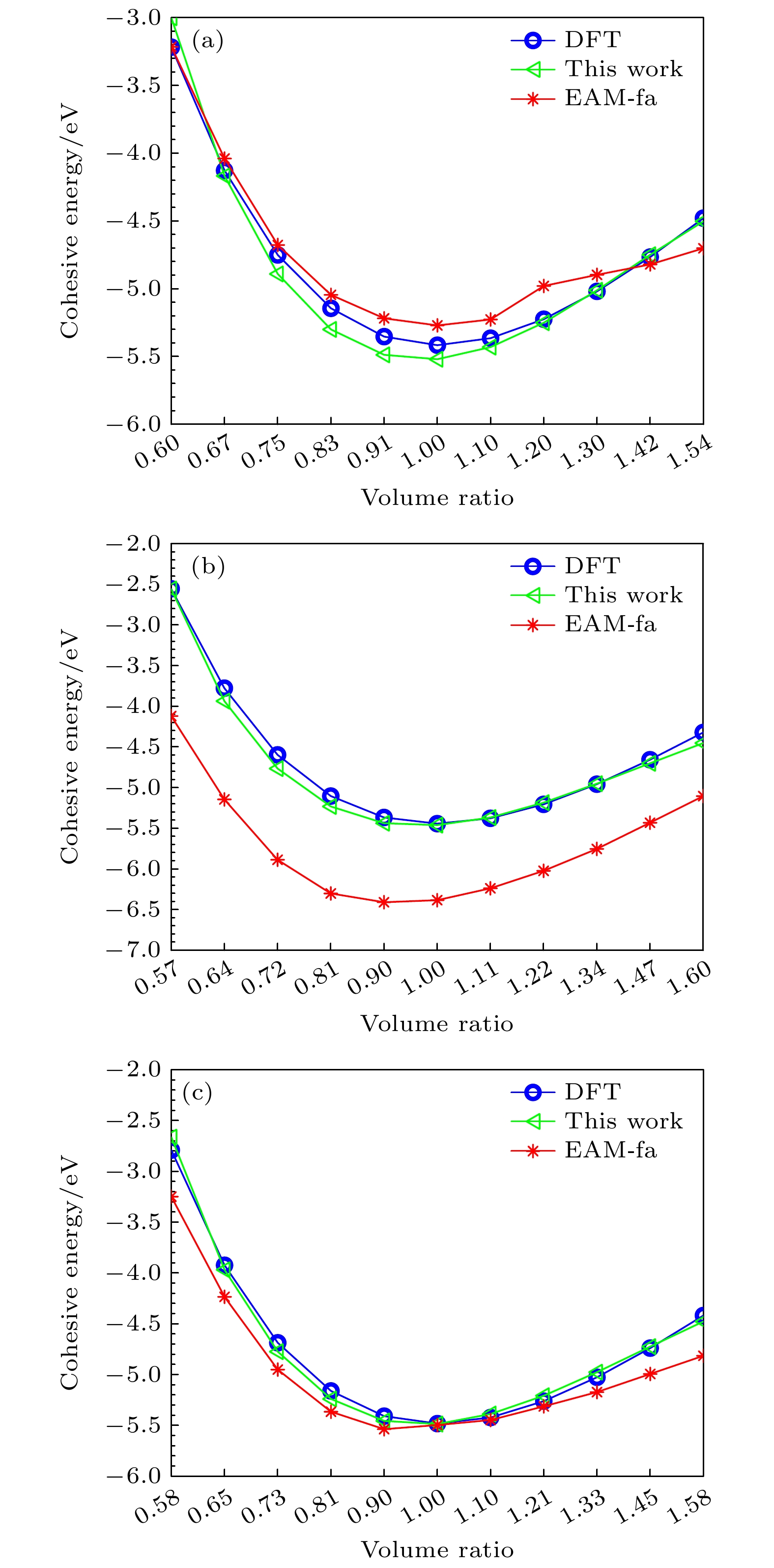
Table 8. Cohesive energies for Ti2AlNb alloys (in eV).
VB2 60% 67% 75% 83% 91% 1 110% 120% 130% 142% 154% E-DFT –3.22 –4.13 –4.75 –5.14 –5.35 –5.42 –5.37 –5.22 –5.02 –4.77 –4.48 $ V_{{\rm D0}_{19}} $ 57% 64% 72% 81% 90% 1 111% 122% 134% 147% 160% E-DFT –2.55 –3.78 –4.60 –5.10 –5.37 –5.44 –5.38 –5.21 –4.96 –4.66 –4.32 VO 58% 65% 73% 81% 90% 1 110% 121% 133% 145% 158% E-DFT –2.79 –3.92 –4.69 –5.16 –5.41 –5.48 –5.42 –5.26 –5.03 –4.74 –4.42
DownLoad: CSV
-
[1] Deringer V, Csanyi G 2017 Phys. Rev. B 95 094203
Google Scholar
[2] Alizadeh Z, Mohammadizadeh M R 2019 Physica C 558 7
Google Scholar
[3] Jordan M I, Mitchell T M 2015 Science 349 255
Google Scholar
[4] Smith J S, Nebgen B, Mathew N, Chen J, Lubbers N, Burakovsky L, Tretiak S, Nam H A, Germann T, Fensin S, Barros K 2021 Nat. Commun. 12 1257
Google Scholar
[5] Artrith N, Urban A 2016 Comput. Mater. Sci. 114 135
Google Scholar
[6] Kumpfert J 2001 Adv. Eng. Mater. 3 851
Google Scholar
[7] Boehlert C J, Majumdar B S 1999 Metall. Mater. Trans. A 30 2305
Google Scholar
[8] 冯艾寒, 李渤渤, 沈军 2011 材料与冶金学报 10 30
Google Scholar
Feng A H, Li B B, Shen J 2011 J. Mater. Metall. 10 30
Google Scholar
[9] Gogia T K, Nandy T K, Banerjee D, Carisey T, Strudel J L, Franchet J M 1998 Intermetallics 6 741
Google Scholar
[10] Banerjee D, Gogia A K, Nandi T K, Joshi V A 1988 Acta Metall. 36 871
Google Scholar
[11] Pathak A, Singh A K 2015 Solid State Commun. 204 9
Google Scholar
[12] Daw M S, Baskes M I 1984 Phys. Rev. B 29 6443
Google Scholar
[13] Cheng C, Ma Y L, Bao Q L, Wang X, Sun J X, Zhou G, Wang H, Liu Y X, Xu D S 2019 Comput. Mater. Sci. 173 109432
Google Scholar
[14] Johnson R A 1989 Phys. Rev. B 39 12554
Google Scholar
[15] Voter A F 1994 Intermetallic Compounds (New York: Wiley) pp77—80
[16] Mishin Y, Mehl M J 2002 Phys. Rev. B 65 224114
Google Scholar
[17] Ravi C, Vajeeston P, Mathijaya S, Asokamani R 1999 Phys. Rev. B:Condens. Matter. 60 683
Google Scholar
[18] Kittel C 1976 Introduction to Solid State Physics (New Jersey: John Wiley & Sons) pp57–58
[19] Weast R C 1984 Handbook of Chemistry and Physics (Boca Raton: Chemical Rubber) p64
[20] Johnson R A 1972 Phys. Rev. B 6 2094
Google Scholar
[21] Oh D J, Johnson R A 2011 Mater. Res. 3 471
Google Scholar
[22] Bolef D I 1961 Appl. Phys. 32 100
Google Scholar
[23] Agrawal A, Mishra R, Ward L, Flores K M, Windl W 2013 Modell. Simul. Mater. Sci. Eng. 21 085001
Google Scholar
[24] Farkas D 1999 Modell. Simul Mater. Sci. Eng. 4 23
Google Scholar
[25] Rose J H, Smith J R, Guinea F, Ferrante J 1984 Phys. Rev. B 29 2963
Google Scholar
[26] Julius C S, Martin P 2006 J. Phase Equilib. Diffus. 27 255
Google Scholar
[27] Vasudevan V K, Yang J, Woodfield A P 1996 Scr. Mater. 35 1033
Google Scholar
-
[1] Wang Yue, Shao Bo-Huai, Chen Shuang-Long, Wang Chun-Jie, Gao Chun-Xiao. Effects of defects on electrical transport properties of anatase TiO2 polycrystalline under high pressure: AC impedance measurement. Acta Physica Sinica, 2023, 72(12): 126401. doi: 10.7498/aps.72.20230020 [2] Li Ya-Sha, Liu Shi-Chong, Liu Qing-Dong, Xia Yu, Hu Huo-Ran, Li Guang-Zhu. Electrical properties of ZnO/ ${\boldsymbol{\beta}} $ -Bi2O3 interfaces featuring aggregation defect under external electric fields. Acta Physica Sinica, 2022, 71(2): 026801. doi: 10.7498/aps.71.20210635[3] Cao Zhen, Hao Da-Peng, Tang Gang, Xun Zhi-Peng, Xia Hui. Influence of cluster shaped defects on fracture process of fiber bundle. Acta Physica Sinica, 2021, 70(20): 204602. doi: 10.7498/aps.70.20210310 [4] . Research on electrical properties of ZnO/β-Bi2O3 interfaces featuring aggregation defect within external electric fields. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20210635 [5] Liu Hao-Hua, Wang Shao-Hua, Li Bo-Bo, Li Hua-Lin. Defect induced asymmetric soliton transmission in the nonlinear circuit. Acta Physica Sinica, 2017, 66(10): 100502. doi: 10.7498/aps.66.100502 [6] Zhang Xiu-Zhi, Wang Kai-Yue, Li Zhi-Hong, Zhu Yu-Mei, Tian Yu-Ming, Chai Yue-Sheng. Effect of nitrogen on the defect luminescence in diamond. Acta Physica Sinica, 2015, 64(24): 247802. doi: 10.7498/aps.64.247802 [7] Zhang Ming-Lan, Yang Rui-Xia, Li Zhuo-Xin, Cao Xing-Zhong, Wang Bao-Yi, Wang Xiao-Hui. Study on proton irradiation induced defects in GaN thick film. Acta Physica Sinica, 2013, 62(11): 117103. doi: 10.7498/aps.62.117103 [8] Zhang Pei-Jian, Meng Yang, Liu Zi-Yu, Pan Xin-Yu, Liang Xue-Jin, Chen Dong-Min, Zhao Hong-Wu. Influences of dislocation distribution on the resistive switching effect of Ag-SiO2 thin films. Acta Physica Sinica, 2012, 61(10): 107703. doi: 10.7498/aps.61.107703 [9] Wang Xin-Hua, Pang Lei, Chen Xiao-Juan, Yuan Ting-Ting, Luo Wei-Jun, Zheng Ying-Kui, Wei Ke, Liu Xin-Yu. Investigation on trap by the gate fringecapacitance in GaN HEMT. Acta Physica Sinica, 2011, 60(9): 097101. doi: 10.7498/aps.60.097101 [10] Lu Guang-Xia, Zhang Hui, Zhang Guo-Ying, Liang Ting, Li Dan, Zhu Sheng-Long. Mechanism of the influence of the interaction between interstitial H atom and doped atom on the dehydrogenation performance of LiNH2. Acta Physica Sinica, 2011, 60(11): 117101. doi: 10.7498/aps.60.117101 [11] Lin Min-Dong, Zhu Juan-Juan, Wang Wei, Zhou Bang-Xin, Liu Wen-Qing, Xu Gang. Precipitation of Cu-rich clusters in reactor pressure vessel model steels and embedded-atom method potentials. Acta Physica Sinica, 2010, 59(2): 1163-1168. doi: 10.7498/aps.59.1163 [12] Li Sheng-Tao, Cheng Peng-Fei, Li Jian-Ying. Thermal stimulated current in sandwiched Al2O3 single crystal samples. Acta Physica Sinica, 2008, 57(12): 7783-7788. doi: 10.7498/aps.57.7783 [13] Xin Hao, Han Qiang, Yao Xiao-Hu. Influences of atom vacancies on buckling properties of armchair single-walled carbon nanotubes shown by molecular dynamics simulation. Acta Physica Sinica, 2008, 57(7): 4391-4396. doi: 10.7498/aps.57.4391 [14] Xia Zhi-Lin, Shao Jian-Da, Fan Zheng-Xiu. Effect of bulk inclusion in films on damage probability. Acta Physica Sinica, 2007, 56(1): 400-406. doi: 10.7498/aps.56.400 [15] Hao Xiao-Peng, Wang Bao-Yi, Yu Run-Sheng, Wei Long. Zirconium-ion implantation of zircaloy-4 investiged by slow positron beam. Acta Physica Sinica, 2007, 56(11): 6543-6546. doi: 10.7498/aps.56.6543 [16] Jin Nian-Qing, Teng Yu-Yong, Gu Bin, Zeng Xiang-Hua. Study of rare-gas atom injection into defective carbon nanotube by molecular dynamics simulation. Acta Physica Sinica, 2007, 56(3): 1494-1498. doi: 10.7498/aps.56.1494 [17] Yu Song, Wang Chong-Yu, Yu Tao. Study of the point defect, Re site, and Re cluster in Re embedded Ni3Al using the embedded atom method. Acta Physica Sinica, 2007, 56(6): 3212-3218. doi: 10.7498/aps.56.3212 [18] Li Peng-Fei, Yan Xiao-Hong, Wang Ru-Zhi. . Acta Physica Sinica, 2002, 51(9): 2139-2143. doi: 10.7498/aps.51.2139 [19] PAN BI-CAI. TIGHT-BINDING POTENTIAL WITH CORRECTION OF BONDING ENVIRONMENT FOR SILICON-HYDROGEN. Acta Physica Sinica, 2001, 50(2): 268-272. doi: 10.7498/aps.50.268 [20] TANG XUE-FENG, GU MU, TONG HONG-YONG, LIANG LING, YAO MING-ZHEN, CHEN LING-YAN, LIAO JING-YING, SHEN BIN-FU, QU XIANG-DONG, YIN ZHI-WEN, XU WEI-XIN, WANG JING-C HENG. A STUDY ON La-DOPED PbWO4 SCINTILLATING CRYSTAL. Acta Physica Sinica, 2000, 49(10): 2007-2010. doi: 10.7498/aps.49.2007
DownLoad:
Catalog
Metrics
- Abstract views: 4268
- PDF Downloads: 86
- Cited By: 0
